« Prev Next »
History and Definition
Allee effects occur in small or sparse populations and, although rarely detected, are widely believed to be common in nature. Population growth of populations subject to Allee effects is reduced at low density. The originator and namesake of the phenomenon was Warder Clyde Allee (1885–1955), a University of Chicago zoologist and animal ecologist, whose special interest was group behavior in animals.
Charles Darwin observed that large population size is an important hedge against extinction in the presence of predators or other natural enemies (Darwin 1872, quoted in Stephens et al. 1999). Nevertheless, early twentieth century biologists typically accepted as given both the Malthusian principle — that intra-specific competition does not decrease with population size — and its mathematical expression in the logistic model for population growth (Gause 1934).
Allee, an astute observer of animal behavior, noticed that in many species it was undercrowding, not competition, that limited population growth. He observed, for instance, that aggregation had positive effects on the survival of land isopods, which were subject to rapid desiccation when isolated (Allee 1927). His empirical examples of the benefits of aggregation seemed to contradict both the Malthusian paradigm and the logistic model. Much of Allee's early work focused on documenting this phenomenon in animal populations (Allee 1931).
In modern biology, Allee effects are considered to have two manifestations:
- component Allee effects are exhibited by a population in which there is a positive association between some fitness component (e.g., viability, juvenile survivorship, fecundity; Orr 2009) and population size;
- demographic Allee effects that occur when these component Allee effects produce a positive association between per capita population growth and population size.
Keeping in mind that competition, for instance for resources or space, will limit a population's size, and noting that per capita growth rate of a population at small size equals average absolute individual fitness (Lande et al. 2003), both kinds of Allee effects may be captured by the following general definition.
An Allee effect is a positive association between absolute average individual fitness and population size over some finite interval.
Such a positive association may (but does not necessarily) give rise to a critical population size below which the population cannot persist (Stephens et al. 1999). Allee effects that cause critical population sizes are called strong, while Allee effects that do not result in critical sizes are called weak. Other names for the Allee effect are positive density dependence (in contrast to classical negative density dependence) and depensatory dynamics (in contrast to classical compensatory dynamics).
Mechanisms That Cause Allee Effects
A positive relationship between fitness and population size can be caused by a variety of mechanisms that affect reproduction and survival. A well established example, mate limitation, may result in undercrowding in species that reproduce sexually, because sexual reproduction requires contact between male and female gametes. Mate limitation reduces reproduction when plants or animals release gametes into the environment or when males and females have difficulty locating each other. When behaviors such as breeding, feeding, and defense are cooperative, they become more efficient or successful in larger social groups, resulting in increased reproductive success or survivorship (Courchamp et al. 1999). Although cooperative behaviors are most obvious in social vertebrates, such as prairie dogs, ungulates or birds, Allee effects resulting from group feeding or defense can also arise in insects, such as bark beetles, and aquatic organisms, such as cichlid fish (Friedenberg et al. 2007, Balshine et al. 2001).
Other mechanisms do not require cooperation in the behavioral sense, but merely the presence of conspecific individuals. For example, the per capita risk of predation is smaller in large prey populations than small prey populations (Dennis 1989, Gascoigne and Lipcius 2004). It is also known that the presence of multiple individuals can alter environmental or biotic conditions in favorable ways. Examples of such niche construction include reducing physical damage in intertidal zones (Bertness and Grosholz 1985) or exclusion of competitors via allelopathy (Cappuccino 2004).
Finally, demographic and genetic mechanisms may give rise to Allee effects. In animals, active dispersal away from low-density populations can result in decreased rates of population growth (Bonte et al. 2004). For many organisms, when population size is small, inbreeding depression can cause an Allee effect by reducing average fitness as population size declines (Fischer et al. 2003). While these phenomena differ in form, in the way they affect fitness, and in which species are affected, they all result in the same general pattern: small populations suffer from reduced average individual fitness.
Evidence of Allee Effects
Evidence for Component Allee Effects
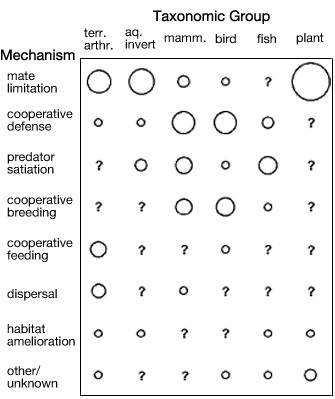
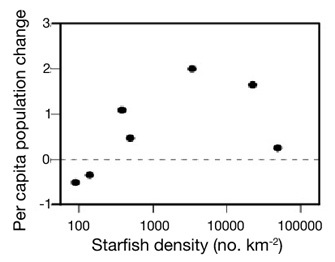
There is evidence from natural populations for component Allee effects due to all of these mechanisms (see references above, Courchamp et al 2008, Kramer et al. 2009; Figure 1). The most commonly observed mechanism is mate limitation, which causes Allee effects in both animals and plants (in the form of pollen limitation). Positive density dependence in survivorship due to either cooperative defense or predator satiation is also found across taxonomic groups. Indeed, because the tendency of predators to become satiated and stop increasing consumption depends on the predator rather than the prey, predator satiation has the potential to affect any population fed upon by a predator that does not numerically track the abundance of the small prey population, such as when a generalist predator feeds on multiple species (Kramer & Drake 2010). Evidence for the other mechanisms described above is less abundant, but each has been found in multiple taxonomic groups, and some studies have detected positive density dependence in reproduction or survival without identifying the mechanism (Kramer et al. 2009), making it likely that there are other mechanisms that lead to component Allee effects. The population level impacts of these mechanisms are less clear.
Evidence for Demographic Allee Effects
Demographic Allee effects have been harder to demonstrate. Two reasons for this include:
- Allee effects in one component of fitness may be offset at low density by increases in other components of fitness, such as decreased competition for resources;
- Natural populations at low density are often difficult to detect and the high variance that results from small sample sizes obscures statistical analysis.
For these reasons, unusual cases of population growth are important to the ongoing study of Allee effects. In particular, monitoring data from invasive species provides an opportunity for improving understanding of Allee effects. Some likely effects of positive density dependence are easier to observe, extinction, for instance, but it is difficult to confirm that an Allee effect was to blame.
Dynamics of Populations with Allee Effects
The most dramatic consequences of Allee effects are associated with strong Allee effects, although weak Allee effects are also predicted to give rise to measurable dynamical differences. The difference between Allee effects and the classical (Malthusian) logistic theory is seen most clearly in the correlation between per capita growth rate and population size. This correlation can be generated using :
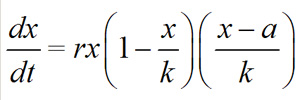
where x is the population size, a is the critical point, and k represents the force of competition and is known as the carrying capacity (Figure 2a).
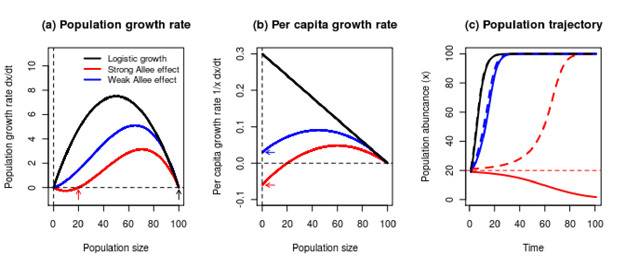
Dividing both sides by the population size (x) yields the per capita population growth rate:
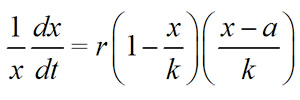
Other dynamical consequences of strong Allee effects include:
- A sigmoidal relationship between the probability of rapid extinction and the initial population size (Dennis 1989), a pattern which contrasts with the concave shape predicted by the classical logistic theory (Dennis 2002) (Figure 4a);
- A critical area that the population must occupy for it to persist (Lewis & Kareiva 1993, Vercken et al. 2011) (Figure 4b);
- A bimodal stationary distribution of population size (Dennis 1989, Drake & Lodge 2006) (Figure 4c).

Evolution
The negative effects of low density, such as difficulty finding mates or increased vulnerability to predators, is expected to often result in strong selection for traits that reduce the influence of these mechanisms. For instance, rare species, which are typically at low density, often have adaptations that allow positive growth at low density, or they will become extinct. However, aggregation and sociality are not adaptations that eliminate Allee effects per se. Rather, they increase population densities so that the densities at which Allee effects are manifest are avoided (Stephens and Sutherland 1999). Other traits may be the result of selection on low-density populations. For example, displays, calls, and pheromones all widen the area over which males and females perceive mates, thus reducing mate limitation. These adaptations can also increase fitness in other ways, however, such as signaling mate quality, so it is difficult to assign causality (Courchamp et al. 2008). Similarly, sperm storage, hermaphroditism, and parthenogenesis are all favored when chances of encountering mates are low, but may provide fitness benefits in other contexts as well, such as accelerated local adaptation (Cousyn et al. 2001). It is reasonable to conclude that species most likely to suffer Allee effects are those that usually have large populations but have suffered a recent reduction in size, such as due to habitat fragmentation or catastrophic natural events. Such populations will be more likely to suffer from mechanisms that reduce fitness at low density, especially if traits conferring fitness at low density incur a cost at high density. Finding such a trade-off may provide the best indication that a trait is an evolutionary response to persistence at low density (Courchamp et al. 2008).
Environmental applications
When the size of populations subject to strong Allee effects is low, then these populations tend towards extinction. This fact argues for a thorough understanding of Allee effects and their mechanisms in order to develop sound management practices for a number of environmental issues. An obvious case is the conservation of rare species. It has been shown, for instance, that small patches of the flowering herb Clarkia concinna concinna attract pollinating insects in fewer numbers than do large patches. As a result, small patches are more prone to extinction (Groom 1998). In general, persistence of species subject to strong Allee effects requires that a minimum population size — specific to each population — be distributed over a population-specific minimal area. This conclusion also applies to efforts to restore extirpated populations through captive breeding and reintroduction.
Another environmental issue that involves the Allee effect is the management of invasive species. Population biologists have long wondered why only a small fraction of introduced species ultimately persist in their introduced locations (Williamson & Fitter 1996). A partial answer to this question is that many introductions are below the critical size associated with Allee effects (Grevstad 1999). It follows that risk management for invasive species could be improved by a better understanding of the comparative biology of Allee effects (i.e., the relative frequency and strength of Allee effects across species) (Drake et al. 2004), coupled with a quantitative understanding of the rates at which introductions occur, a concept referred to as propagule pressure (Leung et al. 2004, Lockwood et al. 2005).
Even populations with weak Allee effects may require consideration of Allee effects for effective management. For instance, the invasive smooth cordgrass, Spartina alterniflora, is known to exhibit Allee effects due to pollination limitation. As a result, new patches of cordgrass initially grow quite slowly. An observer of such an invasion might wrongly conclude that this species' potential for spread is low. However, once the incipient colony grows to a certain size its potential to spread increases dramatically (Taylor 2004).
Conclusion
Allee effects are a small population phenomenon in which population growth rate is reduced by undercrowding. Although Allee effects are widely believed to be common and are important to environmental management, clear documentation of demographic Allee effects in nature has mostly proved elusive.
References and Recommended Reading
Allee, W. C. Animal aggregations. The Quarterly Review of Biology 2, 367–398 (1927).
———. Animal Aggregations. A Study in General Sociology. Chicago, IL: University of Chicago Press, 1931.
Balshine, S. et al. Correlates of group size in a cooperatively breeding cichlid fish (Neolamprologus pulcher). Behavioral Ecology and Sociobiology 50 134–140 (2001).
Bertness, M. D. & Grosholz, E. Population dynamics of the ribbed mussel, Geukensia demissa: The costs and benefits of an aggregated distribution. Oecologia 62, 192–204 (1985).
Bonte, D., Lens, L., & Maelfait, J. Lack of homeward orientation and increased mobility result in high emigration rates from low-quality fragments in a dune wolf spider. Journal of Animal Ecology 73, 643–650 (2004).
Cappucino, N. Allee effect in an invasive alien plant, pale swallow-wort Vicentoxicum rossicum (Asclepiadaceae). Oikos 106, 3–8 (2004).
Courchamp, F., Berec,
L., & Gascoigne, J. Allee Effects in Ecology and Conservation.
Oxford, UK: Oxford University Press, 2008.
Courchamp, F., Clutton-Brock, T., & Grenfell, B. Inverse density dependence and the Allee effect. Trends in Ecology and Evolution 14, 405–410 (1999).
Cousyn, C. et al. Rapid, local adaptation of zooplankton behavior to changes in predation pressure in the absence of neutral genetic changes. Proceedings of the National Academy of Sciences of the USA 98, 6256–6260 (2001).
Dennis, B. Allee effects: Population growth, critical density, and the chance of extinction. Natural Resource Modeling 3, 481–538 (1989).
———. Allee effects in stochastic populations. Oikos 96, 389–401 (2002).
Davis, H. G. et al. Pollen limitation causes an Allee effect in a wind pollinated invasive grass (Spartina alterniflora). Proceedings of the National Academy of Sciences of the USA 101, 13804–13807 (2004).
Drake, J. M. & Lodge, D. M. Allee effects, propagule pressure and the probability of establishment: Risk analysis for biological invasions. Biological Invasions 8, 365–375 (2006).
Dulvy, N. K., Freckleton, R. P., & Polunin, V. N. V. C. Coral reef cascades and the indirect effects of predator removal by exploitation. Ecology Letters 7, 410–416 (2004).
Fischer, M., Hock, M., & Paschke, M. Low genetic variation reduces cross-compatibility and offspring fitness in populations of a narrow endemic plant with a self-incompatibility system. Conservation Genetics 4, 325–336 (2008).
Friedenberg, N. A., Powell, J. A., & Ayres, M. P. Synchrony's double edge: Transient dynamics and the Allee effect in stage structured populations. Ecology Letters 10, 564–573 (2007).
Gascoigne, J. C. & Lipcius, R. N. Allee effects driven by predation. Journal of Applied Ecology 41, 801–810 (2004).
Gause, G. F. The Struggle for Existence. Baltimore, MD: Williams & Wilkins, 1934.
Johnson, D. M. et al. Allee effects and pulsed invasion by the gypsy moth. Nature 444, 361–363 (2006).
Kramer, A. M. & Drake, J. Experimental demonstration of population extinction due to a predator-driven Allee effect. Journal of Animal Ecology 79, 633–639 (2010).
Kramer, A. M. et al. The evidence for Allee effects. Population Ecology 51, 341–354 (2009).
Leung, B., Drake, J. M., & Lodge, D. M. Predicting invasions: Propagule pressure and the gravity of Allee effects. Ecology 85, 1651–1660 (2004).
Lewis, M. A. & Kareiva, P. Allee dynamics and the spread of invading organisms. Theoretical Population Biology 43, 141–158 (1993).
Lockwood, J. L., Cassey, P. & Blackburn, T. The role of propagule pressure in explaining invasions. Trends in Ecology & Evolution 20, 223–228 (2005).
Orr, H. A. Fitness and its role in evolutionary genetics. Nature Reviews Genetics 10, 531–539 (2009).
Stephens, P. A. & Sutherland, W. J. Consequences of the Allee effect for behaviour, ecology and conservation. Trends in Ecology and Evolution 14, 401–405 (1999).Taylor, C. M. et
al. Consequences of an Allee effect on the invasion of a Pacific
estuary by Spartina alterniflora. Ecology 85, 3254–3266
(2004).
Veit, R. R. & Lewis, M.
A. Dispersal, population growth, and the Allee effect dynamics of the house
finch invasion of eastern North America. The American Naturalist 148, 255–274 (1996).
Vercken, E. et al. Critical patch size generated by Allee effect in gypsy moth, Lymantria dispar (L.). Ecology Letters 14, 179–186 (2011).