Abstract
Seismological observations show that, in some regions of the lower mantle, an increase in bulk sound velocity, interestingly, occurs in the same volume where there is a decrease in shear velocity. We show that this anti-correlated behavior occurs on cation substitution in bridgmanite by making single crystal elasticity measurements of MgSiO3 and (Mg,Fe,Al)(Si,Al)O3 using inelastic x-ray scattering in the ambient conditions. Cation substitution of ferrous iron and aluminum may explain large low shear velocity provinces in the lower mantle.
Similar content being viewed by others
Introduction
Bridgmanite, or, Pbnm-type magnesium-silicate perovskite, is the dominant mineral in the Earth’s lower mantle. Materials with perovskite or related structures also attract broad attention since they can display novel physical properties such as colossal magnetoresistance1, multiferroicity2, and high-temperature superconductivity3. At pressures over 125 GPa (corresponding to depths more than ~2700 km) and at temperature greater than 2500 K, bridgmanite transforms to a post-perovskite (pPv) phase4 with the Cmcm-type CaIrO3 structure. It is widely believed that pPv is the main component of the D″ layer at the bottom of the lower mantle, which is 200 km thick just above the core mantle boundary (~2900 km depth).
In the deep mantle, between 2000 and 2891 km in depth, some regions show an increase in bulk sound velocity ( ), and a decrease in shear wave velocity (
), and a decrease in shear wave velocity ( ): ΔVB > 0 > ΔVS, and others show a decrease in VB and an increase in VS: ΔVB < 0 < ΔVS5,6 (KS, G, and ρ are adiabatic bulk modulus, shear modulus, and density, respectively). This feature is called an anti-correlated seismic velocity anomaly. It is reported that the phase transformation of (Mg,Fe,Al)(Si,Al)O3 from Pbnm-type to Cmcm-type can explain the increase in VS and decrease in VB from the average (ΔVB < 0 < ΔVS) in some deeper regions7. However, this cannot explain the anomaly in the shallower part of the mantle where the pPv phase is not stable. More importantly, it is difficult to interpret the anti-correlated nature of the anomaly where ΔVB and ΔVS have opposite signs. The regions showing this anomaly, which are beneath Africa and the central Pacific, attract attention as large low shear velocity provinces (LLSVPs).
): ΔVB > 0 > ΔVS, and others show a decrease in VB and an increase in VS: ΔVB < 0 < ΔVS5,6 (KS, G, and ρ are adiabatic bulk modulus, shear modulus, and density, respectively). This feature is called an anti-correlated seismic velocity anomaly. It is reported that the phase transformation of (Mg,Fe,Al)(Si,Al)O3 from Pbnm-type to Cmcm-type can explain the increase in VS and decrease in VB from the average (ΔVB < 0 < ΔVS) in some deeper regions7. However, this cannot explain the anomaly in the shallower part of the mantle where the pPv phase is not stable. More importantly, it is difficult to interpret the anti-correlated nature of the anomaly where ΔVB and ΔVS have opposite signs. The regions showing this anomaly, which are beneath Africa and the central Pacific, attract attention as large low shear velocity provinces (LLSVPs).
The origin of the LLSVPs is under debate. Thermal heterogeneity has been considered8, but exclusively thermal effects are insufficient to explain the LLSVPs because usually both VB and VS decrease with temperature. It is thus suggested that the LLSVPs have very different chemical composition from that of the average mantle9 due to accumulations of subducted oceanic slabs10, remnants of Earth’s early magma ocean11, or even chemical reactions with the core12. Recently primordial metallic melt trapped in the mantle was suggested as the nature of LLSVPs13. A complicated model2, including multiple chemical and thermal effects, can reproduce the distribution of the LLSVPs. But this model requires rather a specific distribution of effects that are not internally well correlated. Houser14 suggested that slow VS might be correlated with temperature and chemical anomaly using the parameter set for bridgmanite15, as was used in ref. 6, but did not discuss the anti-correlated anomaly between VB and VS. The theoretical result15 used in both seismological studies6,14 shows anti-correlation only in elastic moduli but not in velocities, and, more importantly, has not yet been experimentally verified.
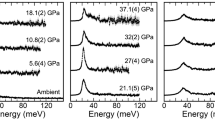
In order to address these issues, we investigated the elastic properties of single-crystal bridgmanite at ambient conditions. Although Brillouin light scattering (BLS) is frequently used to determine elastic properties of high-pressure minerals, the elasticity of iron-bearing bridgmanite has not been determined by BLS due to its opacity, and its instability against strong optical laser irradiation. We used inelastic x-ray scattering (IXS) technique in this study. We prepared two types of bridgmanite: MgSiO3 (Mg-Bdg, hereafter) and Mg0.943Fe0.045Al0.023Si0.988O3 ((Fe,Al)-Bdg hereafter). Iron in (Fe,Al)-Bdg was confirmed by synchrotron Mössbauer spectroscopy to be in high-spin ferrous state and to occupy a large A site of perovskite structure. The sample characterization and more details of the IXS measurements are given in the Methods section. Elastic stiffness tensors (Cij) for Mg-Bdg, and for (Fe,Al)-Bdg was obtained from analysis of IXS spectra based on Christoffel’s equation16. A typical set of IXS spectra is shown in Fig. 1. The elastic moduli obtained are listed in Table 1 together with literature values15,17,18,19,20,21,22,23. The velocity surface plots of Mg-Bdg from the present Cij determined from two sets of IXS measurements are shown in Fig. 2 together with those calculated from BLS results17,18,19. The patterns of the velocity surfaces are similar to each other: the longitudinal velocity is the fastest along about b axis and minimum along c axis, etc. The absolute values determined using IXS are generally smaller than those from BLS.
A representative set of IXS spectra of (Fe,Al)-Bdg collected at BL35XU.
Twelve spectra can be measured at once. Reduced q positions in reciprocal lattice unit are shown in parentheses. A total momentum transfer was (2, −2, 0) + q in reciprocal lattice unit. Each spectrum show one or two pairs of clear phonon signals. Blue crosses show data points. Red lines show pseudo-Voight functions fitted to peaks.
The pattern of the velocity surface of (Fe,Al)-Bdg is basically similar to that of Mg-Bdg (red and blue lines in Fig. 2). The present cation substitution affected the velocity surface as follows: 1. VP along the b and c axes is increased; 2. the average VS along the b axis is decreased; 3. the difference of VS along the a and c axes is increased and decreased, respectively. Crystallographic studies24 report that iron substitution enlarges the a axis more than other axes, which is consistent with the present result (see Method section). The large elongation of the a axis probably results in the least change in VP along a axis. Thus qualitatively the velocity surfaces indicate that elastic anisotropy in bridgmanite increases with the present cation substitution. More quantitatively, the acoustic anisotropy defined by 2 × (Vmax − Vmin)/(Vmax + Vmin) increases from 8.81% to 8.92% for longitudinal waves, and from 12.4% to 13.4% for transverse waves. The present results experimentally demonstrate that the degree of anisotropy is increased by the present cation substitution.
The Voigt-Ruess-Hill average of bulk and shear moduli calculated from Cij are listed in Table 1. We determine KS and G to be 236 and 166 GPa, respectively for Mg-Bdg. The value of KS in the present study is consistent with that determined from RUS20 and that by a calculational study22, but is lower than the other values by ~15 GPa (6%). G is also smaller by ~10 GPa (also 6%) than those in the previous results. These differences correspond to 3% in velocity. The origin of the differences in KS and G between two techniques should be further investigated. Nevertheless, this study experimentally demonstrated that the present cation substitution in bridgmanite increases KS and VB and decreases G and VS: an anti-correlated behavior.
This anti-correlated behavior in elastic moduli and velocities by cation substitution has not been reported. Bulk and shear moduli and velocities are summarized in Fig. 3 together with previous results. Previously, the effect of Fe substitution was investigated using ultrasonic interferometry21 (UI) and calculations15,22. The sample used in the UI study contained not only Fe2+ but also Fe3+. The results of the UI study disagree with one calculation15, where Fe2+ substituted for Mg2+, but rather agree with another22, where Fe3+ and Al3+ substituted for Mg2+ and Si4+. These results21,22 imply that Fe3+ substituting for Mg2+ degreases both KS and G. The effect of aluminum substitution was reported using BLS25 and theoretical calculation21. An experimental study25 reported that the substitution of only Al decreases both elastic moduli and slightly increases VB. A theoretical study22 showed that the substitution of only Al decrease VB and VS as well as KS and G. That study22 also investigated the effect of coupled substitution of Fe3+ and Al, demonstrating that the effect of this pair substitution is qualitatively the same as that of the substitution of aluminum only.
Elastic moduli and elastic velocities of bridgmanite with chemical substitution of Fe and Al for Mg and Si.
The present results are shown by solid (red) circles. Open circles, squares, and triangles indicate values determined by RUS20, BLS17,18,19,25 and US21,59. Diamonds indicate theoretical values (open for ferrous iron (LDA)15 and solid for aluminum and ferric iron (GGA)22). Crosses indicate values by GGA-DFPT-IXS23. Lines are guide for eyes (solid (red): present study; dotted: previous experiments; dashed: theory).
Water content sometimes reduces elastic moduli. The present samples contain a certain amount of water (140 and 460 ppm). However, it is not known how much water content affects the elasticity of bridgmanite. If water content decreased shear modulus for bridgmanite, e.g. by 0.3 GPa/100 ppm or shear velocity by 0.02 km/s/100 ppm, the present cation substitution for dry bridgmanite would show a positive correlated behavior, or increase both KS and G. The anti-correlated behavior observed in this study may be due to a combination of the cation substitution and the water content.
We simply consider the effect of iron and aluminum separately thought these substitutions may be coupled. Many investigations have been done about the effect of cation substitution on isothermal bulk modulus of bridgmanite by measuring compression curves. It is well known that KT0 and K′ derived from a compression-curve fitting are strongly correlated. KT0 also depends on the pressure range of the measurement, sample conditions, etc. Nevertheless, the relative change in KT0 determined by the same technique is reliable. The effect of a small amount of Fe2+ substitution on KT0 is reported to be positive26,27,28,29. This is qualitatively consistent with the theoretical study15. In contrast, the effect of aluminum on KT0 is still controversial; a positive effect (increasing KT0) is reported in some studies26,30 and negative effect (decreasing KT0) in others30,31,32. Based on the BLS studies17,18,19,25 and the theoretical one21, the effect of aluminum substitution on KS can be considered negative. Note that the theoretical study22 also investigated the effect of coupling substitution of Fe3+ and Al, demonstrating that the effect of this pair substitution is qualitatively the same as that of the substitution of aluminum only. The effect of only Fe2+ on the velocities can be calculated from the present study by subtracting the effect of Al from the BLS results17,18,19,25, assuming that the effects of Fe2+ and Al are independent. This analysis suggests that Fe2+ substitution increases both VB and VS (Table 2).
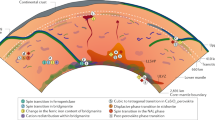
We apply the present results to a geochemical and geothermal model to estimate if this effect is sufficient to explain the LLSVPs. We assume a perovskitic lower mantle33 for simplicity. The seismic anomaly observed in the LLSVPs (+1 and −1% of ΔVB/VB and ΔVS/VS, respectively)5,6 may, then be explained by variation of Fe2+ and Al substitution into bridgmanite at temperature conditions for 2000–2891 km depth (2250–2450 K34). The temperature effects on VB and VS were assumed to be independent of pressure and composition (Table 2). The observed anomaly of +/−1% for VB and −/+1% for VS corresponds to the compositional variation between MgSiO3 and Mg0.959Fe0.027Al0.028Si0.986O3, +/−2.0 atom% of ΔFe/(Mg + Fe + Si + Al) and +/−1.3 atom% of ΔAl/(Mg + Fe + Si + Al) in temperature range of 2250–2450 K34. This compositional heterogeneity of bridgmanite then explains the anti-correlated seismic anomaly (Figs 4 and 5AB). This model indicates that cation substitution of a few atomic percent causes an anti-correlated anomaly comparable to that observed in the LSSVPs.
Required ΔX/(Mg + Fe + Si + Al) (X = Fe or Al) to explain the velocity anomaly of +1% for VB and −1% for VS with temperature difference ΔT.
Solid and open circles are for Fe and Al, respectively. With increasing ΔT, required ΔFe and ΔAl increases and decreases respectively. When ΔT is around 110 K, the velocity anomaly can be explained only with ΔFe and the distribution of Al should be uniform.
Schematic image of regional variation of seismic velocities, chemical composition of bridgmanite, and temperature variation at depth between 2000 and 2891 km.
(A) Red color indicate the anomaly of ΔVB > 0 > ΔVS. The map outline was made using CraftMAP (http://www.craftmap.box-i.net/). (B) The case of ΔT (difference from the average temperature) = 0. Fe and Al contents are the highest in the red regions. (C) The case of ΔAl/(Mg + Fe + Si + Al) = 0. Fe content is the highest in the red regions, where temperature is higher than the average temperature by 113 K. The map was colored based on ref. 6.
We now consider to include the effect of temperature since the LLSVPs may correlate with local temperature changes. We assume the temperature difference ΔT between the regions with the highest VB and the average value, i.e. ΔT = T(ΔVB/VB = 1%) −T(ΔVB/VB = 0%). The chemical inhomogeneity, ΔX/(Mg + Fe + Si + Al) (X = Fe or Al), needed to explain the velocity anomaly is then shown in Fig. 4. Especially when ΔT is about 113 K, the LSSVPs can be explained by only 2.7 atom% of Fe2+ substitution without Al variation (Fig. 5AC). More detailed modeling requires ferropericlase and taking the effect of spin transition for these two materials into account.
We have experimentally demonstrated that cation substitutions in bridgmanite enhances elastic anisotropy and causes anti-correlated behavior in elastic wave velocities. This result indicates that seismic anomalies observed in the lower mantle could be explained by chemical heterogeneity in bridgmanite.
Methods
Sample synthesis and characterizations
The single crystals examined in this study were synthesized at 24 GPa and 1500 °C using a Kawai-type multi anvil press (USSA-5000) installed at ISEI, Okayama University35. The isotope ratios of chemical reagents were at natural abundance.
The chemical compositions are confirmed as MgSiO3 and Mg0.943Fe0.045Al0.023Si0.988O3 by an electron microprobe analyzer. The number ratios of Fe/(Mg + Fe + Si + Al) and Al/(Mg + Fe + Al + Si) of this sample are 0.023 and 0.012, respectively. Assuming all Fe is bivalent, the sum of the charge estimated from the EPMA results is −0.003. This is negligible, taking the uncertainty of the chemical analysis into account. A typical amount of water content of single crystals in the run product was 140 ± 52 and 460 ± 45 ppm according to synchrotron IR absorption analyses35.
The Fe3+/ΣFe ratio of (Fe,Al)-Bdg was evaluated with synchrotron Mössbauer spectroscopy at BL10XU of SPring-836. An obtained spectrum were analyzed using program MossA37. Without any prejudice, the spectrum seems to consist of two absorption lines with different intensities (Fig. 6). They can be interpreted either as two singlets, as an asymmetric doublet, or as combination of a doublet and a singlet. If the spectrum consists of two singlets, an isomer shift of 1.96(9) mm/s corresponds to that of monovalent high-spin iron. Considering the charge neutrality of the system, it is difficult for Fe+ to substitute for Mg or Si in perovskite structure. Therefore, the absorption line at 1.96 mm is the higher velocity one of a doublet. Analysis based on an asymmetric doublet gives the isomer shift of 1.05(6) mm/s and quadrupole splitting of 1.8(1) mm/s. These values indicate that iron in this sample was in a divalent high-spin state38 and substitute for magnesium39. The higher intensity at the lower velocity side is attributed either to that the sample was a single crystal or to that iron existed in another state. The former case is more plausible than the latter due to the following reasons. 1. The linewidths determined using two singlets (0.97(16) and 0.76(30) mm/s for lower and higher velocity lines) are consistent within the fitting uncertainty; 2. The line shape of the lower velocity signal looks symmetric and additional singlet/doublet to the lower velocity signal has not improved the fitting quality at all. We off course have paid much attention to possible existence of iron in a trivalent high-spin state, which should give a doublet with an isomer shift of ~0.5 mm/s38 and a quadrupole splitting of 0.5–1.0 mm/s39. Since parameter fitting assuming two doublets (one for HS Fe2+ and another for HS Fe3+) was not converged, we were not able to detect the amount of ferric iron if existed. The asymmetric doublet is probably attributed to a certain angle between the principal electric field gradient in the Fe site and the incident X-ray beam direction because the sample was a single crystal. The intensity ratio (2.7: 1) indicates the sign of the quadrupole splitting was negative. The linewidth assuming one doublet is 0.93(13) mm/s, which is much broader than a typical energy resolution of the Mössbauer spectrometer at BL10XU (0.43 mm/s). This is perhaps due to variation of the local environment around Fe in this sample given by Mg/Al/Si distribution in neighboring sites, hydrogen, and/or oxygen vacancy. The results of the synchrotron Mössbauer measurement conclude that most iron atoms were in a divalent high-spin state and occupied a magnesium site. Consequently, the simplest substitution model, where the iron substitutes for magnesium and aluminum substitutes for both magnesium and silicon in perovskite structure is consistent with the results of these analyses.
Mössbauer spectrum of 57Fe in (Fe,Al)-Bdg.
Blue circles are raw data from which the backgrounds have been subtracted. The red and green lines indicate one doublet fitted to the data and fitting residuals. The isomer shift of 1.05 mm/s and the quadrupole splitting of 1.8 mm/s indicate that the sample contains high-spin Fe2+ in the magnesium site38,39.
The investigated grains were confirmed to be single domains using a four-circle diffractometer with a laboratory x-ray source at room temperature. The lattice constants a, b, and c were 4.7784(3), 4.9306(4), 6.9005(8) Å and 4.787(1), 4.934(1), 6.904(1) Å for the Mg-Bdg and (Fe,Al)-Bdg, respectively. It was reported that the unit cell volume of MgSiO3 bridgmanite does not change even with 100 ppm water content40. An analytical curve drawn by fitting a linear function to literature values19,20,21,24,26,27,28,30,31,32,41,42,43,44,45,46,47,48,49,50,51,52,53 is shown in Fig. 7. The obtained analytical line for iron substitution is consistent with literature29. The unit cell volumes of Mg-Bdg is larger than the present analytical line by only 0.05%. Since these differences are comparable to the experimental error, the water content of 140 ppm seems to give a negligible effect. In contrast, the unit cell volume of the (Fe-Al)-Bdg is larger than those of the analytical lines by 0.29%. This excess volume may be explained by effect of aluminum and water content. It is known that aluminum incorporation increases the unit cell volume26,30,31,32. Estimating from the previous results, the value of 0.012 for Al/(Mg + Fe + Al + Si) makes the unit cell volume larger by 0.09%. Although the degree of water effect on the unit cell volume of magnesium silicate perovskite is uncertain, the water content of 460 ppm probably made the unit cell volume larger by 0.20%. The densities of the Mg-Bdg and (Fe,Al)-Bdg are 4103.3 and 4139.5 g/cm3, respectively.
An analytical curves between unit cell volume of bridgmanite and chemical substitutions (a) by iron and (b) by aluminum. The solid (red) circles are the present results. The dotted lines were fitted to literature values shown by open cirlces19,20,21,24,25,26,27,28,29,30,31,32,41,42,43,44,45,46,47,48,49,50,51,52,53. (a) 11.741x + 162.5 and (b) 12.926x + 162.5, where x is (Fe + Al)/(Mg + Fe + Si + Al) and V at x = 0 is fixed to 162.5 Å3.
IXS measurement and data analysis
Inelastic X-ray scattering with a single crystal sample in conjunction with an analysis based on Christoffel’s equation has been recently used for accurate determination of elastic moduli16,54,55,56. This technique has been adopted to data along high-symmetry directions about samples at high-pressure and high-temperature conditions55,56. In this study, we did not limit data along high-symmetry directions, but measured rather redundant data at off-symmetry positions to determine Cij precisely and to utilize all measured data with an analyzer array16,54 (see Fig. 1). We performed IXS measurements at BL35XU of SPring-857 at 21.747 and 17.794 keV, with which typical energy resolutions were 1.5 and 3.0 meV full-width-half-maximum (FWHM), respectively. 21.747 keV x-ray was used for Mg-Bdg and 17.794 keV for (Fe,Al)-Bdg. The size of the incident X-ray beam was ~70 μm in diameter. We performed another measurement for Mg-Bdg to insure the quality of our results. We measured another grain from the same sample growth run at BL43LXU of SPring-858. At BL43LXU, x-ray beam with size of ~20 μm and energy of 17.794 keV was used. The energy resolution was 3.0 meV (FWHM).
For each observed phonon mode, the elastic wave velocity was calculated assuming a linear relationship between phonon energy and momentum. Single crystal elasticity at ambient conditions was determined by least-square fitting to the observed velocities using the measured densities. Details of the fitting are given in ref. 16. Phonons with momentum transfers, |q| from 1 to 3 nm−1 away from Bragg peaks were used for analysis.
The elastic moduli determined from data at BL35XU and BL43LXU are consistent in contrast to different BLS studies which are not so consistent. The individual results are listed in Tables 1 and 3. The different IXS measurement agree to better than 14% (the maximum deviation) whereas those from three BLS studies are spread more (26%, the maximum deviation). Therefore these two sets of IXS data for Mg-Bdg were analyzed as one set (giving 461 modes) to obtain more reliable elastic properties. For (Fe,Al)-Bdg, 319 modes were used. The residuals of the fitting are shown in Fig. 8. There is a slightly linear relationship between ΔE and |q| observed, probably meaning the assumed linear relationship between ΔE and |q| is not completely valid at this q range.
Additional Information
How to cite this article: Fukui, H. et al. Effect of cation substitution on bridgmanite elasticity: A key to interpret seismic anomalies in the lower mantle. Sci. Rep. 6, 33337; doi: 10.1038/srep33337 (2016).
References
Ramirez, A. P. Colossal magnetoresistance. J. Phys. Cond. Mat. 9, 8171 (1997).
Kimura, T. et al. Magnetic control of ferroelectric polarization. Nature 426, 55 (2003).
Bednorz, J. G. & Müller, K. A. Perovskite-type oxides-The new approach to high-TC superconductivity. Rev. Mod. Phys. 60, 585 (1998).
Murakami, M., Hirose, K., Kawamura, K., Sata, N. & Ohishi, Y. Post-perovskite phase transition in MgSiO3 . Science 304, 855 (2004).
Masters, G., Laske, G., Bolton, H. & Dziewonski, A. The Relative Behavior of Shear Velocity, Bulk Sound Speed, and Compressional Velocity in the mantle: Implications for Chemical and Thermal Structure. In Earth’s deep interior. Mineral physics and tomography from the atomic to the global scale vol. 117, edited by Karato, S.-I., Forte, A., Liebermann, R. C., Masters, G. & Stixrude, L. pp. 63–87, American Geophysical Union, Washington, DC (2000).
Trampert, J., Deschamps, F., Resovsky, J. & Yuen, D. Probabilistic Tomography Maps Chemical Heterogeneities Throughout the Lower Mantle. Science 306, 853–856 (2004).
Hutko, A. R., Lay, T., Revenaugh, J. & Garnero, E. J. Anticorrelated seismic velocity anomalies from post-perovskite in the lowermost mantle. Science 320, 1070, (2008).
Davies, D. R., Goes, S., Davies, J. H., Schuberth, B. S. A., Bunge, H. P. & Ritsema, J. Reconciling dynamic and seismic models of Earth’s lower mantle: The dominant role of thermal heterogeneity. Earth Planet. Sci. Lett. 353, 253–269 (2012).
Kennett, B. L. N., Widiyanto, S. & van der Hilst, R. D. Joint seismic tomography for bulk sound and shear wave speed in the Earth’s mantle. J. Geophys. Res. 103 B6, 12469–12493 (1998).
Tackley, P. J. Living dead slabs in 3-D: The dynamics of compositionally-stratified slabs entering a “slab graveyard” above the core-mantle boundary. Phys. Earth Planet. Interiors 188, 150–162 (2011).
Labrosse, S., Hernlund, J. W. & Coltice, N. A crystallizing dense magma ocean at the base of the Earth’s mantle. Nature 450, 866–869 (2007).
Kellogg, L. H. & King, S. D. Effect of mantle plumes on the growth of D′′ by reaction between the core and mantle. Geophys. Res. Lett. 20, 379 (1993).
Zhang, Z. et al. Primordial metallic melt in the deep mantle. Geophys. Res. Lett. 43, 3693 (2016).
Houser, C. Constraints on the Presence or Absence of Post-Perovskite in the Lowermost Mantle From Long-Period Seismology. In Post-Perovskite: The Last Mantle Phase Transition Geophysical Monograph Series 174, pp. 191–216 (2007).
Kiefer, B., Stixrude, L. & Wentzcovitch, R. M. Elasticity of (Mg,Fe)SiO3-Perovskite at high pressure. Geophys. Res. Lett. 29, 1539 (2002).
Fukui, H. et al. Precise determination of elastic constants by high-resolution inelastic X-ray scattering. J. Synchro. Radiat. 15, 618 (2008).
Yeganeh-Haeri, A., Weidner, D. J. & Ito, E. Elasticity of MgSiO3 in the Perovskite Structure. Science 243, 787 (1989).
Yeganeh-Haeri, A. Synthesis and re-investigation of the elastic properties of single-crystal magnesium silicate perovskite. Phys. Earth Planet. Inter. 87, 111–121 (1994).
Sinogeikin, S. V., Zhang, J. & Bass, J. D. Elasticity of single crystal and polycrystalline MgSiO3 perovskite by Brillouin spectroscopy. Geophys. Res. Lett. 31, L06620 (2004).
Aizawa, Y. et al. Temperature derivatives of elastic moduli of MgSiO3 perovskite. Geophys. Res. Lett. 31, L01602 (2004).
Chantel, J., Frost, D. J., McCammon, C. A., Jing, Z. & Wang, Y. Acoustic velocities of pure and iron-bearing magnesium silicate perovskite measured to 25 GPa and 1200 K. Geophys. Res. Lett. 39, L19307 (2012).
Li, L. et al. Elasticity of (Mg, Fe)(Si, Al)O3-perovskite at high pressure. Earth Planet. Sci. Lett. 240, 529 (2005).
Wehinger, B. et al. Dynamical and elastic properties of MgSiO3 perovskite (bridgmanite). Geophys. Res. Lett. 43, 2568 (2016).
Kudoh, Y., Prewitt, C. T., Finger, L. W., Darovskikh, A. & Ito, E. Effect of Fe on the crystal structure of (Mg,Fe)SiO3 perovskite. Geophys. Res. Lett. 17, 1481–1484 (1990).
Jackson, J. M., Zhang, J. & Bass J. D. Sound velocities and elasticity of aluminous MgSiO3 perovskite: Implications for aluminum heterogeneity in Earth’s lower mantle. Geophys. Res. Lett. 31, L10614 (2004).
Andrault, D., Bolfan-Casanova, N. & Guignot, N. Equation of state of lower mantle (Al,Fe)-MgSiO3 perovskite. Earth. Planet. Sci. Lett. 193, 501 (2001).
Boffa Ballaran, T. et al. Effect of chemistry on the compressibility of silicate perovskite in the lower mantle. Earth Planet. Sci. Lett. 333–334, 181 (2012).
Lundin, S. et al. Effect of Fe on the equation of state of mantle sliciate perovskite over 1 Mbar. Phys. Earth Planet. Interiors 168, 97–102 (2008).
Dorfman, S. M., Meng, Y., Prakapenka, V. B. & Duffy, T. S. Effects of Fe-enrichment on the equation of state and stability of (Mg,Fe)SiO3 perovskite. Earth Planet. Sci. Lett. 361, 249 (2013).
Yagi, T., Okabe, K., Nishiyama, N., Kubo, A. & Kikegawa, T. Complicated effects of aluminum on the compressibility of silicate perovskite. Phys. Earth Planet. Interirors 143–144, 81–91 (2004).
Daniel, I. et al. Effect of aluminum on the compressibility of silicate perovskite. Geophys. Res. Lett. 31, L15608 (2004).
Zhang, J. & Weidner D. J. Thermal Equation of State of Aluminum-Enriched Silicate Perovskite. Sicence 284, 782 (1999).
Murakami M. et al. A perovskitic lower mantle inferred from high-pressure, high-temperature sound velocity data. Nature 485, 90–94 (2012).
Brown, J. M. & Shankland, T. J. Thermodynamic parameters in the Earth as determined from seismic profiies. Geophys. J. Roy. Astron. Soc. 66, 579–596 (1981).
Shatskiy, A. et al. Growth of large (1 mm) MgSiO3 perovskite single crystals: A thermal gradient method at ultrahigh pressure. Am. Min. 92, 1744 (2007).
Hirao, N. et al. Development of combination method with SR x-ray diffraction and Mössbauer spectroscopy for high-pressure research. Special issue of Rev. High Pressure Sci. Tech. 23, 2C08 (2013).
Prescher, C., McCammon, C. & Dubrovinsky, L. MossA: a program for analyzing energy-domain Mössbauer spectra from conventional and synchrotron sources. J. Appl. Cryst. 45, 329–331 (2012).
Gütlich, P. Mössbauer Spectroscopy in Chemistry In Mössbauer Spectroscopy, Topics in Applied Physics Vol. 5 (eds Gonser, U. ) (Springer-Verlag Berlin Heidelberg New York, 1975).
Hsu, H., Blaha, P., Cococcioni, M. & Wentzcovitch, R. M. Spin-State Crossover and Hyperfine Interactions of Ferric Iron in MgSiO3 Perovskite. Phys. Rev. Lett. 106, 118501 (2011).
Litasov, K. et al. Water solubility in Mg-perovskites and water storage capacity in the lower mantle. Earth Planet. Sci. Lett. 211,189 (2003).
Ito, E. & Matsui, Y. Synthesis & crystal-chemical characterization of MgSiO3 perovskite. Earth Planet. Sci. Lett. 38, 443–450 (1978).
Yagi, T., Mao, H. K. & Bell, P. M. Structure and crystal chemistry of perovskite-type MgSiO3 . Phys. Chem. Minerals 3, 97–110 (1978).
Yagi, T., Mao, H. K. & Bell, P. M. Lattice parameters and specific volume for perovskite phase of orthopyroxene composition (Mg,Fe)SiO3 Carnegie Inst.: Washington Yearbook, 78, 612–613 (1979).
Ito, E. & Yamada, H. Stability relations of silicate spinels, ilmenites and perovskites. In Akimoto, S. & Manghani, M. H. Eds High-Pressure Research in Geophysics p. 405–419. Center for Academic Publications, Tokyo, Japan (1982).
Kudoh Y., Ito, E. & Takeda, H. Effect of Pressure on the Crystal Structure of Perovskite-type MgSiO3 . Phys. Chem. Minerals 14, 350 (1989).
Horiuchi, H., Ito, E. & Weidner, D. J. Perovskite-type MgSiO3: single crystal X-ray diffraction study. American Mineralogist 72, 357–360 (1987).
Parise, J. B., Wang, Y., Yeganeh-Haeri, A., Cox, D. E. & Fei, Y. Crystal structure and thermal expansion of (Mg,Fe)SiO3 perovskite. Geophys. Res. Lett. 17, 2089–2092 (1990).
Ross, N. L. & Hazen, R. M. High-pressure crystal chemistry of MgSiO3 perovskite. Phys. Chem. Minerals 17, 228–237 (1990).
Jephcoat, A. P. et al. High-resolution synchrotron X-ray powder diffraction and Rietveld structure refinement of two (Mg0.95,Fe0.05)SiO3 perovskite samples synthesized under different oxygen fugacity conditions. American Mineralogist, 84, 214–220 (1999).
Dobson, D. P. & Jacobsen, S. D. The flux growth of magnesium silicate perovskite single crystals. American Mineralogist 89, 807–811 (2004).
Sugahara, M. et al. Reinvestigation of the MgSiO3 perovskite structure at high pressure. American Mineralogist 91, 533–536 (2006).
Katsura, T. et al. P-V-T relations of MgSiO3 perovskite determined by in situ X-ray diffraction using a large-volume high-pressure apparatus. Geophys. Res. Lett. 36, L01305 (2009).
Tange, Y., Takahashi, E., Nishihara, Y., Funakoshi, K. & Sata, N. Phase relations in the system MgO-FeO-SiO2 to 50 GPa and 2000 °C: An application of experimental techniques using multianvil apparatus with sintered diamond anvils. J. Geophys. Res. 114, B02214 (2009).
Yoneda, A. et al. Elastic anisotropy of experimental analogues of perovskite and post-perovskite help to interpret D″ diversity. Nature Comm. 5, 3453 (2014).
Antonangeli, D. et al. Elasticity of Cobalt at High Pressure Studied by Inelastic X-Ray Scattering. Phys. Rev. Lett. 93, 215505 (2004).
Antonangeli, D. et al. Elasticity of Hexagonal-Closed-Packed Cobalt at High Pressure and Temperature: A Quasiharmonic Case. Phys. Rev. Lett. 100, 085501 (2008).
Baron, A. Q. R. et al. X-ray scattering beamline for studying dynamics. J. Phys. Chem. Solids 61, 461 (2000).
Baron, A. Q. R. Status of the RIKEN Quantum NanoDynamics Beamline (BL43LXU): The Next Generation for Inelastic X-Ray Scattering. SPring-8 Inf. Newsl. 15, 14 (2010).
Li, B. & Zhang, J. Pressure and temperature dependence of elastic wave velocity of MgSiO3 perovskite and the composition of the lower mantle. Phys. Earth Planet. Interiors 151, 143–154 (2005).
Li, B. Characteristics of lateral heterogeneities with thermal and chemical origins in the pyrolitic lower mantle. Progress in Natural Science 19, 1603–1611 (2009).
Acknowledgements
We appreciate help by Longjian Xie, Ryo Watanabe, and Tatsuya Hiratoko during IXS experiments. This work was performed using joint-use facilities of the Institute for Study of the Earth’s Interior, Okayama University and supported in part by Grants-in-Aid for Scientific Research (Grant Nos 22224008 and 15H021128 awarded to AY and Nos 22000002 and 15H05748 to EO) from the Japan Society for the Promotion of Science. Inelastic X-ray scattering measurements at BL35XU57 were done with the approval of JASRI (Proposal Nos 2012B1196, 2013A1047, 2013B1054, and 2014B1290). Measurements at RIKEN BL43LXU58 were made during commissioning time. The synchrotron Mössbauer spectroscopy was performed on the BL10XU at SPring-8 (Proposal No. 2014A0104).
Author information
Authors and Affiliations
Contributions
H.F. planned the present study. A.S. synthesized crystals. A.N. crystallographically characterized synthesized crystals. N.T. analyzed the chemical compositions of the samples. S.K., E.O. and N.H. performed the synchrotron Mössbauer measurements. S.K. analyzed the Mössbauer spectrum with input from S.T., H.F., A.Y., S.T., H.U. and A.Q.R.B. performed the IXS measurements at BL35XU and H.F., A.Y. and A.Q.R.B. at BL43LXU. H.F. analyzed the IXS data with input from A.Y. and A.Q.R.B., H.F., A.Y. and A.Q.R.B. wrote the manuscript.
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Fukui, H., Yoneda, A., Nakatsuka, A. et al. Effect of cation substitution on bridgmanite elasticity: A key to interpret seismic anomalies in the lower mantle. Sci Rep 6, 33337 (2016). https://doi.org/10.1038/srep33337
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/srep33337
This article is cited by
-
Incorporation mechanism of Fe and Al into bridgmanite in a subducting mid-ocean ridge basalt and its crystal chemistry
Scientific Reports (2021)
-
Elasticity of lower-mantle bridgmanite
Nature (2018)
-
Discovery of MgTiSi2O7: a new high-pressure silicate with the weberite structure synthesized at transition-zone conditions
Physics and Chemistry of Minerals (2017)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.