Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

  • Review Article
  • Published:

Tensor networks for complex quantum systems

Nature Reviews Physics volume 1, pages 538–550 (2019)Cite this article

Subjects

Abstract

Originally developed in the context of condensed-matter physics and based on renormalization group ideas, tensor networks have been revived thanks to quantum information theory and the progress in understanding the role of entanglement in quantum many-body systems. Moreover, tensor network states have turned out to play a key role in other scientific disciplines. In this context, here I provide an overview of the basic concepts and key developments in the field. I briefly discuss the most important tensor network structures and algorithms, together with an outline of advances related to global and gauge symmetries, fermions, topological order, classification of phases, entanglement Hamiltonians, holografic duality, artificial intelligence, the 2D Hubbard model, 2D quantum antiferromagnets, conformal field theory, quantum chemistry, disordered systems and many-body localization.

Key points

  • Tensor networks are mathematical representations of quantum many-body states based on their entanglement structure.

  • Different tensor network structures describe different physical situations, such as low-energy states of gapped 1D systems, 2D systems and scale-invariant systems.

  • Variational methods over families of tensor networks enable the approximation of the low-energy properties of complex quantum Hamiltonians. Other methods also allow the simulation of time evolution, the calculation of low-energy excitations and much more.

  • Symmetric tensor network states enable more efficient simulation methods and the description of fermionic systems, lattice gauge theories, topological order and the classification of phases of quantum matter.

  • Tensor networks, such as the multiscale entanglement renormalization ansatz, have been linked to a possible lattice realization of the holographic principle in quantum gravity.

  • Tensor networks also provide a natural framework for understanding machine learning and probabilistic language models.

This is a preview of subscription content, access via your institution

Access options

Buy this article

  • Purchase on Springer Link
  • Instant access to full article PDF
Buy now

Prices may be subject to local taxes which are calculated during checkout

Fig. 1: Diagrammatic representation of a tensor network and several examples of tensor networks.
Fig. 2: Symmetries in tensors and tensor networks.
Fig. 3: 2D projected entangled pair state (PEPS) on a cylinder.
Fig. 4: 1D MERA.

Similar content being viewed by others

References

  1. Orús, R. A practical introduction to tensor networks: matrix product states and projected entangled pair states. Ann. Phys. -New Y. 349, 117158 (2014). Broad introduction to basic concepts about TNs, MPS and PEPS.

    MathSciNet  MATH  Google Scholar 

  2. Kramers, H. A. & Wannier, G. H. Statistics of the two-dimensional ferromagnet. Part II. Phys. Rev. 60, 263 (1941).

    ADS  MathSciNet  MATH  Google Scholar 

  3. Baxter, R. J. Corner transfer matrices. Phys. A 106, 18–27 (1981).

    MathSciNet  Google Scholar 

  4. White, S. R. Density matrix formulation for quantum renormalization groups. Phys. Rev. Lett. 69, 28632866 (1992). Original DMRG paper, which includes many of the fundamental ideas of TNs.

    Google Scholar 

  5. White, S. R. Density-matrix algorithms for quantum renormalization groups. Phys. Rev. B 48, 10345 (1992).

    ADS  Google Scholar 

  6. Fannes, M., Nachtergaele, B. & Werner, R. F. Finitely correlated states on quantum spin chains. Commun. Math. Phys. 144, 443–490 (1992).

    ADS  MathSciNet  MATH  Google Scholar 

  7. Klümper, A., Schadschneider, A. & Zittartz, J. Equivalence and solution of anisotropic spin-1 models and generalized t-J fermion models in one dimension. J. Phys. A 24, L955 (1991).

    ADS  MathSciNet  Google Scholar 

  8. Klümper, A., Schadschneider, A. & Zittartz, J. Matrix product ground states for one-dimensional spin-1 quantum antiferromagnets. Europhys. Lett. 24, 293 (1993).

    ADS  Google Scholar 

  9. Hackbusch, W. Tensor Spaces and Numerical Tensor Calculus (Springer, 2012).

  10. Östlund, S. & Rommer, S. Thermodynamic limit of density matrix renormalization. Phys. Rev. Lett. 75, 3537 (1995).

    ADS  Google Scholar 

  11. Vidal, G., Latorre, J. I., Rico, E. & Kitaev, A. Entanglement in quantum critical phenomena. Phys. Rev. Lett. 90, 227902 (2003). Scaling of entanglement at (1+1) d conformal field theories. A basic result to understand the motivation for TNs.

    ADS  Google Scholar 

  12. Hastings, M. B. Solving gapped Hamiltonians locally. Phys. Rev. B 73, 085115 (2006).

    ADS  Google Scholar 

  13. Verstraete, F. & Cirac, J. I. Renormalization algorithms for quantum-many body systems in two and higher dimensions. Preprint at https://arxiv.org/abs/cond-mat/0407066 (2004). An example of PEPS being used as variational ansatz for 2D systems.

  14. Vidal, G. Entanglement renormalization. Phys. Rev. Lett. 99, 220405 (2007). Paper on entanglement renormalization, discussing its fundamental ideas.

    ADS  Google Scholar 

  15. Swingle, B. Entanglement renormalization and holography. Phys. Rev. D 86, 065007 (2012). Paper on the connection between MERA and the geometry of space-time.

    ADS  Google Scholar 

  16. Levine, Y., Sharir, O., Cohen, N. & Shashua, A. Quantum entanglement in deep learning architectures. Phys. Rev. Lett. 122, 065301 (2019).

    ADS  MathSciNet  Google Scholar 

  17. Wolf, M. M., Verstraete, F., Hastings, M. B. & Cirac, J. I. Area laws in quantum systems: mutual information and correlations. Phys. Rev. Lett. 100, 070502 (2008).

    ADS  MathSciNet  MATH  Google Scholar 

  18. Vidal, G. Efficient classical simulation of slightly entangled quantum computations. Phys. Rev. Lett. 91, 147902 (2003). An introduction to the basics of the canonical decomposition of an MPS.

    ADS  Google Scholar 

  19. Vidal, G. Efficient simulation of one-dimensional quantum many-body systems. Phys. Rev. Lett. 939, 040502 (2004).

    Google Scholar 

  20. Vidal, G. Classical simulation of infinite-size quantum lattice systems in one spatial dimension. Phys. Rev. Lett. 98, 070201 (2007).

    ADS  Google Scholar 

  21. Orús, R. & Vidal, G. Infinite time-evolving block decimation algorithm beyond unitary evolution. Phys. Rev. B 78, 155117 (2008).

    ADS  Google Scholar 

  22. Tagliacozzo, L., de Oliveira, R. R., Iblisdir, S. & Latorre, J. I. Scaling of entanglement support for matrix product states. Phys. Rev. B 78, 024410 (2008).

    ADS  Google Scholar 

  23. Pollmann, F., Mukerjee, S., Turner, A. & Moore, J. E. Theory of finite-entanglement scaling at one-dimensional quantum critical points. Phys. Rev. Lett. 102, 255701 (2009).

    ADS  Google Scholar 

  24. Zou, Y., Milsted, A. & Vidal, G. Conformal data and renormalization group flow in critical quantum spin chains using periodic uniform matrix product states. Phys. Rev. Lett. 121, 230402 (2018).

    ADS  Google Scholar 

  25. Perez-García, D., Verstraete, F., Cirac, J. I. & Wolf, M. M. PEPS as unique ground states of local Hamiltonians. Quant. Inf. Comp. 8, 0650–0663 (2008).

    MathSciNet  MATH  Google Scholar 

  26. Verstraete, F., Wolf, M. M., Pérez-García, D. & Cirac, J. I. Criticality, the area law, and the computational power of projected entangled pair states. Phys. Rev. Lett. 96, 220601 (2006).

    ADS  MathSciNet  MATH  Google Scholar 

  27. Schuch, N., Wolf, M. M., Verstraete, F. & Cirac, J. I. Computational complexity of projected entangled pair states. Phys. Rev. Lett. 98, 140506 (2007).

    ADS  MathSciNet  MATH  Google Scholar 

  28. Dubail, J. & Read, N. Tensor network trial states for chiral topological phases in two dimensions and a no-go theorem in any dimension. Phys. Rev. B 92, 205307 (2015).

    ADS  Google Scholar 

  29. Buerschaper, O., Aguado, M. & Vidal, G. Explicit tensor network representation for the ground states of string-net models. Phys. Rev. B 79, 085119 (2009).

    ADS  Google Scholar 

  30. Gu, Z.-C., Levin, M., Swingle, B. & Wen, X.-G. Tensor-product representations for string-net condensed states. Phys. Rev. B 79, 085118 (2009).

    ADS  Google Scholar 

  31. Shi, Y., Duan, L. & Vidal, G. Classical simulation of quantum many-body systems with a tree tensor network. Phys. Rev. A 74, 022320 (2006).

    ADS  Google Scholar 

  32. Silvi, P. et al. Homogeneous binary trees as ground states of quantum critical Hamiltonians. Phys. Rev. A 81, 062335 (2010).

    ADS  Google Scholar 

  33. Tagliacozzo, L., Evenbly, G. & Vidal, G. Simulation of two-dimensional quantum systems using a tree tensor network that exploits the entropic area law. Phys. Rev. B 80, 235127 (2009).

    ADS  Google Scholar 

  34. Evenbly, G. & Vidal, G. Strongly Correlated Systems: Numerical Methods (eds Avella, A. & Mancini, F.) Ch. 4 (Springer, 2013).

  35. Evenbly, G. & Vidal, G. Class of highly entangled many-body states that can be efficiently simulated. Phys. Rev. Lett. 112, 240502 (2014).

    ADS  Google Scholar 

  36. Zwolak, M. & Vidal, G. Mixed-state dynamics in one-dimensional quantum lattice systems: a time-dependent superoperator renormalization algorithm. Phys. Rev. Lett. 93, 207205 (2004).

    ADS  Google Scholar 

  37. Czarnik, P. & Dziarmaga, J. Variational approach to projected entangled pair states at finite temperature. Phys. Rev. B 92, 035152 (2015).

    ADS  Google Scholar 

  38. Verstraete, F., Garcia-Ripoll, J. J. & Cirac, J. I. Matrix product density operators: simulation of finite-temperature and dissipative systems. Phys. Rev. Lett. 93, 207204 (2004).

    ADS  Google Scholar 

  39. De las Cuevas, G., Schuch, N., Pérez-García, D. & Cirac, J. I. Purifications of multipartite states: limitations and constructive methods. New J. Phys. 15, 123021 (2013).

    Google Scholar 

  40. Verstraete, F. & Cirac, I. Continuous matrix product states for quantum fields. Phys. Rev. Lett. 104, 190405 (2010).

    ADS  MathSciNet  Google Scholar 

  41. Haegeman, J., Osborne, T. J., Verschelde, H. & Verstraete, F. Entanglement renormalization for quantum fields in real space. Phys. Rev. Lett. 110, 100402 (2013).

    ADS  Google Scholar 

  42. Brockt, C. & Haegeman, J. & Jennings, D. & Osborne, T. J. & Verstraete, F. The continuum limit of a tensor network: a path integral representation. Preprint at https://arxiv.org/abs/1210.5401 (2012).

  43. Schollwöck, U. The density-matrix renormalization group. Rev. Mod. Phys. 77, 259 (2005).

    ADS  MathSciNet  MATH  Google Scholar 

  44. Schollwöck, U. The density-matrix renormalization group in the age of matrix product states. Ann. Phys. 326, 96 (2011).

    ADS  MathSciNet  MATH  Google Scholar 

  45. McCulloch, I. P. Infinite size density matrix renormalization group, revisited. Preprint at https://arxiv.org/abs/0804.2509 (2008).

  46. Crosswhite, G. M., Doherty, A. C. & Vidal, G. Applying matrix product operators to model systems with long-range interactions. Phys. Rev. B 78, 035116 (2008).

    ADS  Google Scholar 

  47. Porras, D., Verstraete, F. & Cirac, J. I. Density matrix renormalization group and periodic boundary conditions: a quantum information perspective. Phys. Rev. Lett. 93, 227205 (2004).

    ADS  Google Scholar 

  48. Porras, D., Verstraete, F. & Cirac, J. I. Renormalization algorithm for the calculation of spectra of interacting quantum systems. Phys. Rev. B 73, 014410 (2006).

    ADS  Google Scholar 

  49. Chepiga, N. & Mila, F. Excitation spectrum and density matrix renormalization group iterations. Phys. Rev. B 96, 054425 (2017).

    ADS  Google Scholar 

  50. Evenbly, G. & Vidal, G. Algorithms for entanglement renormalization. Phys. Rev. B 79, 144108 (2009).

    ADS  Google Scholar 

  51. Haegeman, J., Osborne, T. J. & Verstraete, F. Post-matrix product state methods: to tangent space and beyond. Phys. Rev. B 88, 075133 (2013).

    ADS  Google Scholar 

  52. Vanderstraeten, L. & Haegeman, J. & Verstraete, F. Tangent-space methods for uniform matrix product states. Preprint at https://arxiv.org/abs/1810.07006 (2018).

  53. Haegeman, J. et al. Time-dependent variational principle for quantum lattices. Phys. Rev. Lett. 107, 070601 (2011).

    ADS  Google Scholar 

  54. Haegeman, J. et al. Variational matrix product ansatz for dispersion relations. Phys. Rev. B 85, 100408(R) (2012).

    ADS  Google Scholar 

  55. Zauner-Stauber, V., Vanderstraeten, L., Fishman, M. T., Verstraete, F. & Haegeman, J. Variational optimization algorithms for uniform matrix product states. Phys. Rev. B 97, 045145 (2018).

    ADS  Google Scholar 

  56. Bañuls, M. C., Hastings, M. B., Verstraete, F. & Cirac, J. I. Matrix product states for dynamical simulation of infinite chains. Phys. Rev. Lett. 102, 240603 (2009).

    ADS  Google Scholar 

  57. Daley, A. J. & Kollath, C. & Schollwöck, U. & Vidal, G. Time-dependent density-matrix renormalization-group using adaptive effective Hilbert spaces. J. Stat. Mech. P04005 (2004).

  58. Pollock, F. A., Rodriguez-Rosario, C., Frauenheim, T., Paternostro, M. & Modi, K. Non-Markovian quantum processes: Complete framework and efficient characterization. Phys. Rev. A 97, 012127 (2018).

    ADS  Google Scholar 

  59. Luchnikov, I. A., Vintskevich, S. V., Ouerdane, H. & Filippov, S. N. Simulation complexity of open quantum dynamics:connection with tensor networks. Phys. Rev. Lett. 122, 160401 (2019).

    ADS  Google Scholar 

  60. Jordan, J., Orús, R., Vidal, G., Verstraete, F. & Cirac, J. I. Classical simulation of infinite-size quantum lattice systems in two spatial dimensions. Phys. Rev. Lett. 101, 250602 (2008). Paper on infinite PEPS for 2D systems.

    ADS  Google Scholar 

  61. Jordan, J., Orús, R. & Vidal, G. Numerical study of the hard-core Bose–Hubbard model on an infinite square lattice. Phys. Rev. B 79, 174515 (2009).

    ADS  Google Scholar 

  62. Nishino, T. & Okunishi, K. Corner transfer matrix renormalization group method. J. Phys. Soc. Jpn 65, 891–894 (1996).

    ADS  MATH  Google Scholar 

  63. Orús, R. & Vidal, G. Simulation of two-dimensional quantum systems on an infinite lattice revisited: corner transfer matrix for tensor contraction. Phys. Rev. B 80, 094403 (2009). Paper on corner transfer matrices in the context of iPEPS.

    ADS  Google Scholar 

  64. Orús, R. Exploring corner transfer matrices and corner tensors for the classical simulation of quantum lattice systems. Phys. Rev. B 85, 205117 (2012).

    ADS  Google Scholar 

  65. Vanderstraeten, L., Mariën, M., Verstraete, F. & Haegeman, J. Excitations and the tangent space of projected entangled-pair states. Phys. Rev. B 92, 201111 (2015).

    ADS  Google Scholar 

  66. Levin, M. & Nave, C. P. Tensor renormalization group approach to two-dimensional classical lattice models. Phys. Rev. Lett. 99, 120601 (2007).

    ADS  Google Scholar 

  67. Xie, Z. Y., Jiang, H. C., Chen, Q. N., Weng, Z. Y. & Xiang, T. Second renormalization of tensor-network states. Phys. Rev. Lett. 103, 160601 (2009).

    ADS  Google Scholar 

  68. Xie, Z. Y. et al. Coarse-graining renormalization by higher-order singular value decomposition. Phys. Rev. B 86, 045139 (2012).

    ADS  Google Scholar 

  69. Gu, Z.-C. & Wen, X.-G. Tensor-entanglement-filtering renormalization approach and symmetry-protected topological order. Phys. Rev. B 80, 155131 (2009).

    ADS  Google Scholar 

  70. Evenbly, G. & Vidal, G. Tensor network renormalization. Phys. Rev. Lett. 115, 180405 (2015).

    ADS  MathSciNet  Google Scholar 

  71. Yang, S., Gu, Z.-C. & Wen, X.-G. Loop optimization for tensor network renormalization. Phys. Rev. Lett. 118, 110504 (2017).

    ADS  Google Scholar 

  72. Bal, M., Mariën, M., Haegeman, J. & Verstraete, F. Renormalization group flows of Hamiltonians using tensor networks. Phys. Rev. Lett. 118, 250602 (2017).

    ADS  MathSciNet  Google Scholar 

  73. Zhao, H.-H., Xie, Z.-Y., Xiang, T. & Imada, M. Tensor network algorithm by coarse-graining tensor renormalization on finite periodic lattices. Phys. Rev. B 93, 125115 (2016).

    ADS  Google Scholar 

  74. Xie, Z. Y. et al. Optimized contraction scheme for tensor-network states. Phys. Rev. B 96, 045128 (2017).

    ADS  Google Scholar 

  75. Stoudenmire, E. M. & White, S. R. Studying two dimensional systems with the density matrix renormalization group. Ann. Rev. Cond. Matt. Phys. 3, 111–128 (2012).

    Google Scholar 

  76. White, S. R. & Scalapino, D. J. Ground states of the doped four-leg t–J ladder. Phys. Rev. B 55, R14701 (1997).

    ADS  Google Scholar 

  77. White, S. R. & Scalapino, D. J. Density matrix renormalization group study of the striped phase in the 2D t–J model. Phys. Rev. Lett. 80, 1272 (1998).

    ADS  Google Scholar 

  78. Jiang, H. C., Weng, Z. Y. & Sheng, D. N. Density matrix renormalization group numerical study of the kagome antiferromagnet. Phys. Rev. Lett. 101, 117203 (2008).

    ADS  Google Scholar 

  79. Yan, S., Huse, D. A. & White, S. Spin liquid ground state of the S = 1/2 kagome Heisenberg model. Science 332, 1173 (2011).

    ADS  Google Scholar 

  80. Depenbrock, S., McCulloch, I. P. & Schollwöck, U. Nature of the spin-liquid ground state of the S = 1/2 Heisenberg model on the kagome lattice. Phys. Rev. Lett. 109, 067201 (2012).

    ADS  Google Scholar 

  81. Ehlers, G., White, S. R. & Noack, R. M. Hybrid-space density matrix renormalization group study of the doped two-dimensional Hubbard model. Phys. Rev. B 95, 125125 (2017).

    ADS  Google Scholar 

  82. Tagliacozzo, L. & Vidal, G. Entanglement renormalization and gauge symmetry. Phys. Rev. B 83, 115127 (2011).

    ADS  Google Scholar 

  83. Murg, V., Verstraete, F. & Cirac, J. I. Variational study of hard-core bosons in a two-dimensional optical lattice using projected entangled pair states. Phys. Rev. A 75, 033605 (2007).

    ADS  Google Scholar 

  84. Vanderstraeten, L., Haegeman, J., Corboz, P. & Verstraete, F. Gradient methods for variational optimization of projected entangled-pair states. Phys. Rev. B 94, 155123 (2016).

    ADS  Google Scholar 

  85. Corboz, P. Variational optimization with infinite projected entangled-pair states. Phys. Rev. B 94, 035133 (2016).

    ADS  Google Scholar 

  86. Jiang, H. C., Weng, Z. Y. & Xiang, T. Accurate determination of tensor network state of quantum lattice models in two dimensions. Phys. Rev. Lett. 101, 090603 (2008). Simple update scheme for iPEPS algorithms.

    ADS  Google Scholar 

  87. Phien, H. N., Bengua, J. A., Tuan, H. D., Corboz, P. & Orús, R. Infinite projected entangled pair states algorithm improved: fast full update and gauge fixing. Phys. Rev. B 92, 035142 (2015).

    ADS  Google Scholar 

  88. Vanderstraeten, L., Haegeman, J. & Verstraete, F. Phys. Rev. B 99, 165121 (2019).

    ADS  Google Scholar 

  89. Corboz, P. Improved energy extrapolation with infinite projected entangled-pair states applied to the two-dimensional Hubbard model. Phys. Rev. B 93, 045116 (2016).

    ADS  Google Scholar 

  90. Jahromi, S. S. & Orús, R. A universal tensor network algorithm for any infinite lattice. Phys. Rev. B 99, 195105 (2019).

    ADS  Google Scholar 

  91. Evenbly, G. & Vidal, G. Entanglement renormalization in two spatial dimensions. Phys. Rev. Lett. 102, 180406 (2009).

    ADS  Google Scholar 

  92. Evenbly, G. & Vidal, G. Frustrated antiferromagnets with entanglement renormalization: ground state of the spin-1/2 Heisenberg model on a kagome lattice. Phys. Rev. Lett. 104, 187203 (2010).

    ADS  Google Scholar 

  93. Corboz, P., Evenbly, G., Verstraete, F. & Vidal, G. Simulation of interacting fermions with entanglement renormalization. Phys. Rev. A 81, 010303(R) (2010).

    ADS  Google Scholar 

  94. Corboz, P. & Vidal, G. Fermionic multiscale entanglement renormalization ansatz. Phys. Rev. B 80, 165129 (2009).

    ADS  Google Scholar 

  95. Sandvik, A. W. & Vidal, G. Variational quantum Monte Carlo simulations with tensor-network states. Phys. Rev. Lett. 99, 220602 (2007).

    ADS  Google Scholar 

  96. Schuch, N., Wolf, M. M., Verstraete, F. & Cirac, J. I. Simulation of quantum many-body systems with strings of operators and Monte Carlo tensor contractions. Phys. Rev. Lett. 100, 040501 (2008).

    ADS  Google Scholar 

  97. Lubasch, M. et al. Systematic construction of density functionals based on matrix product state computations. New J. Phys. 18, 083039 (2016).

    ADS  Google Scholar 

  98. Wolf, F. A., McCulloch, I. P., Parcollet, O. & Schollwöck, U. Chebyshev matrix product state impurity solver for dynamical mean-field theory. Phys. Rev. B 90, 115124 (2014).

    ADS  Google Scholar 

  99. Evenbly, G. & White, S. R. Entanglement renormalization and wavelets. Phys. Rev. Lett. 116, 140403 (2016).

    ADS  MathSciNet  Google Scholar 

  100. Evenbly, G. & White, S. R. Representation and design of wavelets using unitary circuits. Phys. Rev. A 97, 052314 (2018).

    ADS  Google Scholar 

  101. Haegeman, J. et al. Rigorous free-fermion entanglement renormalization from wavelet theory. Phys. Rev. X 8, 011003 (2018).

    Google Scholar 

  102. Sahin, S., Schmidt, K. P. & Orús, R. Entanglement continuous unitary transformations. Eur. Lett. 117, 20002 (2017).

    ADS  Google Scholar 

  103. Wegner, F. Flow equations for Hamiltonians. Ann. Phys. 3, 77 (1994).

    MATH  Google Scholar 

  104. Vanderstraeten, L. et al. Bridging perturbative expansions with tensor networks. Phys. Rev. Lett. 119, 070401 (2017).

    ADS  MathSciNet  Google Scholar 

  105. Halko, N., Martinsson, P. G. & Tropp, J. A. Finding structure with randomness: probabilistic algorithms for constructing approximate matrix decompositions. SIAM Rev. 53, 217 (2011).

    MathSciNet  MATH  Google Scholar 

  106. Tamascelli, D., Rosenbach, R. & Plenio, M. B. Improved scaling of time-evolving block-decimation algorithm through reduced-rank randomized singular value decomposition. Phys. Rev. E 91, 063306 (2015).

    ADS  Google Scholar 

  107. Huang, R.-Z. et al. Generalized Lanczos method for systematic optimization of tensor network states. Preprint at https://arxiv.org/abs/1611.09574 (2016).

  108. Murg, V., Korepin, V. E. & Verstraete, F. Algebraic Bethe ansatz and tensor networks. Phys. Rev. B 86, 045125 (2012).

    ADS  Google Scholar 

  109. Ferris, A. J. Fourier transform for fermionic systems and the spectral tensor network. Phys. Rev. Lett. 113, 010401 (2014).

    ADS  Google Scholar 

  110. Rams, M. M., Zauner, V., Bal, M., Haegeman, J. & Verstraete, F. Truncating an exact matrix product state for the XY model: transfer matrix and its renormalization. Phys. Rev. B 92, 235150 (2015).

    ADS  Google Scholar 

  111. Schmoll, P. & Orús, R. Kitaev honeycomb tensor networks: exact unitary circuits and applications. Phys. Rev. B 95, 045112 (2017).

    ADS  Google Scholar 

  112. McCulloch, I. & Gulacsi, M. The non-Abelian density matrix renormalization group algorithm. Eur. Phys. Lett. 57, 852 (2002).

    ADS  Google Scholar 

  113. Singh, S., Pfeifer, R. N. C. & Vidal, G. Tensor network states and algorithms in the presence of a global U(1) symmetry. Phys. Rev. B 83, 115125 (2011).

    ADS  Google Scholar 

  114. Bauer, B., Corboz, P., Orús, R. & Troyer, M. Implementing global Abelian symmetries in projected entangled-pair state algorithms. Phys. Rev. B 83, 125106 (2011).

    ADS  Google Scholar 

  115. Liu, T., Li, W., Weichselbaum, A., von Delft, J. & Su, G. Simplex valence-bond crystal in the spin-1 kagome Heisenberg antiferromagnet. Phys. Rev. B 91, 060403 (2015).

    ADS  Google Scholar 

  116. Weichselbaum, A. Non-Abelian symmetries in tensor networks: a quantum symmetry space approach. Ann. Phys. 327, 2972–3047 (2012).

    ADS  MathSciNet  MATH  Google Scholar 

  117. Schmoll, P. & Singh, S. & Rizzi, M. & Orús, R. A programming guide for tensor networks with global SU(2) symmetry. Preprint at https://arxiv.org/abs/1809.08180 (2018).

  118. Penrose, R. Angular Momentum: An Approach to Combinatorial Space-time (http://math.ucr.edu/home/baez/penrose/) (1971).

  119. Cornwell, J. F. Group Theory in Physics (Academic, 1997).

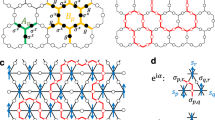
  120. Corboz, P., Orús, R., Bauer, B. & Vidal, G. Simulation of strongly correlated fermions in two spatial dimensions with fermionic projected entangled-pair states. Phys. Rev. B 81, 165104 (2010).

    ADS  Google Scholar 

  121. Kraus, C. V., Schuch, N., Verstraete, F. & Cirac, J. I. Fermionic projected entangled pair states. Phys. Rev. A 81, 052338 (2010).

    ADS  MathSciNet  Google Scholar 

  122. Pizorn, I. & Verstraete, F. Fermionic implementation of projected entangled pair states algorithm. Phys. Rev. B 81, 245110 (2010).

    ADS  Google Scholar 

  123. Barthel, T., Pineda, C. & Eisert, J. Contraction of fermionic operator circuits and the simulation of strongly correlated fermions. Phys. Rev. A 80, 042333 (2009).

    ADS  Google Scholar 

  124. Orús, R. Advances on tensor network theory: symmetries, fermions, entanglement, and holography. Eur. Phys. J. B 87, 280 (2014).

    ADS  Google Scholar 

  125. Tagliacozzo, L., Celi, A. & Lewenstein, M. Tensor networks for lattice gauge theories with continuous groups. Phys. Rev. X 4, 041024 (2014).

    Google Scholar 

  126. Buyens, B., Haegeman, J., Van Acoleyen, K., Verschelde, H. & Verstraete, F. Matrix product states for gauge field theories. Phys. Rev. Lett. 113, 091601 (2014).

    ADS  Google Scholar 

  127. Sugihara, T. Matrix product representation of gauge invariant states in a Bbb Z2 lattice gauge theory. J. High Energy Phys. 7, 022 (2005).

    ADS  Google Scholar 

  128. Byrnes, T. M. R., Sriganesh, P., Bursill, R. J. & Hamer, C. J. Density matrix renormalisation group approach to the massive Schwinger model. Nucl. Phys. B 109, 202 (2002).

    MATH  Google Scholar 

  129. Banuls, M. C. & Cichy, K. & Cirac, J. I. & Jansen, K. & Saito, H. Matrix product states for lattice field theories. PoS (LATTICE 2013) 332 (2013).

  130. Saito, H. & Banuls, M. C. & Cichy, K. & Cirac, J. I. & Jansen, K. The temperature dependence of the chiral condensate in the Schwinger model with matrix product states. Preprint at https://arxiv.org/abs/1412.0596 (2014).

  131. Banuls, M. C., Cichy, K., Jansen, K. & Cirac, J. I. The mass spectrum of the Schwinger model with matrix product states. JHEP 11, 158 (2013).

    ADS  Google Scholar 

  132. Buyens, B. & Haegeman, J. & Hebenstreit, F. & Verstraete, F. & Van Acoleyen, K. Real-time simulation of the Schwinger effect with matrix product states. Phys. Rev. D 96, 114501 (2017)

  133. Buyens, B. & Montangero, S. & Haegeman, J. & Verstraete, F. & Van Acoleyen, K. Finite-representation approximation of lattice gauge theories at the continuum limit with tensor networks. Phys. Rev. D 95, 094509 (2017).

  134. Banuls, M. C., Cichy, K., Cirac, J. I. & Kühn, S. Density induced phase transitions in the Schwinger model: a study with matrix product states. Phys. Rev. Lett. 118, 071601 (2017).

    ADS  Google Scholar 

  135. Zapp, K. & Orús, R. Tensor network simulation of QED on infinite lattices: learning from (1+1)d, and prospects for (2+1)d. Phys. Rev. D. 95, 114508 (2017).

    ADS  MathSciNet  Google Scholar 

  136. Buyens, B., Haegeman, J., Verschelde, H., Verstraete, F. & Van Acoleyen, K. Confinement and string breaking for QED2 in the Hamiltonian Picture. Phys. Rev. X 6, 041040 (2016).

    Google Scholar 

  137. Pilcher, T., Dalmonte, M., Rico, E., Zoller, P. & Montangero, S. Real-time dynamics in U(1) lattice gauge theories with tensor networks. Phys. Rev. X 6, 011023 (2016).

    Google Scholar 

  138. Kühn, S., Zohar, E., Cirac, J. I. & Banuls, M. C. Non-Abelian string breaking phenomena with matrix product states. JHEP 07, 130 (2015).

    ADS  MathSciNet  MATH  Google Scholar 

  139. Silvi, P., Rico, E., Dalmonte, Tschirsich, F. & Montangero, S. Finite-density phase diagram of a (1+1)d non-Abelian lattice gauge theory with tensor networks. Quantum 1, 9 (2017).

    Google Scholar 

  140. Zohar, E. & Burrello, M. & Wahl, T. B. & Cirac, J. I. Fermionic projected entangled pair states and local U(1) gauge theories. Ann. Phys. 363, 385–439 (2015).

  141. Zohar, E., Burrello, M., Wahl, T. B. & Cirac, J. I. Projected entangled pair states with non-Abelian gauge symmetries: an SU(2) study. Ann. Phys. 374, 84–137 (2016).

    ADS  MathSciNet  MATH  Google Scholar 

  142. Levin, M. A. & Wen, X.-G. String-net condensation: a physical mechanism for topological phases. Phys. Rev. B 71, 045110 (2005).

    ADS  Google Scholar 

  143. Aguado, M. & Vidal, G. Entanglement renormalization and topological order. Phys. Rev. Lett. 100, 070404 (2008).

    ADS  Google Scholar 

  144. Dusuel, S., Kamfor, M., Orús, R., Schmidt, K. P. & Vidal, J. Robustness of a perturbed topological phase. Phys. Rev. Lett. 106, 107203 (2011).

    ADS  Google Scholar 

  145. Zaletel, M. P., Mong, R. S. K. & Pollmann, F. Topological characterization of fractional quantum Hall ground states from microscopic Hamiltonians. Phys. Rev. Lett. 110, 236801 (2013).

    ADS  Google Scholar 

  146. Pollmann, F., Turner, A., Berg, E. & Oshikawa, M. Entanglement spectrum of a topological phase in one dimension. Phys. Rev. B 81, 064439 (2010).

    ADS  Google Scholar 

  147. Estienne, B., Papic, Z., Regnault, N. & Bernevig, B. A. Matrix product states for trial quantum Hall states. Phys. Rev. B 87, 161112(R) (2013).

    ADS  Google Scholar 

  148. Pfeifer, R. N. C. et al. Simulation of anyons with tensor network algorithms. Phys. Rev. B 82, 115126 (2010).

    ADS  Google Scholar 

  149. Bultinck, N. et al. Anyons and matrix product operator algebras. Ann. Phys. 378, 183–233 (2017).

    ADS  MathSciNet  MATH  Google Scholar 

  150. Williamson, D. J. & Bultinck, N. & Verstraete, F. Symmetry-enriched topological order in tensor networks: defects, gauging and anyon condensation. Preprint at https://arxiv.org/abs/1711.07982 (2017).

  151. Wen, X.-G. Colloquium: Zoo of quantum-topological phases of matter. Rev. Mod. Phys. 89, 41004 (2017).

    MathSciNet  Google Scholar 

  152. Chen, X., Gu, Z.-C. & Wen, X.-G. Classification of gapped symmetric phases in one-dimensional spin systems. Phys. Rev. B 83, 035107 (2011).

    ADS  Google Scholar 

  153. Schuch, N., Pérez-García, D. & Cirac, J. I. Classifying quantum phases using matrix product states and projected entangled pair states. Phys. Rev. B 84, 165139 (2011).

    ADS  Google Scholar 

  154. Buerschaper, O. Twisted injectivity in projected entangled pair states and the classification of quantum phases. Ann. Phys. 351, 447–476 (2014).

    ADS  MathSciNet  MATH  Google Scholar 

  155. Williamson, D. J. & Bultinck, N. & Haegeman, J. & Verstraete, F. Fermionic matrix product operators and topological phases of matter. Preprint at https://arxiv.org/abs/1609.02897 (2016).

  156. Bultinck, N., Williamson, D. J., Haegeman, J. & Verstraete, F. Fermionic projected entangled-pair states and topological phases. J. Phys. A 51, 025202 (2017).

    ADS  MathSciNet  MATH  Google Scholar 

  157. Bultinck, N., Williamson, D. J., Haegeman, J. & Verstraete, F. Fermionic matrix product states and one-dimensional topological phases. Phys. Rev. B 95, 075108 (2017).

    ADS  Google Scholar 

  158. Li, H. & Haldane, F. D. M. Entanglement spectrum as a generalization of entanglement entropy: identification of topological order in non-Abelian fractional quantum Hall effect states. Phys. Rev. Lett. 101, 010504 (2008).

    ADS  Google Scholar 

  159. Cirac, J. I., Poilblanc, D., Schuch, N. & Verstraete, F. Entanglement spectrum and boundary theories with projected entangled-pair states. Phys. Rev. B 83, 245134 (2011).

    ADS  Google Scholar 

  160. Haferkamp, J. & Hangleiter, D. & Eisert, J. & Gluza, M. Contracting projected entangled pair states is average-case hard. Preprint at https://arxiv.org/abs/1810.00738 (2018).

  161. Kastoryano, M. J. & Lucia, A. & Pérez-García, D. Locality at the boundary implies gap in the bulk for 2D PEPS. Preprint at https://arxiv.org/abs/1709.07691 (2017).

  162. Maldecena, J. M. The large N limit of superconformal field theories and supergravity. Adv. Theor. Math. Phys. 2, 231 (1998).

    ADS  MathSciNet  Google Scholar 

  163. Evenbly, G. & Vidal, G. Tensor network states and geometry. J. Stat. Phys. 145, 891–918 (2011).

    ADS  MathSciNet  MATH  Google Scholar 

  164. Swingle, B. Constructing holographic spacetimes using entanglement renormalization. Preprint at https://arxiv.org/abs/1209.3304 (2012).

  165. Nozaki, M., Ryu, S. & Takayanagi, T. Holographic geometry of entanglement renormalization in quantum field theories. JHEP 10, 193 (2012).

    ADS  MathSciNet  MATH  Google Scholar 

  166. Pastawski, F., Yoshida, B., Harlow, D. & Preskill, J. Holographic quantum error-correcting codes: toy models for the bulk/boundary correspondence. J. High Energy Phys. 06, 149 (2015).

    ADS  MathSciNet  MATH  Google Scholar 

  167. Bény, C. Causal structure of the entanglement renormalization ansatz. New J. Phys. 15, 023020 (2013).

    ADS  MathSciNet  Google Scholar 

  168. Milsted, A. & Vidal, G. Geometric interpretation of the multi-scale entanglement renormalization ansatz. Preprint at https://arxiv.org/abs/1812.00529 (2018).

  169. Chen, J., Cheng, S., Xie, H., Wang, L. & Xiang, T. Equivalence of restricted Boltzmann machines and tensor network states. Phys. Rev. B 97, 085104 (2018).

    ADS  Google Scholar 

  170. Glasser, I., Pancotti, N., August, M., Rodriguez, I. D. & Cirac, J. I. Neural-network quantum states, string-bond states, and chiral topological states. Phys. Rev. X 8, 011006 (2018).

    Google Scholar 

  171. Stoudenmire, E. M. & Schwab, D. J. Supervised learning with quantum-inspired tensor networks. Adv. Neur. Inf. Proc. Syst. 29, 4799 (2016).

    Google Scholar 

  172. Stoudenmire, E. M. Learning relevant features of data with multi-scale tensor networks. Quant. Sci. Tech. 3, 034003 (2018).

    Google Scholar 

  173. Liu, D. et al. Machine learning by unitary tensor network of hierarchical tree structure. Preprint at https://arxiv.org/abs/1710.04833 (2017).

  174. Han, Z.-Y., Wang, J., Fan, H., Wang, L. & Zhang, P. Unsupervised generative modeling using matrix product states. Phys. Rev. X 8, 031012 (2018).

    Google Scholar 

  175. Huggins, W. & Patel, P. & Whaley, K. B. & Stoudenmire, E. M. Towards quantum machine learning with tensor networks. Preprint at https://arxiv.org/abs/1803.11537 (2018).

  176. Glasser, I. & Pancotti, N. & Cirac, J. I. Supervised learning with generalized tensor networks. Preprint at https://arxiv.org/abs/1806.05964 (2018).

  177. Gállego, A. J. & Orús, R. Language design as information renormalization. Preprint at https://arxiv.org/abs/1708.01525 (2017).

  178. Corboz, P., White, S. R., Vidal, G. & Troyer, M. Stripes in the two-dimensional t–J model with infinite projected entangled-pair states. Phys. Rev. B 84, 041108 (2011).

    ADS  Google Scholar 

  179. Xie, Z. Y. et al. Tensor renormalization of quantum many-body systems using projected entangled simplex states. Phys. Rev. X 4, 011025 (2014).

    Google Scholar 

  180. Picot, T., Ziegler, M., Orús, R. & Poilblanc, D. Spin-S kagome quantum antiferromagnets in a field with tensor networks. Phys. Rev. B 93, 060407 (2016).

    ADS  Google Scholar 

  181. Corboz, P., Laeuchli, A. M., Penc, K., Troyer, M. & Mila, F. Simultaneous dimerization and SU(4) symmetry breaking of 4-color fermions on the square lattice. Phys. Rev. Lett. 107, 215301 (2011).

    ADS  Google Scholar 

  182. Czech, B. et al. Tensor network quotient takes the vacuum to the thermal state. Phys. Rev. B 94, 085101 (2016).

    ADS  Google Scholar 

  183. Hauru, M., Evenbly, G., Ho, W. W., Gaiotto, D. & Vidal, G. Topological conformal defects with tensor networks. Phys. Rev. B 94, 115125 (2016).

    ADS  Google Scholar 

  184. Hu, Q. & Vidal, G. Spacetime symmetries and conformal data in the continuous multiscale entanglement renormalization ansatz. Phys. Rev. Lett. 119, 010603 (2017).

    ADS  MathSciNet  Google Scholar 

  185. Milsted, A. & Vidal, G. Tensor networks as conformal transformations. Preprint at https://arxiv.org/abs/1805.12524 (2018).

  186. Milsted, A. & Vidal, G. Tensor networks as path integral geometry. Preprint at https://arxiv.org/abs/1807.02501 (2018).

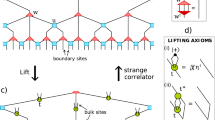
  187. Bal, M. et al. Mapping topological to conformal field theories through strange correlators. Preprint at https://arxiv.org/abs/1801.05959 (2018).

  188. Chan, G. K.-L. et al. An introduction to the density matrix renormalization group ansatz in quantum chemistry. Preprint at https://arxiv.org/abs/0711.1398 (2007).

  189. Krumnow, C., Veis, L., Legeza, Ö. & Eisert, J. Fermionic orbital optimization in tensor network states. Phys. Rev. Lett. 117, 210402 (2016).

    ADS  Google Scholar 

  190. Szalay, S. et al. Tensor product methods and entanglement optimization for ab initio quantum chemistry. Int. J. Quant. Chem. 115, 1342 (2015).

    Google Scholar 

  191. Paredes, B., Verstraete, F. & Cirac, J. I. Exploiting quantum parallelism to simulate quantum random many-body systems. Phys. Rev. Lett. 95, 140501 (2005).

    ADS  Google Scholar 

  192. Chandran, A., Carrasquilla, J., Kim, I. H., Abanin, D. A. & Vidal, G. Spectral tensor networks for many-body localization. Phys. Rev. B 92, 024201 (2015).

    ADS  Google Scholar 

  193. Pollmann, F., Khemani, V., Cirac, J. I. & Sondhi, S. L. Efficient variational diagonalization of fully many-body localized Hamiltonians. Phys. Rev. B 94, 041116 (2016).

    ADS  Google Scholar 

  194. Wahl, T. B., Pal, A. & Simon, S. H. Efficient representation of fully many-body localized systems using tensor networks. Phys. Rev. X 7, 021018 (2017).

    Google Scholar 

  195. Wahl, T. B. Tensor networks demonstrate the robustness of localization and symmetry-protected topological phases. Phys. Rev. B 98, 054204 (2018).

    ADS  Google Scholar 

  196. Ryu, S. & Takayanagi, T. Holographic derivation of entanglement entropy from the anti–de Sitter space/conformal field theory correspondence. Phys. Rev. Lett. 96, 181602 (2006).

    ADS  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The author acknowledged financial support from Ikerbasque, Donostia International Physics Center (DIPC) and Deutsche Forschungsgemeinschaft (DFG), as well as discussions over the years with many people on topics presented here. The author also acknowledges M. Rizzi and P. Schmoll for critical reading.

Author information

Authors and Affiliations

  1. Donostia International Physics Center, San Sebastián, Spain

    Román Orús

  2. Ikerbasque Foundation for Science, Bilbao, Spain

    Román Orús

Authors
  1. Román Orús
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Román Orús.

Ethics declarations

Competing interests

The author declares no competing interests.

Additional information

Publisher’s note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary information

Glossary

Renormalization

The process of removing degrees of freedom that are not relevant to describe a complex system at different scales of some physical variable (energy, length…).

Anti-de Sitter

(AdS). A geometric space with negative curvature.

Conformal field theory

(CFT). A quantum field theory with conformal symmetry, which includes scale invariance. Low-energy field theories of quantum critical systems are usually CFTs.

Topological order

A type of order in quantum matter entirely due to global entanglement properties and which does not exist classically. Other characterizations: excitations are anyonic, the topological entanglement entropy is non-zero, ground states are topologically degenerate, and reduced density matrices are locally equivalent.

Area-law

Property by which the entanglement entropy of a region scales proportionally to the size of the boundary of the region.

Tensor contraction

Sum over the common indices of a set of tensors (for example, matrix multiplication).

Correlation length

Non-mathematically, this is the length scale at which correlations are sizeable in a many-body system.

Wilsonian renormalization

In this context, a renormalization scheme by which different length scales are obtained purely by removing short-distance degrees of freedom, without having previously disentangled them. In many cases, this is done by removing high-energy (and/or high-momenta) degrees of freedom.

Trotter decomposition

Decomposition of the exponential of the sum of two matrices A and B as \({{\rm{e}}}^{(A+B)t}=\mathop{{\rm{lim}}}\limits_{n\to \infty }{({{\rm{e}}}^{At/n}{{\rm{e}}}^{Bt/n})}^{n}\), with t some real parameter.

Daubechies wavelets

Family of orthogonal wavelets defining a discrete wavelet transform and characterized by a maximal number of vanishing moments for some given support.

Wegner’s flow

Flow of continuous unitary transformations that diagonalizes a Hamiltonian, as \({H}_{{\rm{D}}}=\mathop{{\rm{lim}}}\limits_{l\to \infty }{U}^{\dagger }(l)HU(l)\) with l the flow parameter and HD the diagonal Hamiltonian.

Lanczos methods

An adaptation of a power method to find the eigenvalues and eigenvectors of a matrix.

Clebsch–Gordan coefficient

Coefficient of the change of basis in angular momentum, from a tensor product basis to a coupled basis (for instance, from spins \(1/2\otimes 1/2\) to spins \(0\oplus 1\).

SWAP gate

Unitary gate that swaps the quantum states of two physical systems.

Parent Hamiltonian

Hamiltonian that has a given PEPS or MPS as unique ground state.

Boltzmann machine

A specific type of neural network in which the target is to reproduce some Gibbs thermal probabilities.

MERGE

Linguistic operation introduced by Noam Chomsky, which picks up two entities (for example, noun and adjective) and produces a new one from the two (for instance, noun phrase).

Many-body localization

(MBL). Property of interacting quantum many-body systems with disorder leading to a phase of matter that does not self-thermalize.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Orús, R. Tensor networks for complex quantum systems. Nat Rev Phys 1, 538–550 (2019). https://doi.org/10.1038/s42254-019-0086-7

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/s42254-019-0086-7

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing