Introduction

Weyl semimetals and their classical analogues feature two-fold linear band crossings in three-dimensional (3D) momentum space, known as Weyl points1,2,3,4,5, analogous to the Dirac points in two-dimensional (2D) momentum space. These Weyl points act as monopoles of Berry flux and carry topological chiral charges defined by the Chern number. Consequently, the 2D Fermi-arc surface states are formed, connecting the projections of two oppositely charged Weyl points, according to the celebrated bulk-boundary correspondence. On the other hand, the recent discovery of higher-order topological phases has revolutionized the study of topological matters6,7,8,9,10,11,12,13,14,15,16. The higher-order topological phases give rise to unconventional bulk-boundary correspondence as they host boundary states in at least two dimensions lower than the bulk, in contrast to the previous first-order topological phases, where the topological boundary states living in just one dimension lower than the bulk.

Higher-order topology can also be incorporated into Weyl semimetals, resulting in higher-order Weyl semimetals that possess simultaneously chiral Fermi-arc surface states in two dimensions and Fermi-arc hinge states in one dimension17,18,19,20,21,22. These states stem from the chiral charge and the higher-order charge of Weyl points, respectively, and bridge the projections of Weyl points in multiple dimensions, thereby revealing the dimensional hierarchy of higher-order topological physics. In sharp contrast to the extensive study of higher-order topology in insulators, their Weyl semimetal counterparts have been severely lagged behind, with only a handful of experimental demonstrations in phononics19,20,22 and circuits21, where the couplings can be engineered flexibly to implement discrete lattice models. Nevertheless, the higher-order Weyl semimetal phases in photonics remain uncharted territory, both theoretically and experimentally, due to the inherent challenge of discretely modeling most photonic systems, particularly those in three dimensions.

Our work reports on the experimental discovery of a higher-order Weyl photonic crystal (PhC). It differs from the previously achieved higher-order topological Weyl semimetal phases19,20,21,22 in following three ways. First, the newly proposed higher-order Weyl points separate the 3D Brillouin zone (BZ) into the Chern insulator phase and the generalized real Chern insulator phase23,24, as opposed to the previously discovered higher-order Weyl points that separate the BZ into the Chern insulator phase and a higher-order topological phase with quadrupole or dipole bulk polarizations19,20,21,22. Particularly, the kz = 0 slice can be viewed as a previously overlooked real Chern insulator, belonging to the Stiefel-Whitney class with real Bloch wavefunctions, which is fundamentally different from the Chern class with complex Bloch wavefunctions25. We thus coin the proposed Weyl points “real higher-order Weyl points”. Second, the real higher-order Weyl points are achieved through a symmetry-enforced topological phase transition process, where a three-fold spin-1 Weyl point26 splits into two real higher-order Weyl points. Though inspired by a tight-binding model, our design principle is symmetry-guided, beyond the conventional lattice models that unfavorable to many physical systems, as exemplified by the PhCs (see Supplementary Information Note 3 and Fig. S4). Third, our work provides the first example of higher-order photonic Weyl points, manifesting the dimensional hierarchy of higher-order topological physics in a 3D PhC. All previous photonic Weyl points were limited to the first-order27,28,29,30,31,32,33. Interestingly, our Weyl points are isolated from other trivial bands, representing a higher-order version of the ideal Weyl points31,33,34. The realization of ideal higher-order photonic Weyl points paves a way toward the robust manipulation of the flow of light across multiple dimensions, including 2D surfaces and 1D hinges, in a single integrable 3D platform.

Results

Design of the real higher-order Weyl PhC

The 3D cubic unit cell of the PhC with lattice constant a = 15 mm is displayed in Fig. 1a. Each unit cell contains four junctions, at (x, y, z), (−x + 0.5a, −y, z + 0.5a), (−x, y + 0.5a, −z + 0.5a), (x + 0.5a, −y + 0.5a, −z), respectively, where x = y = 0.3a, z = 0.2a. Each junction connects to four neighboring junctions via square perfect electric conductor (PEC) rods with two different widths w1 = 3.5 mm (green rods), w2 = 4.5 mm (red rods); the rest of the volume is filled with free space. The resulting 3D PhC has the non-centrosymmetric space group P212121 (No. 19) with three two-fold screw symmetries, S2x:= (x, y, z) → (x + 0.5a, −y + 0.5a, −z), S2y:= (x, y, z) → (−x, y + 0.5a, −z + 0.5a), and S2z:= (x, y, z) → (−x + 0.5a, −y, z + 0.5a).

Fig. 1: 3D real higher-order Weyl PhC.
figure 1

a Unit cell of the 3D PhC. The red and green regions represent square perfect electric conductor (PEC) rods with width w1 = 4.5 mm and w2 = 3.5 mm, respectively. b 3D Brillouin zone of the PhC. c 3D Band structure of the PhC. d 2D band structure in the vicinity of the real higher-order Weyl point. e 2D band structure in the vicinity of the charge-2 Dirac point. f Chern number C and generalized real Chern number vR in the projected 2D subsystems parameterized by kz. g Band structures of the 2D projected subsystems with kz = 0, 0.5 π/a, 0.75 π/a, and π/a, respectively. The C2z eigenvalues at Γ, Y, S and X of the four lowest bands are marked. The positive and negative sign represents the C2z eigenvalue of +1 and −1, respectively.

Full size image

The band structure of the 3D PhC has been numerically calculated, as depicted in Fig. 1b, c, d, e, g. The analysis reveals two ideal real higher-order two-fold Weyl points at k = (0, 0, ±kwp) (illustrated as yellow dots), and an ideal charge-2 four-fold 3D Dirac point at R (indicated by red dots), which are isolated from the other trivial bands31,33. Note that, the pair of Weyl points can move along the kz axis, by tuning the geometric parameters but preserving the lattice symmetry; for the convenience of measurement, kwp = 0.5 π/a in our experiments. Besides, the resulting 3D Dirac point carries a chiral charge +226, which is a direct sum of two identical Weyl points, in stark contrast to the conventional 3D Dirac points, which is a sum of two oppositely charged Weyl points35,36. Interestingly, there exist three nodal surfaces on the kx = π/a, ky = π/a, and kz = π/a planes, carrying a vanishing chiral charge, in contrast to the previously demonstrated nodal surface with a chiral charge 237,38.

The two real higher-order Weyl points divide the 3D BZ into three sections parametrized by kz. As depicted in Fig. 1f, each section can be viewed as a kz-dependent 2D phase characterized by the Chern number

$${C}_{n}=\frac{1}{2\pi }{\int }_{\!\!\!\!\!BZ}{\varOmega }_{n,xy}{d}^{2}k$$
(1)

where \({\varOmega }_{n,xy}\) is Berry curvature and k is the wavevector. Via the first-principle calculation as well as the symmetry indicator analysis shown in Fig. 1g, we can prove that the |kz| < kwp planes possess a vanishing Chern number 0, while the kz > kwp (kz < −kwp) planes have a Chern number +1 (−1) (see Supplementary Information Note 8 and Fig. S9). The higher-order Weyl points as the transition point, thus, carry a chiral charge +1. Consequently, within the |kz| > kwp region, the chiral edge states emerge in the 2D subsystems, corresponding to the chiral surface Fermi arcs connecting the projections of the higher-order Weyl points and the Dirac point in the 3D system.

Though the 2D subsystems between two Weyl points are topologically trivial in terms of the Chern number, it is topologically nontrivial in terms of the generalized real Chern number (vR) that characterizes the higher-order topology. This generalized real Chern number is well defined in the C2z-invariant system with a vanishing Chern number and calculated from the C2z eigenvalues at C2z-invariant momenta

$${(-1)}^{{\nu }_{R}}=\mathop{\prod }\limits_{i=1}^{4}{(-1)}^{[{N}_{occ}^{-}({\varGamma }_{{{{{{\rm{i}}}}}}})/2]}$$
(2)

where Γi denotes the four C2z-invariant momenta at Γ, X, Y and S, and \({N}_{occ}^{-}({\varGamma }_{i})\) is the number of occupied bands with negative inversion eigenvalues at Γi, with the bracket denoting the greatest integer function. The C2z eigenvalues are calculated from the TB models (see Supplementary Information Note 1 and Table. S1). The C2z eigenvalues of the lowest four bands at Γ, X, Y and S are displayed in Fig. 1g, from which a nontrivial vR can be obtained for the |kz| <  kwp plane. Particularly, the kz = 0 slice has the C2zT symmetry (the combination of two-fold rotation and time-reversal symmetry) that enforces the bands to be real23,24,25, as opposite to the complex bands in the previous higher-order topological insulator phases6,7,11,13,14,15,16. Such a higher-order topological phase is known as the real Chern insulator23,24, belonging to the previously overlooked Stiefel-Whitney class25. Though at the kz ≠ 0 planes, the bands are not real, the higher-order topology is still determined by the generalized real Chern number, we thus term all the |kz| < kwp planes as the generalized real Chern insulator (see Supplementary Information Note 8 and Fig. S9). The nontrivial generalized real Cheren number in the |kz| < kwp region gives rise to the corner states in the 2D subsystems, corresponding to the 1D hinge Fermi arcs protected by the C2z symmetry in the 3D system. Interestingly, the |kz| > kwp planes also host first-order topological index, i.e., nontrivial Zak phase, resulting in the floating surface states at the (100) and (010) surfaces (see Supplementary Information Note 9 and Fig. S10).

Note that the current structure is transformed from the PhC with the space group No. 198 that has three C2 screw symmetry along the x, y, z axis, and a C3 rotational symmetry along the <111> axis. The original PhC hosts the symmetry-enforced spin-1 Weyl point and the charge-2 Dirac point. Interestingly, the C3,111 symmetry is crucial for the spin-1 Weyl point, but it is not necessary for the existence of the charge-2 Dirac point. By breaking the C3,111 symmetry, the spin-1 Weyl point splits into two real higher-order Weyl points, while the charge-2 Dirac point persists; the corresponding space group is reduced to No. 19 (see Supplementary Information Note 3 and Fig. S4).

Experimental demonstration of the real higher-order Weyl points

As depicted in Fig. 2a, b, the 3D metallic PhC can be fabricated directly from AlSi10Mg (acting approximately as PEC at microwave frequencies, see Supplementary Information Note 10 and Fig. S11) via additive manufacturing techniques, owing to its self-supporting structure. The holes on the vertical surfaces of the sample allow us to insert the probe into the sample to measure the field distributions. To determine the bulk band structure, we conduct microwave pump-probe measurements on the sample. As illustrated in Fig. 2c, the source is vertically inserted into the middle of the sample to excite the bulk modes, and the probe is horizontally inserted into the sample to measure the complex field distribution along a vertical plane situated 15 mm away from the source. After performing 2D Fourier transform to the measured real-space field distribution, we obtain the bulk band structure projected onto the ky-kz plane, as indicated in Fig. 2d. The results, presented in Fig. 2e, reveal that the real higher-order Weyl point is projected onto the middle of the \(\overline{\varGamma }-\overline{Z}\) path at 10.9 GHz, and the charge-2 Dirac point is projected onto \(\overline{{{{{{\rm{M}}}}}}}\) at 11.3 GHz. The measurement results are in good agreement with the simulated projected bulk dispersion shown in Fig. 2f.

Fig. 2: Projected bulk dispersion of the real higher-order Weyl points.
figure 2

a, b Photographs of the fabricated 3D PhC. c Experimental setup. The field distributions are measured along the vertical plane in the middle of the sample. d Schematic view of the projected 2D surface BZ. e Measured projected bulk dispersion. The color bar indicates the energy intensity. f Simulated projected bulk dispersion.

Full size image

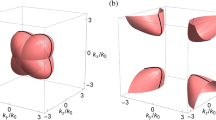
Next, we perform experiments to measure the topological surface Fermi arcs from the real higher-order Weyl points on the (100) surface. The experimental setup configuration is depicted in Fig. 3a. All vertical surfaces of the sample are covered with a 0.75 mm thick metallic layer, acting as PEC boundaries. A source is positioned at the center of the surface to excite the surface modes. Upon Fourier-transforming the measured field distributions from real space to reciprocal space, we acquire the surface dispersion depicted in Fig. 3b, c, e. The insert in Fig. 3b displays the momentum space distribution corresponding to the filed pattern shown in Fig. 3a. The two open arcs are the photonic Fermi arcs connecting the projections of the charge-2 3D Dirac point and the real higher-order Weyl points. The measured surface dispersion along the high symmetry line \(\overline{\varGamma }-\overline{{{{{{\rm{Z}}}}}}}-\overline{{{{{{\rm{M}}}}}}}-\overline{{{{{{\rm{Y}}}}}}}-\overline{\varGamma }\) is shown in Fig. 3c, demonstrating excellent consistence with the simulated surface dispersion (red lines) depicted in Fig. 3d. In addition, we present the measured two-dimensional surface isofrequency contours at frequencies ranging from 10.9 to 11.9 GHz in Fig. 3e, corroborating the outstanding concurrence with the simulated outcomes in Fig. 3f. Apart from the Fermi arc surface states, the topological floating surface states protected by nontrivial Zak phase in the |kz| < kwp plane also exist on the surface, as shown in Fig. 3c, d, along \(\overline{{{{{{\rm{Y}}}}}}}-\overline{\varGamma }\) and \(\overline{\varGamma }-\overline{{{{{{\rm{Z}}}}}}}\). These floating surface states coexist with the Fermi arc surface states.

Fig. 3: Surface Fermi arcs from the real higher-order Weyl points.
figure 3

a Experimental setup. The field distributions are measured on the (100) sample surface covered by a metallic layer. b Schematic of the 2D surface BZ. The right inset shows measured results obtained by Fourier transforming the field pattern measured in (a). c Measured surface dispersions along high-symmetry lines. d Simulated surface dispersions along high-symmetry lines. e Measured surface isofrequency contours from 10.9 to 11.9 GHz. The color map measures the energy intensity. f Simulated surface isofrequency contours from 10.9 to 11.9 GHz.

Full size image

Finally, we experimentally characterize the topological hinge Fermi arc originating from the real higher-order Weyl points. A hinge between (100) and (110) surfaces is selected, by analyzing the Wannier centers (see Supplementary Information Note 1 and Fig. S3). To excite the hinge modes, a source is placed at the middle of the hinge. Subsequently, we employ a probe to scan the field distributions along the hinge. The experimental setup and the measured field distributions on the two surfaces adjacent to the hinge is shown in Fig. 4a. The field is predominantly localized on the sample’s hinge, signifying the presence of topological hinge states. The measured hinge dispersion along the kz direction is obtained through Fourier transformation, as presented in Fig. 4b, which shows excellent agreement with the simulated predictions depicted in Fig. 4d (red line). To thoroughly and clearly illustrate the characteristics of our sample, in which the real higher-order Weyl points separate the BZ zone into the generalized real Chern insulator and the Chern insulator in the kz direction, we further plot the measured energy intensity for varying kz in a small area around the hinge of the sample, as shown in Fig. 4c. By exciting both the hinge and surface modes, it is evident that the hinge Fermi arcs manifest themselves well for |kz| < 0.5 π/a, connecting the projection of the two Weyl points.

Fig. 4: Hinge Fermi arc from the real higher-order Weyl points.
figure 4

a Measured field distributions of the two surfaces adjacent to the hinge at 10.7 GHz. b Measured projected hinge dispersion along the kz direction. c Measured energy intensity for varying kz in a small area around the hinge at 10.7 GHz, with 5, 5, and 20 unit cells in the x, y, and z direction respectively. d Simulated projected hinge dispersion along the kz direction.

Full size image

Discussion

We have thus accomplished successfully the experimental realization of an ideal real higher-order Weyl PhC exhibiting simultaneously one-dimensional (1D) hinge Fermi arcs, 2D surface Fermi arcs, and 2D floating surface states, originating from the nontrivial generalized real Chern number, Chern number, and Zak phase, respectively. The distinct topological origins of the boundary states pave a way toward the robust manipulation of the flow of light and creation of photonic devices across multiple dimensions, including the 2D surfaces and the 1D hinges, in a single integrable 3D PhC. Besides, our work provides the experimental evidences of the charge-2 3D Dirac point and the 2D nodal surface with the vanishing chiral charge, neither of which has been realized previously in photonics. Finally, our findings broaden our understanding of the higher-order Weyl semimetals and the Stiefel-Whitney class of topological phases, and establish an ideal photonic platform to explore exotic physical phenomena related to higher-order Weyl points, such as chiral anomaly, pseudo-gauge fields, and fractional charges.

Methods

Numerical simulations

All simulations are performed using the COMSOL Multiphysics software package. The metallic material of the 3D PhC is considered as PEC, and the rest volume is air. To calculate the bulk dispersion of the unit cell, periodic boundary conditions are applied in all three spatial directions. To calculate the surface dispersion, we consider a super cell composed of 15 unit cells; Periodic boundary conditions are imposed in the y and z directions, and PEC boundary conditions in the x direction. For the hinge dispersion, the supercell has 11 by 11 unit cells; Periodic boundary conditions are imposed in the z direction, and PEC boundary conditions in the x and y directions. In simulation, the size of the system has been varied to confirm convergence (see Supplementary Information Note 11, Figs. S12 and S13).

Experiment

The sample is fabricated via 3D metal printing. The material is AlSi10Mg, with high conductivity at microwave frequencies. Notably, AlSi10Mg at microwave frequencies has a negligible metallic loss, which can be regarded approximately as PEC (see Supplementary Information Note 10 and Fig. S11). In the measurements, the amplitude and phase of the fields are collected by a vector network analyzer (VNA). The VNA is connected to two electric dipole antennas, serving as the source and the probe, respectively. To excite the bulk states, the source is vertically inserted into the middle of the sample, and the probe is horizontally inserted into the sample to measure the field distribution along a vertical plane 15 mm away from the source. To excite the surface states, A source is placed at the center of the surface; the distance between the source and the measured plane is about 11 mm. For the hinge states, the source is placed at the middle of the hinge, and the probe is moved along the hinge to scan the field.