Introduction

When two identical particles adiabatically exchange their positions ri=1,2, their final state (up to dynamical phase) is related to the initial one through an exchange statistics phase θ*,

with θ*=0 (π) for bosons (fermions)1.

Anyons2,3,4 are quasiparticles in two dimensions, not belonging to the two classes of elementary particles, bosons and fermions. Abelian anyons appear in the fractional quantum Hall (FQH) system of filling factor ν=1/(2n+1), n=1,2,⋯. They carry a fraction e*=νe of the electron charge e and obey fractional exchange statistics, satisfying equation (1) with θ*=±πν. Two anyons gain a phase ±2πν from a braiding, whereby one winds around the other; ± depends on the winding direction. Although fractional charges have been detected5,6,7,8, experimental measurement of statistics phase πν has been so far elusive. Existing theoretical proposals for the measurement involve quantities inaccessible in current experiments or suffer from unintended change of a proposed setup with external parameters9,10,11,12,13,14,15,16,17,18.

In many-body quantum theory1, Feynman diagrams are used to compute the expectation value of observables. This approach invokes vacuum bubble diagrams, which describe virtual particles excited from vacuum and self-annihilating without interacting with real particles. According to the linked cluster theorem1, each diagram possessing vacuum bubbles comes with, hence is exactly cancelled by, a partner diagram of the same magnitude but of the opposite sign. Consequently, vacuum bubbles do not contribute to physical observables.

In the following, we demonstrate that this common wisdom has to be revised for anyons: a certain class of vacuum bubbles of Abelian anyons does affect observables. These virtual particles, which we call topological vacuum bubbles, wind around a real anyonic excitation, gaining the braiding phase ±2πν. We propose a realistic setup for detecting them and θ*=πν.

Results

Topological vacuum bubble

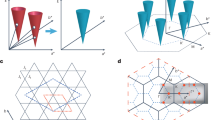
We illustrate topological vacuum bubbles. In Fig. 1a, a Feynman diagram represents interference between processes a1 and a2 of propagation of a real particle. In a1, a virtual particle-hole pair is excited then self-annihilates after the virtual particle winds around the real particle, forming a vacuum bubble, while it is not excited in a2. The winding results in a braiding phase 2πν and an Aharonov–Bohm phase from the magnetic flux Φ enclosed by the winding path, contributing to the interference signal as is the anyon flux quantum9,19.

Figure 1: Topological vacuum bubble.
figure 1

Feynman diagrams for interference involving a real particle and a virtual particle–hole excitation from vacuum. Full (empty) circles represent particles (holes). Solid (dashed) lines denote propagations of real (virtual) particles. (a) Diagram for the interference of two processes: (a1, blue) A real particle propagates, a virtual particle–hole pair is excited, then the pair self-annihilates after the virtual particle winds around the real one. (a2, magenta) A real particle propagates. The entire virtual process constitutes a vacuum bubble. For anyons, the bubble gains a topological braiding phase 2πν from the winding. (b) Partner diagram of a. Here a virtual particle, constituting another bubble, does not encircle a real one and hence gains no braiding phase. The diagrams in a and b contribute to observables for anyons, while they do not for bosons and fermions.

Full size image

The limiting cases of bosons (ν=0) and fermions (ν=1) imply that this bubble diagram appears together with, and is cancelled by, a partner diagram in Fig. 1b. The partner diagram has a bubble not encircling the real particle and involves only . The two diagrams (and their complex conjugates) yield

For bosons and fermions, the two diagrams fully cancel each other with sin(πν)=0 in agreement with the linked cluster theorem; hence, the signal disappears. By contrast, for anyons they cancel only partially, producing the non-vanishing interference in an observable, and are topological as the braiding phase is involved.

Interferometer setup

In Fig. 2a, we propose a minimal setup for observing topological vacuum bubbles. It is a Fabry–Perot interferometer9,17,20,21,22,23 in the ν=1/(2n+1) FQH regime, coupled to an additional edge channel (Edge 1) via a quantum point contact (QPC1). At QPCi, there occurs tunnelling of a single anyon (rather than anyon bunching), fulfilled24 with ; γi is the tunnelling strength and T is the temperature. Gate voltage VG is applied, to change the interferometer loop enclosing Aharonov–Bohm flux Φ. The interference part of charge current at drain D3 is measured with bias voltage V applied to source S1; the other Si’s and Di’s are grounded. Together with ‘virtual’ (thermal) anyon excitations in the interferometer, a voltage-biased ‘real’ anyon, dilutely injected at QPC1 from Edge 1 to the interferometer, forms topological vacuum bubbles, as shown below. The bubbles contribute to at the leading order in QPC tunnelling, as Edges 2 and 3 are unbiased. It is noteworthy that in the setups previously studied9,10,11,12,13,14,15,16,17,18, topological bubbles do not contribute to current at the leading order.

Figure 2: Interferometry for detecting topological vacuum bubbles.
figure 2

(a) In the setup, anyons move (see arrows) along FQH edge channel i=1,2,3 that connects source Si and drain Di, and jump (dashed) between the channels via tunnelling at QPCs. The loop defined by Edges i=2,3, QPC2 and QPC3 encloses magnetic flux Φ, forming a Fabry–Perot interferometer. Distance between QPC2 and QPC3 (QPC1) is L (d). (b) Two interfering paths (i) and (ii) of each main interference process at . Following Fig. 1, filled (empty) circles represent particle-like (hole-like) anyons and solid (dashed) lines denote propagation of an anyon injected from S1 (anyon pair excitation at QPCs). Type II and III processes involve a topological vacuum bubble.

Full size image

We consider the regime of , where the size LV≡ℏvp/(e*V) of the dilutely injected anyons is much smaller than interferometer size L and the injection of hole-like anyons at QPC1 is ignored; vp is anyon velocity along the edges and e*V should be much smaller than the FQH energy gap. Because of the dilute injection and , anyon braiding is well defined in the interferometer. As shown below, the dependence of on Φ or on VG provides a clear signature of the topological bubbles, consequently, θ*=πν in both of the pure Aharonov–Bohm regime (where Coulomb interaction of the edge channels with bulk anyons localized inside the interferometer loop is negligible) and the Coulomb-dominated regime (where the interaction is strong)22,25. Below, we first ignore bulk anyons.

Interference current

Employing the chiral Luttinger liquid theory26,27 for FQH edges and Keldysh Green’s functions10,12, we compute at the leading order in γ. There are four types of the processes mainly contributing to ; see Fig. 2b. For , we obtain the analytical expression of : The interference current contributed by Type I-1 processes is , which by Type I-2 is , and those by Type II and III are

where is a thermal suppression factor, thermal length LT≡ℏvp/(πνkBT) and ; see Methods and the Supplementary Note 3.

Type I-1 processes describe interference between two paths of an anyon moving from S1 to D3 via (i) QPC2 and (ii) QPC3, respectively. They were previously studied9.

In Type I-2, an anyon injected from S1 interferes with a particle-like anyon excited at a QPC or annihilates a hole-like anyon; particle-like and hole-like anyons are pairwise excited thermally at QPCs. For example, consider the two following interfering histories: (i) an anyon is injected from S1 to D2, an anyon pair is excited at QPC3 and then the particle-like (hole-like) anyon of the pair moves to D3 (D2). (ii) An anyon is injected from S1 to D3 via QPC2 without any excitations. The hole-like anyon annihilates the injected anyon on Edge 2 in history (i) and the particle-like anyon of (i) interferes with the injected anyon of (ii) on Edge 3. The sum of such interference processes yields . The sin2πν factor appears, because relative locations of anyons on Edge 2 or 3 differ between the processes, leading to an exchange phase ±πν, and because a process with an excitation (of a particle-like anyon moving to D2 and a hole-like one to D3) yields charge current in the opposite direction to another with its particle-hole conjugated excitation (of a particle to D3 and a hole to D2).

In Types II and III, a real anyon injected from S1 moves to D2 and a virtual anyon pair excited at QPC2 interferes with another at QPC3. The interference path effectively encloses the real anyon, forming a topological vacuum bubble (cf. Fig. 1). In Type II, when the real anyon is located on Edge 2 between QPC2 and QPC3, a virtual pair is excited at QPC2 in history (i) and at QPC3 in history (ii). Next, the hole-like (particle-like) anyon of each pair moves, for example, to D2 (D3). The interference of the two histories corresponds to the winding of a virtual anyon around the real one and Φ, forming a topological bubble with interference phase ; ±depends on whether the hole-like anyon moves to D2 or D3. In the interference, the winding of a virtual anyon around the real one effectively occurs through the exchanges of the positions of the anyons in each of Edges 2 and 3, as relative locations of anyons on Edges 2 and 3 differ between (i) and (ii) (see Supplementary Fig. 2 and Supplementary Note 6). This interference is accompanied by a partner process. The latter has a bubble that winds around Φ (gaining phase ), but not around a real anyon. The two partner processes partially cancel each other, yielding ; the remaining sin πν factor in equations (3) and (4) has a similar origin to the sin2 πν factor of .

In Type III, the two interfering histories are as follows: (i) a virtual pair is excited at QPC2 before a real anyon injected from S1 arrives at QPC2 and (ii) another pair is excited at QPC3 after the real one arrives at QPC3. The ensuing chronological sequence on Edge 2 is opposite to Type II: an anyon excited at QPC2 arrives at QPC3; the real one arrives at QPC3; a pair is excited at QPC3. The resulting topological bubble effectively winds around the real anyon in the direction opposite to its winding around Φ, yielding a phase . Partial cancellation of the bubble and its partner leads to . The factor 2L+CLT of (CLT in ) in equation (3) (equation (4)) comes from the time window compatible with the chronological sequence on Edge 2.

Type II and III processes of topological bubbles do not affect any observables at ν=1 (fermions), due to full cancellation between partner bubbles (the linked cluster theorem). They are distinct from I-2. I-2 processes produce, for example, non-vanishing current noise at ν=1, as the particle-hole conjugated excitations (mentioned before) equally contribute to the noise (although the contributions of the conjugations to cancel each other, leading to ).

In the more general regime of , we employ the parametrization

The phase θ is determined by competition between the various contributions to and contains information about statistics phase πν. At , is much larger than and dominates , because the interfering anyon of Types I-1 and I-2 is voltage biased and has width LV∝V−1 much narrower than the thermal anyon excitations (whose width LT∝T−1) of II and III, showing much weaker interference. From and , we find

The arctan 2LV/LT term represents an error in the braiding phase 2πν of the topological bubbles. It occurs when the size LV of a real anyon is not sufficiently smaller than the winding radius of a virtual anyon around the real one. It is negligible at , as the radius is effectively large when ; those corresponding to the error are ignored in equations (3) and (4), and are shown in Supplementary Note 3. For , dominates and θ→πν. Remarkably, θ depends on T, contrary to common practice in electron interferometry28.

Coulomb-dominated regime

In experimental situations of a Fabry–Perot interferometer in the FQH regime, it is expected that there exist bulk anyons localized inside the interferometer loop. There are two regimes of Fabry–Perot interferometers, the pure Aharonov–Bohm regime and the Coulomb-dominated regime. In the former regime, Coulomb interaction between the bulk anyons and the edge of the interferometer is negligible, whereas it is crucial in the latter25. The Fabry–Perot interferometers of recent experiments17,20,21,22,23 in the FQH regime are in the Coulomb-dominated regime. Below we compute the interference current in the presence of the Coulomb interaction and show that equation (6) is applicable to both of the pure Aharonov–Bohm regime and the Coulomb-dominated regime.

For , we numerically compute in Fig. 3, combining our theory with the capacitive interaction model25 that successfully describes thermally fluctuating bulk anyons and the interaction (see the Method and Supplementary Note 5). We find the gate-voltage dependence of ∝cos(2πVG/VG,0+θ) with periodicity VG,0 in the Coulomb-dominated regime and in the pure Aharonov–Bohm limit; here the periodicity of the Φ dependence is Φ0≡h/e rather the period of equation (5), because of the fluctuation of the number of bulk anyons9,19. In both the regimes, the interference processes discussed before (Fig. 2) appear in the same manner; hence, θ satisfies the analytic expression in equation (6) (cf. Fig. 3c).

Figure 3: Detection of anyon phase πν from interference phase shift θ.
figure 3

(a) Dependence of (blue, normalized) and (cyan, normalized) on Φ in the pure Aharonov–Bohm regime and (b) their dependence on VG in the Coulomb dominated regime. We choose ν=1/3, T=30 mK, e*V=45 μeV, L=3 μm and vp=104 m s−1 (L/LT=1.2 and L/LV=20); see Supplementary Note 5 for the Coulomb interaction parameter of the regimes. For these parameters, the phase shift θ between and is 0.9πν. (c) Dependence of θ on T. The same parameters (except T) with (a) and (b) are chosen. θ→πν as T increases (yet ). In both the pure Aharonov–Bohm regime and the Coulomb-dominated regime, the same numerical result (blue curve) of θ(T), which agrees with equation (6) (magenta), is obtained.

Full size image

How to measure the phase θ

Experimental measurements of θ can be affected by possible side effects, including the external-parameter (magnetic fields, gate voltages and bias voltages) dependence of the size, shape, QPC tunnelling and bulk anyon excitations. Below we propose how to detect θ with avoiding the side effects, using the setup in Fig. 2a.

The phase θ is experimentally measurable, by comparing with a reference current . is measured at D3 in the same setup under the same external parameters (temperature, gate voltages, magnetic field and so on) with , but with applying infinitesimal bias voltage Vref/2 to S2 and −Vref/2 to S3 and keeping S1 and all Di’s grounded9 (cf. Supplementary Note 4). In any regimes shows the same interference pattern with , but is phase-shifted from by θ; in the Coulomb-dominated (pure Aharonov–Bohm) limit. Importantly, the side effects modify and in the same manner; hence, the phase shift between the patterns remains as θ.

The fractional statistics phase is directly and unambiguously identifiable in experiments, by observing θ→πν at with excluding the side effects as above, or one applies the fit function of arctan [A1/(1+A2/T)] with fit parameters A1 and A2 to measured data of θ(T) and extracts arctan A1=πν from the fit (cf. the second line of equation (6)). Observation of θ=πν or θ(T) will suggest a strong evidence of anyon braiding and topological bubbles.

The parameters in Fig. 3 are experimentally accessible17,20,21,22,23. For the QPCs, there are constraints (i) that the number of voltage-biased anyons injected through QPC1 is at most one in the interferometer loop at any instance (to ensure that the braiding phase of a topological vacuum bubble is 2πν), (ii) that the anyon tunnelling probabilities at QPC2 and QPC3 are sufficiently small (to ensure that the double winding of an anyon along the interferometer loop is negligible) and (iii) that anyon tunnelling (rather than electron tunnelling) occurs at the QPCs. The constraint (i) is satisfied when the anyon tunnelling probability at QPC1 is <ℏvp/(2Le*V), which is ∼0.05 under the parameters. To achieve the constraints (ii) and (iii), each tunnelling probability of QPC2 and QPC3 is typically set to be 0.4 in experiments22,29; then the amplitude of the double winding is smaller than that of the single winding by the factor 0.4 exp(−2L/LT), which is ∼0.04 at 30 mK. With the constraints we estimate the amplitude of , which is 1.5 pA at 30 mK and 0.6 pA at 40 mK under the parameters. It is noteworthy that θ=0.9πν is reached at 30 mK, while θ=0.95πν at 40 mK under the parameters. The estimation is within a measurable range in experiments, where current  pA is well detectable30.

We remark that the above strategy of detecting the fractional statistics phase is equally applicable to the more general quantum Hall regime22 of filling factor ν′=ν+ν0, in which the edge channels from the integer filling ν0 are fully transmitted through the QPCs, while the channel from the fractional filling ν forms the interferometry in Fig. 2. For this case we compute and , and find that in both of the pure Aharonov–Bohm regime and the Coulomb-dominated regime the interference-pattern phase shift between them is identical to the phase θ of the ν0=0 case discussed in equation (6) and Fig. 3 (cf. Supplementary Note 5).

Certain anyonic vacuum bubbles involve topological braiding and affect physical observables surprisingly, contrary to vacuum bubbles of bosons and fermions. They can be detected with current experiment tools, which will provide an unambiguous evidence of anyonic fractional statistics. We expect that they are relevant also for other filling fractions ν=p/(2np+1), non-Abelian anyons17,21 and topological quantum computation setups31.

Methods

Hamiltonian for the interferometer

We present the Hamiltonian for the setup. We recall the chiral Luttinger liquid theory for FQH edges.

The Hamiltonian H=∑iHedge,i+Htun for the interferometer in Fig. 2a consists of Hedge,i for edge channel i and Htun for anyon tunnelling at QPCs. Edge channel 1 is biased by V and its Hamiltonian, employing the bosonization26 for chiral Luttinger liquids, is given by

For the other unbiased channels, Here, e*>0, is the anyon number operator of channel i and φi(x) is the bosonic field of channel i at position x, which describes the plasmonic excitation of anyons. The tunnelling Hamiltonian is , is the operator from Edge channel 1 to 2 at QPC 1, from Edge 2 to 3 at QPC2 and from Edge 2 to 3 at QPC3. These are written as

where creates an (particle-like) anyon at position x and time t on Edge i, a is the short-length cutoff, γi is the tunnelling strength at QPC i (chosen as real) and the Aharonov–Bohm flux Φ enclosed by Edges i=2,3, QPC2 and QPC3 is attached to and , respectively, under certain gauge transformation; the dynamical phase common to the all edge channels is absorbed to . The Klein factor increases the number of anyons on Edge i by 1 and satisfies and .

The exchange rule in equation (1) is described by φi and Fi. On Edge i, it is satisfied by

The exchange rule between anyons on different edges is achieved with the commutators of Fi,

A conventional way32 for obtaining the commutators is to think of an extended edge connecting the different channel segments with no twist (cf. Fig. 4). The connection should preserve the chiral propagation direction of the channels. The exchange rule of anyons of the extended edge agrees with equations (11) and (12).

Figure 4: Extended edge channel scheme.
figure 4

It is obtained by connecting the edge channels of the setup in Fig. 2a. The connection is represented by dashed arcs, whereas anyon propagation direction and anyon tunnelling at QPCs are represented by arrows and dashed lines, respectively.

Full size image

We consider the regime of weak tunnelling of anyons and treat Htun as a perturbation on ∑iHedge,i. Perturbation theory is applicable24 in the renormalization group sense, when e*V and kBT are higher than Cγ1/(1−ν), C being a non-universal constant.

The current is expressed as . is decomposed, , into direct current and interference current depending on Φ (the leading-order contribution). Equations (3) and (4) are obtained by employing Keldysh Green’s function technique with semiclassical approximation (see Supplementary Fig. 1 and Supplementary Notes 1, 2 and 3).

Coulomb interaction

In the presence of Coulomb interaction between bulk anyons and edge channels, we compute , combining our chiral Luttinger liquid theory with the capacitive interaction model25 that successfully describes the Coulomb-dominated regime. The interferometer Hamiltonian H=∑iHedge,i+Htun is modified by the Coulomb interaction as

Here, is the number of the net charges localized within the interferometer bulk (inside the interference loop), Aarea is the area of the interferometer, NL is the net number of quasiparticles minus quasiholes and is the number of positive background charges induced by the gate voltage applied to the interferometer. Uint is the strength of Coulomb interaction between the charges of the interferometer edge and the charges localized in the interferometer bulk, and Ubulk is the strength of interaction between the bulk charges. In the second equality of equation (13), we introduce a boson field for each Edge i, , where K2(x)=1 for d<x<d+L, K3(x)=1 for 0<x<L and Ki(x)=0 otherwise. The second term of describes the charges induced per unit length by the interaction. In equation (13), the Hamiltonian is quadratic in and has . It is noteworthy that . The main interference signal and the reference signal are computed by taking ensemble average over the thermal fluctuations of NL (see Supplementary Note 5).

Additional information

How to cite this article: Han, C. et al. Topological vacuum bubbles by anyon braiding. Nat. Commun. 7:11131 doi: 10.1038/ncomms11131 (2016).