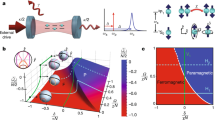
Abstract
Using cold atoms to simulate strongly interacting quantum systems is an exciting frontier of physics. However, because atoms are nominally neutral point particles, this limits the types of interaction that can be produced. We propose to use the powerful new platform of cold atoms trapped near nanophotonic systems to extend these limits, enabling a novel quantum material in which atomic spin degrees of freedom, motion and photons strongly couple over long distances. In this system, an atom trapped near a photonic crystal seeds a localized, tunable cavity mode around the atomic position. We find that this effective cavity facilitates interactions with other atoms within the cavity length, in a way that can be made robust against realistic imperfections. Finally, we show that such phenomena should be accessible using one-dimensional photonic crystal waveguides in which coupling to atoms has already been experimentally demonstrated.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Bloch, I., Dalibard, J. & Nascimbene, S. Quantum simulations with ultracold quantum gases. Nature Phys. 8, 267–276 (2012).
Batrouni, G. G., Scalettar, R. T., Zimanyi, G. T. & Kampf, A. P. Supersolids in the Bose–Hubbard Hamiltonian. Phys. Rev. Lett. 74, 2527–2530 (1995).
Wigner, E. On the interaction of electrons in metals. Phys. Rev. 46, 1002–1011 (1934).
Micheli, A., Brennen, G. K. & Zoller, P. A toolbox for lattice-spin models with polar molecules. Nature Phys. 2, 341–347 (2006).
Campa, A., Dauxois, T. & Ruffo, S. Statistical mechanics and dynamics of solvable models with long-range interactions. Phys. Rep. 480, 57–159 (2009).
Shahmoon, E., Mazets, I. & Kurizki, G. Non-additivity in laser-illuminated many-atom systems. Opt. Lett. 39, 3674–3677 (2014).
Hauke, P. & Tagliacozzo, L. Spread of correlations in long-range interacting quantum systems. Phys. Rev. Lett. 111, 207202 (2013).
Richerme, P. et al. Non-local propagation of correlations in quantum systems with long-range interactions. Nature 511, 198–201 (2014).
Jurcevic, P. et al. Quasiparticle engineering and entanglement propagation in a quantum many-body system. Nature 511, 202–205 (2014).
Lahaye, T., Menotti, C., Santos, L., Lewenstein, M. & Pfau, T. The physics of dipolar bosonic quantum gases. Rep. Prog. Phys. 72, 126401 (2009).
Griesmaier, A., Werner, J., Hensler, S., Stuhler, J. & Pfau, T. Bose–Einstein condensation of chromium. Phys. Rev. Lett. 94, 160401 (2005).
Lu, M., Burdick, N. Q., Youn, S. H. & Lev, B. L. Strongly dipolar Bose–Einstein condensate of dysprosium. Phys. Rev. Lett. 107, 190401 (2011).
Saffman, M., Walker, T. G. & Mølmer, K. Quantum information with Rydberg atoms. Rev. Mod. Phys. 82, 2313–2363 (2010).
Ni, K.-K. et al. A high phase-space-density gas of polar molecules. Science 322, 231–235 (2008).
Khitrova, G., Gibbs, H. M., Kira, M., Koch, S. W. & Scherer, A. Vacuum Rabi splitting in semiconductors. Nature Phys. 2, 81–90 (2006).
Vetsch, E. et al. Optical interface created by laser-cooled atoms trapped in the evanescent field surrounding an optical nanofiber. Phys. Rev. Lett. 104, 203603 (2010).
Goban, A. et al. Demonstration of a state-insensitive, compensated nanofiber trap. Phys. Rev. Lett. 109, 033603 (2012).
Thompson, J. D. et al. Coupling a single trapped atom to a nanoscale optical cavity. Science 340, 1202–1205 (2013).
Goban, A. et al. Atom–light interactions in photonic crystals. Nature Commun. 5, 3808 (2014).
Kimble, H. J. The quantum internet. Nature 453, 1023–1030 (2008).
Greentree, A. D., Tahan, C., Cole, J. H. & Hollenberg, L. C. L. Quantum phase transitions of light. Nature Phys. 2, 856–861 (2006).
Hartmann, M. J., Brandao, F. G. S. L. & Plenio, M. B. Strongly interacting polaritons in coupled arrays of cavities. Nature Phys. 2, 849–855 (2006).
Angelakis, D. G., Santos, M. F. & Bose, S. Photon-blockade-induced Mott transitions and xy spin models in coupled cavity arrays. Phys. Rev. A 76, 031805 (2007).
Joannopoulos, J. D., Johnson, S. G., Winn, J. N. & Meade, R. D. Photonic Crystals: Molding the Flow of Light 2nd edn (Princeton Univ. Press, 2008).
Kurizki, G. Two-atom resonant radiative coupling in photonic band structures. Phys. Rev. A 42, 2915–2924 (1990).
John, S. & Wang, J. Quantum optics of localized light in a photonic band gap. Phys. Rev. B 43, 12772–12789 (1991).
John, S. & Wang, J. Quantum electrodynamics near a photonic band gap: photon bound states and dressed atoms. Phys. Rev. Lett. 64, 2418–2421 (1990).
Bay, S., Lambropoulos, P. & Mølmer, K. Atom–atom interaction in strongly modified reservoirs. Phys. Rev. A 55, 1485–1496 (1997).
Lambropoulos, P., Nikolopoulos, G. M., Nielsen, T. R. & Bay, S. Fundamental quantum optics in structured reservoirs. Rep. Prog. Phys. 63, 455 (2000).
Shahmoon, E. & Kurizki, G. Nonradiative interaction and entanglement between distant atoms. Phys. Rev. A 87, 033831 (2013).
González-Tudela, A., Hung, C.-L., Chang, D. E., Cirac, J. I. & Kimble, H. J. Subwavelength vacuum lattices and atom–atom interactions in two-dimensional photonic crystals. Nature Photon. http://dx.doi.org/10.1038/nphoton.2015.54 (2015).
Yu, S.-P. et al. Nanowire photonic crystal waveguides for single-atom trapping and strong light–matter interactions. Appl. Phys. Lett. 104, 111103 (2014).
Agarwal, G., Gupta, S. & Puri, R. Fundamentals of Cavity Quantum Electrodynamics (World Scientific Publishing, 1995).
Plenio, M. B., Huelga, S. F., Beige, A. & Knight, P. L. Cavity-loss-induced generation of entangled atoms. Phys. Rev. A 59, 2468–2475 (1999).
Domokos, P. & Ritsch, H. Collective cooling and self-organization of atoms in a cavity. Phys. Rev. Lett. 89, 253003 (2002).
Black, A. T., Chan, H. W. & Vuletić, V. Observation of collective friction forces due to spatial self-organization of atoms: from Rayleigh to Bragg scattering. Phys. Rev. Lett. 91, 203001 (2003).
Baumann, K., Guerlin, C., Brennecke, F. & Esslinger, T. Dicke quantum phase transition with a superfluid gas in an optical cavity. Nature 464, 1301–1306 (2010).
Hung, C.-L., Meenehan, S. M., Chang, D. E., Painter, O. & Kimble, H. J. Trapped atoms in one-dimensional photonic crystals. New J. Phys. 15, 083026 (2013).
Markos, P. & Soukoulis, C. M. Wave Propagation: From Electrons to Photonic Crystals and Left-Handed Materials (Princeton Univ Press, 2010).
Porras, D. & Cirac, J. I. Effective quantum spin systems with trapped ions. Phys. Rev. Lett. 92, 207901 (2004).
Islam, R. et al. Emergence and frustration of magnetism with variable-range interactions in a quantum simulator. Science 340, 583–587 (2013).
Gross, M. & Haroche, S. Superradiance: an essay on the theory of collective spontaneous emission. Phys. Rep. 93, 301–396 (1982).
Mattioli, M., Dalmonte, M., Lechner, W. & Pupillo, G. Cluster Luttinger liquids of Rydberg-dressed atoms in optical lattices. Phys. Rev. Lett. 111, 165302 (2013).
Basko, D. M., Aleiner, I. L. & Altshuler, B. L. On the problem of many-body localization. Preprint at http://arXiv.org/abs/cond-mat/0602510 (2006).
Longo, P., Schmitteckert, P. & Busch, K. Few-photon transport in low-dimensional systems. Phys. Rev. A 83, 063828 (2011).
Firstenberg, O. et al. Attractive photons in a quantum nonlinear medium. Nature 502, 71–75 (2013).
Nayak, K. P. & Hakuta, K. Photonic crystal formation on optical nanofibers using femtosecond laser ablation technique. Opt. Express 21, 2480–2490 (2013).
Chang, D. E., Jiang, L., Gorshkov, A. V. & Kimble, H. J. Cavity QED with atomic mirrors. New J. Phys. 14, 063003 (2012).
Eichenfield, M., Chan, J., Camacho, R. M., Vahala, K. J. & Painter, O. Optomechanical crystals. Nature 462, 78–82 (2009).
Dung, H. T., Knöll, L. & Welsch, D.-G. Resonant dipole–dipole interaction in the presence of dispersing and absorbing surroundings. Phys. Rev. A 66, 063810 (2002).
Acknowledgements
The authors thank L. Tagliacozzo, P. Hauke, M. Lewenstein, A. González-Tudela, J.I. Cirac, L. Jiang, J. Preskill, O. Painter, M. Lukin, J. Thompson and S. Gopalakrishnan for discussions. This work was supported by Fundacio Privada Cellex Barcelona, the MINECO Ramon y Cajal Program, the Marie Curie Career Integration Grant, the IQIM, an NSF Physics Frontiers Center, the DoD NSSEFF programme, DARPA ORCHID, AFOSR QuMPASS MURI, NSF PHY-1205729, NSF PFC at the JQI, NSF PIF, ARO, AFOSR, ARL and AFOSR MURI on Ultracold Polar Molecules.
Author information
Authors and Affiliations
Contributions
J.S.D., H.H. and C.-L.H. performed the calculations. All authors contributed ideas. J.S.D. and D.E.C. wrote the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary information
Supplementary information (PDF 489 kb)
Rights and permissions
About this article
Cite this article
Douglas, J., Habibian, H., Hung, CL. et al. Quantum many-body models with cold atoms coupled to photonic crystals. Nature Photon 9, 326–331 (2015). https://doi.org/10.1038/nphoton.2015.57
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphoton.2015.57
This article is cited by
-
Light–matter interactions in quantum nanophotonic devices
Nature Reviews Physics (2024)
-
A diamond nanophotonic interface with an optically accessible deterministic electronuclear spin register
Nature Photonics (2024)
-
MoSe2/WS2 heterojunction photodiode integrated with a silicon nitride waveguide for near infrared light detection with high responsivity
Light: Science & Applications (2023)
-
Chimera patterns in conservative Hamiltonian systems and Bose–Einstein condensates of ultracold atoms
Scientific Reports (2023)
-
Mixed state behavior of Hermitian and non-Hermitian topological models with extended couplings
Scientific Reports (2023)