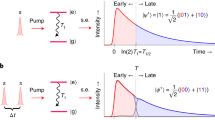
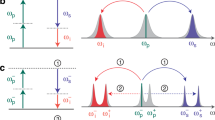
Abstract
Entanglement between quantum and classical objects is of special interest in the context of fundamental studies of quantum mechanics and potential applications for quantum information processing. In quantum optics, single photons are treated as light quanta while coherent states are considered the most classical of pure states. Recently, entanglement between a single photon and a coherent state in a free-travelling field was identified as a useful resource for optical quantum information processing. However, the extreme difficulty involved in generating such states was highlighted, as it requires clean cross-Kerr nonlinearities. Here, we devise and experimentally demonstrate a scheme to generate such hybrid entanglement by implementing a superposition of two distinct quantum operations. The generated states clearly show entanglement between the two different types of states. Our work opens the way to the generation of hybrid entanglement of greater size and the development of efficient quantum information processing using a new type of qubit.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Schrödinger, E. Die gegenwaertige Situation in der Quantenmechanik. Naturwissenschaften 23, 823–828 (1935).
Glauber, R. J. Coherent and incoherent states of the radiation field. Phys. Rev. 131, 2766–2788 (1963).
Zurek, W. H. Decoherence, einselection, and the quantum origins of the classical. Rev. Mod. Phys. 75, 715–775 (2003).
Lee, S.-W. & Jeong, H. Near-deterministic quantum teleportation and resource-efficient quantum computation using linear optics and hybrid qubits. Phys. Rev. A 87, 022326 (2013).
Kwon, H. & Jeong, H. Violation of the Bell–Clauser–Horne–Shimony–Holt inequality using imperfect photodetectors with optical hybrid states. Phys. Rev. A 88, 052127 (2013).
Bell, J. S. On the problem of hidden variables in quantum mechanics. Rev. Mod. Phys. 38, 447–452 (1966).
Pearle, P. M. Hidden-variable example based upon data rejection. Phys. Rev. D 2, 1418–1425 (1970).
Gerry, C. Generation of optical macroscopic quantum superposition states via state reduction with a Mach–Zehnder interferometer containing a Kerr medium. Phys. Rev. A 59, 4095–4098 (1999).
Nemoto, K. & Munro, W. J. Nearly deterministic linear optical controlled-NOT gate. Phys. Rev. Lett. 93, 250502 (2004).
Jeong, H. Using weak nonlinearity under decoherence for macroscopic entanglement generation and quantum computation. Phys. Rev. A 72, 034305 (2005).
Shapiro, J. H. Single-photon Kerr nonlinearities do not help quantum computation. Phys. Rev. A 73, 062305 (2006).
Shapiro, J. H. & Razavi, M. Continuous-time cross-phase modulation and quantum computation. New J. Phys. 9, 16 (2007).
Gea-Banacloche, J. Impossibility of large phase shifts via the giant Kerr effect with single-photon wave packets. Phys. Rev. A 81, 043823 (2010).
Kreis, K. & van Loock, P. Classifying, quantifying, and witnessing qudit–qumode hybrid entanglement. Phys. Rev. A 85, 032307 (2012).
Lee, C.-W. & Jeong, H. Quantification of macroscopic quantum superpositions within phase space. Phys. Rev. Lett. 106, 220401 (2011).
Ourjoumtsev, A., Jeong, H., Tualle-Brouri, R. & Grangier, P. Generation of optical ‘Schrödinger cats’ from photon number states. Nature 448, 784–786 (2007).
Afek, I., Ambar, O. & Silberberg, Y. High-NOON states by mixing quantum and classical light. Science 328, 879–881 (2010).
Sekatski, P. et al. Exploring macroscopic entanglement with a single photon and coherent states. Phys. Rev. A 86, 060301 (2012).
Bruno, N. et al. Displacing entanglement back and forth between the micro and macro domains. Nature Phys. 9, 545–548 (2013).
Lvovsky, A. I., Ghobadi, R., Chandra, A., Prasad, A. S. & Simon, C. Observation of micro–macro entanglement of light. Nature Phys. 9, 541–544 (2013).
Marquardt, F., Abel, B. & von Delft, J. Measuring the size of a quantum superposition of two many-body states. Phys. Rev. A 78, 012109 (2008).
Nimmrichter, S. & Hornberger, K. Macroscopicity of mechanical quantum superposition states. Phys. Rev. Lett. 110, 160403 (2013).
Sekatski, P., Sangouard, N. & Gisin, N. The size of quantum superpositions as measured with ‘classical’ detectors. Phys. Rev. A 89, 012116 (2014).
Zavatta, A., Viciani, S. & Bellini, M. Quantum-to-classical transition with single-photon-added coherent states of light. Science 306, 660–662 (2004).
Lamas-Linares, A., Simon, C., Howell, J. C. & Bouwmeester, D. Experimental quantum cloning of single photons. Science 296, 712–714 (2002).
Parigi, V., Zavatta, A., Kim, M. S. & Bellini, M. Probing quantum commutation rules by addition and subtraction of single photons to/from a light field. Science 317, 1890–1893 (2007).
Zavatta, A., Parigi, V., Kim, M. S., Jeong, H. & Bellini, M. Experimental demonstration of the bosonic commutation relation via superpositions of quantum operations on thermal light fields. Phys. Rev. Lett. 103, 140406 (2009).
Peres, A. Separability criterion for density matrices. Phys. Rev. Lett. 77, 1413–1415 (1996).
Horodecki, M., Horodecki, P. & Horodecki, R. Separability of mixed states: necessary and sufficient conditions. Phys. Lett. A 223, 1–8 (1996).
Lee, J., Kim, M. S., Park, Y. J. & Lee, S. Partial teleportation of entanglement in a noisy environment. J. Mod. Opt. 47, 2151–2164 (2000).
Andersen, U. L. & Neergaard-Nielsen, J. S. Heralded generation of a micro–macro entangled state. Phys. Rev. A 88, 022337 (2013).
Zavatta, A., D'Angelo, M., Parigi, V. & Bellini, M. Remote preparation of arbitrary time-encoded single-photon ebits. Phys. Rev. Lett. 96, 020502 (2006).
Lvovsky, A. I. Iterative maximum-likelihood reconstruction in quantum homodyne tomography. J. Opt. B 6, S556–S559 (2004).
Hradil, Z., Řeháček, J., Knill, E. & Lvovsky, A. I. Diluted maximum-likelihood algorithm for quantum tomography. Phys. Rev. A 75, 042108 (2007).
Ralph, T. C., Gilchrist, A., Milburn, G. J., Munro, W. J. & Glancy, S. Quantum computation with coherent optical states. Phys. Rev. A 68, 042319 (2003).
Neergaard-Nielsen, J. S., Eto, Y., Lee, C.-W., Jeong, H. & Sasaki, M. Quantum tele-amplification with a continuous-variable superposition state. Nature Photon. 7, 439–443 (2013).
Takahashi, H. et al. Generation of large-amplitude coherent-state superposition via ancilla-assisted photon subtraction. Phys. Rev. Lett. 101, 233605 (2008).
Gerrits, T. et al. Generation of optical coherent-state superpositions by number-resolved photon subtraction from the squeezed vacuum. Phys. Rev. A 82, 031802(R) 10.1103/PhysRevA.82.031802(2010).
Park, K., Lee, S.-W. & Jeong, H. Quantum teleportation between particlelike and fieldlike qubits using hybrid entanglement under decoherence effects. Phys. Rev. A 86, 062301 (2012).
Kok, P. et al. Linear optical quantum computing with photonic qubits. Rev. Mod. Phys. 79, 135–174 (2007).
Ralph, T. C. & Pryde, G. J. Optical quantum computation. Prog. Opt. 54, 209–269 (2010).
Acknowledgements
A.Z., L.S.C., S.G. and M.B. acknowledge support from the European Union under the CHIST-ERA (European Coordinated Research on Long-term Challenges in Information and Communication Sciences & Technologies ERA-Net) project QSCALE (Quantum Technologies for Extending the Range of Quantum Communications) and from the Italian Ministry of Education, University and Research under the FIRB (Fondo per gli Investimenti della Ricerca di Base; contract no. RBFR10M3SB). H.J., M.K. and S.-W.L. were supported by the National Research Foundation of Korea (grant no. 2010-0018295) funded by the Ministry of Science, ICT and Future Planning of Korea. T.C.R. acknowledges support from the Australian Research Council Centre of Excellence for Quantum Computation and Communication Technology (project no. CE110001027).
Author information
Authors and Affiliations
Contributions
H.J. and T.C.R. proposed the experiment and developed the theoretical models. M.K. and S.-W.L. performed theoretical analysis and numerical simulations. L.S.C. and S.G. ran the experiment and data analysis. A.Z. and M.B. proposed, planned and coordinated the experiment and data analysis. All authors discussed the results and implications of the experiment and contributed to writing the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary information
Supplementary information (PDF 628 kb)
Rights and permissions
About this article
Cite this article
Jeong, H., Zavatta, A., Kang, M. et al. Generation of hybrid entanglement of light. Nature Photon 8, 564–569 (2014). https://doi.org/10.1038/nphoton.2014.136
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphoton.2014.136
This article is cited by
-
Quantum secure direct communication with hybrid entanglement
Frontiers of Physics (2024)
-
Entanglement monogamy in indistinguishable particle systems
Scientific Reports (2023)
-
Converting qubits
Nature Photonics (2023)
-
A quantum-bit encoding converter
Nature Photonics (2023)
-
Entanglement of Hybrid State in Noninertial Frame
International Journal of Theoretical Physics (2023)