Rayleigh approximation to ground state of the Bose and Coulomb glasses

Abstract

Glasses are rigid systems in which competing interactions prevent simultaneous minimization of local energies. This leads to frustration and highly degenerate ground states the nature and properties of which are still far from being thoroughly understood. We report an analytical approach based on the method of functional equations that allows us to construct the Rayleigh approximation to the ground state of a two-dimensional (2D) random Coulomb system with logarithmic interactions. We realize a model for 2D Coulomb glass as a cylindrical type II superconductor containing randomly located columnar defects (CD) which trap superconducting vortices induced by applied magnetic field. Our findings break ground for analytical studies of glassy systems, marking an important step towards understanding their properties.

Introduction

Understanding the complexity of glassy behaviors resulting from the exponential degeneracy of their ground states is key to gaining insight into a variety of systems in nature, including spins and magnetic moments in magnetism^1,2,3, electrons in metals^4,5, Cooper pairs in disordered superconductors^6,7, vortices and interfaces in superconductors and magnets^1,8,9,10,11, social¹² and neural¹³ networks and protein folding¹⁴ remains one of the major challenges in physics. One of the furthest-reaching problems in the physics of glasses is that of understanding the nature of Coulomb or electronic glasses where concurring effects of long-range Coulomb interactions and disorder result in a depletion of the density of electronic states (referred to as the opening of a ‘Coulomb gap’) which turns metal into an insulator. This causes two key aspects of glassiness, namely disorder and frustration, to become maximally pronounced. Disorder means that, unlike periodic structures, glasses do not possess long-range order, while frustration refers to the competition among conflicting interactions. Consequently, the system does not find an accommodation that complies with all constraints but arrives instead at a multitude of degenerate, i.e. almost equally advantageous states the number of which exponentially grows with the system size. These states are separated by nearly infinitely large free-energy barriers in a phase space that acquires complex hierarchical structure. As a result, glasses are effectively non-ergodic and manifestly lack equilibrium, that is they cannot equilibrate with their environment. This gives rise to remarkable aging and memory effects and highly nonlinear dynamics. The reason that the properties of glasses are still not thoroughly understood is that the same complex structure of a highly degenerate ground state that makes glasses interesting and appealing, impedes the application of our standard theoretical physics machinery. Moreover, frustration and non-ergodicity denigrate efficiency of even the best numerical algorithms, which are commonly used to study glasses, because there is no guarantee that the process of a search for the ground state will take the system close to the global minimum rather than getting stuck within some local potential well, see refs 15, 16, 17 and references therein. The nature of the ground state of Coulomb or electronic glasses poses a special challenge since electronic glasses are agreeably at the heart of physics of many strongly correlated systems, most notably, high temperature superconductors¹⁸.

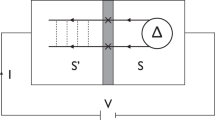
Here we address this challenge and offer an analytical approach to construct an approximation to a ground state of the 2D random finite system in the first order with respect to disorder. We consider a two-dimensional Coulomb gas subject to quenched disorder and take advantage of the latter's equivalence to the system of 3D vortices in type II superconductors containing randomly distributed columnar defects⁹, see Fig. 1a. Complete identity is achieved by choosing a lateral size for the system not exceeding the London penetration depth, λ. Then vortex-vortex logarithmic interactions remain unscreened and the vortex system becomes isomorphic to the 2D Coulomb gas of logarithmically interacting electric charges, Fig. 1b, c. Hence the equivalence of the problems of the lowest energy states of these two systems: the global minimum of the Ginzburg-Landau (GL) functional, describing the configuration of vortices corresponding to the lowest energy, defines the ground state of both.

Figure 1

Superconducting cylinder with columnar defects and corresponding single-particle potential relief.

(a): Sketch of a superconducting cylinder with arbitrarily distributed columnar defects. The characteristic lengths are related by the inequalities chain, . (b): Egg-crate energy potential relief for a single particle in the related two-dimensional Coulomb gas of charged particles. (c): Cross-section of the egg-crate potential relief for a charge emphasizing its random character.

We consider a large, i.e. containing a macroscopic number of defects, but finite superconducting sample in a form of a cylinder, with a circular base of the diameter . The cylinder is stuffed with columnar cavities or defects of radius R, mean spacing between defects being a, see Fig. 1a. We take and assume the chain of inequalities, , where ξ is the superconducting coherence length. In addition we impose the even stronger scale separation condition that . We restrict ourselves to the range of magnetic fields H < H_c1, where H_c1 is the lower critical field, so that vortices do not exist in the bulk of the sample but can only be trapped by CDs. We develop an analytical approach for finding the vortex distribution , where vorticity or degree {d_j} measures the number of flux quanta trapped by the jth CD, minimizing the system energy, i.e. for finding the vortex distribution corresponding to the ground state of the system. The maximal filling of a CD is defined by the condition¹⁹ n_max = [R/2ξ]. Vortex quantization, i.e. the condition that the degrees must be integers imposes a constraint on the minimization of the energy. Our technique is based on the method of functional equations^{20,21,22,23,24}, see Methods and Supplementary Information (SI) for details. That the found energy-minimizing distribution (minimizer) corresponds to a unique global energy minimum follows from convexity of the energy functional. If the array of CDs is regular, the vortex system can assume a terrace-like ground state i.e. form a hierarchical nested domain structure^25,26.

Each domain is characterized by its filling factor {d_j}, which grows upon traversing from the sample border to its center. To emphasize the net effects of disorder we consider initially a regular arrangement of CDs and then watch how the nested domain's ground state structure evolves upon randomizing the distribution of the defects. We reveal that, as the randomness grows, the vortex count within each vortex domain remains approximately the same but the smooth domain walls of the regular defect array acquire fractal character and turn into fractal interfaces with non-universal fractal dimensionality depending on the degree of disorder. We find that disorder generates energy states for vortices, that lie below those corresponding to the regular array of CDs. These low-lying vortex energy states that can be viewed as a hallmark of emergent glassiness manifest themselves, in particular, in decreasing the field of the first vortex trapping with increasing degree of disorder.

We describe our system with the Ginzburg-Landau (GL) functional:

where Ω is the volume of the sample, Ψ(r) is the superconducting order parameter, H is the external magnetic field applied along the z-axis aligned with the cylinder, r the coordinate vector in the plane of Ω perpendicular to z, m and −e are the electron mass and charge, respectively and A(r) is the vector potential of the magnetic field so that B = curl(A). The London penetration depth λ² = (mc²β)/(8πe²|α|) and the coherence length ξ² = ħ²/(4m|α|) are encoded in the coefficients of the GL functional. For the sake of convenience, we define the standard dimensionless order parameter u = Ψ/Ψ₀ where , measure lengths in units of λ and the magnetic field in the units of Φ₀/(2πλξ), Φ₀ = πħc/e is the magnetic flux quantum. The dimensionless columnar defect radius . We consider the case when the CD's radii are small as compared to their spacing and are parameterized by ρ = exp(−γ/ε²), where γ is a constant of the order of unity, which implies an exponential separation of the characteristic lengths. Since the spatial scale of the variation of the order parameter ξ is the smallest scale in the problem, we can set the order parameter |u| = 1 everywhere besides the CDs. It has been established^25,26 that the distribution of vortices obtained from the minimization of (1) is identical to that obtained by minimizing the energy

where the distribution of the field corresponding to the ground state of the system, or the minimizer h = h({d_k}) satisfies the following Euler-Lagrange equations

with ensuring the correct boundary conditions at the CDs interfaces: h(r) = h_a = constant on the exterior boundary and constant boundary conditions on interfaces, d_j represents the degree of the vortex in the jth CD, i.e. the number of flux quanta trapped by jth CD. The task now is to derive the unique global minimizer of (2) as an asymptotic solution to boundary value problem (3) for the magnetic field given an n-tuple of degrees d = (d₁, …, d_N), N being the total number of CDs. To this end one first finds the magnetic field h as a function of the degrees by employing the analytical method of functional equations developed for Poisson equation²³, which we outline below, see also SI. The derived h({d_j}) is plugged in into the energy functional (2) and then the numerical minimization over the class of all n-tuples of degrees with the additional constraint that each degree must be an integer is carried out.

We seek an analytical solution to (3) as a series h = h_a(h⁽⁰⁾ + h⁽¹⁾ + h⁽²⁾ + …) with respect to a small parameter 1/|ln ρ|, where , I₀(x) is the modied Bessel function of order zero and each term h^(k) = O(1/|ln^k ρ|). This expansion solves equations from the cascade of problems²⁷: Δh⁽⁰⁾ = h⁽⁰⁾, Δh⁽¹⁾ = 0, Δh⁽²⁾ = h⁽¹⁾, …, Δh^(k) = h^(k−1). We find the minimizer for the random distribution of CDs within Rayleigh approximation truncating the expansion at the lowest order terms^28,29. Let ζ = x₁ + ix₂ be a complex variable corresponding to r and complex numbers a_k be coordinates of CD centers in the ζ-plane. We look for a solution to (3) in the form h ≈ h_a(h⁽⁰⁾ + h⁽¹⁾) with

where the perforated domain D is a cross section of Ω and φ(ζ) is an unknown function holomorphic in D. One can prove the convergence of the successive approximations for the functional equations for ψ(ζ) (see SI), where ψ(ζ) = φ′(ζ). The solution to (4) in the multiconnected domain D is found up to the order of O(1/ln² ρ) as

with unknown . From the boundary conditions at the CDs, we express c₀ linearly through β_k and derive a system of linear algebraic equations for the constants β_k, k = 1, …, N. The coefficients of this system depend on ρ and we retain terms up to O(1/ln² ρ). This system has a dominant diagonal part for small ρ implying the uniqueness of the solution. One can observe that in the lowest order in 1/ln ρ terms, β_k ~ 1/ln ρ and hence . If more terms are included into the Rayleigh expansion (with each term h^(p) ~ 1/ln^p ρ), then the series would converge to h. The linear system for β_k can be solved numerically which completes the calculation of the magnetic field h in the Rayleigh approximation for given d = (d₁, …, d_N). Once all β_k are obtained, we minimize the energy as a function of degrees, d and determine d corresponding to the energy minimum. Since the energy functional is convex, the local minimizer is a global minimizer, which solves the problem of finding the ground state. The derivation of d = (d₁, …, d_N) for random CD array constitutes the main result of our work.

A fundamental implication of the randomization of the CD arrangement is the formation of a Bose glass state endowed with arbitrarily low-lying energy states for vortices⁹. While the Rayleigh approximation cannot guarantee the construction of a true glassy state, the observed decrease of the effective lower critical field, i.e. the field of the first vortex trapping H_G as compared to the critical field H_reg where first vortices appear at the regular (periodic) CD array, can be viewed as a direct manifestation of emerging glassiness in a finite superconducting cylinder. Shown in Fig. 2a, b is the is the behavior of H_G as function of the degree of disorder and of the total number of CDs in a fully randomized array. Trapping vortices modifies the phase diagram of the type II superconductor as shown in Fig. 2c.

Figure 2

Emergence of glass.

(a): The field of the first emergence of a vortex trapped by CD, H_G, as function of the number of the randomization steps M. Incremental decrease of H_G reflects the decrease of the lowest energy of the vortex trapped by CD, i.e. emergence of glassiness. (b): Fields of the first penetration H_reg for periodic array of CDs and H_G for random array as functions of the number of CDs in the sample. Upon increasing the number of CDs, H_reg saturates, but H_G continues to gradually decrease. This illustrates the increasing glassiness of the system: as the number of CDs grows, more and more deep potential wells associated with CDs appear. (c): Low-temperature part of the H-T vortex phase diagram.

The emergence of glassy features in the sample containing N = 770 columnar defects and n_v = 1105 vortices is illustrated in Fig. 3. Initially CDs are arranged into a regular lattice. Accordingly, vortices form two nested domains with the degrees d = 0 and d = 1. Panels a–c of Fig. 3 demonstrates how the terrace structure of the nested domains evolves and gets blurred upon incremental growth of the randomness in the CD's locations. While the domains corresponding to different degrees retain their identities, the interfaces between them acquire a fractal structure. Making use of the Box-Counting method³⁰, we find the fractal dimension of the interface, , where N_a is the number of cells the interface curve crosses and s_a is the scale for the trial a. The evolution of the interface between the d = 0 and d = 1 domains, clearly seen in Fig 3, visualizes incremental fractalization. The fractal dimensionality as a function of the degree of disorder quantified by the number of steps M in the randomization procedure, see SI, is presented in Fig. 3d. Its initial part is reasonably fitted as with c = 0.060 ± 0.01 and ν = 0.335 ± 0.01. Upon further increase of the random steps, fractal dimensionality converges to . Fractalization of the interface leads to the shrinking of the vortex-free shell near the sample surface: its average width first decreases with the growing level of randomness (see Fig. 3e) as , with ν₁ = 0.323 ± 0.01, c₁ = −0.025 ± 0.01 and c₂ = 0.257 ± 0.01 and then saturates, which parallels the behavior of . Note that ν₁ appears to be close to ν.

Figure 3

Fractal dimension of vortex domain interface.

(a)–(c): Developing fractality with increasing degree of disorder. (d): Fractal dimensionality as function of the number of random steps M. Fractal dimension of the interface grows with increasing randomness and saturates to the value of at about . The growing part of is well approximated as with c = 0.060 ± 0.01, ν = 0.335 ± 0.01 (the red line). (e): Average width d of the vortex-free shell near the edge of the sample as function of M. The red line is fit with c₁ = −0.025 ± 0.01, c₂ = 0.257 ± 0.01 and ν₁ = 0.323 ± 0.01.

A special situation, where the defect radius is chosen to be comparable with the superconducting coherence length, so that n_max = 1, is identical to a 2D Coulomb glass of bosons in which the double occupancy is forbidden. The calculated set d describes thus the distribution of charges corresponding to the minimal energy, i.e. the ground state, of the system. Thus, our finding offers a firm ground for numerical study of hopping conductivity of the Coulomb glass. Using the minimizer d an initial state in numerical simulations of hopping ensures that the transport indeed occurs due to excitations over the ground state as it should in reality. Note further that although our derivation is done for a particular situation where the lateral size of the system is close to the screening length of the 2D Coulomb interaction, our results carry importance well beyond their immediate context and break ground for a general analytical description of the ground state of glassy systems.

Methods

Since the spatial scale of the variation of the order parameter ξ is the smallest scale in the problem we can set the dimensionless order parameter |u| = 1 everywhere besides the CDs. Then the problem of minimizing GL functional is formulated as a problem of finding a minimizer for the so-called harmonic map-type functional²⁵,

which, in its turn, is reduced to minimizing the energy given by Eq. (2). The solution for the field distribution is sought in a form given by function φ of Eq. (4), having the general representation

where is single-valued, A_m are real constants²³ and the summation is taken over all arbitrarily located CDs. By extending this function to the CD boundaries and taking its derivative (ψ(ζ): = dφ/dζ) one arrives at the classical Riemann-Hilbert problem for ψ analytic in the multiply connected domain D, which results in a solution in a form of (5). Now the key step is to apply the method of functional equations that allows us to express d through β_k. Further, it is convenient to minimize numerically the energy as a function in β_k (k = 1, 2, …, N) and after to calculate the optimal values of d = (d₁, …, d_N) by exact formulas through the optimal values of β_k.