Abstract
Recently, ultrathin metal-oxide thin film transistors (TFTs) have shown very high on-off ratio and ultra-sharp subthreshold swing, making them promising candidates for applications beyond conventional large-area electronics. While the on-off operation in typical TFTs results primarily from the modulation of charge carrier density by gate voltage, the high on-off ratio in ultrathin oxide TFTs can be associated with a large carrier mobility modulation, whose origin remains unknown. We investigate 3.5 nm-thick TiOx-based ultrathin TFTs exhibiting on-off ratio of ~106, predominantly driven by ~6-decade gate-induced mobility modulation. The power law behavior of the mobility features two regimes, with a very high exponent at low gate voltages, unprecedented for oxide TFTs. We find that this phenomenon is well explained by the presence of high-density tail states near the conduction band edge, which supports carrier transport via variable range hopping. The observed two-exponent regimes reflect the bi-exponential distribution of the density of band-tail states. This improved understanding would be significant in fabricating high-performance ultrathin oxide devices.
Similar content being viewed by others
Introduction
Thin film transistors (TFTs) made of semiconducting metal oxide have been extensively investigated over the past decades, primarily as a higher-mobility, low-cost alternative to conventional amorphous Si in large area electronics applications such as active matrix displays1,2,3. On the other end of the spectrum, in the domain of high-performance computing, the single-crystalline Si-based technology is approaching its physical limits, and atomically thin two-dimensional material systems have been garnering extensive interests in pursuit of gaining ultimate electronic control on the transistor channel. As a matter of fact, fully depleted Si-on-insulator (FD-SOI) technology, using ultrathin Si layer has shown profound impacts on low-power computing devices4. To this end, complex oxide materials have also been used to fabricate TFTs based on two-dimensional electron gas (2DEG), where the carrier transport is highly confined near the insulator-semiconductor interface5. Primarily, such reports have been focused on epitaxially grown oxides, limiting their scalability in practical applications. However, the 2DEG formation has been reported recently in a rather simple TiO2/Al2O3 interface fabricated by atomic layer deposition (ALD)6,7, showing promises for scaling up this device architecture for more practical applications. In addition, sputter-deposited 1-nm-thick indium tin oxide transistors have shown to reach near-ideal subthreshold swing and nearly no drain-induced barrier lowering8. These recent reports clearly indicate growing interests in ultrathin oxide TFTs.
An interesting observation among the ultrathin channel devices and 2DEG transistors is that they typically exhibit sharp and very high on–off ratios in the transfer characteristics with extremely low off currents; the channel is essentially fully depleted of charge carriers in the off-state, and the gate induced charge accumulation leads to many decades of increases in the drain current, driving the transistor into on-state. In general, the working of conventional TFTs exhibit many orders of gate-dependent charge carrier density modulation, while the extent of gate-induced mobility modulation has been limited to only a few decades9,10,11,12 or has not been studied in detail13,14,15. A large gate-dependent mobility modulation is uncommon in conventional amorphous Si TFT devices, and a clear picture elucidating the origin of mobility modulation in ultrathin metal oxide TFTs has not been reported so far11,16. Since the on–off current modulation in TFTs is essentially from the combined effect of both carrier density and mobility modulations, it is worth examining if such mobility modulation is a universal phenomenon in amorphous-oxide TFTs. Moreover, it may have general implications on further improving the performance of the ultrathin oxide devices.
TiO2 as a semiconductor is extensively used in solar cells17,18,19,20 and photocatalysis21,22,23,24, but its application as a TFT channel material has not been much explored. For those reported so far, TiO2 layers were mostly thicker than ~10 nm and crystalline9,25,26,27,28,29,30,31,32,33,34, with only a few reporting the mobility modulation9,10. A large extent of mobility modulation, as high as ~9 decades, has been recently reported from mixed amorphous-crystalline TiOx TFT35, with its origin remaining unexplained nevertheless.
In this report, we utilize ultrathin (3.5 nm) amorphous TiOx film deposited by low-temperature ALD to fabricate TFT devices and investigate the origin of large gate-induced mobility modulation. Based on the analysis of gate-dependent mobility and control experiments involving post-ALD thermal treatments, we find that the mobility modulation is caused by the presence of high density of band-tail states that mediate the variable range hopping (VRH) of charge carriers near the mobility edge of conduction band.
Results and discussion
Performance of ultrathin TiOx TFT
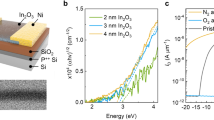
We fabricated bottom-gate TFTs based on the 3.5-nm-thick ultrathin amorphous TiOx thin film deposited on Si substrates with 300 nm SiO2 by low-temperature ALD at 85 °C using titanium (IV) isopropoxide and water as reactants. The deposited film was subjected to a two-step forming gas annealing at 400 °C and 600 °C (each for 15 min), and Al-based source and drain electrodes with channel length of 5 µm and width 500 µm were patterned by electron-beam lithography as shown in the schematic in Fig. 1a. The ultrathin TiOx film, after the default forming gas annealing treatment, was found to be nearly amorphous with minor nano-crystallinity, as it can be seen in the X-ray diffraction (XRD) spectra depicted in Fig. 1b. Despite using slow acquisition rate of the XRD spectra (scan rate of 0.38° min−1; total scan time of 3 h), no significant crystallinity could be observed, except for the two very weak scattering peaks likely originating from either rutile or anatase TiOx17,24. Moreover, the highly surface-sensitive, low-energy electron diffraction (LEED) pattern acquired from the TiOx (inset in Fig. 1b) shows uniform, diffused background with no discernable diffraction features, further confirming the amorphous nature of prepared ultrathin TiOx film.
a Schematic of the TiOx TFT device investigated; b XRD pattern acquired from 3.5-nm-thick TiOx thin film displaying no significant crystallinity except for weak scattering peaks potentially corresponding to anatase/rutile phases. The inset shows LEED image of the TiOx thin film where the lack of any discernable crystalline peaks confirms the near amorphous structure of ultrathin TiOx.
The transistor output characteristics measured from the fabricated ultrathin TiOx TFT shown in Fig. 2a display linear ohmic characteristics at low drain voltage (Vd) across the entire range of gate voltages (Vg), whereas toward the high Vd the drain current (Id) tends to saturate. Accordingly, the transfer characteristics measured in the saturation regime (Vd = 70 V), as depicted in Fig. 2b, shows ~6 decades of on–off ratio, the subthreshold swing of 3.6 V/dec, the turn-on voltage (Von) of ~24.6 V, and the threshold voltage (Vth) of ~48.5 V, with the relatively large difference between Von and Vth hinting the presence of a large population of trap states36. The saturation mobility (μsat) of the TFT was then calculated using the standard expression,
where L represents channel length, W channel width, and Ci insulator capacitance per unit area. The μsat in the device under the discussion was ~1.6 × 10−4 cm2 V−1 s−1. While the acquired mobility is lower than the crystalline TiO2 TFTs reported previously25,27,29,32, it is comparable to those reported from the TFTs fabricated using lower crystallinity TiO210,33,37. We further evaluated the gate dependence of field-effect mobility (μFE) using the general expression (Eq. (2))38,39 and observed a large, ~6-decade gate-dependent modulation of μFE (Fig. 2c).
Electrical characteristics of the TiOx TFT (W/L = 500 μm/5 μm) fabricated using ultrathin TiOx film annealed in forming gas (15 min at 400 °C followed by 15 min at 600 °C). a Output characteristics depicting linear-ohmic behavior at low Vd evolving into hard saturation at high Vd; b Transfer characteristics at Vd = 70 V showing high on–off ratio (~6 decades), Von of ~24.6 V, and Vth of ~48.5 V; c Vg-dependent µFE with power law fitting for Vg > 60 V and Vg < 60 V, respectively. The exponent value of 0.78 for Vg > 60 V corresponds to the TLC, whereas the value of 2.18 for Vg < 60 V suggests VRH transport.
Interestingly, as seen in Fig. 2c, the TFT also exhibits ~6 decades of mobility modulation. While the magnitude of mobility modulation generally observed in TiOx TFTs has been 2–3 decades or less9,10, modulation of ~9 decades has also been reported for sputter-deposited 20-nm-thick TiO2−x TFTs followed by vacuum annealing35.
Power law behavior of charge mobility
Given the ~6 decades of on–off ratio observed in our ultrathin TiOx TFT, the current modulation is thus predominantly originating from the gate-induced mobility modulation while the carrier density modulation by gate providing only a minor contribution. We can safely exclude possible effects of contact Schottky barrier on the observed mobility modulation considering the choice of Al electrode that has been shown to form an ohmic contact on TiOx26,29,33,37,40 and the negligible barrier height lowering effected by gate bias (Supplementary Note 1). The origin of gate-induced mobility modulation has been previously speculated to be related with the sub-bandgap trap states (localized tail states)41,42, typically present near the conduction band edge (Ec) of amorphous semiconductors with an exponential decrease in their density of states. Particularly, Lee et al. have proposed a power law dependence of the saturation-regime μFE on Vg with the following expression16,42:
where the exponent γ was correlated to the assumed carrier transport mode, and VT,P to the threshold voltage or charge percolation threshold at a given temperature. Based on the less than a decade of gate-dependent μFE modulation observed from amorphous InZnO/InGaZnO TFTs, they described the charge transport at low Vg as a trap-limited conduction (TLC) with the associated exponent of 0.7 and the high-Vg transport as mediated by percolation transport with the related exponent of 0.1. Similar gate-dependent μFE and exponents have been observed in the case of polycrystalline ZnO TFTs11,12.
Upon utilizing the power law relationship to empirically analyze the μFE vs. Vg dependence, two different regimes are similarly identified in our gate-dependent mobility data obtained from the ultrathin TiOx TFT, but, with very different exponent values as shown in Fig. 2c; at high Vg (>60 V), the exponent of 0.78 was obtained, indicating that the transport lies around the TLC according to the model proposed by Lee et al. However, at Vg < 60 V, the obtained exponent is 2.18, far outside of the previously observed upper limit of 0.716; in fact, such a high exponent value has never been reported for metal oxide TFT to our knowledge. As discussed, next, we attribute this to a high density of band-tail states that mediate the charge transport via VRH with the varying position of Fermi level (EF) prescribed by given Vg accessing different distributions of band-tail states capable of supporting the VRH.
Origin of band-tail states
First, in order to ascertain the stoichiometry of the TiOx film and examine the presence of defects such as oxygen vacancies, high-resolution X-ray photoelectron spectroscopy (XPS) spectra were obtained from the prepared TiOx film subjected to the post-ALD forming gas annealing (Fig. 3a, c) and compared with the data from a control TiOx film after similar post-ALD annealing carried out in pure O2 (Fig. 3b, d). The defect states in metal oxides, typically originating from oxygen vacancies or interstitial hydrogen, are by nature shallow states generally existing very close to the conduction band edge. In the case of TiO2, various reports have estimated that such shallow states exist at ~10–260 meV below the conduction band edge, among which those related with hydrogen interstitials are relatively closer to the conduction band edge than the oxygen vacancy states43,44,45,46. When the TiOx ALD is carried out at lower temperatures, such as 85 °C in our case, a high density of defect states is generally expected to exist within the deposited film.
As seen in the XPS spectra (Fig. 3a, b), both the Ti4+ peaks, viz. Ti4+ 2p3/2 and Ti4+ 2p1/2, correspond to TiO2 lattice, whereas the Ti3+ peak (Ti3+2p1/2) is related to a reduced, Ti2O3 lattice structure. The change in the peak intensities can be attributed to the difference in the electronic states of Ti in the two different samples. The sample annealed in forming gas (Fig. 3a) exhibited ~90 % larger area for Ti3+ peaks and ~17 % area decrease for Ti4+ peak, in comparison with oxygen-annealed sample (Fig. 3b). This observation indicates that the annealing in forming gas removes oxygen atoms from the TiO2 lattice, reducing the concentration of Ti4+, resulting in the increase in Ti3+ and the fraction of Ti2O347,48,49. The difference in the composition of the above samples was cross-confirmed by the peak shift and estimating the change in area of the O 1s XPS spectrum (Fig. 3c, d). The as-acquired O 1s peak was deconvoluted into three peaks with binding energies of 529.73, 531.22, and 532.23 eV, each corresponding to lattice oxygen (TiO2), reduced Ti2O3, and non-lattice oxygen (e.g., –OH, wherein hydrogen can originate from water used as an oxidant during ALD or forming gas annealing), respectively. In the forming-gas-annealed sample (Fig. 3c), the lattice oxygen peak (529.76 eV) exhibited a decrease in its area by ~28%, while the area of the Ti2O3 peak (530.92 eV) and non-lattice oxygen peak (532.03 eV) increased by ~55% and ~12%, respectively, compared with the oxygen-annealed sample (Fig. 3d). These data, along with the Ti 2p XPS spectral analysis, thus confirm that the forming gas annealing led to the formation of oxygen vacancies in the lattice by the consumption of lattice oxygen to form a larger proportion of reduced Ti2O347.
To identify the effect of oxygen vacancies on the gate-dependent mobility, transfer characteristics were measured from a control TFT fabricated on the oxygen-annealed ultrathin TiOx film (Fig. 4a). Compared with the forming-gas-annealed TiOx TFT (Fig. 2b), the on-state drain current was decreased by 2 decades even at higher Vd (=100 V), whereas the off-state current was also increased by 2 decades, rendering the current modulation ratio significantly decreased down to only 2 decades. The observed increase in off-state currents is consistent to the notion that annealing the defective ultrathin TiOx film in oxygen environments reduces the concentration of oxygen vacancy (i.e., charge trap) and the number of trapped charge carriers within the TiOx film. Moreover, the oxygen-annealed device featured a markedly smaller difference between Vth (~55.2 V) and Von (~46.4 V) compared with the forming-gas-annealed TiOx device (i.e., Vth = ~48.5 V and Von = ~24.6 V), reaffirming the reduced charge trap density, given that the difference between Vth and Von can be majorly attributed to the charge trap concentration within the channel of a TFT, since within the Von < Vg < Vth regime, most charge carriers are induced into trap states and/or tail states that exhibit low mobility36. Meanwhile, as we have postulated earlier, the primary charge transport in the on-state is likely mediated by the defect states associated with oxygen vacancies, and, thus, the decreased oxygen vacancy concentration should decrease the on-state current, as observed in the oxygen-annealed TiOx TFT (Fig. 4a). Likewise, the field-effect mobility was decreased by ~3 decades (Fig. 4b) with mobility modulation decreased to 4 decades compared with the forming-gas-annealed TiOx device (Fig. 2c) with ~6 decades of mobility modulation, strongly suggesting the role of defect states in the Vg-induced mobility modulation.
a Transfer characteristics of the TiOx TFT (W/L = 500 µm/5 µm) fabricated using ultrathin TiOx film annealed in oxygen atmosphere (15 min at 400 °C followed by 15 min at 600 °C); b µFE variation with Vg with power law fits for Vg > 50 V and Vg < 50 V. The exponent values of 1.85 and 3.28 indicate that the carrier transport occurs via VRH. The decrease in the number of available defects states however restricts the charge mobility. c Transfer characteristics of the TiOx TFT (W/L = 500 µm/5 µm) fabricated using forming gas annealing and the PMMA top-coat, leading to the passivation of surface defects and, consequently, a small increase in the on-state current due to decreased carrier scattering. d µFE variation with gate voltage and power law fit for Vg > 40 V and Vg < 40 V with the exponents of 0.79 and 2.99 indicating the VRH transport at low Vg changing toward TLC transport at high Vg.
Considering the ultrathin thickness of the TiOx film, it is also possible that the surface defects and associated states may have an impact on the TFT characteristics. In order to test this premise, we spin-coated 300 nm of poly(methyl methacrylate) (PMMA) on top of the TFT devices fabricated from the forming-gas-annealed ultrathin TiOx film. While the PMMA-topcoat is expected to only passivate the surface defects, the device characteristics of the PMMA-topcoat TiOx TFT (Fig. 4c) displayed only a marginal improvement in the transfer characteristics. For instance, both on- and off-state currents were only slightly increased, resulting in a minor increase in the maximum saturation mobility. The device also featured ~6 decades of current on–off ratio, largely identical to the value obtained without the PMMA surface passivation while Vth and Von exhibited a slight decrease (by ~10 V). As depicted in Fig. 4d, the field-effect mobility showed ~6-decade modulation with the power law exponents of 0.79 and 2.99, respectively, for high-Vg and low-Vg regimes, all nearly same as the values obtained from the TiOx device without the PMMA-topcoat, highlighting that the surface defect states have a minor influence on the observed, large gate-induced modulation of charge mobility, not interfering with the inherent charge carrier transport in the ultrathin TiOx thin film.
Bi-exponential density of states
Based on the correlations drawn between the transfer characteristics and XPS analysis discussed above, it can be established that the oxygen vacancies within the bulk of the TiOx film mediate the charge carrier transport in the ultrathin TiOx TFT. However, the meaning of the large power law exponent still remains unexplained, which to our knowledge has never been reported in the case of oxide semiconductors. During the postulation of Eq. (3) for TLC mode in oxide TFTs, Lee et al. have correlated the value of exponent to the characteristics of the band-tail density of states through 2(To/T – 1), where To is the characteristic temperature of band-tail states16. In fact, a similar empirical relationship has been discussed previously in other semiconductor systems such as organic TFTs50,51,52. Interestingly, Meijer et al. have analytically derived the identical mobility vs. Vg power law relationship based on the VRH transport of charge carriers through the band-tail states in organic semiconductors50. They report the expression,
where To being the characteristic temperature of band-tail density of states, and A the proportionality constant dependent on T and other material parameters. Considering that \(\sigma _{\mathrm{d}} = en\mu\), where e is the elementary charge and \(n = \frac{{C_{\mathrm{i}}\left( {V_{\mathrm{g}} - V_{{\mathrm{on}}}} \right)}}{{et_{\mathrm{s}}}}\) with ts being the thickness of the charge accumulation layer, the Eq. (4) becomes expressing the identical power relation to Eq. (3) suggested by Lee et al. Despite the fact that both the models originate from slightly different analytical approaches, they arrive at the same power law relation between mobility and Vg, especially with the identical power law exponent. Lee’s model has presented the TLC transport in oxide semiconductors while Meijer et al. have formally referred the transport mode as VRH; the origin of both the transport mechanisms can be essentially attributed to localized band-tail states originating from the material being amorphous or highly defective. In TLC, the free electrons in the conduction band are responsible for the current flow with defect states acting as charge traps, whereas in the case of VRH, high density of trap states supports the hopping transport of charge carriers, thus effectively exhibiting the current flow. Our large exponent value suggests that VRH being the governing charge transport mechanism at low Vg. There are numerous past literatures studying VRH transport in organic TFT utilizing the same model and reporting similarly large exponents. The parameter To describes the width of exponential distribution of density of band-tail states53. In the case of our device, the value of exponent at low Vg (2.18 in Fig. 2c) corresponds to To = 622.8 K (i.e. 53.6 meV). This value is comparable to the values reported for VRH in organic TFTs (~450–550 K for poly(3-hexylthiophene) (P3HT) and poly(p-phenylene vinylene)) which, similar to our system, is predominantly amorphous54,55. This evidence supports that the VRH through localized band states is indeed mediating the charge transport in our system and suggests that it is also playing a role in exhibiting large mobility modulation.
Finally, it is possible to explain the observed two different power-law exponents in the Vg-dependent mobility data. Essentially, when Vg is varied in a given voltage range, the EF in the transistor channel is sweeping through the density of band-tail states (g(E)) having a bi-exponential distribution56. Vg causes the change in the carrier density, n, though \(n = \frac{{C_{\mathrm{i}}\left( {V_{\mathrm{g}} - V_{{\mathrm{on}}}} \right)}}{{et_{\mathrm{s}}}}\), which in turn varies the EF through \(E_{\mathrm{c}} - E_{\mathrm{F}} = k_{\mathrm{b}}T\log \left( {N_{\mathrm{c}}/n} \right)\), with kb being the Boltzmann constant and Nc the effective density of states of conduction band. These Vg-dependent n and EF are illustrated in Fig. 5a. As Vg is increased, EF is being moved closer to Ec (i.e., decreasing \(E_{\mathrm{c}} - E_{\mathrm{F}}\) from 180 meV to 50 meV). During the course of this sweep, EF is probing the exponential distribution of localized band-tail states with two different kbTo regimes (Fig. 5b), which manifest themselves as the two linear regimes in the \(\log \left( {g(E)} \right)\) vs. E plot with the corresponding g(E) expressed as:
with A and B being proportional constants, and \(\frac{{ - 1}}{{k_{\mathrm{b}}T_{{\mathrm{o}}1}}}\) and \(\frac{{ - 1}}{{k_{\mathrm{b}}T_{{\mathrm{o}}2}}}\) indicating the two slopes in the schematic plot, respectively. In the mobility vs. Vg plots, a smaller γ1 (at high Vg) gives a larger slope and a large γ2 (at low Vg) a lower slope. Thus, our data shows that by sweeping Vg, we have surveyed g(E) and revealed the bi-exponential distribution of band-tail density of states, supporting the notion of the VRH charge transport mediated by localized band-tails states. Using the exponent values estimated in Fig. 2c, γ2 = 2.18 and γ1 = 0.78, the values for both the To parameters can be calculated as To2 = 622.8 K (53.6 meV) and To1 = 298.4 K (25.7 meV), respectively. It should also be noted that over the course of Vg sweep assessed in this study, EF is tracing the sub-badgap region within 180 meV to 50 meV, which is much larger than the thermal energy at room temperature (~25 meV). Thus, at low-Vg when the EF is residing in deeper trap states, the carriers do not have sufficient energy to get thermally released into the conduction band and VRH transport prevails. However, when EF starts accessing the trap states much closer to 50 meV at high-Vg, a stochastic thermal release of the charge carriers into the conduction band starts taking place and the transport evolves towards majorly TLC, which is also sometimes referred to as multiple trapping and thermal release (MTR) transport. Previously, exhibition of VRH at room temperature has been demonstrated in the case of amorphous organic semiconductors50,52. In the case of amorphous oxide semiconductors, however, the prevalence of TLC or VRH has been reported to depend on temperature, where TLC prevails at room temperature and on decreasing the temperature VRH dominates the carrier transport42.
a Variation in n (blue line) and the position of EF compared with Ec with respect to the Vg (red line) changed above Von. b Schematic showing transistor band diagram across the gate-insulator-semiconductor stack (left); more details of the area marked by a red circle (top-middle) showing the electron energy (E) vs. density of states (g), where CB denotes the conduction band with characteristic parabolic g(E); bi-exponential variation of density of states (g(E)) with energy near Ec (bottom-middle); EF is inside the band-tail and moving closer to Ec when Vg > Von, thus supporting the charge (red dot) conduction via hopping through the localized states (indicated by the red arrow) depicting VRH phenomena (right). c Linear-regime transfer characteristics extracted from the output characteristics of ultrathin TiOx TFT prepared by forming-gas annealing. d Corresponding linear mobility variation with gate voltage with the power-law exponent for VRH regime at low Vg exhibiting the value of 3.04 and the TLC transport regime at high Vg exhibiting the value of 0.8.
Meanwhile, it is worth noting that the To values observed from the VRH model in organic TFTs have been estimated from the linear-regime mobility vs. Vg data55, not from the saturation regime as discussed so far. To test whether the Vg-dependent, linear-regime mobility in the ultrathin TiOx TFT still follows the VRH charge transport, we utilized the output characteristics of the ultrathin TiOx TFT (Fig. 2a), extracting the linear-regime transfer characteristics at Vd = 1 V (Fig. 5c), which allows the evaluation of linear-regime μFE.
The resulting μFE vs. Vg plot in Fig. 5d clearly exhibits the two-exponent power law with 5-decade gate-dependent mobility modulation and the exponent values largely consistent with those observed in the saturation regime. This not only confirms the bi-exponential distribution of band-tail states but also further supports the correlation between the VRH transport and the large exponent at low Vg in the ultrathin TiOx TFT regardless of the saturation or linear device operation regimes.
Conclusions
In summary, the ultrathin amorphous TiOx TFT fabricated by low-temperature ALD was investigated to explore the origin of large gate-dependent mobility modulation observed in ultrathin metal oxide TFTs. The large ~6-decade, gate-induced mobility modulation observed in the studied ultrathin TiOx TFT could be associated with the presence of band-tail defects, such as bulk oxygen vacancies, based on the physicochemical analysis of TiOx prepared under varying post-ALD thermal treatments and the accompanying TFT device characterization. By comparing the empirical power law relationship of the gate-dependent mobility in metal oxide TFTs with the analytic model previously derived for organic semiconductors, the large power-law exponent, which drives the large gate-induced mobility modulation, was identified to be originating from the VRH transport of charge carriers through the band-tail states of ultrathin TiOx, with its bi-exponential density of states reflected on the two different power-law exponent regimes in the gate-dependent mobility. The results highlight rather unusual and counterintuitive roles of defect states within ultrathin metal oxides in possibly enabling some of the high performance parameters in associated TFT devices, as best exemplified by the increased off-state current accompanied by the reduced device on–off ratio upon the oxidative annealing of ultrathin TiOx against the typical notion that such an oxidative annealing in metal oxide transistors would reduce the background carrier density while increasing the on–off ratio.
Methods
Fabrication of TiOx TFT
The TiOx thin film was deposited using Cambridge Nanotech Savannah S100 ALD system (base pressure∼0.45 Torr) at 85 °C on oxygen-plasma-cleaned Si (p++) substrate with 300 nm dry thermal oxide. During each ALD cycle, titanium (IV) isopropoxide and water vapors were alternatively pulsed each for 0.4 s with 5-s interval under continuous N2 flow. In total, 75 cycles were used to deposit TiOx film thickness of 3.5 nm (estimated by spectroscopic ellipsometry; J.A. Woollam M-2000FI). Unless otherwise stated, as deposited films were then annealed in forming gas (4% H2/Ar) using a rapid thermal processor (RTP; Modular Process Technology, RTP-600S) at 400 °C for 15 min followed by 600 °C for 15 min. The TFT devices were fabricated with electron-beam lithography using the bilayer resist scheme (PMGI SF6 underlayer and ZEP 5200 A imaging layer) followed by lift-off of the evaporated contact metal stack consisting of 30 nm Al, 30 nm Ti, and 40 nm Au (bottom to top), to pattern source-drain electrodes with channel length of 5 µm and width 500 µm. The schematic of the bottom gate top contact TFT device investigated in this study is shown in Fig. 1a.
Material characterization
The crystallinity of the TiOx film was characterized by XRD (Rigaku Ultima III diffractometer), operating in the Bragg configuration using Cu Kα radiation (1.54 Å) with a scan range from 10° to 80° and a scan rate of 0.38° min−1 to acquire the XRD spectra. The chemical bonding characteristics of prepared samples were characterized by XPS using a custom-built XPS system equipped with a hemispherical electron energy analyzer (SPECS) and Al Kα X-ray source (1.487 keV, SPECS). The sample crystallinity was also further examined by extremely surface-sensitive, LEED inside an ultra-high-vacuum (UHV) chamber (base pressure, 2 × 10−10 Torr) using a low energy electron microscope (LEEM) located at the Electron Spectro-Microscopy (ESM, 21-ID-2) beamline of the National Synchrotron Light Source II (NSLS-II). After being transferred to the UHV chamber, the samples were annealed at mild temperature of 250 °C to remove adsorbed surface contaminants from air. All measurements were conducted at room temperature and using 30 eV incident electron energy.
Data availability
All the relevant data acquired or analyzed in support of the findings reported in this study are available from Materials Cloud Archive (https://doi.org/10.24435/materialscloud:1t-g7) or the corresponding author upon request.
References
Thomas, S. R., Pattanasattayavong, P. & Anthopoulos, T. D. Solution-processable metal oxide semiconductors for thin-film transistor applications. Chem. Soc. Rev. 42, 6910 (2013).
Yu, X., Marks, T. J. & Facchetti, A. Metal oxides for optoelectronic applications. Nat. Mater. 15, 383–396 (2016).
Wager, J. F. TFT technology: advancements and opportunities for improvement. Inf. Display 36, 9–13 (2020).
Sugii, N. Low-power-consumption fully depleted silicon-on-insulator technology. Microelectron. Eng. 132, 226–235 (2015).
Kornblum, L. Conductive oxide interfaces for field effect devices. Adv. Mater. Interfaces 6, 1–22 (2019).
Lee, H. J., Moon, T., An, C. H. & Hwang, C. S. 2D electron gas at the interface of atomic-layer-deposited Al2O3/TiO2 on SrTiO3 single crystal substrate. Adv. Electron. Mater. 5, 1–7 (2019).
Seok, T. J. et al. Field-effect device using quasi-two- dimensional electron gas in mass-producible atomic-layer-deposited Al2O3/TiO2 ultrathin (<10 nm) film heterostructures. ACS Nano 12, 10403–10409 (2018).
Li, S. et al. Nanometre-thin indium tin oxide for advanced high-performance electronics. Nat. Mater. 18, 1091–1097 (2019).
Nagao, Y. et al. Experimental characterization of the electronic structure of anatase TiO2: Thermopower modulation. Appl. Phys. Lett. 97, 172112 (2010).
Jae-Woo, Park, Dongyun, Lee, Hakyoung, Kwon & Seunghyup, Yoo Improvement of on–off-current ratio in TiOx ACtive-channel TFTs using N2O plasma treatment. IEEE Electron Device Lett. 30, 362–364 (2009).
Lin, Y.-H. et al. High-performance ZnO transistors processed via an aqueous carbon-free metal oxide precursor route at temperatures between 80–180 °C. Adv. Mater. 25, 4340–4346 (2013).
Tiwale, N., Senanayak, S. P., Rubio-Lara, J., Alaverdyan, Y. & Welland, M. E. Optimization of transistor characteristics and charge transport in solution processed ZnO thin films grown from zinc neodecanoate. Electron. Mater. Lett. 15, 702–711 (2019).
Kuan, C. I., Lin, H. C., Li, P. W. & Huang, T. Y. High-performance submicrometer ZnON thin-film transistors with record field-effect mobility. IEEE Electron Device Lett. 37, 303–305 (2016).
Wei Shih, C., Chin, A., Fu Lu, C. & Fang Su, W. Remarkably high mobility ultra-thin-film metal-oxide transistor with strongly overlapped orbitals. Sci. Rep. 6, 2–7 (2016).
Ju, S. et al. High performance ZnO nanowire field effect transistors with organic gate nanodielectrics: effects of metal contacts and ozone treatment. Nanotechnology 18, 155201 (2007).
Lee, S. et al. Trap-limited and percolation conduction mechanisms in amorphous oxide semiconductor thin film transistors. Appl. Phys. Lett. 98, 203508 (2011).
Zhu, L. et al. Ligand-free rutile and anatase TiO2 nanocrystals as electron extraction layers for high performance inverted polymer solar cells. RSC Adv 7, 20084–20092 (2017).
Lu, H., Tian, W., Gu, B., Zhu, Y. & Li, L. TiO2 electron transport bilayer for highly efficient planar perovskite solar cell. Small 13, 1–9 (2017).
Liu, M., Johnston, M. B. & Snaith, H. J. Efficient planar heterojunction perovskite solar cells by vapour deposition. Nature 501, 395–398 (2013).
Zhang, Y. et al. Dopamine-crosslinked TiO2/perovskite layer for efficient and photostable perovskite solar cells under full spectral continuous illumination. Nano Energy 56, 733–740 (2019).
Liu, H. et al. Self-cleaning triboelectric nanogenerator based on TiO2 photocatalysis. Nano Energy 70, 104499 (2020).
Meng, A., Zhang, L., Cheng, B. & Yu, J. Dual cocatalysts in TiO2 photocatalysis. Adv. Mater. 31, 1–31 (2019).
Qian, R. et al. Charge carrier trapping, recombination and transfer during TiO2 photocatalysis: An overview. Catal. Today 335, 78–90 (2019).
Wang, J., Yu, J., Zhu, X. & Kong, X. Z. Preparation of hollow TiO2 nanoparticles through TiO2 deposition on polystyrene latex particles and characterizations of their structure and photocatalytic activity. Nanoscale Res. Lett. 7, 646 (2012).
Zhao, W. et al. Fabrication and characterization of high-performance thin-film transistors based on epitaxial Ta-doped TiO2 films. IEEE Trans. Electron Devices 66, 4193–4197 (2019).
Katayama, M. et al. Field-effect transistor based on atomically flat rutile TiO2. Appl. Phys. Lett. 89, 242103 (2006).
Zhang, J. et al. High performance anatase-TiO2 thin film transistors with a two-step oxidized TiO2 channel and plasma enhanced atomic layer-deposited ZrO2 gate dielectric. Appl. Phys. Express 12, 096502 (2019).
Sekizaki, S., Osada, M. & Nagashio, K. Molecularly-thin anatase field-effect transistors fabricated through the solid state transformation of titania nanosheets. Nanoscale 9, 6471–6477 (2017).
Yajima, T., Oike, G., Nishimura, T. & Toriumi, A. Independent control of phases and defects in TiO2 thin films for functional transistor channels. Phys. Status Solidi 213, 2196–2202 (2016).
Ok, K.-C., Park, Y., Chung, K.-B. & Park, J.-S. The effect of Ta doping in polycrystalline TiOx and the associated thin film transistor properties. Appl. Phys. Lett. 103, 213501 (2013).
Zhong, N., Cao, J. J., Shima, H. & Akinaga, H. Effect of annealing temperature on TiO2-based thin-film-transistor performance. IEEE Electron Device Lett. 33, 1009–1011 (2012).
Shih, W. S., Young, S. J., Ji, L. W., Water, W. & Shiu, H. W. TiO2-based thin film transistors with amorphous and anatase channel layer. J. Electrochem. Soc. 158, H609 (2011).
Wöbkenberg, P. H. et al. TiO2 thin-film transistors fabricated by spray pyrolysis. Appl. Phys. Lett. 96, 082116 (2010).
Park, J.-W., Lee, D., Kwon, H., Yoo, S. & Huh, J. Performance improvement of N-type TiOx active-channel TFTs grown by low-temperature plasma-enhanced ALD. IEEE Electron Device Lett. 30, 739–741 (2009).
Zhong, N., Shima, H. & Akinaga, H. Mechanism of the performance improvement of TiO2-x-based field-effect transistor using SiO2 as gate insulator. AIP Adv. 1, 032167 (2011).
Hoffman, R. L. ZnO-channel thin-film transistors: channel mobility. J. Appl. Phys. 95, 5813–5819 (2004).
Park, J.-W., Han, S.-W., Jeon, N., Jang, J. & Yoo, S. Improved electrical characteristics of amorphous oxide TFTs based on $\hbox{TiO}_{x}$ channel layer grown by low-temperature MOCVD. IEEE Electron Device Lett. 29, 1319–1321 (2008).
Petti, L. et al. Metal oxide semiconductor thin-film transistors for flexible electronics. Appl. Phys. Rev. 3, 021303 (2016).
Fortunato, E., Barquinha, P. & Martins, R. Oxide semiconductor thin-film transistors: a review of recent advances. Adv. Mater. 24, 2945–2986 (2012).
Zhang, J. et al. Ultrathin-body TiO2 thin film transistors with record on-current density, on/off current ratio, and subthreshold swing via O2 annealing. IEEE Electron Device Lett. 40, 1463–1466 (2019).
Bubel, S., Meyer, S., Kunze, F. & Chabinyc, M. L. Ionic liquid gating reveals trap-filled limit mobility in low temperature amorphous zinc oxide. Appl. Phys. Lett. 103, 152102 (2013).
Lee, S. et al. Temperature dependent electron transport in amorphous oxide semiconductor thin film transistors. 2011 International Electron Devices Meeting 14.6.1-14.6.4 (IEEE, 2011). https://doi.org/10.1109/IEDM.2011.6131554.
Panayotov, D. A. & Yates, J. T. n-Type doping of TiO2 with atomic hydrogen-observation of the production of conduction band electrons by infrared spectroscopy. Chem. Phys. Lett. 436, 204–208 (2007).
Yamakata, A., Ishibashi, T. & Onishi, H. Time-resolved infrared absorption spectroscopy of photogenerated electrons in platinized TiO2 particles. Chem. Phys. Lett. 333, 271–277 (2001).
Antila, L. J., Santomauro, F. G., Hammarström, L., Fernandes, D. L. A. & Sá, J. Hunting for the elusive shallow traps in TiO2 anatase. Chem. Commun. 51, 10914–10916 (2015).
Han, B. & Hang, Hu,Y. Investigation on H-containing shallow trap of hydrogenated TiO2 with in situ Fourier transform infrared diffuse reflection spectroscopy. Nanotechnology 28, 304001 (2017).
Bharti, B., Kumar, S., Lee, H.-N. & Kumar, R. Formation of oxygen vacancies and Ti3+ state in TiO2 thin film and enhanced optical properties by air plasma treatment. Sci. Rep. 6, 32355 (2016).
Sanjinés, R. et al. Electronic structure of anatase TiO2 oxide. J. Appl. Phys. 75, 2945–2951 (1994).
Bertóti, I., Mohai, M., Sullivan, J. L. & Saied, S. O. Surface characterisation of plasma-nitrided titanium: an XPS study. Appl. Surf. Sci. 84, 357–371 (1995).
Meijer, E. J. et al. Switch-on voltage in disordered organic field-effect transistors. Appl. Phys. Lett. 80, 3838–3840 (2002).
Lustig, N., Kanicki, J., Wisnieff, R. & Griffith, J. Temperature dependent characteristics of hydrogenated amorphous silicon thin film transistors. MRS Proc. 118, 267 (1988).
Horowitz, G., Hajlaoui, M. E. & Hajlaoui, R. Temperature and gate voltage dependence of hole mobility in polycrystalline oligothiophene thin film transistors. J. Appl. Phys. 87, 4456–4463 (2000).
Vissenberg, M. C. J. M. & Matters, M. Theory of the field-effect mobility in amorphous organic transistors. Phys. Rev. B 57, 12964–12967 (1998).
Tanase, C., Meijer, E. J., Blom, P. W. M. & de Leeuw, D. M. Unification of the hole transport in polymeric field-effect transistors and light-emitting diodes. Phys. Rev. Lett. 91, 216601 (2003).
Tanase, C., Meijer, E. J., Blom, P. W. M. & de Leeuw, D. M. Local charge carrier mobility in disordered organic field-effect transistors. Org. Electron. 4, 33–37 (2003).
Torricelli, F. Charge transport in organic transistors accounting for a wide distribution of carrier energies—part I: theory. IEEE Trans. Electron Devices 59, 1514–1519 (2012).
Acknowledgements
The research was carried out at the Center for Functional Nanomaterials (CFN) and National Synchrotron Light Source II (NSLS-II) of Brookhaven National Laboratory (BNL), which are supported by the U.S. Department of Energy, Office of Basic Energy Sciences, under Contract No. DE-SC0012704. C.-Y.N. and S.S. acknowledge the financial support by the U.S. Department of Energy, Office of Energy Efficiency & Renewable Energy, under Contract No. DE-EE0008423.
Author information
Authors and Affiliations
Contributions
N.T. and A.S. prepared TiOx samples. N.T. carried out device fabrication and electrical measurements. A.S. and S.S. carried out XPS measurements. A.S. conducted XRD measurements. Z.D. and J.T.S. performed LEED measurements. N.T. and C.-Y.N. planned experiments, analyzed data, assimilated and prepared initial draft. C.-Y.N. and J.T.S. conceptualized and supervised the study. All the authors discussed, commented on the draft and participated in finalizing the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Peer review information Primary handling editor: Aldo Isidori.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Tiwale, N., Subramanian, A., Dai, Z. et al. Large mobility modulation in ultrathin amorphous titanium oxide transistors. Commun Mater 1, 94 (2020). https://doi.org/10.1038/s43246-020-00096-w
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s43246-020-00096-w
This article is cited by
-
Priming self-assembly pathways by stacking block copolymers
Nature Communications (2022)