Introduction

While the eigenvalues of a Hermitian Hamiltonian are always real, the Hermicity condition is more stringent than is strictly necessary1. It was shown by Bender and co-workers that Hamiltonians that obey parity-time (\({{{\mathcal{PT}}}}\)) symmetry can both admit real eigenvalues and describe physical systems2,3. The condition of combined space and time reflection symmetry has immediate utility for some open systems, where there is balanced loss into and gain from the surrounding environment. The application of the concept of \({{{\mathcal{PT}}}}\) symmetry into both classical and quantum physics has already led to some remarkable advances and unconventional phenomena, which cannot be captured with standard Hermitian Hamiltonians4,5,6,7,8.

An important concept within \({{{\mathcal{PT}}}}\) symmetry is that of exceptional points. Let us consider the simplest case of a pair of coupled oscillators, each of resonance frequency ω0 and interacting via the coupling constant g. The two resulting eigenfrequencies ω2 and ω1 are given by ω2,1 = ω0 ± g. After including gain at a rate κ into the first oscillator and an equivalent loss κ out of the second oscillator, the renormalized eigenfrequencies \({\omega }_{2}^{\prime}\) and \({\omega }_{1}^{\prime}\) of this \({{{\mathcal{PT}}}}\)-symmetric setup become \({\omega }_{2,1}^{\prime}={\omega }_{0}\pm \sqrt{{g}^{2}-{\kappa }^{2}/4}\) [see Supplementary Note 1]. The exceptional point (for this \({{{{{{{\mathcal{N}}}}}}}}=2\) oscillator system) is

$${\left(\frac{g}{\kappa }\right)}_{{{{{{{{\mathcal{N}}}}}}}} = 2}=\frac{1}{2},$$
(1)

which defines the crossover between the unbroken \({{{\mathcal{PT}}}}\) phase with wholly real \({\omega }_{2,1}^{\prime}\), and the broken phase with complex \({\omega }_{2,1}^{\prime}\). Therefore, by modulating the ratio g/κ one can induce a plethora of (sometimes unexpected) phenomena intrinsically linked to \({{{\mathcal{PT}}}}\) symmetry, for example in light transport where amplification and attenuation readily arise4,5,6,7,8.

Recently, optical and photonic systems have become popular playgrounds to test \({{{\mathcal{PT}}}}\)-symmetric effects in the laboratory9,10,11. Indeed, recent experiments in the area have seen non-reciprocal light propagation in coupled waveguides12, single-mode lasing in microring cavities13, extraordinary transmission in microtoroidal whispering-gallery-mode resonators14, and the development of hybrid optoelectronic devices15. In parallel, there has been much theoretical work on many-mode \({{{\mathcal{PT}}}}\)-symmetric systems16, including considerations of trimers17,18, quadrimers19,20,21,22,23,24,25,26,27,28,29, and more generally oligomers30,31,32,33,34,35,36,37,38,39,40,41,42,43,44,45.

Inspired by the pioneering experiments of Hodaei and co-workers with chains of ring-shaped optical resonators46, we develop a simple theory of short oligomer chains in an open quantum systems approach. In particular, we study dimer (\({{{{{{{\mathcal{N}}}}}}}}=2\)), trimer (\({{{{{{{\mathcal{N}}}}}}}}=3\)) and quadrimer (\({{{{{{{\mathcal{N}}}}}}}}=4\)) chains in detail [see also Supplementary Notes 1 and 2]. We derive the locations of the exceptional points, and explore the influence of the \({{{\mathcal{PT}}}}\) symmetry phase on both the population dynamics (revealing regions of amplification) and for correlations (showing areas of perfect coherence and incoherence). Our open quantum systems approach follows in the wake of a number of recent theoretical works47,48,49,50,51,52,53,54, which employ the concept of \({{{\mathcal{PT}}}}\) symmetry with quantum master equations. We note that a related and pioneering experiment with superconducting qubits has latterly been reported55, highlighting the timeliness of quantum \({{{\mathcal{PT}}}}\)-symmetry. We also uncover how the exceptional point of Eq. (1) is generalized for an oligomer chain of an arbitrary size \({{{{{{{\mathcal{N}}}}}}}}\), where there is gain into the first oscillator and an equivalent loss out of the last oscillator, with neutral oscillators in between. Using a transfer matrices approach, we derive an interesting scaling with \({{{{{{{\mathcal{N}}}}}}}}\) of \({(g/\kappa )}_{{{{{{{{\mathcal{N}}}}}}}}}\), and we find a feature due to the parity of the oligomer which provides tantalizing opportunities for experimental detection. Finally, we investigate the emergent and rich \({{{\mathcal{PT}}}}\) symmetry phase diagrams when long-range coupling (beyond nearest-neighbor) is taken into account, which crucially determines whether the exceptional point is of higher order (compared to the dimer case) or not.

Results and discussion

Trimer chain: model

Here, we look at the simplest nontrivial linear chain of harmonic oscillators: the trimer chain (that is, a \({{{{{{{\mathcal{N}}}}}}}}=3\) site oligomer). The trimer [which is sketched in Fig. 1a] already displays some interesting phenomena which is common across all odd-sited oligomers, and yet it retains some beauty due to its simplicity. The Hamiltonian operator \(\hat{H}\) for this system is (we set ℏ = 1 throughout this manuscript)

$$\hat{H}=\,{\omega }_{0}\left({b}_{1}^{{{{\dagger}}} }{b}_{1}+{b}_{2}^{{{{\dagger}}} }{b}_{2}+{b}_{3}^{{{{\dagger}}} }{b}_{3}\right)+g\left({b}_{1}^{{{{\dagger}}} }{b}_{2}+{b}_{2}^{{{{\dagger}}} }{b}_{3}+{{{{{{{\rm{h.c.}}}}}}}}\right),$$
(2)

where \({b}_{{{{{{\mathrm{n}}}}}}}^{{{{\dagger}}} }\) and bn, which satisfy bosonic commutation relations, are the creation and annihilation operators, respectively for site n. All of the oscillators are associated with the identical resonance frequency ω0, while the nearest-neighbor coupling strength between sites is given by g. Diagonalization of Eq. (2) leads to the three eigenfrequencies ωn, which read

$${\omega }_{3,1}={\omega }_{0}\pm \sqrt{2}g,$$
(3a)
$${\omega }_{2}={\omega }_{0}.$$
(3b)

This analysis reveals a solitary eigenfrequency ω2, which is unshifted from the bare resonance ω0, while the two other eigenfrequencies ω3 and ω1 exhibit a splitting of \(\sqrt{2}g\) from the central resonance. Incoherent processes in the chain are taken into account via a quantum master equation in Lindblad form56,57,58

$${\partial }_{{{{{{\mathrm{t}}}}}}}\rho ={{{{{{{\rm{i}}}}}}}}[\rho ,\hat{H}]+\mathop{\sum}\limits_{{{{{{\mathrm{n}}}}}}=1,2,3}\frac{{\gamma }_{{{{{{\mathrm{n}}}}}}}}{2}{{{{{{{\mathcal{L}}}}}}}}{b}_{{{{{{\mathrm{n}}}}}}}+\mathop{\sum}\limits_{{{{{{\mathrm{n}}}}}}=1,2,3}\frac{{P}_{{{{{{\mathrm{n}}}}}}}}{2}{{{{{{{{\mathcal{L}}}}}}}}}^{\prime}{b}_{{{{{{\mathrm{n}}}}}}},$$
(4)

where the two Lindblad superoperators are

$${{{{{{{\mathcal{L}}}}}}}}{b}_{{{{{{\mathrm{n}}}}}}}=2{b}_{{{{{{\mathrm{n}}}}}}}\rho {b}_{{{{{{\mathrm{n}}}}}}}^{{{{\dagger}}} }-{b}_{{{{{{\mathrm{n}}}}}}}^{{{{\dagger}}} }{b}_{{{{{{\mathrm{n}}}}}}}\rho -\rho {b}_{{{{{{\mathrm{n}}}}}}}^{{{{\dagger}}} }{b}_{{{{{{\mathrm{n}}}}}}},$$
(5a)
$${{{{{{{{\mathcal{L}}}}}}}}}^{\prime}{b}_{{{{{{\mathrm{n}}}}}}}=2{b}_{{{{{{\mathrm{n}}}}}}}^{{{{\dagger}}} }\rho {b}_{{{{{{\mathrm{n}}}}}}}-{b}_{{{{{{\mathrm{n}}}}}}}{b}_{{{{{{\mathrm{n}}}}}}}^{{{{\dagger}}} }\rho -\rho {b}_{{{{{{\mathrm{n}}}}}}}{b}_{{{{{{\mathrm{n}}}}}}}^{{{{\dagger}}} }.$$
(5b)

The unitary evolution is supplied by the commutator term on the right-hand-side of Eq. (4), where the Hamiltonian operator \(\hat{H}\) is given by Eq. (2). The external environment surrounding the chain allows for energy exchange. Losses are tracked by the first Lindbladian term in Eq. (4), where γn ≥ 0 is the damping decay rate of the nth oscillator into its heat bath. Incoherent gain processes, where Pn ≥ 0 is the pumping rate into oscillator n, are similarly modeled by the final term in Eq. (4).

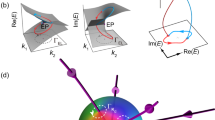
Fig. 1: The \({{{{{{{\mathcal{PT}}}}}}}}\)-symmetric trimer and its eigenfrequencies.
figure 1

a A cartoon of the three-site chain (colored balls), where each oscillator has the resonance frequency ω0. The left oscillator (green sphere) is subject to gain κ (yellow arrow), while the right oscillator (cyan sphere) suffers an equivalent loss κ (purple arrow), such that the arrangement fulfills \({{{{{{{\mathcal{PT}}}}}}}}\) symmetry. The coupling strength is g. b The real parts of the eigenfrequencies \({\omega }_{n}^{\prime}\), as a function of g [Eq. (11)]. c The imaginary parts. Dashed lines: exceptional points at the transition between the broken and unbroken \({{{{{{{\mathcal{PT}}}}}}}}\)-symmetric phases [Eq. (13)].

Full size image

In order to probe the mean-field dynamics of the chain, we exploit the property \(\langle {{{{{{{\mathcal{O}}}}}}}}\rangle ={{{{{{{\rm{Tr}}}}}}}}\ \left({{{{{{{\mathcal{O}}}}}}}}\rho \right)\) for some operator \({{{{{{{\mathcal{O}}}}}}}}\). The cyclic properties of the trace operator, along with the quantum master equation introduced Eq. (4), leads to the following Schrödinger-like equation for the first moments 〈bn〉 of the chain

$${{{{{{{\rm{i}}}}}}}}{\partial }_{t}\psi ={{{{{{{\mathcal{H}}}}}}}}\psi ,$$
(6)

where the three-dimensional Bloch vector ψ reads

$$\psi =\left(\begin{array}{l}\langle {b}_{1}\rangle \\ \langle {b}_{2}\rangle \\ \langle {b}_{3}\rangle \end{array}\right),$$
(7)

and with the 3 × 3 dynamical matrix \({{{{{{{\mathcal{H}}}}}}}}\), given by

$${{{{{{{\mathcal{H}}}}}}}}=\left(\begin{array}{lll}{\omega }_{0}-{{{{{{{\rm{i}}}}}}}}\frac{{{{\Gamma }}}_{1}}{2}&g&0\\ g&{\omega }_{0}-{{{{{{{\rm{i}}}}}}}}\frac{{{{\Gamma }}}_{2}}{2}&g\\ 0&g&{\omega }_{0}-{{{{{{{\rm{i}}}}}}}}\frac{{{{\Gamma }}}_{3}}{2}\end{array}\right).$$
(8)

In Eq. (8) we have introduced the renormalized damping decay rate Γn for each oscillator n, which is necessary due to the incoherent pumping Pn and the bosonic statistics. Explicitly, this quantity reads

$${{{\Gamma }}}_{{{{{{\mathrm{n}}}}}}}={\gamma }_{{{{{{\mathrm{n}}}}}}}-{P}_{{{{{{\mathrm{n}}}}}}}.$$
(9)

Let us now consider the specific chain configuration where the left oscillator is subject to gain via P1 = κ (and γ1 = 0), while the right oscillator is described by the equivalent loss γ3 = κ (and P3 = 0). The central oscillator is neutral (since P2 = γ2 = 0). Then the mean-field theory of Eq. (8) implies a \({{{\mathcal{PT}}}}\)-symmetric Hamiltonian \(\hat{H}^{\prime}\) may be written down as

$$\hat{H}^{\prime} =\, \left({\omega }_{0}+{{{{{{{\rm{i}}}}}}}}\frac{\kappa }{2}\right){b}_{1}^{{{{\dagger}}} }{b}_{1}+{\omega }_{0}{b}_{2}^{{{{\dagger}}} }{b}_{2}+\left({\omega }_{0}-{{{{{{{\rm{i}}}}}}}}\frac{\kappa }{2}\right){b}_{3}^{{{{\dagger}}} }{b}_{3}\\ +g\left({b}_{1}^{{{{\dagger}}} }{b}_{2}+{b}_{2}^{{{{\dagger}}} }{b}_{3}+{{{{{{{\rm{h.c.}}}}}}}}\right).$$
(10)

Equation (10) is indeed invariant under the necessary combined transformations of space (\({{{{{{{\mathcal{P}}}}}}}}\)) and time (\({{{{{{{\mathcal{T}}}}}}}}\)), essentially because the twin replacements i → −i and (1, 3) → (3, 1) leave \(\hat{H}^{\prime}\) unchanged. This \({{{\mathcal{PT}}}}\)-symmetric arrangement of the trimer chain is portrayed in Fig. 1a. Upon diagonalizing Eq. (10), the three eigenfrequencies \({\omega }_{n}^{\prime}\) are [cf. Eq. (3) for the closed system]

$${\omega }_{3,1}^{\prime}={\omega }_{0}\pm {{\Omega }},$$
(11a)
$${\omega }_{2}^{\prime}={\omega }_{0},$$
(11b)

where we have introduced the frequency Ω, where

$${{\Omega }}=\frac{1}{2}\sqrt{8{g}^{2}-{\kappa }^{2}}.$$
(12)

Equation (11) reveals the renormalization of the upper and lower eigenfrequencies \({\omega }_{3}^{\prime}\) and \({\omega }_{1}^{\prime}\), as compared to ω3 and ω1 in the closed system modeled in Eq. (3). This is due to the incoherent processes captured by κ. In particular, there is now an exceptional point located at

$${\left(\frac{g}{\kappa }\right)}_{{{{{{{{\mathcal{N}}}}}}}} = 3}=\frac{1}{2\sqrt{2}}\simeq 0.353...,$$
(13)

which marks the border between the regime when the \({{{\mathcal{PT}}}}\) Hamiltonian \(\hat{H}^{\prime}\) is in its unbroken phase with real eigenvalues, \(g\ge \kappa /(2\sqrt{2})\), and the broken phase with complex eigenvalues, \(g \; < \; \kappa /(2\sqrt{2})\) [cf. Eq. (1) for the dimer result, \({(g/\kappa )}_{{{{{{{{\mathcal{N}}}}}}}} = 2}\)].

We plot the \({{{\mathcal{PT}}}}\)-symmetric regime eigenfrequencies \({\omega }_{{{{{{\mathrm{n}}}}}}}^{\prime}\) in Fig. 1 using Eq. (11). The real parts are given in panel b, while the imaginary parts are displayed in panel c. The exceptional point of Eq. (13) is marked by the dashed gray line, and makes explicit the broken and unbroken \({{{\mathcal{PT}}}}\)-symmetric phases. There are several features of Fig. 1 which are shared amongst all odd-sited oligomers, namely: the purely real resonance frequency ω0 (orange line) is always a valid eigenfrequency; two eigenfrequencies always become complex in the broken \({{{\mathcal{PT}}}}\)-symmetric phase; and these two aforementioned eigenfrequencies are always the two eigenfrequencies closest to ω0 (neglecting the aforementioned, guaranteed ω0 eigensolution). Under the popular classification where an nth order exceptional point refers to when n eigenvalues coalesce at the exceptional point46, Fig. 1b, c exposes a higher order exceptional point of the 3rd order (compared to 2nd order for a dimer, see Supplementary Note 1). These remarks are further justified in Supplementary Note 2, where analogous behavior with the quadrimer chain (\({{{{{{{\mathcal{N}}}}}}}}=4\)) is analyzed in detail, and some features associated with all even-sited oligomers are discussed in Supplementary Note 3.

Trimer chain: dynamics

The equation of motion for the second moments \(\langle {b}_{{{{{{\mathrm{n}}}}}}}^{{{{\dagger}}} }{b}_{{{{{{\mathrm{m}}}}}}}\rangle\) of the trimer gives access to the mean populations along the chain, \(\langle {b}_{{{{{{\mathrm{n}}}}}}}^{{{{\dagger}}} }{b}_{{{{{{\mathrm{n}}}}}}}\rangle\). Similar to the calculation leading to Eq. (6), we obtain the first-order matrix differential equation

$$\frac{{{{{d}}}}}{{{{{{{{\rm{d}}}}}}}}t}{{{{{{{\bf{u}}}}}}}}={{{{{{{\bf{P}}}}}}}}-{{{{{{{\bf{M}}}}}}}}{{{{{{{\bf{u}}}}}}}},$$
(14)

for the 9-vector of correlators u and the inhomogenous pumping term P, where

$${{{{{{{\bf{u}}}}}}}}=\left(\begin{array}{l}{{{{{{{{\bf{u}}}}}}}}}_{1}\\ {{{{{{{{\bf{u}}}}}}}}}_{2}\\ {{{{{{{{\bf{u}}}}}}}}}_{2}^{{{{\dagger}}} }\end{array}\right),\quad {{{{{{{\bf{P}}}}}}}}=\left(\begin{array}{l}{P}_{1}\\ {P}_{2}\\ {P}_{3}\\ \,{{{{{{{{\boldsymbol{0}}}}}}}}}_{6}\end{array}\right),$$
(15)

where 0n is the zero matrix (of n-rows and a single column). The sub-vectors of u read

$${{{{{{{{\bf{u}}}}}}}}}_{1}=\left(\begin{array}{l}\langle {b}_{1}^{{{{\dagger}}} }{b}_{1}\rangle \\ \langle {b}_{2}^{{{{\dagger}}} }{b}_{2}\rangle \\ \langle {b}_{3}^{{{{\dagger}}} }{b}_{3}\rangle \end{array}\right),\quad {{{{{{{{\bf{u}}}}}}}}}_{2}=\left(\begin{array}{l}\langle {b}_{1}^{{{{\dagger}}} }{b}_{2}\rangle \\ \langle {b}_{2}^{{{{\dagger}}} }{b}_{3}\rangle \\ \langle {b}_{3}^{{{{\dagger}}} }{b}_{1}\rangle \\ \end{array}\right).$$
(16)

The matrix M of second moments in Eq. (14) is

$$\quad {{{{{{{\bf{M}}}}}}}}=\left(\begin{array}{lll}{{{{{{{{\bf{M}}}}}}}}}_{11}&{{{{{{{{\bf{M}}}}}}}}}_{12}&{{{{{{{{\bf{M}}}}}}}}}_{12}^{* }\\ {{{{{{{{\bf{M}}}}}}}}}_{12}^{{{{{{{{\rm{T}}}}}}}}}&{{{{{{{{\bf{M}}}}}}}}}_{22}&{{{{{{{{\bf{M}}}}}}}}}_{23}\\ {{{{{{{{\bf{M}}}}}}}}}_{12}^{{{{\dagger}}} }&{{{{{{{{\bf{M}}}}}}}}}_{23}^{* }&{{{{{{{{\bf{M}}}}}}}}}_{22}\end{array}\right),$$
(17)

where the on-diagonal sub-matrices comprising M are

$${{{{{{{{\bf{M}}}}}}}}}_{11}={{{{{{{\rm{diag}}}}}}}}\left({{{\Gamma }}}_{1},{{{\Gamma }}}_{2},{{{\Gamma }}}_{3}\right),$$
(18a)
$${{{{{{{{\bf{M}}}}}}}}}_{22}={{{{{{{\rm{diag}}}}}}}}\left(\frac{{{{\Gamma }}}_{1}+{{{\Gamma }}}_{2}}{2},\frac{{{{\Gamma }}}_{2}+{{{\Gamma }}}_{3}}{2},\frac{{{{\Gamma }}}_{3}+{{{\Gamma }}}_{1}}{2}\right),$$
(18b)

where Γn is defined in Eq. (9), while the two off-diagonal sub-matrices of M are defined by

$${{{{{{{{\bf{M}}}}}}}}}_{12}=\left(\begin{array}{lll}{{{{{{{\rm{i}}}}}}}}g&0&0\\ -{{{{{{{\rm{i}}}}}}}}g&{{{{{{{\rm{i}}}}}}}}g&0\\ 0&-{{{{{{{\rm{i}}}}}}}}g&0\end{array}\right),\,{{{{{{{{\bf{M}}}}}}}}}_{23}=\left(\begin{array}{lll}0&0&{{{{{{{\rm{i}}}}}}}}g\\ 0&0&-{{{{{{{\rm{i}}}}}}}}g\\ -{{{{{{{\rm{i}}}}}}}}g&{{{{{{{\rm{i}}}}}}}}g&0\end{array}\right).$$
(19)

In Eq. (17), the symbols *, †, and T represent taking the conjugate, conjugate transpose, and transpose, respectively.

Let us consider the \({{{\mathcal{PT}}}}\)-symmetric arrangement of the trimer, as sketched in Fig. 1a. In this special configuration, the nontrivial eigenvalues of the matrix M in Eq. (17) are \(\pm {{{{{{{\rm{i}}}}}}}}\sqrt{8{g}^{2}-{\kappa }^{2}}\) and \(\pm {{{{{{{\rm{i}}}}}}}}\sqrt{8{g}^{2}-{\kappa }^{2}}/2\), recovering the criticality first expounded at the level of the non-Hermitian Hamiltonian in Eq. (13). Using the frequency Ω as defined in Eq. (12), we find the following analytic expressions for the populations

$$\langle {b}_{1}^{{{\dagger}} }{b}_{1}\rangle =\frac{48{g}^{4}{{\Omega }}+32\kappa {g}^{2}{{{\Omega }}}^{2}\sin \left({{\Omega }}t\right)+\kappa \left({\kappa }^{4}-12{g}^{2}{\kappa }^{2}+32{g}^{4}\right)\sin \left(2{{\Omega }}t\right)+16{g}^{2}{{\Omega }}\left(4{g}^{2}-{\kappa }^{2}\right)\cos \left({{\Omega }}t\right)+{\tilde{{{\Omega }}}}^{5}\cos \left(2{{\Omega }}t\right)}{32{{{\Omega }}}^{5}},$$
(20a)
$$\langle {b}_{2}^{{{{\dagger}}} }{b}_{2}\rangle =\frac{{g}^{2}}{2{{{\Omega }}}^{5}}{\sin }^{2}\left(\frac{{{\Omega }}t}{2}\right)\left\{\right.8{g}^{2}{{\Omega }}+4\kappa {{{\Omega }}}^{2}\sin \left({{\Omega }}t\right)+2{{\Omega }}\left(4{g}^{2}-{\kappa }^{2}\right)\cos \left({{\Omega }}t\right)\left\}\right.,$$
(20b)
$$\langle {b}_{3}^{{{{\dagger}}} }{b}_{3}\rangle =\frac{4{g}^{4}}{{{{\Omega }}}^{4}}\sin \left(\frac{{{\Omega }}t}{2}\right),$$
(20c)

where \({\tilde{{{\Omega }}}}^{5}=2{{\Omega }}(8{g}^{4}-8{g}^{2}{\kappa }^{2}+{\kappa }^{4})\). Upon approaching the exceptional point (where Ω → 0), Eq. (20) reduces to the algebraically divergent \(\langle {b}_{1}^{{{{\dagger}}} }{b}_{1}\rangle ={(1+\kappa t/4)}^{4}\), \(\langle {b}_{2}^{{{{\dagger}}} }{b}_{2}\rangle ={(\kappa t/2)}^{2}{(1+\kappa t/4)}^{2}/2\), and \(\langle {b}_{3}^{{{{\dagger}}} }{b}_{3}\rangle ={(\kappa t/4)}^{4}\). Below the exceptional point, the trigonometric functions in Eq. (20) are superseded by hyperbolic functions, leading to exponential divergencies.

We plot the populations \(\langle {b}_{{{{{{\mathrm{n}}}}}}}^{{{{\dagger}}} }{b}_{{{{{{\mathrm{n}}}}}}}\rangle\) of the first, second and third oscillators (n = 1, 2, 3) as the thick green, medium orange and thin cyan lines in Fig. 2, using the solutions of Eq. (20). In Fig. 2a, where g = κ, a high frequency population cycle is observed, which is maintained over time due to the balanced loss and gain in the system. In panels b and c, where the coupling strength is reduced to g = 3κ/4 and g = κ/2, respectively, the frequency of the population cycle is successively reduced, while the maxima of the mean populations are increased due to the closening proximity to the exceptional point [cf. Eq. (13)]. The broken \({{{\mathcal{PT}}}}\) phase is exemplified in panel d, where \(g=0.35\kappa \; < \; \kappa /(2\sqrt{2})\), which displays the characteristically diverging population dynamics associated with breakdown beyond the exceptional point.

Fig. 2: Population dynamics.
figure 2

Evolution of the mean populations \(\langle {b}_{{{{{{\mathrm{n}}}}}}}^{{{{\dagger}}} }{b}_{{{{{{\mathrm{n}}}}}}}\rangle\) along the trimer chain, as a function of time t, in units of the inverse loss-gain parameter κ−1 [Eq. 20]. The coupling constant g reduces from above to below the exceptional point upon descending the column of panels [Eq. (13)]. a g = κ. b g = 3κ/4. c g = κ/2. d g = 0.35κ. The results for the first, second, and third oscillators are denoted by the thick green, medium orange and thin cyan lines respectively [see the legend in (d), which applies to the whole figure].

Full size image

Trimer chain: correlations

The temporal coherence can be quantified using the first-order correlation function56

$${g}_{{{{{{\mathrm{n}}}}}}}^{(1)}(\tau )=\mathop{\lim }\limits_{t\to \infty }\frac{\langle {b}_{{{{{{\mathrm{n}}}}}}}^{{{{\dagger}}} }(t){b}_{{{{{{\mathrm{n}}}}}}}(t+\tau )\rangle }{\langle {b}_{{{{{{\mathrm{n}}}}}}}^{{{{\dagger}}} }(t){b}_{{{{{{\mathrm{n}}}}}}}(t)\rangle },$$
(21)

where τ is the time delay, and where the normalization is taken over a long time scale t → ∞. This quantity has the property that perfect coherence is associated with \(| {g}_{{{{{{\mathrm{n}}}}}}}^{(1)}(\tau )| =1\) and complete incoherence corresponds to \(| {g}_{{{{{{\mathrm{n}}}}}}}^{(1)}(\tau )| =0\), while intermediate cases specify the degree of partial coherence. The manipulations resulting in Eq. (6), and an application of the quantum regression theorem, lead to an equation for the first desired two-time correlator \(\langle {b}_{1}^{{{{\dagger}}} }(t){b}_{1}(t+\tau )\rangle\), via

$${\partial }_{\tau }{{{{{{{\bf{v}}}}}}}}+{{{{{{{\bf{Q}}}}}}}}{{{{{{{\bf{v}}}}}}}}=0,\quad \quad {{{{{{{\bf{v}}}}}}}}=\left(\begin{array}{l}\langle {b}_{1}^{{{{\dagger}}} }(t){b}_{1}(t+\tau )\rangle \\ \langle {b}_{1}^{{{{\dagger}}} }(t){b}_{2}(t+\tau )\rangle \\ \langle {b}_{1}^{{{{\dagger}}} }(t){b}_{3}(t+\tau )\rangle \\ \end{array}\right),$$
(22)

with the 3 × 3 regression matrix

$${{{{{{{\bf{Q}}}}}}}}=\left(\begin{array}{lll}{{{{{{{\rm{i}}}}}}}}{\omega }_{0}+\frac{{{{\Gamma }}}_{1}}{2}&{{{{{{{\rm{i}}}}}}}}g&0\\ {{{{{{{\rm{i}}}}}}}}g&{{{{{{{\rm{i}}}}}}}}{\omega }_{0}+\frac{{{{\Gamma }}}_{2}}{2}&{{{{{{{\rm{i}}}}}}}}g\\ 0&{{{{{{{\rm{i}}}}}}}}g&{{{{{{{\rm{i}}}}}}}}{\omega }_{0}+\frac{{{{\Gamma }}}_{3}}{2}\end{array}\right).$$
(23)

Similar equations may be derived for \(\langle {b}_{2}^{{{{\dagger}}} }(t){b}_{2}(t+\tau )\rangle\) and \(\langle {b}_{3}^{{{{\dagger}}} }(t){b}_{3}(t+\tau )\rangle\). The solution of Eq. (22), along with the definition of Eq. (21), leads to the sought after first-order correlation functions. In the \({{{\mathcal{PT}}}}\)-symmetric setup of trimer, as drawn in Fig. 1a, one finds the neat expressions

$${g}_{1}^{(1)}(\tau )={g}_{3}^{(1)}(\tau )=\frac{8{g}^{2}{\cos }^{2}\left(\frac{{{\Omega }}\tau }{2}\right)-{\kappa }^{2}}{4{{{\Omega }}}^{2}}{{{{{{{{\rm{e}}}}}}}}}^{-{{{{{{{\rm{i}}}}}}}}{\omega }_{0}\tau },$$
(24a)
$${g}_{2}^{(1)}(\tau )=\frac{4{g}^{2}{\kappa }^{2}-{\kappa }^{4}+32{g}^{4}\cos \left({{\Omega }}\tau \right)}{4{g}^{2}{\kappa }^{2}-{\kappa }^{4}+32{g}^{4}}{{{{{{{{\rm{e}}}}}}}}}^{-{{{{{{{\rm{i}}}}}}}}{\omega }_{0}\tau },$$
(24b)

which characteristically include the harmonic component \({{{{{{{{\rm{e}}}}}}}}}^{-{{{{{{{\rm{i}}}}}}}}{\omega }_{0}\tau }\), representing a monochromatic field centered on ω0, and a pre-factor accounting for the specific \({{{\mathcal{PT}}}}\)-symmetric setup of the trimer. In the limit of Ω → 0, that is approaching the exceptional point \(g\to \kappa /(2\sqrt{2})\), Eq. (24) tends towards the quadratically divergent results \({g}_{1,3}^{(1)}(\tau )\to \{1-{\kappa }^{2}{\tau }^{2}/16\}{{{{{{{{\rm{e}}}}}}}}}^{-{{{{{{{\rm{i}}}}}}}}{\omega }_{0}\tau }\) and \({g}_{2}^{(1)}(\tau )\to \{1-{\kappa }^{2}{\tau }^{2}/24\}{{{{{{{{\rm{e}}}}}}}}}^{-{{{{{{{\rm{i}}}}}}}}{\omega }_{0}\tau }\). For coupling strengths below the exceptional point the trigonometric functions are replaced with hyperbolic functions, indicating exponentially divergent behavior. We plot the real parts of the coherences \({g}_{1}^{(1)}(\tau )\) and \({g}_{2}^{(1)}(\tau )\) of the first and second oscillators as the thick green and thin orange lines in Fig. 3, using the solutions of Eq. (24). In Fig. 3a, well above the exceptional point at g = κ, the \({{{\mathcal{PT}}}}\) symmetry ensures an undamped periodic response, with rapid oscillations and a dynamic behavior satisfying \(0 \; < \; | {g}_{{{{{{\mathrm{n}}}}}}}^{(1)}(\tau )| \; < \; 1\). Exactly at g = κ/2, where all three coherences are accidentally equal as shown in panel b, a well-defined wave envelope develops. In panel c, at the exceptional point \(g=\kappa /(2\sqrt{2})\), there is initially regular, high frequency oscillations due to short time behavior being essentially dominated by the zeroth order term \({g}_{{{{{{\mathrm{n}}}}}}}^{(1)}(\tau )\simeq {{{{{{{{\rm{e}}}}}}}}}^{-{{{{{{{\rm{i}}}}}}}}{\omega }_{0}\tau }\). Once the quadratic correction in κt becomes non-negligible, the divergence characteristic of the broken \({{{\mathcal{PT}}}}\) symmetric phase finally emerges.

Fig. 3: Dynamics of the correlations.
figure 3

Evolution of the real part of the first-order correlation function g(1)(τ), as a function of the time delay τ, in units of the inverse loss-gain parameter κ−1 [Eq. (24)]. a–c The coupling constant g is reduced from above [a, b] to at c the exceptional point located at \(g=\kappa /(2\sqrt{2})\). The results for the first and second oscillators are denoted by the thick green and thin orange lines respectively [see the legend in c, which applies to the whole figure]. In the figure, ω0 = 20κ.

Full size image

Trimer chain: long-range coupling

Let us now consider the effects of going beyond the nearest-neighbor coupling approximation employed in Eq. (2). To do so, we introduce the second-nearest neighbor coupling constant h, which connects the first and third oscillators, via the generalized Hamiltonian \(\hat{H}^{\prime} =\hat{H}+h({b}_{1}^{{{{\dagger}}} }{b}_{3}+{b}_{3}^{{{{\dagger}}} }{b}_{1})\), where \(\hat{H}\) is defined in Eq. (2). This extension leads to a generalization of the eigenfrequencies of Eq. (3) to \({\tilde{\omega }}_{{{{{{\mathrm{n}}}}}}}\), where

$${\tilde{\omega }}_{3,1}={\omega }_{0}+\frac{h}{2}\pm \frac{1}{2}\sqrt{8{g}^{2}+{h}^{2}},$$
(25a)
$$\tilde{{\omega }_{2}}={\omega }_{0}-h.$$
(25b)

The associated \({{{\mathcal{PT}}}}\)-symmetric setup of trimer chain is sketched in Fig. 4a [cf. Fig. 1a]. The resulting eigenfrequencies \({\tilde{\omega }}_{{{{{{\mathrm{n}}}}}}}^{\prime}\) are [cf. Eq. (11)]

$${\tilde{\omega }}_{3}^{\prime}={\omega }_{0}+\sqrt{\frac{8{g}^{2}+4{h}^{2}-{\kappa }^{2}}{3}}\cos \left(\frac{\alpha }{3}\right),$$
(26a)
$${\tilde{\omega }}_{2}^{\prime}={\omega }_{0}+\sqrt{\frac{8{g}^{2}+4{h}^{2}-{\kappa }^{2}}{3}}\cos \left(\frac{\alpha +4\pi }{3}\right),$$
(26b)
$${\tilde{\omega }}_{1}^{\prime}={\omega }_{0}+\sqrt{\frac{8{g}^{2}+4{h}^{2}-{\kappa }^{2}}{3}}\cos \left(\frac{\alpha +2\pi }{3}\right),$$
(26c)

where we have introduced the quantity

$$\alpha =\arccos \left(\frac{24\sqrt{3}{g}^{2}h}{{\left(8{g}^{2}+4{h}^{2}-{\kappa }^{2}\right)}^{3/2}}\right).$$
(27)

The inclusion of second-nearest neighbor coupling h leads to a significantly richer phase diagram than with nearest-neighbor coupling only, as is demonstrated in Fig. 4 (b). Notably, when h = 0 Eq. (13) is recovered, so that above this threshold strength of \(1/(2\sqrt{2})\) the system is in its unbroken phase. With increasing h, the exceptional point (g/κ)3 increases in value, up until h = κ/2. Above this critical point, the unbroken phase can be explored either with weak enough g, or strong enough g, with a region of broken phase in between. This causes a green stripe in the phase diagram of Fig. 4b, which notably contains the equal coupling (h = g) ring-like limit. The aforementioned broken-unbroken transitions from above and from below can be explicitly seen in Fig. 5, where the real and imaginary parts of \({\tilde{\omega }}_{{{{{{\mathrm{n}}}}}}}^{\prime}\) are shown, as a function of g/κ, in the upper and lower rows respectively. In the first column of Fig. 5, one notices how a nonzero second-nearest neighbor coupling (h = κ/4) has led to a larger exceptional point of (g/κ)3 ≃ 0.660, compared to the nearest-neighbor coupling case when (g/κ)3 ≃ 0.353. The middle column, at the critical point of h = κ/2, shows the onset of a region of unbroken \({{{\mathcal{PT}}}}\) phase for vanishingly small g. This region is even more apparent in the final column of Fig. 5, where h = 3κ/4 and the exceptional point is well below the nearest-neighbor value, being (g/κ)3 ≃ 0.295. Across all of these cases, it is most apparent that the higher (3rd order) exceptional point of the trimer with nearest-neighbor coupling only [cf. Fig. 1b, c] has been downgraded to a standard 2nd order exceptional point in Fig. 5. This is due to the long-range interactions perturbing the eigensolution otherwise residing exactly at ω0. Similarly rich features due to long-range interactions are also seen in the quadrimer chain (\({{{{{{{\mathcal{N}}}}}}}}=4\)), as is demonstrated in Supplementary Note 2.

Fig. 4: The effect of long-range interactions.
figure 4

a A sketch of the \({{{{{{{\mathcal{PT}}}}}}}}\)-symmetric trimer (colored spheres) beyond nearest-neighbor coupling, where the first-neighbor coupling constant is g, and the second-neighbor coupling constant is h. The first oscillator (green sphere) is subject to gain κ (yellow arrow), and the final oscillator (cyan sphere) to loss κ (purple arrow). b The \({{{{{{{\mathcal{PT}}}}}}}}\) symmetry phase diagram of the trimer, given by the evolution of the exceptional point (g/κ)3 with the first and second-neighbor couplings g and h, both in units of κ [Eq. (26)]. White: unbroken phase. Green: broken phase.

Full size image
Fig. 5: The influence of long-range interactions on the exceptional points of the trimer.
figure 5

a–c The real parts of the eigenfrequencies \({\tilde{\omega }}_{{{{{{\mathrm{n}}}}}}}^{\prime}\), as a function of the coupling strength g [Eq. (26)]. d–f The imaginary parts, corresponding to the real parts in a–c. Dashed lines: the exceptional points denote the border between broken and unbroken \({{{{{{{\mathcal{PT}}}}}}}}\)-symmetric phases. The results for the first, second and third eigenfrequencies \({\tilde{\omega }}_{{{{{{\mathrm{n}}}}}}}^{\prime}\) are denoted by the thin green, medium orange and thick cyan lines respectively [see the legend in a, which applies to the whole figure]. In the first, second, and third columns, the second-nearest neighbor coupling constant h = κ/4, κ/2, and 3κ/4, respectively.

Full size image

Oligomer chains

We have seen some fundamental properties of short \({{{\mathcal{PT}}}}\)-symmetric oligomer chains (specifically for \({{{{{{{\mathcal{N}}}}}}}}=3\), and for \({{{{{{{\mathcal{N}}}}}}}}=2\) and \({{{{{{{\mathcal{N}}}}}}}}=4\) in the Supplementary Notes 1 and 2). Let us now consider a general oligomer of arbitrary size \({{{{{{{\mathcal{N}}}}}}}}\), with nearest-neighbor coupling only. The eigenfrequencies read

$${\omega }_{n}({{{{{{{\mathcal{N}}}}}}}})={\omega }_{0}+2g\cos \left(\frac{n\pi }{{{{{{{{\mathcal{N}}}}}}}}+1}\right),$$
(28)

where the index \(n\in [1,{{{{{{{\mathcal{N}}}}}}}}]\) labels each mode [such that the specific results for \({{{{{{{\mathcal{N}}}}}}}}=3\) reproduce Eq. (3)]. If we generalize the \({{{\mathcal{PT}}}}\)-symmetric arrangement of Fig. 1a, that is, if we allow for gain into the first oscillator and an equivalent loss out of the \({{{{{{{\mathcal{N}}}}}}}}\)th oscillator in the chain, so that the setup is [gain]−[neutral]\({}_{{{{{{{{\mathcal{N}}}}}}}}-2}\)−[loss], we can find how the exceptional point \({(g/\kappa )}_{{{{{{{{\mathcal{N}}}}}}}}}\) evolves as a function of \({{{{{{{\mathcal{N}}}}}}}}\). The result of this diagonalization of a chain of an arbitrary \({{{{{{{\mathcal{N}}}}}}}}\)-oscillator oligomer is derived in Supplementary Note 3, using a transfer matrix method59,60,61. This procedure leads to the rather beautiful expression [cf. Eqs. (1 and 13)]

$${\left(\frac{g}{\kappa }\right)}_{{{{{{{{\mathcal{N}}}}}}}}}=\left\{\begin{array}{ll}\frac{1}{2},&{{{{{{{\mathcal{N}}}}}}}}=2,4,6,...\\ \frac{1}{2}\sqrt{\frac{{{{{{{{\mathcal{N}}}}}}}}\;-\;1}{{{{{{{{\mathcal{N}}}}}}}}\;+\;1}},&{{\mbox{}}}{{{{{{{\mathcal{N}}}}}}}}=3,5,7,...\end{array}\right.$$
(29)

We display graphically the formula of Eq. (29) in Fig. 6. Most notably, for oligomers of even size \({{{{{{{\mathcal{N}}}}}}}}\) (red circles), the exceptional point is constant at \({(g/\kappa )}_{{{{{{{{\mathcal{N}}}}}}}}}=1/2\), and is of 2nd order. However, oligomers of odd size \({{{{{{{\mathcal{N}}}}}}}}\) (green circles) are associated with smaller exceptional points than the celebrated dimer result, and are of higher (3rd) order. These exceptional points are bounded by the limiting cases of the trimer result of \({(g/\kappa )}_{3}=1/(2\sqrt{2})\simeq 0.353...\) and the infinitely long chain result of (g/κ)∞ = 1/2, as shown in Fig. 6 for chains up to \({{{{{{{\mathcal{N}}}}}}}}=20\) oscillators. In particular, the even-odd behavior shown in Fig. 6 is ripe for future experimental detection, as is the trend for increasing large values of the exceptional point with increasingly long odd-numbered chains, following the trend encapsulated by Eq. (29), and its inverse-linear asymptotics \({(g/\kappa )}_{{{{{{{{\mathcal{N}}}}}}}}}\simeq (1-{{{{{{{{\mathcal{N}}}}}}}}}^{-1})/2\) for large \({{{{{{{\mathcal{N}}}}}}}}\). While we do not account for disorder, or for dimerization of the chain (which may be interesting from a topological point of view62), such extensions can be readily taken care of within this framework.

Fig. 6: Evolution of the exceptional point in arbitrary chains.
figure 6

The exceptional point \({(g/\kappa )}_{{{{{{{{\mathcal{N}}}}}}}}}\) as a function of the number of sites \({{{{{{{\mathcal{N}}}}}}}}\) in the oligomer chain [Eq. (29)]. Results with even (odd) \({{{{{{{\mathcal{N}}}}}}}}\) are associated with red (green) circles.

Full size image

The addition of next-nearest neighbor hoppings to oligomers of an arbitrary length allows us to generalize our investigation of long-range interactions in a short trimer chain [cf. Fig. 4b]. Similar to the \({{{{{{{\mathcal{N}}}}}}}}=3\) case, we can map the phase diagram marking the regions of broken (colored) and unbroken (white) \({{{\mathcal{PT}}}}\) symmetric phase, as is shown in Fig. 7a–d for oligomers of length \({{{{{{{\mathcal{N}}}}}}}}=\{4,5,6,7\}\). The two relevant parameters are the first and second-neighbor coupling strengths g and h, such that the vertical axis (h = 0) is marked with analytic results from Eq. (29). Away from this point, the influence of nonzero next-nearest neighbor hopping is rather profound: leading to seas (and even enclaves) of unbroken \({{{\mathcal{PT}}}}\) symmetry in a variety of geometries. Recent advances with so-called programmable interactions in atomic arrays suggest that the experimental exploration of such phase diagrams is increasingly accessible63, aside from the demonstrated tunable interaction ranges in trapped atomic ions64,65.

Fig. 7: Phase diagrams of oligomer chains with long-range interactions.
figure 7

a–d \({{{{{{{\mathcal{PT}}}}}}}}\) symmetry phase diagrams of oligomer chains of size \({{{{{{{\mathcal{N}}}}}}}}=\{4,5,6,7\}\), given by the evolution of the exceptional point \({(g/\kappa )}_{{{{{{{{\mathcal{N}}}}}}}}}\), as a function of the first and second-neighbor couplings g and h, both in units of κ. White: unbroken phase. Colors: broken phase. The analytic results in the h → 0 limit are marked on the vertical axis [Eq. (29)]. The \({{{{{{{\mathcal{N}}}}}}}}=3\) case was already presented in Fig. 4b.

Full size image

Conclusions

We have considered some fundamental properties of oligomers of an arbitrary size which satisfy \({{{\mathcal{PT}}}}\) symmetry due to having gain into the first oscillator and an equivalent loss out of the final oscillator. We have unveiled analytically the behavior of the exceptional points as a function of the chain length, which governs the stability of the population dynamics in the system and the presence of amplification. In particular, we have reported an even-odd effect for oligomers of increasing size, derived the bounds on all possible exceptional points, and mapped the relevant phase diagrams when long-range interactions are taken into account.

Focusing on short oligomers, we have provided simple quantum theories locating their exceptional points, and in doing so we found unconventional population dynamics and interesting first-order coherences near to the unbroken-broken \({{{\mathcal{PT}}}}\)-symmetric phases. We have also discussed effects beyond nearest-neighbor coupling, which leads to rich \({{{\mathcal{PT}}}}\) symmetry phase diagrams. In particular, we have shown that reaching the unbroken \({{{\mathcal{PT}}}}\) symmetric phase is no longer purely dependent on going above a threshold value of coupling-to-dissipation strength g/κ, rather one may also go below a different threshold value, such that the broken phase can live in a sweet-spot in-between.

Our versatile theory is relevant across a number of optical and photonic platforms, including coupled ring resonators66, coupled cavities67, coupled waveguides68,69, and meta-atoms70. Our theoretical results provide a route-map for the scaling up of \({{{\mathcal{PT}}}}\)-symmetric systems, and paves the way for the observation of cooperative effects in arbitrarily large systems. There are clear perspectives for the experimental detection of our predictions, including finite size effects, even-odd behaviors, unconventional light transport and correlations, and long-range interactions leading to sweet spot regions of \({{{\mathcal{PT}}}}\) symmetry phase breakdown.

Methods

In this theoretical work, the methods used are quantum master equations (as described in the main text [cf. Eq. (4)] and Supplementary Note 1), and an extended transfer matrices method (as detailed in Supplementary Note 3).