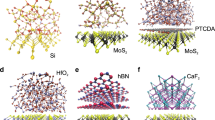
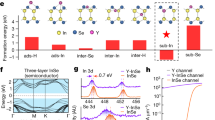
Abstract
Complementary metal–oxide–semiconductor (CMOS) logic circuits at their ultimate scaling limits place extreme demands on the properties of all materials involved. The requirements for semiconductors are well explored and could possibly be satisfied by a number of layered two-dimensional (2D) materials, such as transition metal dichalcogenides or black phosphorus. The requirements for gate insulators are arguably even more challenging. At present, hexagonal boron nitride (hBN) is the most common 2D insulator and is widely considered to be the most promising gate insulator in 2D material-based transistors. Here we assess the material parameters and performance limits of hBN. We compare experimental and theoretical tunnel currents through ultrathin layers (equivalent oxide thickness of less than 1 nm) of hBN and other 2D gate insulators, including the ideal case of defect-free hBN. Though its properties make hBN a candidate for many applications in 2D nanoelectronics, excessive leakage currents lead us to conclude that hBN is unlikely to be suitable for use as a gate insulator in ultrascaled CMOS devices.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 digital issues and online access to articles
$119.00 per year
only $9.92 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout





Similar content being viewed by others
Data availability
The data that support the findings of this study are available from the corresponding authors upon reasonable request.
Code availability
For the Tsu–Esaki/WKB calculations, we used the Comphy code, which is publicly available from https://comphy.eu/ (ref. 39). For the full-band transport simulations, the matrices were calculated with the CP2K package, which is publicly available from https://www.cp2k.org/ (ref. 44). These matrices were loaded into the quantum transport solver OMEN, as described at https://www.cp2k.org/howto:cp2k_omen (ref. 45). Both code packages can be downloaded from https://github.com/cp2k/cp2k.
References
Chhowalla, M., Jena, D. & Zhang, H. Two-dimensional semiconductors for transistors. Nat. Rev. Mater. 1, 16052 (2016).
Akinwande, D. et al. Graphene and two-dimensional materials for silicon technology. Nature 573, 507–518 (2019).
Geim, A. K. & Grigorieva, I. V. Van der Waals heterostructures. Nature 499, 419–425 (2013).
Liu, Y. et al. Van der Waals heterostructures and devices. Nat. Rev. Mater. 1, 16042 (2016).
Dean, C. R. et al. Boron nitride substrates for high-quality graphene electronics. Nat. Nanotechnol. 5, 722–726 (2010).
Zhang, K., Feng, Y., Wang, F., Yang, Z. & Wang, J. Two dimensional hexagonal boron nitride (2D-hBN): synthesis, properties and applications. J. Mater. Chem. C 5, 11992–12022 (2017).
Rhodes, D., Chae, S. H., Ribeiro-Palau, R. & Hone, J. Disorder in van der Waals heterostructures of 2D materials. Nat. Mater. 18, 541–549 (2019).
Britnell, L. et al. Electron tunneling through ultrathin boron nitride crystalline barriers. Nano Lett. 12, 1707–1710 (2012).
Ji, Y. et al. Boron nitride as two dimensional dielectric: reliability and dielectric breakdown. Appl. Phys. Lett. 108, 012905 (2016).
Chen, T. A. et al. Wafer-scale single-crystal hexagonal boron nitride monolayers on Cu (111). Nature 579, 219–223 (2020).
Taniguchi, T. & Watanabe, K. Synthesis of high-purity boron nitride single crystals under high pressure by using Ba–BN solvent. J. Cryst. Growth 303, 525–529 (2007).
Paciĺ, D., Meyer, J. C., Girit, Ç. & Zettl, A. The two-dimensional phase of boron nitride: few-atomic-layer sheets and suspended membranes. Appl. Phys. Lett. 92, 133107 (2008).
Shi, Z. et al. Vapor–liquid–solid growth of large-area multilayer hexagonal boron nitride on dielectric substrates. Nat. Commun. 11, 849 (2020).
Lee, J. et al. Atomic layer deposition of layered boron nitride for large-area 2D electronics. ACS Appl. Mater. Interfaces 12, 36688–36694 (2020).
Kim, K. K. et al. Synthesis of monolayer hexagonal boron nitride on Cu foil using chemical vapor deposition. Nano Lett. 12, 161–166 (2012).
Jang, S. K., Youn, J., Song, Y. J. & Lee, S. Synthesis and characterization of hexagonal boron nitride as a gate dielectric. Sci. Rep. 6, 30449 (2016).
Kim, S. M. et al. Synthesis of large-area multilayer hexagonal boron nitride for high material performance. Nat. Commun. 6, 8662 (2015).
Li, X. et al. Large-area two-dimensional layered hexagonal boron nitride grown on sapphire by metalorganic vapor phase epitaxy. Cryst. Growth Des. 16, 3409–3415 (2016).
Elias, C. et al. Direct band-gap crossover in epitaxial monolayer boron nitride. Nat. Commun. 10, 2639 (2019).
Wang, L. et al. One-dimensional electrical contact to a two-dimensional material. Science 342, 614–617 (2013).
Cui, X. et al. Multi-terminal transport measurements of MoS2 using a van der Waals heterostructure device platform. Nat. Nanotechnol. 10, 534–540 (2015).
Baugher, B. W. H., Churchill, H. O. H., Yang, Y. & Jarillo-Herrero, P. Intrinsic electronic transport properties of high quality monolayer and bilayer MoS2. Nano Lett. 13, 4212–4216 (2013).
Ma, N. & Jena, D. Charge scattering and mobility in atomically thin semiconductors. Phys. Rev. X 4, 011043 (2014).
Konar, A., Fang, T. & Jena, D. Effect of high-κ gate dielectrics on charge transport in graphene-based field effect transistors. Phys. Rev. B 82, 115452 (2010).
Cadiz, F. et al. Excitonic linewidth approaching the homogeneous limit in MoS2-based van der Waals heterostructures. Phys. Rev. X 7, 021026 (2017).
Cao, Y. et al. Unconventional superconductivity in magic-angle graphene superlattices. Nature 556, 43–50 (2018).
Shi, Y. et al. Electronic synapses made of layered two-dimensional materials. Nat. Electron. 1, 458–465 (2018).
Akinwande, D., Petrone, N. & Hone, J. Two-dimensional flexible nanoelectronics. Nat. Commun. 5, 5678 (2014).
Hong, S. et al. Ultralow-dielectric-constant amorphous boron nitride. Nature 582, 511–514 (2020).
Palumbo, F. et al. A review on dielectric breakdown in thin dielectrics: silicon dioxide, high-k, and layered dielectrics. Adv. Funct. Mater. 30, 1900657 (2020).
Chandni, U., Watanabe, K., Taniguchi, T. & Eisenstein, J. P. Evidence for defect-mediated tunneling in hexagonal boron nitride-based junctions. Nano Lett. 15, 7329–7333 (2015).
Weston, L., Wickramaratne, D., Mackoit, M., Alkauskas, A. & Van De Walle, C. G. Native point defects and impurities in hexagonal boron nitride. Phys. Rev. B 97, 214104 (2018).
Greenaway, M. T. et al. Tunnel spectroscopy of localised electronic states in hexagonal boron nitride. Commun. Phys. 1, 94 (2018).
Strand, J., Larcher, L. & Shluger, A. L. Properties of intrinsic point defects and dimers in hexagonal boron nitride. J. Phys. Condens. Matter 32, 055706 (2020).
Jin, C., Lin, F., Suenaga, K. & Iijima, S. Fabrication of a freestanding boron nitride single layer and its defect assignments. Phys. Rev. Lett. 102, 195505 (2009).
Wong, D. et al. Characterization and manipulation of individual defects in insulating hexagonal boron nitride using scanning tunnelling microscopy. Nat. Nanotechnol. 10, 949–953 (2015).
IEEE International Roadmap for Devices and Systems—More Moore Technical Report (IEEE, 2020).
Tsu, R. & Esaki, L. Tunneling in a finite superlattice. Appl. Phys. Lett. 22, 562–564 (1973).
Rzepa, G. et al. Comphy—a compact-physics framework for unified modeling of BTI. Microelectron. Reliab. 85, 49–65 (2018).
Geick, R., Perry, C. & Rupprecht, G. Normal modes in hexagonal boron nitride. Phys. Rev. 146, 543–547 (1966).
Laturia, A., Van de Put, M. L. & Vandenberghe, W. G. Dielectric properties of hexagonal boron nitride and transition metal dichalcogenides: from monolayer to bulk. npj 2D Mater. Appl. 2, 6 (2018).
Xu, Y. N. & Ching, W. Y. Calculation of ground-state and optical properties of boron nitrides in the hexagonal, cubic, and wurtzite structures. Phys. Rev. B 44, 7787–7798 (1991).
Heyd, J., Scuseria, G. E. & Ernzerhof, M. Hybrid functionals based on a screened Coulomb potential. J. Chem. Phys. 118, 8207–8215 (2003).
Kühne, T. D. et al. CP2K: an electronic structure and molecular dynamics software package—Quickstep: efficient and accurate electronic structure calculations. J. Chem. Phys. 152, 194103 (2020).
Brück, S., Calderara, M., Bani-Hashemian, M. H., VandeVondele, J. & Luisier, M. Efficient algorithms for large-scale quantum transport calculations. J. Chem. Phys. 147, 074116 (2017).
Wen, C. et al. Dielectric properties of ultrathin CaF2 ionic crystals. Adv. Mater. 32, 2002525 (2020).
Appenzeller, J., Radosavljević, M., Knoch, J. & Avouris, P. Tunneling versus thermionic emission in one-dimensional semiconductors. Phys. Rev. Lett. 92, 048301 (2004).
Qiu, C. et al. Dirac-source field-effect transistors as energy-efficient, high-performance electronic switches. Science 361, 387–392 (2018).
Salahuddin, S. & Datta, S. Use of negative capacitance to provide voltage amplification for low power nanoscale devices. Nano Lett. 8, 405–410 (2008).
Britnell, L. et al. Field-effect tunneling transistor based on vertical graphene heterostructures. Science 335, 947–950 (2012).
Illarionov, Y. Y. et al. Insulators for 2D nanoelectronics: the gap to bridge. Nat. Commun. 11, 3385 (2020).
Low, C. G. & Zhang, Q. Ultra-thin and flat mica as gate dielectric layers. Small 8, 2178–2183 (2012).
Kim, H. J. et al. Hunting for monolayer oxide nanosheets and their architectures. Sci. Rep. 6, 19402 (2016).
Shin, G. H. et al. High-performance field-effect transistor and logic gates based on GaS-MoS2 van der Waals heterostructure. ACS Appl. Mater. Interfaces 12, 5106–5112 (2020).
Holler, B. A., Crowley, K., Berger, M. H. & Gao, X. P. 2D semiconductor transistors with van der Waals oxide MoO3 as integrated high-κ gate dielectric. Adv. Electron. Mater. 6, 2000635 (2020).
Chamlagain, B. et al. Thermally oxidized 2D TaS2 as a high-κ gate dielectric for MoS2 field-effect transistors. 2D Mater. 4, 031002 (2017).
Li, T. et al. A native oxide high-κ gate dielectric for two-dimensional electronics. Nat. Electron. 3, 473–478 (2020).
Peimyoo, N. et al. Laser-writable high-k dielectric for van der Waals nanoelectronics. Sci. Adv. 5, eaau0906 (2019).
Mleczko, M. J. et al. HfSe2 and ZrSe2: two-dimensional semiconductors with native high-κ oxides. Sci. Adv. 3, e1700481 (2017).
Dean, C. R. et al. Hofstadter’s butterfly and the fractal quantum Hall effect in moiré superlattices. Nature 497, 598–602 (2013).
Gaskell, J. et al. Graphene-hexagonal boron nitride resonant tunneling diodes as high-frequency oscillators. Appl. Phys. Lett. 107, 103105 (2015).
Caldwell, J. D. et al. Photonics with hexagonal boron nitride. Nat. Rev. Mater. 4, 552–567 (2019).
Arnaud, B., Lebègue, S., Rabiller, P. & Alouani, M. Huge excitonic effects in layered hexagonal boron nitride. Phys. Rev. Lett. 96, 026402 (2006).
Cassabois, G., Valvin, P. & Gil, B. Hexagonal boron nitride is an indirect bandgap semiconductor. Nat. Photon. 10, 262–266 (2016).
Haastrup, S. et al. The Computational 2D Materials Database: high-throughput modeling and discovery of atomically thin crystals. 2D Mater. 5, 042002 (2018).
Bersch, E., Rangan, S., Bartynski, R. A., Garfunkel, E. & Vescovo, E. Band offsets of ultrathin high-κ oxide films with Si. Phys. Rev. B 78, 085114 (2008).
Robertson, J. Band offsets of wide-band-gap oxides and implications for future electronic devices. J. Vac. Sci. Technol. B 18, 1785–1791 (2000).
Robertson, J. High dielectric constant oxides. Eur. Phys. J. Appl. Phys. 28, 265–291 (2004).
Vexler, M. I., Tyaginov, S. E. & Shulekin, A. F. Determination of the hole effective mass in thin silicon dioxide film. J. Phys. Condens. Matter 17, 8057–8068 (2005).
Städele, M., Sacconi, F., Di Carlo, A. & Lugli, P. Enhancement of the effective tunnel mass in ultrathin silicon dioxide layers. J. Appl. Phys. 93, 2681–2690 (2003).
Specht, M., Städele, M., Jakschik, S. & Schröder, U. Transport mechanisms in atomic-layer-deposited Al2O3 dielectrics. Appl. Phys. Lett. 84, 3076–3078 (2004).
Aarik, J., Mändar, H., Kirm, M. & Pung, L. Optical characterization of HfO2 thin films grown by atomic layer deposition. Thin Solid Films 466, 41–47 (2004).
Campera, A., Iannaccone, G. & Crupi, F. Modeling of tunnelling currents in Hf-based gate stacks. IEEE Trans. Electron Devices 54, 83–89 (2007).
Lin, Y. S., Puthenkovilakam, R. & Chang, J. P. Dielectric property and thermal stability of HfO2 on silicon. Appl. Phys. Lett. 81, 2041–2043 (2002).
Hou, Y. T., Li, M. F., Yu, H. Y. & Kwong, D. L. Modeling of tunneling currents through HfO2 and (HfO2)x(Al2O3)1−x gate stacks. IEEE Electron Device Lett. 24, 96–98 (2003).
Kim, S. S. et al. Tunable bandgap narrowing induced by controlled molecular thickness in 2D mica nanosheets. Chem. Mater. 27, 4222–4228 (2015).
Park, S. et al. Characterization of luminescence properties of exfoliated mica via sonication technique. Chem. Phys. 522, 238–241 (2019).
Wang, L. & Sasaki, T. Titanium oxide nanosheets: graphene analogues with versatile functionalities. Chem. Rev. 114, 9455–9486 (2014).
Osada, M. & Sasaki, T. Two-dimensional dielectric nanosheets: novel nanoelectronics from nanocrystal building blocks. Adv. Mater. 24, 210–228 (2012).
Rizzo, A., De Blasi, C., Galassini, S., Micocci, G. & Ruggiero, L. Electrical properties of n-type GaS single crystals. Solid State Commun. 40, 641–644 (1981).
Guo, Y. & Robertson, J. Origin of the high work function and high conductivity of MoO3. Appl. Phys. Lett. 105, 222110 (2014).
Cho, B. O., Wang, J., Sha, L. & Chang, J. P. Tuning the electrical properties of zirconium oxide thin films. Appl. Phys. Lett. 80, 1052–1054 (2002).
Rubloff, G. W. Far-ultraviolet reflectance spectra and the electronics structure of ionic crystals. Phys. Rev. B 5, 178–188 (1972).
Vexler, M. I. et al. Electrical characterization and modeling of the Au/CaF2/nSi (111) structures with high-quality tunnel-thin fluoride layer. J. Appl. Phys. 105, 083716 (2009).
Wang, J. et al. High-temperature relaxations in CaF2 single crystals. Mater. Sci. Eng. B 188, 31–34 (2014).
Gurram, M. et al. Spin transport in fully hexagonal boron nitride encapsulated graphene. Phys. Rev. B 93, 115441 (2016).
Mayorov, A. S. et al. Micrometer-scale ballistic transport in encapsulated graphene at room temperature. Nano Lett. 11, 2396–2399 (2011).
Dean, C. R. et al. Boron nitride substrates for high-quality graphene electronics. Nat. Nanotechnol. 5, 722–726 (2010).
Kretinin, A. V. et al. Electronic properties of graphene encapsulated with different two-dimensional atomic crystals. Nano Lett. 14, 3270–3276 (2014).
Wang, J. I. et al. Electronic transport of encapsulated graphene and WSe2 devices fabricated by pick-up of prepatterned hBN. Nano Lett. 15, 1898–1903 (2015).
Doganov, R. A. et al. Transport properties of ultrathin black phosphorus on hexagonal boron nitride. Appl. Phys. Lett. 106, 083505 (2015).
Gillgren, N. et al. Gate tunable quantum oscillations in air-stable and high mobility few-layer phosphorene heterostructures. 2D Mater. 2, 011001 (2015).
Long, G. et al. Achieving ultrahigh carrier mobility in two-dimensional hole gas of black phosphorus. Nano Lett. 16, 7768–7773 (2016).
Movva, H. C. et al. High-mobility holes in dual-gated WSe2 field-effect transistors. ACS Nano 9, 10402–10410 (2015).
Chen, X. et al. High-quality sandwiched black phosphorus heterostructure and its quantum oscillations. Nat. Commun. 6, 7315 (2015).
Cao, Y. et al. Quality heterostructures from two-dimensional crystals unstable in air by their assembly in inert atmosphere. Nano Lett. 15, 4914–4921 (2015).
Liu, W., Sarkar, D., Kang, J., Cao, W. & Banerjee, K. Impact of contact on the operation and performance of back-gated monolayer MoS2 field-effect-transistors. ACS Nano 9, 7904–7912 (2015).
Zhang, Y., Ye, J., Matsuhashi, Y. & Iwasa, Y. Ambipolar MoS2 thin flake transistors. Nano Lett. 12, 1136–1140 (2012).
Kaushik, N. et al. Reversible hysteresis inversion in MoS2 field effect transistors. npj 2D Mater. Appl. 1, 34 (2017).
Chen, J. H., Jang, C., Xiao, S., Ishigami, M. & Fuhrer, M. S. Intrinsic and extrinsic performance limits of graphene devices on SiO2. Nat. Nanotechnol. 3, 206–209 (2008).
Karnatak, P. et al. Current crowding mediated large contact noise in graphene field-effect transistors. Nat. Commun. 7, 13703 (2016).
Farmer, D. B. et al. Utilization of a buffered dielectric to achieve high field-effect carrier mobility in graphene transistors. Nano Lett. 9, 4474–4478 (2009).
Radisavljevic, B. & Kis, A. Mobility engineering and a metal–insulator transition in monolayer MoS2. Nat. Mater. 12, 815–820 (2013).
Neal, A. T., Liu, H., Gu, J. & Ye, P. D. Magneto-transport in MoS2: phase coherence, spin–orbit scattering, and the Hall factor. ACS Nano 7, 7077–7082 (2013).
Ponomarenko, L. A. et al. Effect of a high-κ environment on charge carrier mobility in graphene. Phys. Rev. Lett. 102, 100–103 (2009).
Koenig, S. P., Doganov, R. A., Schmidt, H., Castro Neto, A. H. & Özyilmaz, B. Electric field effect in ultrathin black phosphorus. Appl. Phys. Lett. 104, 103106 (2014).
Perello, D. J., Chae, S. H., Song, S. & Lee, Y. H. High-performance n-type black phosphorus transistors with type control via thickness and contact-metal engineering. Nat. Commun. 6, 7809 (2015).
Allain, A. & Kis, A. Electron and hole mobilities in single-layer WSe2. ACS Nano 8, 7180–7185 (2014).
Pradhan, N. R. et al. Hall and field-effect mobilities in few layered p-WSe2 field-effect transistors. Sci. Rep. 5, 8979 (2015).
Acknowledgements
T.K., Y.Y.I. and T.G. acknowledge the financial support through FWF grant numbers I2606-N30, I4123-N30 and P29119-N35. Y.Y.I. and M.I.V. acknowledge financial support by the Ministry of Science and Higher Education of the Russian Federation under project number 075-15-2020-790. F.D. and M. Luisier thank CSCS for giving them access to the Piz Daint supercomputer under project number s876. C.S. and M.W. gratefully acknowledge financial support by the Austrian Federal Ministry for Digital and Economic Affairs and the National Foundation for Research, Technology and Development and the Christian Doppler Research Association. The computational results presented have been achieved in part using the Vienna Scientific Cluster (VSC). S.W. and T.M. acknowledge financial support through the Graphene Flagship number 785219 and number 881603. K.W. and T.T. acknowledge support from the Elemental Strategy Initiative conducted by the MEXT, Japan, number JPMXP0112101001, JSPS KAKENHI grant number JP20H00354 and the CREST(JPMJCR15F3), JST. M. Lanza acknowledges support from the Ministry of Science and Technology of China (grant numbers 2018YFE0100800, 2019YFE0124200) and the National Natural Science Foundation of China (grant number 61874075).
Author information
Authors and Affiliations
Contributions
T.K. and T.G. conceived the ideas and led the study; T.K., Y.Y.I. and T.G. prepared the manuscript draft; F.D. and M. Luisier prepared and performed the DFT+NEGF calculations in frequent discussions with T.K. and T.G.; C.S. implemented the Tsu–Esaki model within the Comphy framework under the guidance of M.W.; T.K. and M.I.V. performed the Tsu–Esaki calculations in frequent discussions with Y.Y.I. and T.G.; S.W. fabricated devices under the supervision of T.M. using crystals provided by K.W. and T.T.; M. Lanza fabricated devices and provided advice for data analysis; T.K. performed the electrical characterization of the devices; and all authors reviewed and revised the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Peer review information Nature Electronics thanks Tania Roy, Hailin Peng and Ruge Quhe for their contribution to the peer review of this work.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Information
Details about device fabrication, electrical characterization, and the simulation methodology for using the Tsu–Esaki model and the DFT+NEGF model, and discussion of the impact of the metal work function on the simulation results. Supplementary Figs. 1 and 2.
Rights and permissions
About this article
Cite this article
Knobloch, T., Illarionov, Y.Y., Ducry, F. et al. The performance limits of hexagonal boron nitride as an insulator for scaled CMOS devices based on two-dimensional materials. Nat Electron 4, 98–108 (2021). https://doi.org/10.1038/s41928-020-00529-x
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41928-020-00529-x
This article is cited by
-
Van der Waals enabled formation and integration of ultrathin high-κ dielectrics on 2D semiconductors
npj 2D Materials and Applications (2024)
-
Low Ohmic contact resistance and high on/off ratio in transition metal dichalcogenides field-effect transistors via residue-free transfer
Nature Nanotechnology (2024)
-
Ultrashort vertical-channel MoS2 transistor using a self-aligned contact
Nature Communications (2024)
-
The Roadmap of 2D Materials and Devices Toward Chips
Nano-Micro Letters (2024)
-
Scalable integration of hybrid high-κ dielectric materials on two-dimensional semiconductors
Nature Materials (2023)