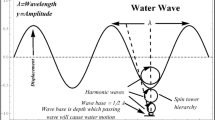
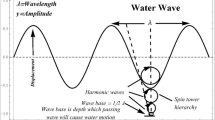
Abstract
This paper aims to analyze the coupled nonlinear fractional Drinfel’d-Sokolov-Wilson (FDSW) model with beta derivative. The nonlinear FDSW equation plays an important role in describing dispersive water wave structures in mathematical physics and engineering, which is used to describe nonlinear surface gravity waves propagating over horizontal sea bed. We have applied the travelling wave transformation that converts the FDSW model to nonlinear ordinary differential equations. After that, we applied the generalized rational exponential function method (GERFM). Diverse types of soliton solution structures in the form of singular bright, periodic, dark, bell-shaped and trigonometric functions are attained via the proposed method. By selecting a suitable parametric value, the 3D, 2D and contour plots for some solutions are also displayed to visualize their nature in a better way. The modulation instability for the model is also discussed. The results show that the presented method is simple and powerful to get a novel soliton solution for nonlinear PDEs.
Similar content being viewed by others
Introduction
A solitary wave is a special type of wave that maintains its shape as it propagates through a medium, without changing its speed or amplitude. Solitary waves can arise in various fields, including water waves, metamaterials, engineering, plasma waves, and optical fibers1,2,3,4,5,6,7,8,9,10,11,12. In recent years, there has been increasing interest in the study of solitary waves in nonlinear fractional differential equations (NFDEs), which are differential equations involving fractional derivatives. NFDEs are generalizations of classical differential equations, in which the order of the derivative is not necessarily an integer. Solitary wave solutions of NFDEs have important applications in various fields, including physics, mathematics, engineering, and biology13,14,15,16,17,18,19,20. The study of solitary waves in NFDEs is a challenging task, due to the nonlinearity and fractional nature of these equations.
In recent few decades, many efficient methods or techniques have been used to find the analytical solutions for nonlinear models, such as the Ricatti approach21, the Kudryashov method22, the Darboux transformation23, the Jacobi elliptic function approach24, the sine-cosine approach25, the direct algebraic technique26, the extended tanh function method27,28,29,30,31, sine-Gordon approach32,33, Fokas technique34, the Hirota bilinear transformation approach35,36, the first integral approach37, the trial solution technique38, the \(\left( \frac{G^{'}}{G}\right)\)-expansion approach39, \(\left( \frac{G^{'}}{G^2}\right)\)-expansion technique40,\(\left( \frac{G^{'}}{G},\frac{1}{G}\right)\)-expansion technique41,42,43, Lie Symmetry method44, the unified method45, and so on. The travelling wave solution of DSW was attained by utilizing the auxiliary equation method46. By utilizing the modified extended direct algebraic method bell, anti-bell, periodic and dark solitary wave solution of DSW has been attained in47. The series solution of the DSW model was attained by using the Adomian decomposition method48.
The coupled (1+1)-dimensional DSW model49 which read as,
We can write the above system in the form of fractional derivative with respect to time is given by,
Here, \(a, \gamma _1, \lambda _1\) and \(\eta _1\) are the constant and the \(\alpha\) represents the order of fractional derivative with \(0<\alpha \le 1\). When \(\alpha =1\) Eq. (2) is converted to classical DSW equation, which was first introduced by Drinfel’d and Sokolov50,51 and studied by Wilson52. In this article, we will construct an exact solution for the Drinfel’d-Sokolov-Wilson model using the generalized rational exponential function method approach with the help of well-known Beta derivative. The solutions are attained in the form of singular bright, dark, periodic, bell and lump-type water wave structures. The achieved solutions might be useful to comprehend nonlinear phenomena. It is worth noting that the implemented method for solving NPDEs is efficient, and simple to find further and new-fangled solutions in the area of mathematical physics and coastal engineering. Diverse types of fractional derivatives have been used in the past, such as Caputo fractional53, Beta derivative54, Conformable fractional55, Reimann-Liouville56 and truncated M-fractional derivative57 etc. have importance in fractional calculus.
The remaining article is distributed into various sections. Section (2) contain definition from fractional calculus relevant to our study. In Sect. (3) we have discussed the main step of the method. In Sect. (4) solitary wave solutions have been described. Numerical simulations of some attained solutions are given in (5). In Sects. (6) and (7) modulus instability, a conclusion is presented.
Beta derivative
Definition
Let \(\Pi (t)\) be a function defined for all non-negative t. The function \(\Pi (t)\)58 is,
Theorem
Let \(\Pi\) and g be any two function, \(\Pi \ne 0\), and \(\alpha \in (0,1]\) then
1: \(D_t^\alpha \{b_1\Pi (t)+b_2\Upsilon (t)\}=b_1D_t^\alpha \Pi (t)+b_2D_t^\alpha \Upsilon (t)\),
where \(b_1,b_2\in \Re\)
2: \(D_t^\alpha \{\Pi (t).\Upsilon (t)\}=\Pi (t)D_t^\alpha \{\Upsilon (t)\}+\Upsilon (t)D_t^\alpha \{\Pi (t)\}\),
3: For c any constant, the following relation can be easily satisfied \(D_t^\alpha c=0,\)
4: \(D_t^\alpha (\frac{\Pi (t)}{\Upsilon (t)})=\frac{\Upsilon (t) D_t^\alpha \{\Pi (t)\}-\Pi (t) D_t^\alpha \{\Upsilon (t)\}}{\Upsilon (t)^2}\),
5: \(D_t^\alpha \{\Pi (t)\}=(t+\frac{1}{\Gamma (\alpha )})^{1-\alpha }\frac{d\Pi (t)}{dt}\),
Methodology
The GERF method is a quite novel technique for nonlinear partial differential equations (NLPDE)49. The main steps are given as:
Step:1
Consider the NLPDE as,
Suppose the travelling wave transformation,
Substituting (5) into (4) then we get ODE given as,
Step:2
Solution of equation of (7) is,
Here, \(a_0, a_{n}\), and \(b_n\) are unknown parameters to be found. The function \(\phi (\varpi )\) is defined as
Step:3
We apply the homogeneous balance technique on (7) to attain the value of N.
Step:4 Substituting (7) with equation (8) into (6), then we attain the system of algebraic equations. The system is solved by utilizing Mathematica software, and then the achieved solution of (8) is put into (7) by using (5). Finally, the solution of (4) is attained.
Solitary wave structure
We consider the travelling wave transformation for FDSW (2) as follows,
Using (9) to (2) and then we get ,
From (10), we have
Putting the value of \(\Phi\) into (11) and integrating one time then we get,
Now we have to apply the balancing technique on (13) then we get \(N=1\). Utilizing \(N=1\) in (7) then we get,
where \(a_0, a_1\), and \(b_1\) are unknown constants to be find. The solution of (2) is discussed as,
Case-1 If \(\left[ \sigma _1, \sigma _2, \sigma _3 ,\sigma _4 \right]\)=\([1, -1, 1, 1]\) and \([\mu _1, \mu _2, \mu _3, \mu _4]\)=\([1, -1, 1, -1]\) then (8) become,
When equations (14) and (15) are putting into equation (13), we arrive at a system of algebraic linear equations. By solving these equations simultaneously, we obtain the following set of solitary wave solutions. set-1
Putting (16) into (14) then solution of (2) is,
Set-2
Substituting (19) into (14) then solution of (2) is,
Set-3
Putting (22) into (14) then solution of (2) is,
Set-4
Substituting (25) into (14) then solution of (2) is,
Case-2 If \(\left[ \sigma _1, \sigma _2, \sigma _3 ,\sigma _4 \right] =[\imath , -\imath , 1, 1]\) and \([\mu _1, \mu _2, \mu _3, \mu _4]=[\imath , -\imath , \imath , -\imath ]\) then (8) become,
When equations (28) and (15) are putting into equation (13), we arrive at a system of algebraic linear equations. By solving these equations simultaneously, we obtain the following set of solitary wave solutions.
Set-1
Putting (29) into (14) then solution of (2) is,
Set-2
Substituting (32) into (14) then solution of (2) is,
Set-3
Putting Eq. (35) into (14) then solution of (2) is,
Set-4
Substituting (38) into (14) then solution of (2) is,
Case-3 If \(\left[ \sigma _1, \sigma _2, \sigma _3 ,\sigma _4 \right] =[1+\imath , 1-\imath , 1, 1]\) and \([\mu _1, \mu _2, \mu _3, \mu _4]=[\imath , -\imath , \imath , -\imath ]\) then (8) become,
When equations (41) and (15) are putting into equation (13), we arrive at a system of algebraic linear equations. By solving these equations simultaneously, we obtain the following set of solitary wave solutions.
Set-1
Putting (42) into (14) then solution of (2) is,
Case-4 If \(\left[ \sigma _1, \sigma _2, \sigma _3 ,\sigma _4 \right] =[2+\imath , 2-\imath , 1, 1]\) and \([\mu _1, \mu _2, \mu _3, \mu _4]=[\imath , -\imath , \imath , -\imath ]\) then (8) become,
When equations (45) and (15) are putting into equation (13), we arrive at a system of algebraic linear equations. By solving these equations simultaneously, we obtain the following set of solitary wave solutions.
Set-1
Substituting (46) into (14) then solution of (2) is,
Case-5 If \(\left[ \sigma _1, \sigma _2, \sigma _3 ,\sigma _4 \right] =[2, 1, 1, 1]\) and \([\mu _1, \mu _2, \mu _3, \mu _4]=[1, 0, 1, 0]\) then (8) become,
When equations (49) and (15) are putting into equation (13), we arrive at a system of algebraic linear equations. By solving these equations simultaneously, we obtain the following set of solitary wave solutions.
Set-1
Putting (50) into (14) then solution of (2) is,
Set-2
Putting (53) into (14) then solution of (2) is,
Case-6 If \(\left[ \sigma _1, \sigma _2, \sigma _3 ,\sigma _4 \right] =[2, 0, 1, 1]\) and \([\mu _1, \mu _2, \mu _3, \mu _4]=[-1, 0, 1, -1]\) then (8) become,
When equations (56) and (15) are putting into equation (13), we arrive at a system of algebraic linear equations. By solving these equations simultaneously, we obtain the following set of solitary wave solutions.
Set-1
Putting (57) into (14) then solution of (2) is,
Case-7 If \(\left[ \sigma _1, \sigma _2, \sigma _3 ,\sigma _4 \right] =[-3, -1, -1, 1]\) and \([\mu _1, \mu _2, \mu _3, \mu _4]=[-1, 1, -1, 1]\) then (8) become,
When equations (60) and (15) are putting into equation (13), we arrive at a system of algebraic linear equations. By solving these equations simultaneously, we obtain the following set of solitary wave solutions.
Set-1
Putting (61) into (14) then solution of (2) is,
Set-2
Putting (64) into (14) then solution of (2) is,
Case-8 If \(\left[ \sigma _1, \sigma _2, \sigma _3 ,\sigma _4 \right] =[1, 0, 1, 1]\) and \([\mu _1, \mu _2, \mu _3, \mu _4]=[0, 0, 1, 0]\) then (8) become,
When equations (67) and (15) are putting into equation (13), we arrive at a system of algebraic linear equations. By solving these equations simultaneously, we obtain the following set of solitary wave solutions.
Set-1
Putting (68) into (14) then solution of (2) is,
Set-2
Substituting (71) into (14) then solution of (2) is,
Numerical simulation and discussion
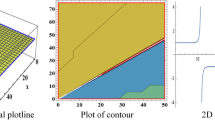
In this section, we have drawn the graph of some attained solutions for the structure solution of solitary waves. The value fractional parameter \(\alpha =1\) is fixed in all 2D graphs. Figs. (1 and 2) shows the singular bright soliton wave structure. Figures 3,4,6, 5, 7 and 8 shows the dark, periodic, bell and lump type soliton wave structure. In59 authors have attained the bright soliton solutions of the FDSW model by using the homotopy analysis transform method. Similarly in60 authors have achieved bright type soliton solution with the help of the Laplace Adomian decomposition method. Periodic-type soliton solutions have been attained by using the sine-cosine method61. But in this study, we get more generalized soliton solutions such as bright, dark, periodic, bell and lump.
Graphical solution of (20) with parameters \(\kappa _1 =-0.1, \varpi _1=-0.5, a_1=0.01\).
Graphical solution of (21) with parameters \(\kappa _1=0.2, \varpi _1=-0.8, a_1=0.1, a=0.5\).
Graphical solution of (23) with parameters \(\kappa _1=1, \varpi _1=0.5, a_1=2\).
Graphical solution of (24) with parameters \(\kappa _1 =1, \varpi _1 =0.1, a_1=1, a=2\).
Graphical solution of (47) with parameters \(\kappa _1=1, \varpi _1=0.01, a_1=1\).
Graphical solution of (52) with parameters \(\kappa _1=2, \varpi _1=0.01, b_1=2, a=0.8\).
Graphical solution of (69) with parameters \(\kappa _1=-0.8, \gamma _1=0.01, \lambda _1=0.02, a=-5, a_0=0.3, a_1=-5\).
Graphical solution of (70) with parameters \(\kappa _1=-0.8, \gamma _1=0.1, \lambda _1=0.2, a=-5, a_0=-2\).
Modulus instability
We have found the modulation instability of the coupled nonlinear DSW model (1) through linear stability. We consider the steady-state solution,
Substituting (74) into (1) then after linearize we get,
It is supposed that the solution of (75) has as,
where \(\kappa\) and \(\omega\) are the wave number and frequency of perturbation. Putting (76) into (75), the dispersion relation (DR) is acquired as
from (77), one can see that the real component is negative for all values of \(\kappa\) then any superposition of the results will appear to decay. So, the dispersion is stable.
Conclusion
In this work, we have successfully achieved some fresh and further general traveling wave solutions to the nonlinear fractional Drinfel’d-Sokolov-Wilson (FDSW) model with beta derivative. The solutions attained by using the GERF method for the proposed model are competent to examine the scientific model of gravity water waves in shallow water. It is capable of investigating plasma waves in the seaside oceans and breaking down the unidirectional spread of long waves in oceans and harbors. The proposed method is not only more powerful than previous approaches but has also introduced novel solutions that have not been reported before.
Data availability
All data that support the findings of this study are included in the article.
References
Shakeel, M., Bibi, A., Chou, D. & Zafar, A. Study of optical solitons for Kudryashov’s Quintuple power-law with dual form of nonlinearity using two modified techniques. Optik 273, 170364 (2023).
Irshad, S., Shakeel, M., Bibi, A., Sajjad, M. & Nisar, K. S. A comparative study of nonlinear fractional Schrödinger equation in optics. Mod. Phys. Lett. B 37(05), 2250219 (2023).
Irshad, S., Shakeel, M., Ali, A., Rezazadeh, H. & Bekir, A. Analytical study of complex Ginzburg-Landau equation arising in nonlinear optics. J. Nonlinear Opt. Phys. Mater. 32(01), 2350010 (2023).
Junaid-U-Rehman, M., Almusawa, H., Awrejcewicz, J., Kudra, G., Abbas, N., & Rasool, A. Propagation of electrostatic potential with dynamical behaviours and conservation laws of the (3+ 1)-dimensional nonlinear extended quantum Zakharov-Kuznetsov equation. Int. J. Geometric Methods Mod. Phys. (2023)
Almusawa, H. & Jhangeer, A. A study of the soliton solutions with an intrinsic fractional discrete nonlinear electrical transmission line. Fractal Fract. 6(6), 334 (2022).
Hussain, A., Jhangeer, A., Abbas, N., Khan, I. & Sherif, E. S. M. Optical solitons of fractional complex Ginzburg-Landau equation with conformable, beta, and M-truncated derivatives: A comparative study. Adv. Difference Equ. 2020, 1–19 (2020).
Zafar, A., Shakeel, M., Ali, A., Akinyemi, L. & Rezazadeh, H. Optical solitons of nonlinear complex Ginzburg-Landau equation via two modified expansion schemes. Opt. Quant. Electron. 54, 1–15 (2022).
Yao, S. W. et al. Novel solutions to the coupled KdV equations and the coupled system of variant Boussinesq equations. Results Phys. 45, 106249 (2023).
Shakeel, M., Bibi, A., Zafar, A. & Sohail, M. Solitary wave solutions of Camassa-Holm and Degasperis-Procesi equations with Atangana’s conformable derivative. Comput. Appl. Math. 42(2), 101 (2023).
Hussain, A., Jhangeer, A. & Abbas, N. Symmetries, conservation laws and dust acoustic solitons of two-temperature ion in inhomogeneous plasma. Int. J. Geometric Methods Mod. Physi. 18(05), 2150071 (2021).
Feng, Ll., & Zhu, Zn. Darboux Transformation and Soliton Solutions for a Nonlocal Two-Component Complex Modified Korteweg-de Vries Equation. J. Nonlinear Math. Phys. (2023).
Junaid-U-Rehman, M., Kudra, G. & Awrejcewicz, J. Conservation laws, solitary wave solutions, and lie analysis for the nonlinear chains of atoms. Sci. Rep. 13(1), 11537 (2023).
Rasool, T., Hussain, R., Rezazadeh, H. & Gholami, D. The plethora of exact and explicit soliton solutions of the hyperbolic local (4+ 1)-dimensional BLMP model via GERF method. Results Phys. 46, 106298 (2023).
Li, B. Q. & Ma, Y. L. Optical soliton resonances and soliton molecules for the Lakshmanan-Porsezian-Daniel system in nonlinear optics. Nonlinear Dyn. 111(7), 6689–6699 (2023).
Li, Z. & Huang, C. Bifurcation, phase portrait, chaotic pattern and optical soliton solutions of the conformable Fokas-Lenells model in optical fibers. Chaos, Solitons & Fractals 169, 113237 (2023).
Zhang, R. F., Li, M. C., Cherraf, A. & Vadyala, S. R. The interference wave and the bright and dark soliton for two integro-differential equation by using BNNM. Nonlinear Dyn. 111(9), 8637–8646 (2023).
Wang, K. J. Diverse soliton solutions to the Fokas system via the Cole-Hopf transformation. Optik 272, 170250 (2023).
Rasool, T., Hussain, R., Al Sharif, M. A., Mahmoud, W. & Osman, M. S. A variety of optical soliton solutions for the M-truncated Paraxial wave equation using Sardar-subequation technique. Opt. Quant. Electron. 55(5), 396 (2023).
Shakeel, M. et al. Dynamical study of a time fractional nonlinear Schrödinger model in optical fibers. Opt. Quant. Electron. 55, 1010 (2023).
Wang, K. J., Si, J. & Liu, J. H. Diverse optical soliton solutions to the Kundu-Mukherjee-Naskar equation via two novel techniques. Optik 273, 170403 (2023).
Cenesiz, Y., Tasbozan, O. & Kurt, A. Functional variable method for conformable fractional modified kdv-zkequation and Maccari system. Tbilisi Math J. 10, 117–125 (2017).
Zafar, A., Shakeel, M., Ali, A., Akinyemi, L. & Rezazadeh, H. Optical solitons of nonlinear complex Ginzburg-Landau equation via two modified expansion schemes. Opt. Quant. Electron. 54, 1–15 (2022).
Saha, D., Chatterjee, P. & Raut, S. Multi-shock and soliton solutions of the Burgers equation employing Darboux transformation with the help of the Lax pair. Pramana 97(2), 54 (2023).
Pankaj, R. D. Extended jacobi elliptic function technique: a tool for solving nonlinear wave equations with emblematic software. J. Comput. Anal. Appl. 31(1). (2023)
Fendzi-Donfack, E. et al. Exotical solitons for an intrinsic fractional circuit using the sine-cosine method. Chaos, Solitons & Fractals 160, 112253 (2022).
Ghayad, M. S., Badra, N. M., Ahmed, H. M. & Rabie, W. B. Derivation of optical solitons and other solutions for nonlinear Schrödinger equation using modified extended direct algebraic method. Alex. Eng. J. 64, 801–811 (2023).
El-shamy, O., El-barkoki, R., Ahmed, H. M., Abbas, W. & Samir, I. Exploration of new solitons in optical medium with higher-order dispersive and nonlinear effects via improved modified extended tanh function method. Alex. Eng. J. 68, 611–618 (2023).
Arefin, M. A. et al. Explicit Soliton Solutions to the Fractional Order Nonlinear Models through the Atangana Beta Derivative. Int. J. Theor. Phys. 62, 134 (2023).
Zaman, M. & U. H., Arefin, M. A., Akbar, M. A., & Uddin, M. H.,. Study of the soliton propagation of the fractional nonlinear type evolution equation through a novel technique. PLOS ONE18(5), e0285178 (2023).
Sadiya, U., Inc, M., Arefin, M. A. & Uddin, M. H. Consistent travelling waves solutions to the non-linear time fractional Klein-Gordon and Sine-Gordon equations through extended tanh-function approach. J. Taibah Univ. Sci. 16(1), 594–607 (2022).
Zaman, U., Arefin, M. A., Akbar, M. A. & Uddin, M. H. Stable and effective traveling wave solutions to the non-linear fractional Gardner and Zakharov-Kuznetsov-Benjamin-Bona-Mahony equations. Part. Differ. Equ. Appl. Math. 7, 100509 (2023).
Khatun, M. A., Arefin, M. A., Akbar, M. A., & Uddin, M. H. Numerous explicit soliton solutions to the fractional simplified Camassa-Holm equation through two reliable techniques. Ain Shams Eng. J. 102214. (2023)
Khatun, M. A., Arefin, M. A., Islam, M. Z., Akbar, M. A. & Uddin, M. H. New dynamical soliton propagation of fractional type couple modified equal-width and Boussinesq equations. Alex. Eng. J. 61(12), 9949–9963 (2022).
Caudrelier, V., Crampé, N., Ragoucy, E., & Zhang, C. Nonlinear Schrödinger equation on the half-line without a conserved number of solitons. Physica D: Nonlinear Phenomena, 133650. (2023)
Yin, T., Xing, Z., & Pang, J. Modified Hirota bilinear method to (3+ 1)-D variable coefficients generalized shallow water wave equation. Nonlinear Dyn. 1-12. (2023)
Tang, W. Soliton dynamics to the Higgs equation and its multi-component generalization. Wave Motion 120, 103144 (2023).
Canzian, E. P., Santiago, F., Lopes, A. B., Barbosa, M. R. & Barañano, A. G. On the application of the double integral method with quadratic temperature profile for spherical solidification of lead and tin metals. Appl. Therm. Eng. 219, 119528 (2023).
Biswas, A. et al. Optical solitons in nano-fibers with spatio-temporal dispersion by trial solution method. Optik 127(18), 7250–7257 (2016).
Zafar, A., Shakeel, M., Ali, A., Rezazadeh, H. & Bekir, A. Analytical study of complex Ginzburg-Landau equation arising in nonlinear optics. J. Nonlinear Opt. Phys. Mater. 32(01), 2350010 (2023).
Zafar, A., Inc, M., Shakeel, M. & Mohsin, M. Analytical study of nonlinear water wave equations for their fractional solution structures. Mod. Phys. Lett. B 36(14), 2250071 (2022).
Arefin, M. A., Khatun, M. A., Uddin, M. H. & Inc, M. Investigation of adequate closed form travelling wave solution to the space-time fractional non-linear evolution equations. J. Ocean Eng. Sci. 7(3), 292–303 (2022).
Uddin, M. H., Khan, M. A., Akbar, M. A. & Haque, M. A. Analytical wave solutions of the space time fractional modified regularized long wave equation involving the conformable fractional derivative. Karbala Int. J. Mod. Sci. 5(1), 7 (2019).
Uddin, M. H., Akbar, M. A., Khan, M. A. & Haque, M. A. Families of exact traveling wave solutions to the space time fractional modified KdV equation and the fractional Kolmogorov-Petrovskii-Piskunovequation. J. Mech. Continua Math. Sci. 13(1), 17–33 (2018).
Yan, X., Liu, J., Yang, J. & Xin, X. Lie symmetry analysis, optimal system and exact solutions for variable-coefficients (2+ 1)-dimensional dissipative long-wave system. J. Math. Anal. Appl. 518(1), 126671 (2023).
Raza, N. & Rafiq, M. H. Abundant fractional solitons to the coupled nonlinear Schrödinger equations arising in shallow water waves. Int. J. Mod. Phys. B 34(18), 2050162 (2020).
Tariq, K. U. & Seadawy, A. R. On the soliton solutions to the modified Benjamin-Bona-Mahony and coupled Drinfel’d-Sokolov-Wilson models and its applications. J. King Saud Univ. - Sci. 32(1), 156–162 (2020).
Arshad, M., Seadawy, A., Lu, D. & Wang, J. Travelling wave solutions of Drinfel’d-Sokolov-Wilson, Whitham-Broer-Kaup and (2+1)-dimensional Broer-Kaup-Kupershmit equations and their applications. Chin. J. Phys. 55(3), 780–797 (2017).
Saifullah, S., Ali, A., Shah, K. & Promsakon, C. Investigation of Fractal Fractional nonlinear Drinfeld-Sokolov-Wilson system with Non-singular Operators. Results Phys. 33, 105145 (2022).
Kumar Raj, & Ravi Shankar Verma. Dynamics of some new solutions to the coupled DSW equations traveling horizontally on the seabed. J. Ocean Eng. Sci. (2022)
Drinfel’d, V. G. & Sokolov, V. V. Equations of Korteweg-de Vries type and simple Lie algebras. Sov. Math. Dokl. 23, 457–462 (1981).
Drinfel’d, V. G. & Sokolov, V. V. Lie algebras and equations of Korteweg-de Vries type. J. Sov. Math. 30(2), 1975–2036 (1985).
Wilson, G. The affine Lie algebra C (1) 2 and an equation of Hirota and Satsuma. Phys. Lett. A 89(7), 332–334 (1982).
Ghayad, M. S., Badra, N. M., Ahmed, H. M. & Rabie, W. B. Derivation of optical solitons and other solutions for nonlinear Schrödinger equation using modified extended direct algebraic method. Alex. Eng. J. 64, 801–811 (2023).
Atangana, A., Baleanu, D. & Alsaedi, A. Analysis of time-fractional Hunter-Saxton equation: a model of neumatic liquid crystal. Open Phys. 14(1), 145–149 (2016).
Khalil, R., Al Horani, M., Yousef, A. & Sababheh, M. A new definition of fractional derivative. J. Comput. Appl. Math. 264, 65–70 (2014).
Kilbas, A. A., Srivastava, H. M., & Trujillo, J. J. Theory and applications of fractional differential equations (Vol. 204). Elsevier. (2006)
Sousa, J. V. D. C., & de Oliveira, E. C. A new truncated \(M\)-fractional derivative type unifying some fractional derivative types with classical properties. arXiv preprint arXiv:1704.08187. (2017)
Zafar, A. et al. Optical solitons of nonlinear complex Ginzburg-Landau equation via two modified expansion schemes. Opt. Quant. Electron. 54, 5 (2022).
Gao, W., Veeresha, P., Prakasha, D., Baskonus, H. M. & Yel, G. A powerful approach for fractional Drinfeld-Sokolov-Wilson equation with Mittag-Leffler law. Alex. Eng. J. 58(4), 1301–1311 (2019).
Saifullah, S., Ali, A., Shah, K. & Promsakon, C. Investigation of fractal fractional nonlinear Drinfeld-Sokolov-Wilson system with non-singular operators. Results Phys. 33, 105145 (2022).
Yao, S. et al. Analytical solutions of conformable Drinfel’d-Sokolov-Wilson and Boiti Leon Pempinelli equations via sine-cosine method. Results Phys. 42, 105990 (2022).
Acknowledgements
The authors extend their appreciation to the Deputyship for Research & Innovation, Ministry of Education in Saudi Arabia for funding this research (IFKSURC-1-7119).
Author information
Authors and Affiliations
Contributions
All Authors are contributed equally.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher's note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Shakeel, M., AlQahtani, S.A., Rehman, M.J.U. et al. Construction of diverse water wave structures for coupled nonlinear fractional Drinfel’d-Sokolov-Wilson model with Beta derivative and its modulus instability. Sci Rep 13, 17528 (2023). https://doi.org/10.1038/s41598-023-44428-5
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41598-023-44428-5
This article is cited by
-
Solution of convection-diffusion model in groundwater pollution
Scientific Reports (2024)
-
Noval soliton solution, sensitivity and stability analysis to the fractional gKdV-ZK equation
Scientific Reports (2024)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.