Introduction

Solar thermal system is one of the few scalable technologies capable of delivering dispatch-able renewable power and, as such, many expect it to shoulder a significant share of system balancing in a renewable electricity future power by cheap, intermittent PV and wind power. To efficiently convert the solar radiation into useful heat energy, various technologies are being investigated. Among these, nanofluids are considered as promising heat transfer medium. In recent years, nanofluids have received growing interests by researchers from various fields because of their enhanced thermo-physical properties such as convective heat transfer, viscosity, thermal conductivity and thermal diffusivity. Thanks to these enhanced properties, nanofluids can be used in a wide range of engineering applications, particularly to enhance the thermal performance of solar systems.

This study offers important insights into the 2D unsteady magnetohydrodynamic (MHD) flow with entropy production of nanoliquids subjected to chemical reaction and thermal radiative flux. MHD is the examination of dynamics of magnetic fields of electrically-conducting liquids. The induced current subject to magnetic field in an electrically conducting liquid polarizes the fluid, resulting in a change in the magnetic field. Liquids can be electrically conductive in numerous applications for material processing in both chemical and mechanical engineering and so they react to applied magnetic fields. Such systems can be employed in various industrial applications, for example to monitor the rate of heat transfer levels over a stretch sheet and to get pre-processing materials properties and tuning the thermomechanical processing of materials to industry requirements. This process is very significant subject to Lorentz force, which reduces the liquid flow in a applied field direction.

Investigations of MHD flow of nanofluids with radiative effects are reported by various studies.

Rashad et al.1 numerically inspected effects of the sink on MHD flow with entropy production of Cu-water nanoliquid in an inclined permeable enclosure. Their consequences reveals that the heat gradient decreases with swelling the volume fraction of nanoliquid and the intensity of magnetic field. Dharmaiah et al.2 deliberated the MHD viscous nanomaterial flow by a stretchable wedge subject to convective conditions, Ohmic heating and radiative heat flux. Izady et al.3 investigated CuO/water based nanomaterial flow via permeable expanding surface with MHD and radiative effects. It is revealed that double branch solutions occurs for a certain domain of the surface expanding variable. Dinarvand4 scrutinized viscous flow of CuO–Ag/water based nanoliquid over a circular cylinder with sinusoidal radius variation by considering different physical parameters. Jabbaripour et al.5 investigated the 3D MHD stagnation-point boundary layer flow of aluminium–copper/water hybrid nanomaterial over a wavy cylinder considering subjected to temperature jump boundary conditions and velocity slip. Mousavi et al.6 employs experimental relations to improve model for envisaging the presentation of water-based MHD Casson nanofluid fluid flow over an expanding surface with radiative effect. Some other important recent studies on this topic are listed in Refs.7,8,9,10,11,12,13,14,15,16.

Entropy production is a innovative prospective in numerous thermodynamic developments and displays dynamic utilizations in polymer processing and thermal optimization. The consequence of entropy production is witnessed in combustion, thermal systems, heat exchangers, turbine systems, nuclear reactions, porous media etc. Thermodynamics second law is employed to discuss the irreversibility analysis. Here a model of entropy generation rate caused by fluid friction, magnetic field effect and solutal transfer rate across a low temperature and concentration difference in the liquid flow is constructed. By minimizing and evaluating the entropy production, the effectiveness of a thermal system can be enhanced, and losses of energy can be minimized. The theoretical analysis of entropy optimization problem in thermal convective flow is investigated by Bejan17,18. Kurnia et al.19 reported the thermal transport and entropy analyses in viscous flow in helically coiled tubes. Their conclusions showed that the entropy production inside the pipe is higher for thermal transfer than compared to fluid friction. Irreversibility investigation in water-based iron oxide nanofluid with variable magnetic force inside circular tube was interpreted by Gorjaei et al.20. Khan et al.21 considered melting and irreversibility effects for MHD nanomaterials flow with slip condition. Few advancements regarding entropy problems are mentioned in Refs.22,23,24,25,26,27,28,29,30. Refs.31,32,33,34,35,36 highlights the importance of fluid flow regarding stretchable surfaces.

From above literature review, it shows there are numerous existing studies in the literature pertaining MHD flow of the nanofluids under and boundary conditions and different geometric configurations, however limited studies consider all the effects considered in the present work simultaneously. At the same time, their analysis focuses primarily on local entropy generation. Additionally, no work has been reported so far entropy generation in chemically reactive unsteady flow of Prandtl nanoliquid with Lorentz force over an infinite plate. Therefore in recent communication our prime objective is to analyze the entropy examination in reactive time-dependent flow Prandtl nanomaterials with Lorentz force over an infinite plate. Energy expression is modeled through magnetic force and thermal radiation. Significant behaviors of random and thermophoresis motion are further accounted. Physical features of entropy production are deliberated. Moreover, chemical reaction is also addressed. Nonlinear system are converted to dimensionless system by employing appropriate transformations. The achieved dimensionless problem are tackled through numerical approach (finite difference method). Significant impact of physical variables on fluid flow, entropy generation, thermal field and concentration are discussed via various plots.

Mathematical description

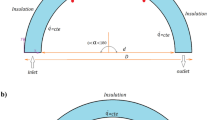
Here 2D (two-dimensional) chemically reactive unsteady flow of Prandtl nanofluid with Lorentz force over an infinite plate is discussed. Thermal radiation and magnetic force are considered in energy expression. In addition, innovative characteristics of random and thermophoresis motion are considered. Thermodynamics second law is employed to discuss entropy analysis. Furthermore, chemical reaction at the surface of plate is considered. Constant magnetic force \((B_{0} )\) is applied. Consider \(u = u_{w} = ax\) as stretching velocity with (\(a > 0\)). The schematic flow examination is displayed in Fig. 1.

Figure 1
figure 1

Flow sketch.

Full size image

The extra stress tensor of Prandtl fluid satisfies37,38,39,40:

$$\tau_{ij} = \frac{{A\sinh^{ - 1} \left( {\tfrac{\gamma }{{C_{1} }}} \right)}}{{\left( {\tfrac{\gamma }{{C_{1} }}} \right)}}A_{1}$$
(1)

where

$$\gamma = \sqrt {\frac{1}{2}tr\left( {A_{1} } \right)^{2} }$$
(2)

and

$$A_{1} = L + L^{t} ,\,$$
(3)

Here \(C_{1}\) and \(A\) signify the fluid parameters.

The flow equations are

$$\frac{\partial u}{{\partial x}} + \frac{\partial v}{{\partial y}} = 0,\,$$
(4)
$$\frac{\partial u}{{\partial t}} + u\frac{\partial u}{{\partial x}} + v\frac{\partial u}{{\partial y}} = \frac{A}{{\rho_{f} C_{1} }}\frac{{\partial^{2} u}}{{\partial y^{2} }} - \frac{A}{{2\rho_{f} C_{1}^{3} }}\left( {\frac{\partial u}{{\partial y}}} \right)^{2} \frac{{\partial^{2} u}}{{\partial y^{2} }} - \frac{{\sigma_{f} B_{0}^{2} }}{{\rho_{f} }}u,\,$$
(5)
$$\left. {\begin{array}{*{20}c} {\tfrac{\partial T}{{\partial t}} + u\tfrac{\partial T}{{\partial x}} + v\tfrac{\partial T}{{\partial y}} = \tfrac{{k_{f} }}{{\left( {\rho c_{p} } \right)_{f} }}\left( {1 + \tfrac{{16\sigma^{ * } T_{\infty }^{3} }}{{3kk^{ * } }}} \right)\,\tfrac{{\partial^{2} T}}{{\partial y^{2} }} + \tau D_{B} \tfrac{\partial T}{{\partial y}}\tfrac{\partial C}{{\partial y}}} \\ { + \tau \tfrac{{D_{T} }}{{T_{\infty } }}\left( {\tfrac{\partial T}{{\partial y}}} \right)^{2} + \tfrac{{\sigma B_{0}^{2} }}{{\left( {\rho c_{p} } \right)_{f} }}u^{2} } \\ \end{array} } \right\}\,$$
(6)
$$\frac{\partial C}{{\partial t}} + u\frac{\partial C}{{\partial x}} + v\frac{\partial C}{{\partial y}} = D_{B} \frac{{\partial^{2} C}}{{\partial y^{2} }} + \frac{{D_{T} }}{{T_{\infty } }}\frac{{\partial^{2} T}}{{\partial y^{2} }} - k_{r} \left( {C - C_{\infty } } \right)\,$$
(7)

with,

$$\left. {\begin{array}{*{20}c} {u = 0,\, \, v = 0,\,\;T = T_{\infty } ,\,\;C = C_{\infty } \;\;{\text{at }}t = 0} \\ {u = ax{, }v = 0,\,\;T = T_{w} ,\,\;C = C_{w} \;\;{\text{at }}y = 0} \\ {u = 0,\, \, v = 0,\, \, T = T_{\infty } {, }C = C_{\infty } {\text{ as }}y \to \infty } \\ \end{array} } \right\}\,$$
(8)

Let us consider

$$\left. {\begin{array}{*{20}c} {\tau = \tfrac{\nu }{{L_{1}^{2} }}t{, }\xi = \tfrac{x}{{L_{1} }}{, }\eta = \tfrac{y}{{L_{1} }}{, }U\left( {\tau ,\,\xi ,\,\eta } \right) = \tfrac{{L_{1} }}{\nu }u{, }V\left( {\tau ,\,\xi ,\,\eta } \right) = \tfrac{{L_{1} }}{\nu }v} \\ {\theta \left( {\tau ,\,\xi ,\,\eta } \right) = \tfrac{{T - T_{\infty } }}{{T_{w} - T_{\infty } }}{, }\phi \left( {\tau ,\,\xi ,\,\eta } \right) = \tfrac{{C - C_{\infty } }}{{C_{w} - C_{\infty } }}} \\ \end{array} } \right\},\,$$
(9)

We get

$$\frac{\partial U}{{\partial \xi }} + \frac{\partial V}{{\partial \eta }} = 0,\,$$
(10)
$$\frac{\partial U}{{\partial \tau }} + U\frac{\partial U}{{\partial \xi }} + V\frac{\partial U}{{\partial \eta }} = \alpha \frac{{\partial^{2} U}}{{\partial \eta^{2} }} - \beta \left( {\frac{\partial U}{{\partial \eta }}} \right)^{2} \frac{{\partial^{2} U}}{{\partial \eta^{2} }} - {\text{Re}} MU,\,$$
(11)
$$\frac{\partial \theta }{{\partial \tau }} + U\frac{\partial \theta }{{\partial \xi }} + V\frac{\partial \theta }{{\partial \eta }} = \frac{1}{\Pr }\left( {1 + Rd} \right)\,\frac{{\partial^{2} \theta }}{{\partial \eta^{2} }} + Nb\frac{\partial \theta }{{\partial \eta }}\frac{\partial \phi }{{\partial \eta }} + Nt\left( {\frac{\partial \theta }{{\partial \eta }}} \right)^{2} + MEcU^{2} ,\,$$
(12)
$$\frac{\partial \phi }{{\partial \tau }} + U\frac{\partial \phi }{{\partial \xi }} + V\frac{\partial \phi }{{\partial \eta }} = \frac{{\partial^{2} \phi }}{{\partial \eta^{2} }} + \frac{Nt}{{Nb}}\frac{{\partial^{2} \theta }}{{\partial \eta^{2} }} - Sc\lambda \phi ,\,$$
(13)

subject to

$$\left. {\begin{array}{*{20}c} {U = 0{, }V = 0{, }\theta = 0{, }\phi = 0{\text{ at }}\tau = 0} \\ {U = Re\xi {, }V = 0{, }\theta = 1{, }\phi = 1{\text{ at }}\eta = 0} \\ {U = 0{, }V = 0{, }\theta = 0{, }\phi = 0{\text{ as }}\eta \to \infty } \\ \end{array} } \right\}.$$
(14)

In above expression the dimensionless variables are \(M\left( { = \tfrac{{\sigma_{f} B_{0}^{2} }}{{a\rho_{f} }}} \right),\) \(\alpha \left( { = \tfrac{A}{{\mu_{f} C_{1} }}} \right),\) \(Re\left( { = \tfrac{{aL_{1}^{2} }}{{\nu_{f} }}} \right),\) \(\beta \left( { = \tfrac{{A\nu_{f} }}{{2\rho_{f} C_{1}^{3} L_{1}^{4} }}} \right),\) \(\Pr \left( { = \tfrac{{\mu_{f} c_{p} }}{{k_{f} }}} \right),\) \(Nt\left( { = \tfrac{{\tau D_{T} \left( {T_{w} - T_{\infty } } \right)}}{{\nu_{f} T_{\infty } }}} \right)\), \(Rd\left( { = \tfrac{{16\sigma^{ * } T_{\infty }^{3} }}{{3k^{ * } k_{f} }}} \right),\) \(Nb\left( { = \tfrac{{\tau D_{B} \left( {C_{w} - C_{\infty } } \right)}}{{\nu_{f} }}} \right)\), \(Br\left( { = \Pr Ec} \right),\) \(Sc\left( { = \tfrac{{\nu_{f} }}{{D_{B} }}} \right)\) and \(\delta \left( { = \tfrac{{k_{r} L_{1}^{2} }}{{\nu_{f} }}} \right)\).

Entropy generation

It is defined as

$$\left. {\begin{array}{*{20}c} {E_{G} = \tfrac{k}{{T_{\infty }^{2} }}\left( {1 + \tfrac{{16\sigma^{ * } T_{\infty }^{3} }}{{3k^{ * } k_{f} }}} \right)\,\left( {\tfrac{\partial T}{{\partial y}}} \right)^{2} + \tfrac{{\sigma B_{0}^{2} }}{{T_{\infty } }}u^{2} + \tfrac{{RD_{B} }}{{T_{\infty } }}\tfrac{\partial T}{{\partial y}}\tfrac{\partial C}{{\partial y}}} \\ { + \tfrac{{RD_{B} }}{{C_{\infty } }}\left( {\tfrac{\partial C}{{\partial y}}} \right)^{2} } \\ \end{array} } \right\},\,$$
(15)

Finally we can found

$$S_{G} (\tau ,\,\zeta ,\,\eta ) = \alpha_{1} \left( {1 + Rd} \right)\,\left( {\frac{\partial \theta }{{\partial \eta }}} \right)^{2} + MBrU^{2} + L\frac{\partial \theta }{{\partial \eta }}\frac{\partial \phi }{{\partial \eta }} + L\frac{{\alpha_{2} }}{{\alpha_{1} }}\left( {\frac{\partial \phi }{{\partial \eta }}} \right)^{2} .$$
(16)

Here dimensionless parameters are \(\alpha_{1} \left( { = \tfrac{{\left( {T_{w} - T_{\infty } } \right)}}{{T_{\infty } }}} \right),\) \(S_{G} \left( { = \tfrac{{E_{G} T_{\infty } L_{1}^{2} }}{{k_{f} \left( {T_{w} - T_{\infty } } \right)}}} \right),\) \(\alpha_{2} \left( { = \tfrac{{\left( {C_{w} - C_{\infty } } \right)}}{{C_{\infty } }}} \right)\) and \(L\left( { = \tfrac{{RD_{B} \left( {C_{w} - C_{\infty } } \right)}}{{k_{f} }}} \right)\).

Solution methodology

The dimensionless partial systems are solved by numerical approach (Finite difference method). Finite difference methods for dimensionless partial systems are expressed as41,42,43:

$$\left. {\begin{array}{*{20}c} {\tfrac{\partial U}{{\partial \tau }} = \tfrac{{U_{n,\,m}^{p + 1} - U_{n,\,m}^{p} }}{\Delta \;\tau },\, \, \tfrac{\partial U}{{\partial \xi }} = \tfrac{{U_{n + 1,\,m}^{p} - U_{n,\,m}^{p} }}{\Delta \;\xi },\, \, \tfrac{\partial U}{{\partial \eta }} = \tfrac{{U_{n,\,m + 1}^{p} - U_{n,\,m}^{p} }}{\Delta \;\eta }} \\ {\tfrac{\partial V}{{\partial \tau }} = \tfrac{{V_{n,\,m}^{p + 1} - V_{n,\,m}^{p} }}{\Delta \;\tau },\, \, \tfrac{\partial V}{{\partial \xi }} = \tfrac{{V_{n + 1,\,m}^{p} - V_{n,\,m}^{p} }}{\Delta \;\xi },\, \, \tfrac{\partial V}{{\partial \eta }} = \tfrac{{V_{n,\,m + 1}^{p} - V_{n,\,m}^{p} }}{\Delta \;\eta }} \\ {\tfrac{\partial \theta }{{\partial \tau }} = \tfrac{{\theta_{n,\,m}^{p + 1} - \theta_{n,\,m}^{p} }}{\Delta \;\tau },\, \, \tfrac{\partial \theta }{{\partial \xi }} = \tfrac{{\theta_{n + 1,\,m}^{p} - \theta_{n,\,m}^{p} }}{\Delta \;\xi },\, \, \tfrac{\partial \theta }{{\partial \eta }} = \tfrac{{\theta_{n,\,m + 1}^{p} - \theta_{n,\,m}^{p} }}{\Delta \;\eta }} \\ {\tfrac{\partial \phi }{{\partial \tau }} = \tfrac{{\phi_{n,\,m}^{p + 1} - \phi_{n,\,m}^{p} }}{\Delta \;\tau },\, \, \tfrac{\partial \phi }{{\partial \xi }} = \tfrac{{\phi_{n + 1,\,m}^{p} - \phi_{n,\,m}^{p} }}{\Delta \;\xi },\, \, \tfrac{\partial \phi }{{\partial \eta }} = \tfrac{{\phi_{n,\,m + 1}^{p} - \phi_{n,\,m}^{p} }}{\Delta \;\eta }} \\ {\tfrac{{\partial^{2} U}}{{\partial \xi^{2} }} = \tfrac{{U_{n + 2,\,m}^{p} - 2U_{n + 1,\,m}^{p} + U_{n,\,m}^{p} }}{{\left( {\Delta \;\xi } \right)^{2} }},\, \, \tfrac{{\partial^{2} U}}{{\partial \eta^{2} }} = \tfrac{{U_{n,\,m + 2}^{p} - 2U_{n,\,m + 1}^{p} + U_{n,\,m}^{p} }}{{\left( {\Delta \;\eta } \right)^{2} }}} \\ {\tfrac{{\partial^{2} V}}{{\partial \xi^{2} }} = \tfrac{{V_{n + 2,\,m}^{p} - 2V_{n + 1,\,m}^{p} + V_{n,\,m}^{p} }}{{\left( {\Delta \;\xi } \right)^{2} }},\, \, \tfrac{{\partial^{2} V}}{{\partial \eta^{2} }} = \tfrac{{V_{n,\,m + 2}^{p} - 2V_{n,\,m + 1}^{p} + V_{n,\,m}^{p} }}{{\left( {\Delta \;\eta } \right)^{2} }}} \\ {\tfrac{{\partial^{2} \theta }}{{\partial \xi^{2} }} = \tfrac{{\theta_{n + 2,\,m}^{p} - 2\theta_{n + 1,\,m}^{p} + \theta_{n,\,m}^{p} }}{{\left( {\Delta \;\xi } \right)^{2} }},\, \, \tfrac{{\partial^{2} \theta }}{{\partial \eta^{2} }} = \tfrac{{\theta_{n,\,m + 2}^{p} - 2\theta_{n,\,m + 1}^{p} + \theta_{n,\,m}^{p} }}{{\left( {\Delta \;\eta } \right)^{2} }}} \\ {\tfrac{{\partial^{2} \phi }}{{\partial \xi^{2} }} = \tfrac{{\phi_{n + 2,\,m}^{p} - 2\phi_{n + 1,\,m}^{p} + \phi_{n,\,m}^{p} }}{{\left( {\Delta \;\xi } \right)^{2} }},\, \, \tfrac{{\partial^{2} \phi }}{{\partial \eta^{2} }} = \tfrac{{\phi_{n,\,m + 2}^{p} - 2\phi_{n,\,m + 1}^{p} + \phi_{n,\,m}^{p} }}{{\left( {\Delta \;\eta } \right)^{2} }}} \\ \end{array} } \right\}\,$$
(17)

Using Eq. (17) in Eqs. (10–14) we get

$$\frac{{U_{n + 1,\,m}^{p} - U_{n,\,m}^{p} }}{\Delta \;\xi } + \frac{{V_{n,\,m + 1}^{p} - V_{n,\,m}^{p} }}{\Delta \;\eta } = 0,\,$$
(18)
$$\left. {\begin{array}{*{20}c} {\tfrac{{U_{n,\,m}^{p + 1} - U_{n,\,m}^{p} }}{\Delta \;\tau } + U_{n,\,m}^{p} \tfrac{{U_{n + 1,\,m}^{p} - U_{n,\,m}^{p} }}{\Delta \;\xi } + V_{n,\,m}^{p} \tfrac{{U_{n,\,m + 1}^{p} - U_{n,\,m}^{p} }}{\Delta \;\eta } = \alpha \tfrac{{U_{n,\,m + 2}^{p} - 2U_{n,\,m + 1}^{p} + U_{n,\,m}^{p} }}{{\left( {\Delta \;\eta } \right)^{2} }}} \\ { - \beta \left( {\tfrac{{U_{n,\,m + 1}^{p} - U_{n,\,m}^{p} }}{\Delta \;\eta }} \right)^{2} \tfrac{{U_{n,\,m + 2}^{p} - 2U_{n,\,m + 1}^{p} + U_{n,\,m}^{p} }}{{\left( {\Delta \;\eta } \right)^{2} }} - {\text{Re}} MU_{n,\,m}^{p} } \\ \end{array} } \right\},\,$$
(19)
$$\left. {\begin{array}{*{20}c} {\tfrac{{\theta_{n,\,m}^{p + 1} - \theta_{n,\,m}^{p} }}{\Delta \;\tau } + U_{n,\,m}^{p} \tfrac{{\theta_{n + 1,\,m}^{p} - \theta_{n,\,m}^{p} }}{\Delta \;\xi } + V_{n,\,m}^{p} \tfrac{{\theta_{n,\,m + 1}^{p} - \theta_{n,\,m}^{p} }}{\Delta \;\eta } = \tfrac{1}{\Pr }\left( {1 + Rd} \right)\,\tfrac{{\theta_{n,\,m + 2}^{p} - 2\theta_{n,\,m + 1}^{p} + \theta_{n,\,m}^{p} }}{{\left( {\Delta \;\eta } \right)^{2} }}} \\ {Nb\left( {\tfrac{{\theta_{n,\,m + 1}^{p} - \theta_{n,\,m}^{p} }}{\Delta \;\eta }} \right)\,\left( {\tfrac{{\phi_{n,\,m + 1}^{p} - \phi_{n,\,m}^{p} }}{\Delta \;\eta }} \right) + Nt\left( {\tfrac{{\theta_{n,\,m + 1}^{p} - \theta_{n,\,m}^{p} }}{\Delta \;\eta }} \right)^{2} + MEc\left( {U_{n,\,m}^{p} } \right)^{2} } \\ \end{array} } \right\},\,$$
(20)
$$\left. {\begin{array}{*{20}c} {\tfrac{{\phi_{n,\,m}^{p + 1} - \phi_{n,\,m}^{p} }}{\Delta \;\tau } + U_{n,\,m}^{p} \tfrac{{\phi_{n + 1,\,m}^{p} - \phi_{n,\,m}^{p} }}{\Delta \;\xi } + V_{n,\,m}^{p} \tfrac{{\phi_{n,\,m + 1}^{p} - \phi_{n,\,m}^{p} }}{\Delta \;\eta } = \tfrac{{\phi_{n,\,m + 2}^{p} - 2\phi_{n,\,m + 1}^{p} + \phi_{n,\,m}^{p} }}{{\left( {\Delta \;\eta } \right)^{2} }}} \\ {\tfrac{Nt}{{Nb}}\left( {\tfrac{{\theta_{n,\,m + 2}^{p} - 2\theta_{n,\,m + 1}^{p} + \theta_{n,\,m}^{p} }}{{\left( {\Delta \;\eta } \right)^{2} }}} \right) - Sc\lambda \phi_{n,\,m}^{p} } \\ \end{array} } \right\}\,$$
(21)

with

$$\left. {\begin{array}{*{20}c} {U_{n,\,m}^{0} = 0,\, \, V_{n,\,m}^{0} = 0,\, \, \theta_{n,\,m}^{0} = 0,\, \, \phi_{n,\,m}^{0} = 0} \\ {U_{n,\,0}^{p} = Re\left( {\xi_{n + 1} - \xi_{n} } \right),\, \, V_{n,\,0}^{p} = 0,\, \, \theta_{n,\,0}^{p} = 1,\, \, \phi_{n,\,0}^{p} = 1} \\ {U_{n,\infty }^{p} = 0,\, \, V_{n,\infty }^{p} = 0,\, \, \theta_{n,\infty }^{p} = 0,\, \, \phi_{n,\infty }^{p} = 0} \\ \end{array} } \right\}.$$
(22)

Entropy generation satisfy

$$\left. {\begin{array}{*{20}c} {S_{G} = \alpha_{1} \left( {1 + Rd} \right)\,\left( {\tfrac{{\theta_{n,\,m + 1}^{p} - \theta_{n,\,m}^{p} }}{\Delta \;\eta }} \right)^{2} + MBr\left( {U_{n,\,m}^{p} } \right)^{2} } \\ { + L\tfrac{{\theta_{n,\,m + 1}^{p} - \theta_{n,\,m}^{p} }}{\Delta \;\eta }\tfrac{{\phi_{n,\,m + 1}^{p} - \phi_{n,\,m}^{p} }}{\Delta \;\eta } + L\tfrac{{\alpha_{2} }}{{\alpha_{1} }}\left( {\tfrac{{\phi_{n,\,m + 1}^{p} - \phi_{n,\,m}^{p} }}{\Delta \;\eta }} \right)^{2} } \\ \end{array} } \right\}.$$
(23)

Discussion

Noteworthy presentation of fluid flow, entropy generation, concentration and thermal field against physical variables are graphically scrutinized.

Velocity

Significant effect of fluid parameter on velocity is illustrated in Fig. 2. Clearly velocity is augmented for fluid variable. Physically higher approximation of fluid parameter decreases viscosity, which augments fluid flow. Figure 3 outcomes impact of magnetic variable on velocity. Here velocity is decreased for magnetic variable. This decreasing behavior is because of Lorentz force.

Figure 2
figure 2

\(U\left( {\tau ,\,\xi ,\,\eta } \right)\) via \(\alpha\).

Full size image
Figure 3
figure 3

\(U\left( {\tau ,\,\xi ,\,\eta } \right)\) via \(M\).

Full size image

Temperature

Salient feature of radiation on thermal field is portrayed in Fig. 4. It is renowned that temperature augments via radiation. Figure 5 captured inspiration of random motion parameter on thermal field. Here thermal field increased through random motion variable. Figure 6 is sketched to see thermal field performance versus thermophoresis variable. One can find that temperature boosted with variation in thermophoresis effect. In fact increasing values of thermophoresis variable generates a force corresponds to nanoparticles from warm region to cold region. As a result thermal field boosted. Figure 7 is intrigued to see influence of magnetic variable on thermal field. An intensification in resistive force with variation in magnetic variable, which enhances collision between liquid particles. Therefore thermal field is augmented.

Figure 4
figure 4

\(\theta \left( {\tau ,\,\xi ,\,\eta } \right)\) via \(Rd\).

Full size image
Figure 5
figure 5

\(\theta \left( {\tau ,\,\xi ,\,\eta } \right)\) via \(Nb\).

Full size image
Figure 6
figure 6

\(\theta \left( {\tau ,\,\xi ,\,\eta } \right)\) via \(Nt\).

Full size image
Figure 7
figure 7

\(\theta \left( {\tau ,\,\xi ,\,\eta } \right)\) via \(M\).

Full size image

Concentration

Figure 8 elaborates the performance of concentration versus reaction variable (\(\delta\)). Here concentration decays with rising values of reaction variable (\(\delta\)). Significant features of concentration against random and thermophoresis variables (\(Nb\) and \(Nt\)) are drafted in Figs. 9 and 10. Clearly an intensification in concentration is noted through random and thermophoretic variables (\(Nb\) and \(Nt\)). Figure 11 reflects outcomes of concentration via Schmidt number. Larger estimation of Schmidt number decays diffusion behaviors and as a result concentration decreased.

Figure 8
figure 8

\(\phi \left( {\tau ,\,\xi ,\,\eta } \right)\) via \(\delta\).

Full size image
Figure 9
figure 9

\(\phi \left( {\tau ,\,\xi ,\,\eta } \right)\) via \(Nb\).

Full size image
Figure 10
figure 10

\(\phi \left( {\tau ,\,\xi ,\,\eta } \right)\) via \(Nt\).

Full size image
Figure 11
figure 11

\(\phi \left( {\tau ,\,\xi ,\,\eta } \right)\) via \(Sc\).

Full size image

Entropy generation

Figure 12 shows significance of magnetic variable on entropy production. A development in Lorentz force is noticed subject to magnetic variable, which augments disorderness in thermal system. As a outcome entropy generation is increased. Influence of thermophoretic variable on entropy rate is illuminated in Fig. 13. Clearly entropy optimization boosts up versus thermophoretic variable. Influence of entropy generation via Brinkman number is elucidated in Fig. 14. Larger (\(Br\)) improves the entropy rate. The consequences of radiation factor on entropy profile is displayed in Fig. 15. An intensification occurs in entropy rate with variation in radiation effect.

Figure 12
figure 12

\(S_{G} \left( {\tau ,\,\xi ,\,\eta } \right)\) via \(M\).

Full size image
Figure 13
figure 13

\(S_{G} \left( {\tau ,\,\xi ,\,\eta } \right)\) via \(Nt\).

Full size image
Figure 14
figure 14

\(S_{G} \left( {\tau ,\,\xi ,\,\eta } \right)\) via \(Br\).

Full size image
Figure 15
figure 15

\(S_{G} \left( {\tau ,\,\xi ,\,\eta } \right)\) via \(Rd\).

Full size image

Final remarks

Key main conclusions of present flow problem are displayed as:

  • Velocity field is declined against magnetic field, while opposite effect holds for thermal profile.

  • Larger fluid variable improves fluid flow.

  • An intensification in radiation boosts up entropy rate and thermal field.

  • An amplification in temperature is seen subject to slip mechanisms i.e., Brownian motion and thermophoretic diffusion.

  • An opposite impact holds for concentration through reaction and random motion variable.

  • A decrement in concentration is noticed for Schmidt number.

  • A similar effect holds for entropy production and concentration through thermophoresis variable.

  • An amplification in entropy production is seen subject to magnetic variable and Brinkman number.