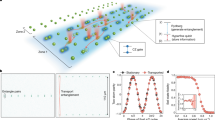
Abstract
Non-Abelian topological order is a coveted state of matter with remarkable properties, including quasiparticles that can remember the sequence in which they are exchanged1,2,3,4. These anyonic excitations are promising building blocks of fault-tolerant quantum computers5,6. However, despite extensive efforts, non-Abelian topological order and its excitations have remained elusive, unlike the simpler quasiparticles or defects in Abelian topological order. Here we present the realization of non-Abelian topological order in the wavefunction prepared in a quantum processor and demonstrate control of its anyons. Using an adaptive circuit on Quantinuum’s H2 trapped-ion quantum processor, we create the ground-state wavefunction of D4 topological order on a kagome lattice of 27 qubits, with fidelity per site exceeding 98.4 per cent. By creating and moving anyons along Borromean rings in spacetime, anyon interferometry detects an intrinsically non-Abelian braiding process. Furthermore, tunnelling non-Abelions around a torus creates all 22 ground states, as well as an excited state with a single anyon—a peculiar feature of non-Abelian topological order. This work illustrates the counterintuitive nature of non-Abelions and enables their study in quantum devices.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout






Similar content being viewed by others
Data availability
The numerical data that support the findings of this study are available from the Zenodo repository at https://doi.org/10.5281/zenodo.784842372.
Code availability
The code used for numerical simulations is available from from the Zenodo repository at https://doi.org/10.5281/zenodo.784842372.
References
Goldin, G. A., Menikoff, R. & Sharp, D. H. Comments on ‘general theory for quantum statistics in two dimensions’. Phys. Rev. Lett. 54, 603–603 (1985).
Moore, G. & Seiberg, N. Classical and quantum conformal field theory. Commun. Math. Phys. 123, 177–254 (1989).
Moore, G. & Read, N. Nonabelions in the fractional quantum Hall effect. Nucl. Phys. B 360, 362–396 (1991).
Wen, X. G. Non-Abelian statistics in the fractional quantum Hall states. Phys. Rev. Lett. 66, 802–805 (1991).
Kitaev, A. Y. Fault-tolerant quantum computation by anyons. Ann. Phys. 303, 2–30 (2003).
Nayak, C., Simon, S. H., Stern, A., Freedman, M. & Das Sarma, S. Non-Abelian anyons and topological quantum computation. Rev. Mod. Phys. 80, 1083–1159 (2008).
Wen, X.-G. Quantum Field Theory of Many-body Systems Oxford Graduate Texts (Oxford Univ. Press, 2010).
Leinaas, J. M. & Myrheim, J. On the theory of identical particles. Nuovo Cim. B 37, 1–23 (1977).
Goldin, G. A., Menikoff, R. & Sharp, D. H. Representations of a local current algebra in nonsimply connected space and the Aharonov–Bohm effect. J. Math. Phys. 22, 1664–1668 (1981).
Wilczek, F. Quantum mechanics of fractional-spin particles. Phys. Rev. Lett. 49, 957–959 (1982).
Fowler, A. G., Mariantoni, M., Martinis, J. M. & Cleland, A. N. Surface codes: towards practical large-scale quantum computation. Phys. Rev. A 86, 032324 (2012).
Nakamura, J., Liang, S., Gardner, G. C. & Manfra, M. J. Direct observation of anyonic braiding statistics. Nat. Phys. 16, 931–936 (2020).
Bartolomei, H. et al. Fractional statistics in anyon collisions. Science 368, 173–177 (2020).
Satzinger, K. J. et al. Realizing topologically ordered states on a quantum processor. Science 374, 1237–1241 (2021).
Semeghini, G. et al. Probing topological spin liquids on a programmable quantum simulator. Science 374, 1242–1247 (2021).
Ryan-Anderson, C. et al. Implementing fault-tolerant entangling gates on the five-qubit code and the color code. Preprint at https://arxiv.org/abs/2208.01863 (2022).
Iqbal, M. et al. Topological order from measurements and feed-forward on a trapped ion quantum computer. Preprint at https://arxiv.org/abs/2302.01917 (2023).
Foss-Feig, M. et al. Experimental demonstration of the advantage of adaptive quantum circuits. Preprint at https://arxiv.org/abs/2302.03029 (2023).
Pan, W. et al. Exact quantization of even-denominator fractional quantum Hall state at ν=5/2 Landau level filling factor. Phys. Rev. Lett. 83, 3530–3533 (1999).
Banerjee, M. et al. Observation of half-integer thermal Hall conductance. Nature 559, 205–210 (2018).
Ma, K. K. W., Peterson, M. R., Scarola, V. W. & Yang, K. in Encyclopedia of Condensed Matter Physics 2nd edn (ed. Chakraborty, T.) 324–365 (Academic Press, 2024); https://www.sciencedirect.com/science/article/pii/B9780323908009001359.
Willett, R. et al. Interference measurements of non-Abelian e/4 & Abelian e/2 quasiparticle braiding. Phys. Rev. X 13, 011028 (2023).
Feldman, D. E. & Halperin, B. I. Fractional charge and fractional statistics in the quantum Hall effects. Rep. Prog. Phys. 84, 076501 (2021).
Kitaev, A. Unpaired Majorana fermions in quantum wires. Phys. Uspekhi 44, 131–136 (2001).
Microsoft Quantum InAs–Al hybrid devices passing the topological gap protocol. Phys. Rev. B 107, 245423 (2023).
Bombin, H. Topological order with a twist: Ising anyons from an Abelian model. Phys. Rev. Lett. 105, 030403 (2010).
Andersen, T. I. et al. Non-Abelian braiding of graph vertices in a superconducting processor. Nature 618, 264–269 (2023).
Xu, S. et al. Digital simulation of projective non-Abelian anyons with 68 superconducting qubits. Chin. Phys. Lett. 40, 060301 (2023).
Cui, S. X., Hong, S.-M. & Wang, Z. Universal quantum computation with weakly integral anyons. Quantum Inf. Process. 14, 2687–2727 (2015).
Barkeshli, M. & Sau, J. D. Physical architecture for a universal topological quantum computer based on a network of Majorana nanowires. Preprint at https://arxiv.org/abs/1509.07135 (2015).
Barkeshli, M., Jian, C.-M. & Qi, X.-L. Theory of defects in Abelian topological states. Phys. Rev. B 88, 235103 (2013).
Barkeshli, M., Jian, C.-M. & Qi, X.-L. Genons, twist defects, and projective non-Abelian braiding statistics. Phys. Rev. B 87, 045130 (2013).
Cong, I., Cheng, M. & Wang, Z. Universal quantum computation with gapped boundaries. Phys. Rev. Lett. 119, 170504 (2017).
Wineland, D. J. et al. Experimental issues in coherent quantum-state manipulation of trapped atomic ions. J. Res. Natl Inst. Stand. Technol. 103, 259–328 (1998).
Kielpinski, D., Monroe, C. & Wineland, D. J. Architecture for a large-scale ion-trap quantum computer. Nature 417, 709–711 (2002).
Moses, S. A. et al. A race track trapped-ion quantum processor. Phys. Rev. X 13, 041052 (2023).
Bravyi, S., Hastings, M. B. & Verstraete, F. Lieb–Robinson bounds and the generation of correlations and topological quantum order. Phys. Rev. Lett. 97, 050401 (2006).
Liu, Y.-J., Shtengel, K., Smith, A. & Pollmann, F. Methods for simulating string-net states and anyons on a digital quantum computer. PRX Quantum 3, 040315 (2022).
Aharonov, D. & Touati, Y. Quantum circuit depth lower bounds for homological codes. Preprint at https://arxiv.org/abs/1810.03912 (2018).
Raussendorf, R., Bravyi, S. & Harrington, J. Long-range quantum entanglement in noisy cluster states. Phys. Rev. A 71, 062313 (2005).
Bolt, A., Duclos-Cianci, G., Poulin, D. & Stace, T. Foliated quantum error-correcting codes. Phys. Rev. Lett. 117, 070501 (2016).
Piroli, L., Styliaris, G. & Cirac, J. I. Quantum circuits assisted by local operations and classical communication: transformations and phases of matter. Phys. Rev. Lett. 127, 220503 (2021).
Tantivasadakarn, N., Vishwanath, A. & Verresen, R. Hierarchy of topological order from finite-depth unitaries, measurement, and feedforward. PRX Quantum 4, 020339 (2023).
Shi, B. Seeing topological entanglement through the information convex. Phys. Rev. Res. 1, 033048 (2019).
Tantivasadakarn, N., Thorngren, R., Vishwanath, A. & Verresen, R. Long-range entanglement from measuring symmetry-protected topological phases. Preprint at https://arxiv.org/abs/2112.01519 (2022).
Verresen, R., Tantivasadakarn, N. & Vishwanath, A. Efficiently preparing Schrödinger’s cat, fractons and non-Abelian topological order in quantum devices. Preprint at https://arxiv.org/abs/2112.03061 (2022).
Bravyi, S., Kim, I., Kliesch, A. & Koenig, R. Adaptive constant-depth circuits for manipulating non-Abelian anyons. Preprint at https://arxiv.org/abs/2205.01933 (2022).
Tantivasadakarn, N., Verresen, R. & Vishwanath, A. Shortest route to non-Abelian topological order on a quantum processor. Phys. Rev. Lett. 131, 060405 (2023).
Yoshida, B. Topological phases with generalized global symmetries. Phys. Rev. B 93, 155131 (2016).
Potter, A. C. & Vasseur, R. Symmetry constraints on many-body localization. Phys. Rev. B 94, 224206 (2016).
Senthil, T. Symmetry-protected topological phases of quantum matter. Annu. Rev. Condensed Matter Phys. 6, 299–324 (2015).
Briegel, H. J. & Raussendorf, R. Persistent entanglement in arrays of interacting particles. Phys. Rev. Lett. 86, 910–913 (2001).
Wang, C. & Levin, M. Topological invariants for gauge theories and symmetry-protected topological phases. Phys. Rev. B 91, 165119 (2015).
Wang, J., Wen, X.-G. & Yau, S.-T. Quantum statistics and spacetime surgery. Phys. Lett. B 807, 135516 (2020).
Putrov, P., Wang, J. & Yau, S.-T. Braiding statistics and link invariants of bosonic/fermionic topological quantum matter in 2+1 and 3+1 dimensions. Ann. Phys. 384, 254–287 (2017).
Kulkarni, A., Mignard, M. & Schauenburg, P. A topological invariant for modular fusion categories. Preprint at https://arxiv.org/abs/1806.03158 (2021).
Dauphinais, G. & Poulin, D. Fault-tolerant quantum error correction for non-Abelian anyons. Commun. Math. Phys. 355, 519–560 (2017).
Lu, T.-C., Lessa, L. A., Kim, I. H. & Hsieh, T. H. Measurement as a shortcut to long-range entangled quantum matter. PRX Quantum 3, 040337 (2022).
Zhu, G.-Y., Tantivasadakarn, N., Vishwanath, A., Trebst, S. & Verresen, R. Nishimori’s cat: stable long-range entanglement from finite-depth unitaries and weak measurements. Phys. Rev. Lett. 131, 200201 (2023).
Lee, J. Y., Ji, W., Bi, Z. & Fisher, M. P. A. Decoding measurement-prepared quantum phases and transitions: from Ising model to gauge theory, and beyond. Preprint at https://arxiv.org/abs/2208.11699 (2022).
Lu, T.-C., Zhang, Z., Vijay, S. & Hsieh, T. H. Mixed-state long-range order and criticality from measurement and feedback. PRX Quantum 4, 030318 (2023).
Mochon, C. Anyon computers with smaller groups. Phys. Rev. A 69, 032306 (2004).
Acknowledgements
We thank the broader team at Quantinuum for discussions, feedback and encouragement, especially D. Hayes, K. Meichanetzidis, L. Coopmans, Y. Kikuchi, P. Lee and I. Khan. R.V. thanks N. Jones and R. Sahay for comments on the manuscript. N.T. is supported by the Walter Burke Institute for Theoretical Physics at Caltech. R.V. is supported by the Harvard Quantum Initiative Postdoctoral Fellowship in Science and Engineering. A.V. and R.V. are supported by the Simons Collaboration on Ultra-Quantum Matter, which is a grant from the Simons Foundation (618615, A.V.). The experimental data in this work were produced by the Quantinuum H2 trapped-ion quantum computer, powered by Honeywell.
Author information
Authors and Affiliations
Contributions
M.I. wrote the code generating the circuits for all experiments. The experiment was built and carried out by S.L.C., J.M.D., C.F., J.P.G., J.J., M.M., S.A.M., J.M.P., A.R., M.R., P.S. and R.P.S. The data analysis and interpretation was done by M.I., N.T., M.F.-F., A.V., R.V. and H.D. N.T., A.V. and R.V. contributed to the ideation, theory and experiment design, including the definition of the operators for anyon creation, movement and annihilation. H.D. drafted the initial paper, which was refined by contributions from all authors, especially M.I., N.T., R.V. and A.V.
Corresponding author
Ethics declarations
Competing interests
H.D. is a shareholder of Quantinuum. The other authors declare no competing interests.
Peer review
Peer review information
Nature thanks Bernard van Heck and the other, anonymous, reviewer(s) for their contribution to the peer review of this work. Peer reviewer reports are available.
Additional information
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Extended data figures and tables
Extended Data Fig. 1 More detail on the steps involved in preparing the ground state.
In step 1a, we apply CZ gates on the blue and green sublattices, using the circuit optimisation techniques in the Supplementary Information. (b) shows the implementation of two adjacent \(\exp \left(\pm \frac{i{\rm{\pi }}}{8}{Z}_{p}{Z}_{\widetilde{p}}{Z}_{\widetilde{\widetilde{p}}}\right)\) gates within each square using 4 two-qubit gates. (c) as shown in (b), two adjecent \(\exp \left(\pm \frac{i{\rm{\pi }}}{8}{Z}_{p}{Z}_{\widetilde{p}}{Z}_{\widetilde{\widetilde{p}}}\right)\) gates within each square can be combined into one parallelogram of four two-qubit gates. (d) we measure the plaquette qubits of green and blue sublattices. In step 2e, we apply CZ gates between vertex and plaquette qubits of the red sublattice. (f) we measure plaquette qubits of the red sublattice. (g) shows a feed-forward action determined by the outcome of red, green and blue plaquette qubits.
Extended Data Fig. 2 Evaluating the product ∏s∈⋆As for a computational basis state.
Shown is a computational basis state with an odd number of blue strings in the horizontal and green strings in the vertical direction on which \((1-{{\mathcal{Z}}}_{GH})/2\times (1-{{\mathcal{Z}}}_{BV})/2=+\,1\). There is an odd number of red stars (one) where these strings cross. By considering the 26 possible bitstrings around a hexagon, one can verify that ∏CZ = −1 around that hexagon if and only if the corresponding star is such a crossing star. Here, the only three CZ in the product ∏s∈⋆As which evaluate to −1 are marked with solid red lines.
Extended Data Fig. 3 Effect of discarding heralded errors and measurement errors.
‘Heralded shots’ refers to the runs of the circuit where the measurement step in the ground state preparation protocol (Fig. 3c) reveals an odd number of Abelions due to noise. The spam error mitigation is described in the Supplementary Information. The average (maximal) standard error on the mean of the star and triangle operators in (a-d) is 0.014 (0.027). The average (maximal) standard error on logical operators in (a-d) is 0.007 (0.01). f denotes the lower bound on the resulting fidelity per qubit, calculated from R, G and B as defined in the Supplementary Information. All main text figures correspond to the setting chosen in (b).
Extended Data Fig. 4 Data analysis with 10-body star terms.
The model (1) has the same ground states when one deletes all but two CZ operators in each As on opposite sides of a hexagon. This figure shows the data with respect to the modified star operators and is computed by discarding heralded shots without any error mitigation (same as setting (b) in Extended Data Fig. 3).
Extended Data Fig. 5 Details on the implementation of the Borromean Rings.
(a) Going up in time the thumbnails on the right show all gates applied during the braiding sequence of the Borromean rings (cf. ‘Braiding non-Abelian anyons’ section). The solid colored lines are CZ gates and the dashed black lines denote the periodic boundary conditions. The yellow ancilla qubit is controlling all blue gates including both X and CZ. After the ground state (2) is prepared, the ancilla is initialised and measured in the X- and Y-basis (in different shots) to extract both the real and the imaginary part of the phase. Since only the trajectory of the blue non-Abelion pair is controlled, this measures the phase of a spacetime diagram that is identical to the one shown on the left plus a disconnected diagram where the blue loop is missing. However, since the red and green loops in that disconnected part of the diagram are contractible, this is topologically equivalent to the Borromean Rings. (b) Measured value for the phase of the braid containing all three colors, as well as the braids where either red or green are removed. Writing \(\left\langle {\psi }_{0}| \,{\rm{braid}}\,| {\psi }_{0}\right\rangle =r{e}^{i\phi }\), the measured values for the different braids are: red-green-blue: r = 0.80(2), ϕ = 1.02(2)π, red-blue: r = 0.81(2), ϕ = 0.07(2)π, green-blue: r = 0.86(2), ϕ = 2.00(2)π. Uncertainty is one standard error on the mean.
Extended Data Fig. 6 Non-contractible loops applied to switch logical sectors.
Operators applied to obtain all 22 ground states of the model in section V, specifically Fig. 6. Solid lines denote CZs, and dashed black lines denote the periodic boundary conditions. In the experiment, horizontal strings are applied before vertical strings. If one is interested in the highest possible fidelity state in a given sector, one may alternatively use the protocol (2) with a computational basis state in the desired sector.
Supplementary information
Supplementary Information
Supplementary Methods describing the hardware implementation, qubit reuse and circuit optimization techniques, and the fidelity lower bound proof. Supplementary Discussion on the anyon content of D4, the non-square degeneracy, as well as the colour algebra of the logical operators and the uniqueness of the prepare ground states.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Iqbal, M., Tantivasadakarn, N., Verresen, R. et al. Non-Abelian topological order and anyons on a trapped-ion processor. Nature 626, 505–511 (2024). https://doi.org/10.1038/s41586-023-06934-4
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41586-023-06934-4
This article is cited by
-
Symmetry fractionalized (irrationalized) fusion rules and two domain-wall Verlinde formulae
Journal of High Energy Physics (2024)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.