Abstract
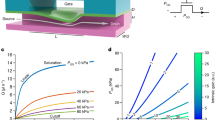
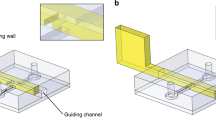
Microfluidic systems are now being designed with precision as miniaturized fluid manipulation devices that can execute increasingly complex tasks. However, their operation often requires numerous external control devices owing to the typically linear nature of microscale flows, which has hampered the development of integrated control mechanisms. Here we address this difficulty by designing microfluidic networks that exhibit a nonlinear relation between the applied pressure and the flow rate, which can be harnessed to switch the direction of internal flows solely by manipulating the input and/or output pressures. We show that these networks— implemented using rigid polymer channels carrying water—exhibit an experimentally supported fluid analogue of Braess’s paradox, in which closing an intermediate channel results in a higher, rather than lower, total flow rate. The harnessed behaviour is scalable and can be used to implement flow routing with multiple switches. These findings have the potential to advance the development of built-in control mechanisms in microfluidic networks, thereby facilitating the creation of portable systems and enabling novel applications in areas ranging from wearable healthcare technologies to deployable space systems.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout





Similar content being viewed by others
Data availability
The datasets generated and/or analysed during the current study are available from the corresponding author on reasonable request.
Code availability
Custom Python code is available from the corresponding author on request.
References
Pennathur, S. Flow control in microfluidics: are the workhorse flows adequate? Lab Chip 8, 383–387 (2008).
Stone, H. A. Microfluidics: tuned-in flow control. Nat. Phys. 5, 178–179 (2009).
Perdigones, F., Luque, A. & Quero, J. M. Correspondence between electronics and fluids in MEMS: designing microfluidic systems using electronics. IEEE Ind. Electron. Mag. 8, 6–17 (2014).
Thorsen, T., Maerkl, S. J. & Quake, S. R. Microfluidic large-scale integration. Science 298, 580–584 (2002).
Geertz, M., Shore, D. & Maerkl, S. J. Massively parallel measurements of molecular interaction kinetics on a microfluidic platform. Proc. Natl Acad. Sci. USA 109, 16540–16545 (2012).
Seker, E. et al. Nonlinear pressure-flow relationships for passive microfluidic valves. Lab Chip 9, 2691–2697 (2009).
Weaver, J. A., Melin, J., Stark, D., Quake, S. R. & Horowitz, M. A. Static control logic for microfluidic devices using pressure-gain valves. Nat. Phys. 6, 218–223 (2010).
Tanyeri, M., Ranka, M., Sittipolkul, N. & Schroeder, C. M. Microfluidic Wheatstone bridge for rapid sample analysis. Lab Chip 11, 4181–4186 (2011).
Kim, S.-J., Lai, D., Park, J. Y., Yokokawa, R. & Takayama, S. Microfluidic automation using elastomeric valves and droplets: reducing reliance on external controllers. Small 8, 2925–2934 (2012).
Li, L., Mo, J. & Li, Z. Nanofluidic diode for simple fluids without moving parts. Phys. Rev. Lett. 115, 134503 (2015).
Chin, C. D., Linder, V. & Sia, S. K. Commercialization of microfluidic point-of-care diagnostic devices. Lab Chip 12, 2118–2134 (2012).
Araci, I. E., Su, B., Quake, S. R. & Mandel, Y. An implantable microfluidic device for self-monitoring of intraocular pressure. Nat. Med. 20, 1074–1078 (2014).
Bhatia, S. N. & Ingber, D. E. Microfluidic organs-on-chips. Nat. Biotechnol. 32, 760–772 (2014).
Sackmann, E. K., Fulton, A. L. & Beebe, D. J. The present and future role of microfluidics in biomedical research. Nature 507, 181–189 (2014).
Leslie, D. C. et al. Frequency-specific flow control in microfluidic circuits with passive elastomeric features. Nat. Phys. 5, 231–235 (2009).
Mosadegh, B. et al. Integrated elastomeric components for autonomous regulation of sequential and oscillatory flow switching in microfluidic devices. Nat. Phys. 6, 433–437 (2010).
Duncan, P. N., Nguyen, T. V. & Hui, E. E. Pneumatic oscillator circuits for timing and control of integrated microfluidics. Proc. Natl Acad. Sci. USA 110, 18104–18109 (2013).
Duncan, P. N., Ahrar, S. & Hui, E. E. Scaling of pneumatic digital logic circuits. Lab Chip 15, 1360–1365 (2015).
Doh, I. & Cho, Y.-H. Passive flow-rate regulators using pressure-dependent autonomous deflection of parallel membrane valves. Lab Chip 9, 2070–2075 (2009).
Collino, R. R. et al. Flow switching in microfluidic networks using passive features and frequency tuning. Lab Chip 13, 3668–3674 (2013).
Stroock, A. D. et al. Chaotic mixer for microchannels. Science 295, 647–651 (2002).
Squires, T. M. & Quake, S. R. Microfluidics: fluid physics at the nanoliter scale. Rev. Mod. Phys. 77, 977–1026 (2005).
Amini, H., Lee, W. & Di Carlo, D. Inertial microfluidic physics. Lab Chip 14, 2739–2761 (2014).
Zhang, J. et al. Fundamentals and applications of inertial microfluidics: a review. Lab Chip 16, 10–34 (2016).
Tesař, V. & Bandalusena, H. C. H. Bistable diverter valve in microfluidics. Exp. Fluids 50, 1225–1233 (2011).
Amini, H. et al. Engineering fluid flow using sequenced microstructures. Nat. Commun. 4, 1826 (2013).
Sudarsan, A. P. & Ugaz, V. M. Multivortex micromixing. Proc. Natl Acad. Sci. USA 103, 7228–7233 (2006).
Di Carlo, D., Edd, J. F., Humphry, K. J., Stone, H. A. & Toner, M. Particle segregation and dynamics in confined flows. Phys. Rev. Lett. 102, 094503 (2009).
Wang, X. & Papautsky, I. Size-based microfluidic multimodal microparticle sorter. Lab Chip 15, 1350–1359 (2015).
Xia, H. M. et al. Analyzing the transition pressure and viscosity limit of a hydroelastic microfluidic oscillator. Appl. Phys. Lett. 104, 024101 (2014).
Braess, D. Über ein Paradoxon aus der Verkehrsplanung. Unternehmensforschung 12, 258–268 (1968).
Braess, D., Nagurney, A. & Wakolbinger, T. On a paradox of traffic planning. Transport. Sci. 39, 446–450 (2005).
Rojas, S. & Koplik, J. Nonlinear flow in porous media. Phys. Rev. E 58, 4776–4782 (1998).
Andrade, J. S. Jr, Costa, U. M. S., Almeida, M. P., Makse, H. A. & Stanley, H. E. Inertial effects on fluid flow through disordered porous media. Phys. Rev. Lett. 82, 5249–5252 (1999).
Fourar, M., Radilla, G., Lenormand, R. & Moyne, C. On the non-linear behavior of a laminar single-phase flow through two and three-dimensional porous media. Adv. Water Resour. 27, 669–677 (2004).
Adams, M. L., Johnston, M. L., Scherer, A. & Quake, S. R. Polydimethylsiloxane based microfluidic diode. J. Micromech. Microeng. 15, 1517–1521 (2005).
Calvert, B. & Keady, G. Braess’s paradox and power-law nonlinearities in networks. J. Aust. Math. Soc. Ser. B 35, 1–22 (1993).
Penchina, C. M. Braess’s paradox and power-law nonlinearities in five-arc and six-arc two-terminal networks. Open Transplant. J. 3, 8–14 (2009).
Ayala, L. F. & Blumsack, S. The Braess paradox and its impact on natural-gas-network performance. Oil Gas Facilities 2, 52–64 (2013).
Cohen, J. E. & Horowitz, P. Paradoxical behavior of mechanical and electrical networks. Nature 352, 699–701 (1991).
Youn, H., Gastner, M. T. & Jeong, H. Price of anarchy in transportation networks: efficiency and optimality control. Phys. Rev. Lett. 101, 128701 (2008).
Nicolaou, Z. G. & Motter, A. E. Mechanical metamaterials with negative compressibility transitions. Nat. Mater. 11, 608–613 (2012).
Pala, M. G. et al. Transport inefficiency in branched-out mesoscopic networks: an analog of the Braess paradox. Phys. Rev. Lett. 108, 076802 (2012).
Motter, A. E. & Timme, M. Antagonistic phenomena in network dynamics. Annu. Rev. Condens. Matter Phys. 9, 463–484 (2018).
Crane Co. Engineering Division. Flow of Fluids through Valves, Fittings, and Pipe. Technical paper no. 410 (Crane Co., 2010).
Khodaparast, S., Borhani, N. & Thome, J. R. Sudden expansions in circular microchannels: flow dynamics and pressure drop. Microfluid. Nanofluidics 17, 561–572 (2014).
Bhargava, K. C., Thompson, B. & Malmstadt, N. Discrete elements for 3D microfluidics. Proc. Natl Acad. Sci. USA 111, 15013–15018 (2014).
OpenFOAM v4.1 (OpenFOAM Foundation, 2016).
Geuzaine, C. & Remacle, J.-F. Gmsh: a three-dimensional finite element mesh generator with built-in pre- and post-processing facilities. Int. J. Numer. Methods Eng. 79, 1309–1331 (2009).
Oh, K. W., Lee, K., Ahn, B. & Furlani, E. P. Design of pressure-driven microfluidic networks using electric circuit analogy. Lab Chip 12, 515–545 (2012).
Zeitoun, R. I., Langelier, S. M. & Gill, R. T. Implications of variable fluid resistance caused by start-up flow in microfluidic networks. Microfluid. Nanofluidics 16, 473–482 (2014).
Zovatto, L. & Pedrizzetti, G. Flow about a circular cylinder between parallel walls. J. Fluid Mech. 440, 1–25 (2001).
Gervais, T., El-ali, J., Gunther, A. & Jensen, K. F. Flow-induced deformation of shallow microfluidic channels. Lab Chip 6, 500–507 (2006).
Christov, I. C., Cognet, V., Shidhore, T. C. & Stone, H. A. Flow rate–pressure drop relation for deformable shallow microfluidic channels. J. Fluid Mech. 841, 267–286 (2018).
Amstad, E., Datta, S. S. & Weitz, D. A. The microfluidic post-array device: high throughput production of single emulsion drops. Lab Chip 14, 705–709 (2014).
Haudin, F., Callewaert, M., De Malsche, W. & De Wit, A. Influence of nonideal mixing properties on viscous fingering in micropillar array columns. Phys. Rev. Fluids 1, 074001 (2016).
Zhao, H., Liu, Z., Zhang, C., Guan, N. & Zhao, H. Pressure drop and friction factor of a rectangular channel with staggered mini pin fins of different shapes. Exp. Therm. Fluid Sci. 71, 57–69 (2016).
Kim, M., Huang, Y., Choi, K. & Hidrovo, C. H. The improved resistance of PDMS to pressure-induced deformation and chemical solvent swelling for microfluidic devices. Microelectron. Eng. 124, 66–75 (2014).
Johnston, I. D., McCluskey, D. K., Tan, C. K. L. & Tracey, M. C. Mechanical characterization of bulk sylgard 184 for microfluidics and microengineering. J. Micromech. Microeng. 24, 035017 (2014).
Martin, R. S., Gawron, A. J., Lunte, S. M. & Henry, C. S. Dual-electrode electrochemical detection for poly(dimethylsiloxane)-fabricated capillary electrophoresis microchips. Anal. Chem. 72, 3196–3202 (2000).
Duffy, D. C., McDonald, J. C., Schueller, O. J. A. & Whitesides, G. M. Rapid prototyping of microfluidic systems in poly(dimethylsiloxane). Anal. Chem. 70, 4974–4984 (1998).
Lachaux, J. et al. Thermoplastic elastomer with advanced hydrophilization and bonding performances for rapid (30 s) and easy molding of microfluidic devices. Lab Chip 17, 2581–2594 (2017).
Acknowledgements
This research was supported by the US National Science Foundation (grants PHY-1001198 and CHE-1900011), the Simons Foundation (award number 342906) and a Northwestern University Presidential Fellowship.
Author information
Authors and Affiliations
Contributions
D.J.C., J.-R.A. and A.E.M. designed the overall study and formulated the theory. Y.L. and I.Z.K. designed and performed the experiments. D.J.C. implemented the numerical simulations and analyses. All authors contributed to the writing of the manuscript, which was led by D.J.C. and A.E.M. All authors reviewed and approved the final manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Peer review information Nature thanks Sujit Datta and Dino Di Carlo for their contribution to the peer review of this work.
Supplementary information
Supplementary Information
Additional theoretical, simulation, and experimental results across six sections, fifteen figures, and one table are included. It contains two sections with details of the theoretical network model and four sections on further simulations and experiments of nonlinear flow behaviour, switching, Braess’s paradox, and multiswitch networks.
Rights and permissions
About this article
Cite this article
Case, D.J., Liu, Y., Kiss, I.Z. et al. Braess’s paradox and programmable behaviour in microfluidic networks. Nature 574, 647–652 (2019). https://doi.org/10.1038/s41586-019-1701-6
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41586-019-1701-6
This article is cited by
-
The fluidic memristor as a collective phenomenon in elastohydrodynamic networks
Nature Communications (2024)
-
Impact of physicality on network structure
Nature Physics (2024)
-
Understanding Braess’ Paradox in power grids
Nature Communications (2022)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.