Abstract
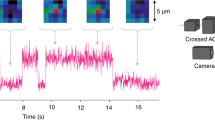
In 1872, Maxwell proposed his famous ‘demon’ thought experiment1. By discerning which particles in a gas are hot and which are cold, and then performing a series of reversible actions, Maxwell’s demon could rearrange the particles into a manifestly lower-entropy state. This apparent violation of the second law of thermodynamics was resolved by twentieth-century theoretical work2: the entropy of the Universe is often increased while gathering information3, and there is an unavoidable entropy increase associated with the demon’s memory4. The appeal of the thought experiment has led many real experiments to be framed as demon-like. However, past experiments had no intermediate information storage5, yielded only a small change in the system entropy6,7 or involved systems of four or fewer particles8,9,10. Here we present an experiment that captures the full essence of Maxwell’s thought experiment. We start with a randomly half-filled three-dimensional optical lattice with about 60 atoms. We make the atoms sufficiently vibrationally cold so that the initial disorder is the dominant entropy. After determining where the atoms are, we execute a series of reversible operations to create a fully filled sublattice, which is a manifestly low-entropy state. Our sorting process lowers the total entropy of the system by a factor of 2.44. This highly filled ultracold array could be used as the starting point for a neutral-atom quantum computer.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Maxwell, J. C. Theory of Heat (Longmans, Green and Co., London, 1871).
Leff, H. S. & Rex, A. F. Maxwell’s Demon: Entropy, Information, Computing (Princeton University Press, Princeton, 1990).
Brillouin, L. Maxwell’s demon cannot operate – information and entropy. 1. J. Appl. Phys. 22, 334–337 (1951).
Landauer, R. Irreversibility and heat generation in the computing process. IBM J. Res. Dev. 5, 183–191 (1961).
Price, G. N., Bannerman, S. T., Viering, K., Narevicius, E. & Raizen, M. G. Single-photon atomic cooling. Phys. Rev. Lett. 100, 093004 (2008).
Barredo, D., de Leseleuc, S., Lienhard, V., Lahaye, T. & Browaeys, A. An atom-by-atom assembler of defect-free arbitrary two-dimensional atomic arrays. Science 354, 1021–1023 (2016).
Endres, M. et al. Atom-by-atom assembly of defect-free one-dimensional cold atom arrays. Science 354, 1024–1027 (2016).
Robens, C. et al. Low-entropy states of neutral atoms in polarization-synthesized optical lattices. Phys. Rev. Lett. 118, 065302 (2017).
Lester, B. J., Luick, N., Kaufman, A. M., Reynolds, C. M. & Regal, C. A. Rapid production of uniformly filled arrays of neutral atoms. Phys. Rev. Lett. 115, 073003 (2015).
Strasberg, P., Schaller, G., Brandes, T. & Esposito, M. Thermodynamics of a physical model implementing a Maxwell demon. Phys. Rev. Lett. 110, 040601 (2013).
Barredo, D., Lienhard, V., de Léséleuc, S., Lahaye, T. & Browaeys, A. Synthetic three-dimensional atomic structures assembled atom by atom. Nature http://dx.doi.org/10.1038/s41586-018-0450-2 (2018).
Kim, H. et al. In situ single-atom array synthesis using dynamic holographic optical tweezers. Nat. Commun. 7, 13317 (2016).
Saffman, M. Quantum computing with atomic qubits and Rydberg interactions: progress and challenges. J. Phys. B 49, 202001 (2016).
Wang, Y., Kumar, A., Wu, T. Y. & Weiss, D. S. Single-qubit gates based on targeted phase shifts in a 3D neutral atom array. Science 352, 1562–1565 (2016).
Weiss, D. S. et al. Another way to approach zero entropy for a finite system of atoms. Phys. Rev. A 70, 040302 (2004).
Bernien, H. et al. Probing many-body dynamics on a 51-atom quantum simulator. Nature 551, 579–584 (2017).
Nelson, K. D., Li, X. & Weiss, D. S. Imaging single atoms in a three-dimensional array. Nat. Phys. 3, 556–560 (2007).
Li, X., Corcovilos, T. A., Wang, Y. & Weiss, D. S. 3D projection sideband cooling. Phys. Rev. Lett. 108, 103001 (2012).
Wang, Y., Zhang, X. L., Corcovilos, T. A., Kumar, A. & Weiss, D. S. Coherent addressing of individual neutral atoms in a 3D optical lattice. Phys. Rev. Lett. 115, 043003 (2015).
Deutsch, I. H. & Jessen, P. S. Quantum-state control in optical lattices. Phys. Rev. A 57, 1972–1986 (1998).
Vala, J. et al. Perfect pattern formation of neutral atoms in an addressable optical lattice. Phys. Rev. A 71, 032324 (2005).
Jaksch, D., Bruder, C., Cirac, J. I., Gardiner, C. W. & Zoller, P. Cold bosonic atoms in optical lattices. Phys. Rev. Lett. 81, 3108–3111 (1998).
Olshanii, M. & Weiss, D. Producing Bose–Einstein condensates using optical lattices. Phys. Rev. Lett. 89, 090404 (2002).
Chu, S., Hollberg, L., Bjorkholm, J. E., Cable, A. & Ashkin, A. Three-dimensional viscous confinement and cooling of atoms by resonance radiation pressure. Phys. Rev. Lett. 55, 48–51 (1985).
Anderson, M. H., Ensher, J. R., Matthews, M. R., Wieman, C. E. & Cornell, E. A. Observation of Bose–Einstein condensation in a dilute atomic vapor. Science 269, 198–201 (1995).
Szilard, L. Über die Entropieverminderung in einem thermodynamischen System bei Eingriffen intelligenter Wesen. Z. Phys. 53, 840–856 (1929).
Gaunt, A. L., Schmidutz, T. F., Gotlibovych, I., Smith, R. P. & Hadzibabic, Z. Bose–Einstein condensation of atoms in a uniform potential. Phys. Rev. Lett. 110, 200406 (2013).
Williams, R. A. et al. Dynamic optical lattices: two-dimensional rotating and accordion lattices for ultracold atoms. Opt. Express 16, 16977–16983 (2008).
Hu, J. Z. et al. Creation of a Bose-condensed gas of Rb-87 by laser cooling. Science 358, 1078–1080 (2017).
Jaksch, D., Briegel, H. J., Cirac, J. I., Gardiner, C. W. & Zoller, P. Entanglement of atoms via cold controlled collisions. Phys. Rev. Lett. 82, 1975–1978 (1999).
Kaufman, A. M. et al. Entangling two transportable neutral atoms via local spin exchange. Nature 527, 208–211 (2015).
Raussendorf, R. & Briegel, H. J. A one-way quantum computer. Phys. Rev. Lett. 86, 5188–5191 (2001).
Labuhn, H. et al. Tunable two-dimensional arrays of single Rydberg atoms for realizing quantum Ising models. Nature 534, 667–670 (2016).
Weimer, H., Muller, M., Lesanovsky, I., Zoller, P. & Buchler, H. P. A Rydberg quantum simulator. Nat. Phys. 6, 382–388 (2010).
Acknowledgements
This work was supported by the US National Science Foundation through grant PHY-1520976.
Author information
Authors and Affiliations
Contributions
All authors contributed to the design, execution and analysis of the experiment and the writing of the manuscript. A.K., T.-Y.W. and F.G. collected all the data.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Extended data figures and tables
Extended Data Fig. 1 Motion step.
A motion step to move n atoms is shown. n atoms are sequentially targeted by the addressing beams and transferred from the ‘stationary’ state to the ‘motion’ state using microwaves. The electro-optic modulator (EO) voltages are ramped up to the half-wave voltage (Vλ/2) in order to move atoms by half of the lattice spacing. After motion, the atoms are optically pumped so that they all return to the stationary state. The EO voltages are then ramped back down. A final optical pumping (OP) ensures optimal preparation for the next motion step.
Extended Data Fig. 2 Motion fidelities.
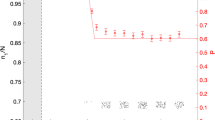
a, b, Measured motion fidelity as a function of the number of motion steps in |F = 4, mF = −4〉 (a) and |F = 3, mF = −3〉 (b) in the x (maroon circles), y (blue squares) and z (green diamonds) directions. The lines are fits to the data. The error bars represent one standard deviation. Each point corresponds to about 600 atoms.
Source data
Rights and permissions
About this article
Cite this article
Kumar, A., Wu, TY., Giraldo, F. et al. Sorting ultracold atoms in a three-dimensional optical lattice in a realization of Maxwell’s demon. Nature 561, 83–87 (2018). https://doi.org/10.1038/s41586-018-0458-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41586-018-0458-7
Keywords
This article is cited by
-
Scalable quantum processors empowered by the Fermi scattering of Rydberg electrons
Communications Physics (2023)
-
Entanglement in the quantum phases of an unfrustrated Rydberg atom array
Nature Communications (2023)
-
Realizing spin squeezing with Rydberg interactions in an optical clock
Nature (2023)
-
Classically verifiable quantum advantage from a computational Bell test
Nature Physics (2022)
-
Universality of Dicke superradiance in arrays of quantum emitters
Nature Communications (2022)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.