Abstract
Exciton–polaritons (polaritons) resulting from the strong exciton–photon interaction stimulates the development of novel low-threshold coherent light sources to circumvent the ever-increasing energy demands of optical communications1,2,3. Polaritons from bound states in the continuum (BICs) are promising for Bose–Einstein condensation owing to their theoretically infinite quality factors, which provide prolonged lifetimes and benefit the polariton accumulations4,5,6,7. However, BIC polariton condensation remains limited to cryogenic temperatures ascribed to the small exciton binding energies of conventional material platforms. Herein, we demonstrated room-temperature BIC polariton condensation in perovskite photonic crystal lattices. BIC polariton condensation was demonstrated at the vicinity of the saddle point of polariton dispersion that generates directional vortex beam emission with long-range coherence. We also explore the peculiar switching effect among the miniaturized BIC polariton modes through effective polariton−polariton scattering. Our work paves the way for the practical implementation of BIC polariton condensates for integrated photonic and topological circuits.
Similar content being viewed by others
Introduction
Exciton–polaritons (polaritons for short), as hybrid bosonic quasiparticles resulting from the strong coupling between semiconductor excitons and microcavity photons, can undergo Bose−Einstein condensation at elevated temperatures, promising for low-threshold coherent emitters8,9, all-optical logic circuits10,11, and quantum simulators12,13. Microcavities with higher quality factors (Q factors) and longer coherence times are always desirous for enhanced exciton–photon coupling. Possessing theoretically infinite-high cavity Q factors and peculiar non-radiative characteristics, the bound states in the continuum (BICs) exhibit great promise in vortex beam generation and topological modulation in the linear regime4,5,14,15, and also low-threshold nanolaser16,17,18, as well as polariton accumulation19,20,21,22,23. However, the potential of polaritons from BICs (BIC polaritons) for providing unrivaled nonlinearities and topological characteristics in strong interaction scenarios is still in its infancy. Recently, BIC polariton condensation has been realized in a patterned GaAs quantum well waveguide at cryogenic temperature (~4 K)6,7,24. The room-temperature operation of this topological macroscopic quantum state is fascinating, while it is a fundamental restriction for conventional III–V semiconductors with low exciton binding energies.
Lead halide perovskites with large exciton binding energies embedded in microcavities are deemed as superb polaritonic platforms for room-temperature polariton condensation25,26,27,28. Various proof-of-principle condensate devices, ranging from Hamiltonian simulators and quantum fluids to investigations of rich polariton physics in non-Abelian gauge fields, have been extensively explored13,29,30,31,32,33. To date, the demonstration of perovskite polariton condensation without vertical Fabry–Pérot bulky microcavities, which is crucial for practical integrated photonics seeking more designable and compact structures, remains limited34. On the other hand, owing to the high optical gain, easily tunable bandgap, high defect tolerance, and good processability35,36,37,38, perovskite single crystals are of particular interest as laser gain media, particularly the recently demonstrated BIC photonic lasing39,40. Nevertheless, the intriguing Bose–Einstein condensation resulting from the strong coupling between excitons and BIC cavity photons has not been exploited yet in such perovskite single crystal-based structure, from which low-threshold, large nonlinearities, and topological characteristics of polariton condensates are expected to be generated at room temperature.
In this study, we demonstrate room-temperature BIC polariton condensation in perovskite artificial photonic crystal (PhC) lattice, by combining the high-quality BIC modes with stable excitons. Single-crystalline perovskite microplatelets with robust excitons enable strong coupling between BIC mode and excitons with a Rabi splitting larger than 150 meV. Long-range coherent vortex emission in the vertical direction with a low divergence angle is obtained from the BIC polariton condensates. Additionally, we successfully switch between multiple orders of BIC polariton modes with preserved topology in a miniaturized structure size through the nonlinear interactions of polariton condensates. Our work provides a platform to couple coherent polariton condensates with orbital angular momentum at room temperature, serving as a significant basis for integrated photonic and topological circuits.
Results and discussion
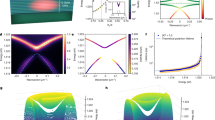
Figure 1a schematically depicts room-temperature BIC polariton condensation based on a perovskite air-hole PhC lattice. All-inorganic cesium lead bromide (CsPbBr3) is selected as a suitable room-temperature polaritonic material owing to its large exciton binding energy and superior optical properties35,41. Relatively large-area (>20 μm), single-crystalline CsPbBr3 microplatelets were synthesized on silicon substrates through chemical vapor deposition, where the lattice match between CsPbBr3 and silicon led to a vertically-grown feature, restricting the deposition only on the microplatelets’ exposed edges (see Methods section). Thereby, this confined growth, together with a slow nucleation rate, results in CsPbBr3 microplatelets with exceptional crystal quality and atomic-level smooth surfaces without grain boundaries (Supplementary Fig. S1). Moreover, the photoluminescence (PL) emission exhibits a narrow full width at half maximum (FWHM) of ~60.7 meV, and a single-exponential decay featuring a lifetime of ~6.0 ns, suggesting a low trap density and reduced non-radiative loss. The strong excitonic peak observed in the reflection spectrum convinces the robust excitons against thermal ionization at room temperature (Supplementary Fig. S2). Subsequently, the periodic air-hole PhC lattice was carved on the CsPbBr3 microplatelet by the focused ion-beam (FIB) milling, with radius r = 57 nm, thickness h = 145 ± 5 nm, and periodicity a = 290−300 nm (a = 300 nm for Fig. 1b). A 30 nm polymethyl methacrylate layer was spin-coated onto the CsPbBr3 microplatelet for both protection and refractive index matching. The sharp sidewalls and smooth surfaces (Supplementary Fig. S3) indicate negligible damage caused by FIB milling (see discussion of different etching conditions in Supplementary Note 1). Meanwhile, both red-shifted emission and shortened lifetime were observed in the PhC lattice compared to the pristine microplatelet ascribing to the enhanced exciton−photon coupling strength (Supplementary Fig. S2).
a Schematic of room-temperature BIC polariton condensation from an air-hole CsPbBr3 PhC lattice. A localized vortex beam is generated along the surface normal direction pumped by a non-resonant pulsed laser. b Tilt-view SEM image of the air-hole CsPbBr3 PhC lattice. The thickness, period, and diameter of the air holes are 150, 300, and 150 nm, respectively. Scale bar: 300 nm. c Calculated energy-angle dispersions of the CsPbBr3 PhC lattice mode in TM polarization. d Calculated Q factors of the four PhC lattice modes plotted in c (Solid curves: eye-guided). The BICs are demonstrated by the near-zero linewidth and ultrahigh Q factor at the normal incidence. e Angle-resolved reflectance spectrum of the air-hole CsPbBr3 PhC lattice in TM polarization. LPB with an anti-crossing characteristic is distinguished resulting from the strong coupling between the exciton and PhC lattice mode. The fittings are based on the coupled harmonic oscillator model, and a Rabi splitting value of 158 meV is obtained.
Figure 1c shows the calculated transverse magnetic (TM) polarized energy-angle dispersion of Bloch resonances in the PhC lattice within the gain bandwidth of CsPbBr3 (see Methods section, Supplementary Note 2). Here, 0° corresponds to the high-symmetry point (Γ) of the first Brillouin zone in the square PhC lattice. Four mode dispersions stretch around the Γ-point, with two breakpoints observed in Modes 1 and 3. The faded visibility and narrowed linewidth of these two modes around the Γ-point suggest dark states with ultrahigh Q factors approaching infinite, corresponding to the symmetry-protected BICs decoupled from the radiation continuum (Fig. 1d). The bright Mode 2 and top of Mode 3 possesses an energy range from 2.34 to 2.39 eV around the detection angle, in near resonance with the excitonic emission of CsPbBr3 (2.41 eV). Moreover, angle and energy-resolved reflectance spectra (see Methods section, Supplementary Fig. S4) reveal similar characters of two dominated modes with decreasing curvature approaching the excitonic resonance, which matches well with the theoretically calculated dispersion of lower polariton (LP) branch, labeled as LP2 and LP3. Notably, the upper polariton (UP) branches could not be identified due to the strong absorption occurring above the exciton energy 42. In addition, the air-hole PhC lattice, fabricated with 40 periods, exhibits a narrow mode linewidth closely resembling that of an infinite structure (Supplementary Fig. S5). A Rabi splitting energy of 158 meV and detuning Δ = −74.6 meV could be extracted from LP3, confirming the realization of strong exciton–photon coupling (Fig. 1e). Remarkably, a similar trend of linewidth narrowing of LP3 could be observed from 18.8 meV to a minimum of 2.5 meV (Q factor ~913) near the Γ-point (Supplementary Fig. S6) in measured reflectance spectrum, demonstrating the formation of symmetry-protected BIC polariton mode.
The presence of BIC polariton mode within a hybrid system provides a state with a theoretically infinite Q factor, enabling ultrastrong net gain while minimizing losses in the mode dispersion, which favors polariton accumulation towards condensation. Here, femtosecond-pulsed laser excitation with an expanded square spot size of ~10 μm was applied to explore the nonlinear regime of polariton interactions (see Methods section, Supplementary Fig. S7). Figure 2a shows the angle-resolved PL spectrum of a sample with a detuning (Δ) of −74.6 meV at room temperature with a low pump density of 0.6 times the condensation threshold (Pth). The dispersion is similar to that in Fig. 1d and emission is dominated at bright polariton mode LP2. As the pump density increased to Pth, a two-lobe emission occurred at the dispersion saddle point of LP3 in reciprocal space (Fig. 2b), and then dominated the entire spectrum as the pump density further increased to 1.5 Pth (Fig. 2c), suggesting the formation of the BIC polariton condensates. This nonlinear behavior is further characterized in detail by the linear-to-superliner transition of the integrated intensity (Fig. 2d), decrease in the linewidth (Fig. 2e, Supplementary Fig. S8), and energy blueshift of the BIC polariton emission (Fig. 2f) as a function of the pump density (extracted from pump density-dependent emission spectra in Supplementary Fig. S9). Remarkably, the blueshift exhibited a larger slope below Pth and a smaller slope above Pth, corresponding to the distinct interaction strengths of polariton−reservoir and polariton−polariton interactions, respectively 43. As a reference, the sample with a weak negative detuning (Δ = −64.1 meV) shows a lower Pth and larger slope of energy blueshift. This enhanced nonlinear performance is consistent with the increase of the excitonic fraction19, further confirming the realization of BIC polariton condensation (Supplementary Fig. S10). More results of BIC polariton condensations at different detuning are available in Supplementary Note 3. Additionally, despite the ubiquitous optical birefringence in orthorhombic CsPbBr3 single crystals, BIC polariton condensation can be only observed in TM polarization, which could be attributed to the longer lifetime of the TM-polarized state resulting from a higher excitonic fraction (Supplementary Fig. S11).
a–c Angle-resolved PL spectra under pump densities at 0.6 Pth (a): the emission exhibits a broad angular distribution (±15°); Pth (b): the emission near the dispersion saddle point shows a sharp increase within a narrow angular distribution (±5°), suggesting the onset of polariton condensation; 1.5 Pth (c): the state at the dispersion saddle point is massively occupied. Two samples with distinct detuning energies of −74.6 and −64.1 meV are explored. d Integrated emission intensity as a function of the pump density, showing a linear-to-superliner transition across Pth. e Linewidth as a function of the pump density, showing a clear narrowing effect across Pth. The error bar represents the standard deviation. f Energy blueshift as a function of the pump density, where two regimes are distinguished below and above Pth attributing to the polariton−reservoir and polariton−polariton interactions, respectively. g Time-resolved and angle-resolved PL emission snapshots of an identical sample at three distinct times of 0.4, 0.8, and 8 ps, respectively. h Integrated emission intensity as a function of the pump density of CsPbBr3 PhC lattices (left two blue lines) and pristine microplatelets (right three orange lines) with similar sizes under quasi-CW excitation at 80 K. BIC polariton condensation exhibits a threshold reduction of over 50% compared to pure photonic lasing.
Furthermore, Fig. 2g presents the time- and angle-resolved emission snapshots of BIC polariton condensation at 1.6 Pth and three specific time points of 0.4, 0.8, and 8 ps (see Methods section and more data in Supplementary Note 4). The rapid establishment and maximum intensity of the two lobes of the BIC polariton condensate occur within 0.8 ps. Subsequently, the emission intensity gradually decreases with a continuous redshift, indicating a decrease in condensed polariton density and weakened polariton nonlinearity. Remarkably, the profile of the two lobes remains unchanged during the time evolution, and the emission always comes from the BIC polariton mode. The BIC polariton lifetime is predominantly limited by the finite cavity quality factor, which could be further alleviated through optimized fabrication processes (see discussion in Supplementary Note 4). Additionally, the BIC polariton condensation can also be realized under quasi-continuous-wave laser excitation (pulse duration: 100 ns, repetition rate: 100 kHz) at 80 K (Supplementary Fig. S12). Compared to the photonic lasing from pristine CsPbBr3 microplatelets with similar sizes, BIC polariton condensation exhibits a threshold reduction of over 50%, reflecting its low-threshold characteristic and the potential for further achieving continuous optically pumped operation at elevated temperatures.
The long-range spatial and temporal coherence is another conclusive evidence for the formation of the polariton condensates, while the correlation length is constrained by the thermal de Broglie wavelength in the thermal phase26,44. The coherence feature was characterized using the first-order correlation function g(1) measured by the Michelson interferometer (see Methods section, Supplementary Fig. S13). Figure 3a illustrates the interference pattern of BIC polariton condensate emission at zero-time delay and the extracted intensity line profile along y = 0. Compared with the limited correlation length below Pth, clear interference fringes, extending over the entire PhC lattice, were observed above Pth. Gaussian fitting of the intensity profile yielded an FWHM of 8.7 μm, suggesting the realization of long-range spatial coherence (Supplementary Fig. S13). Moreover, temporal coherence was probed by scanning time-delayed interference patterns, where the maximum fringe visibility was observed at zero-time delay. As the delay time extended from 0 to 1.5 ps (P1−P3), the fringes gradually blurred (Fig. 3b). The temporal coherence was quantified by fitting the visibility decay from the magnitudes of the time-delayed interference patterns (Fig. 3c). The coherence time of BIC polariton condensate emission, determined by fitting a Gaussian function, was approximately one order of magnitude longer (2.14 ± 0.16 ps) than that of the referenced pump laser (0.31 ± 0.01 ps). Additionally, Fig. 3d reveals a decreasing trend of temporal coherence with increasing pump density, attributed to phase decoherence within the polariton lasing mode resulting from enhanced polariton−polariton scattering 43,45. This trend aligns with the broadening of emission linewidth (Fig. 2e) and accelerated decay rate at higher pump density (Supplementary Note 4) above Pth. Note that longer coherence time can be achieved by further optimization of the material quality and fabrication processes (Supplementary Fig. S14). Thus, the observed long-range spatial and temporal coherences confirm the onset of room-temperature BIC polariton condensation. It should be noted that recently, polariton condensation has also been demonstrated in organic materials combining with BICs in silicon metasurfaces46, further underscoring the broad applicability of BIC polariton condensates at room temperature.
a Top, interference patterns of BIC polariton condensates in a typical CsPbBr3 PhC lattice after the superposition of two mirror-symmetric images by the Michelson interferometer. Bottom, line profile of the coherence intensity extracted from the interference pattern (along y = 0). b Interference patterns (the dashed region in a) acquired at different time delays (Δt) of 0, 0.5, and 1.5 ps (denoted as P1, P2, and P3), respectively. The emission intensity at Δt = 0 was normalized for clarity. c Visibility of interference fringe as a function of delay time for BIC polariton condensation (purple) and the photonic lasing (orange). The visibility is obtained from time-dependent coherence fringes through the Fourier transform and fitted with the Gaussian function. Coherence times of 2.14 ps and 0.31 ps could be obtained for the BIC polariton condensation and the referenced pump laser, respectively. The yellow stars correspond to the three situations (P1, P2, and P3) in b. d Coherence time as a function of the pump density. The dots are taken from the measured temporal coherence for each value of pump density. The fitting line is calculated from power-dependent temporal coherence with an exponential function. The decrease in coherence time with increasing pump density is attributed to polariton–polariton scattering, which induces phase decoherence within the polariton lasing mode.
Next, we investigate the inherent topological properties of the BIC polariton condensates, as the emission of vortex beams with a topological charge is anticipated, stemming from the orbital angular momentum inherited from the BIC mode4,5. Figure 4a, b show the back-focal plane (BFP) images of the CsPbBr3 PhC lattice emission below and above Pth, respectively. Below Pth, the emission covers the entire plane of momentum space, while surpassing Pth leads to the emergence of a donut-shaped radiation pattern dominated by an in-plane momentum of 0.1 k0 (where k0 represents the maximum collecting momentum) This observation indicates nearly collimated output in the normal direction. The central singularity in the donut-shaped pattern arises due to the non-radiative nature of the emission at Γ point in the BIC. Remarkably, the simulation result of Fig. 4c matches well with the measured BFP image (see discussion in Supplementary Note 5). Furthermore, the presence of polarization singularities in BIC polariton condensates is confirmed through a full polarization-resolved measurement of BFP images (Fig. 4d), along with the corresponding Stokes parameters S1−S3 maps (Fig. 4e, see Methods section). These measurements reveal the vortex profile and the unambiguous singularity point at Γ point. The polarization orientations around Γ point align with a vortex of topological charge l = −123. Additionally, the phase singularity of the BIC polariton condensate emission is further demonstrated by the self-interference measurement based on the same Michelson interferometer with momentum-space images (Fig. 4f and Supplementary Fig. S15). As the momentum-space images from the left arm and its inversion counterpart in the horizontal coordinates from the right arm become progressively misaligned with increasing distances, the two fork-shaped stripes remain while the number of fringes between them gets more (Supplementary Fig. S16), confirming the existence of orbital angular momentum in the vortex radiation with a topological charge of l = −1, inherited from the BIC mode4,47,48.
a, b Fourier space images of the PL emission excited with the pump density below (a) and above Pth (b). The dashed circle in b corresponds to an in-plane momentum of 0.2k0, in which a donut-shaped BIC polariton condensate emission pattern is presented. c The simulated Fourier space image corresponding to a. d Far-field radiation patterns of the BIC polariton condensate vortex emission in six representative polarization directions. e Stokes parameters S1−S3 map of the BIC polariton condensate vortex emission above Pth. f Self-interference pattern of Fourier space images observed by the Michelson interferometer. Pairs of mirror-symmetric fork-shaped stripes reveal the nature of orbital angular momentum in BIC polariton condensate vortex emission.
Besides, the finite lateral dimension of the CsPbBr3 PhC lattice and the interface between different optical modes and/or optical bandgap contribute to light trapping in the transverse direction, leading to the formation of discrete photonic modes. Accordingly, the BIC modes can be transformed into a series of localized modes Mpq (p, q are integers) in the transverse direction, which have recently been referred to as “miniaturized BIC”49,50. We experimentally verified the existence of these modes and successfully switched between them by applying a signal beam and a gate beam with different time delays (Fig. 5a), which is ubiquitous for polariton condensates due to the pronounced nonlinear interactions. The profile of the gating beam is set to be half the width of the PhC lattice to enhance the switching performance (Supplementary Figs. S17, 18). The switching of the miniaturized BIC polaritonic mode M12 is achieved through polariton−polariton scattering at the fundamental M11 mode, which is ultimately determined by the temporal evolution of the condensed BIC polariton density (Fig. 5b). The M12 mode undergoes an on/off transition when the delay time is shifted from negative to positive (left panel of Fig. 5c). The far-field image of M12 reveals highly directional emission at the four corners while retaining the central hollow characteristic of M11 (right panel of Fig. 5c). Moreover, the measured Stokes parameters provide clear evidence that the polarization singularity at Γ point is preserved in both the M11 and M12 modes (Fig. 5d). No additional singularity is observed in M12, suggesting that M12 remains a symmetry-protected BIC polariton mode rather than accidental BIC. Additionally, Fig. 5e illustrates the temporal evolution of M12 at various delay times. The intensity of M12 undergoes a rapid reduction near zero-time delay, corresponding to a decrease in intensity by 12.5 dB within a time span of 17 ps. The corresponding far-field pattern also demonstrates the transition from M12-dominated emission to the fundamental M11 mode at positive delay times. This switching of miniaturized BIC polariton modes, exhibiting different energy levels while maintaining the identical topology, holds great promise for on-chip communication with enhanced light−matter interaction.
a Schematic of the experimental setup. A signal beam (~Pth) and a gate beam (~0.2 Pth) with different time delays are applied. The gating beam excited half the width of the PhC lattice, while the signal beam excited the whole CsPbBr3 PhC lattice. b Schematic of the switching mechanism of the miniaturized BIC polariton modes. c Left panel: angle-resolved PL spectra of the miniaturized BIC polariton condensate emission at distinct time delays of 32 ps and −25 ps, respectively. Right panel: the far-field momentum-space images of M11 and M12 modes. d Stokes parameters S1−S3 map of M11 and M12 modes. e Intensity as a function of the time delay for the M12 mode. Inset: the corresponding far-field momentum-space images at time delays of −25, 0, and 15 ps, respectively.
In conclusion, we demonstrate room-temperature BIC polariton condensation in perovskite air-hole PhCs. The utilization of BIC states provides a high-quality cavity, suppressing radiative losses and facilitating polariton accumulation. The resulting BIC polariton condensates exhibit long-range coherence and emit coherent vortex beams with low divergence angles in the vertical direction. Additionally, we realize switching between multiple orders of BIC polariton modes, while preserving their topological properties, in a miniaturized structure through the nonlinear interactions of polariton condensates. Our findings provide a pathway to achieve room-temperature coherent polariton condensates with orbital angular momentum, unlocking new possibilities for the utilization of integrated polaritonic devices at room temperature with enriched degrees of freedom.
Methods
Materials
Single-crystalline CsPbBr3 microplatelets were fabricated by the chemical vapor deposition method. Initially, CsBr (99.99%, Sigma Aldrich) and PbBr2 (99.99%, Sigma Aldrich) powder precursors were thoroughly mixed with a molar ratio of 1:1. The resulting mixture was then placed at the center of a quartz tube equipped with a heating furnace. Next, SiO2/Si substrates were meticulously cleaned in an ultrasonic bath filled successively with ethanol, acetone, and deionized water for 15 min each, then dried with nitrogen gas, and subsequently placed downstream of the precursors. To ensure a controlled growth environment, the chamber was purged with high-purity nitrogen and vacuumed to a pressure of 0.5 Pa. The mixed precursors were heated to 575 °C and maintained at this temperature for 10 minutes, carried by high-purity nitrogen flow under a pressure of 200 Torr and flux rate of 40 sccm. Finally, the entire facility cooled naturally to room temperature.
Fabrications
The periodic air-hole CsPbBr3 PhC lattices were fabricated by FIB milling. Using the FEI Nova 200 NanoLab FIB system, 10 × 10 µm2 arrays of periodic air holes were patterned on the CsPbBr3 microplatelet. The nominal ion-beam current was controlled below 10 pA for a suitable spot size of etching. Note that because perovskite is sensitive to the damage of ion etching, etching paths of ion-beam scanning on the perovskite microplatelets were optimized using the stream file function in the FEI system, and the fabrication dwell time was set as a maximum of 2 ms to minimize damages in repetition.
Morphology and structure characterizations
SEM images were conducted using Zeiss GEMINI II. X-ray measurements were performed on a Rigaku SmartLab diffractometer using CuKα radiation (λ = 1.5406 Å). Atomic force microscope images were obtained using a BRUKER Dimension Icon.
Optical characterizations
The absorption spectrum of individual CsPbBr3 microplatelet was obtained by a home-built micro transmission/absorption spectrometer, which has been described in our previous work51. To obtain angle-resolved reflection and PL spectra, a custom-made Fourier imaging system was utilized, incorporating an objective lens (×50, numerical aperture of 0.8) to focus light and improve spatial resolution. In reflection measurement, the white light beam from a tungsten-halogen light source (SLS201L, Thorlabs) was focused with the objective lens onto the sample. The reflected light was collected to a Horiba iHR-550 spectrometer with a liquid nitrogen-cooled charge-coupled device detector and a grating of 1800 grooves per mm. For pulsed laser excitation, an 800 nm pulse laser beam generated from a Coherent Vitara-s oscillator (35 fs, 80 MHz), which was seeded by the Coherent Astrella amplifier (80 fs, 1 kHz), was doubled to 400 nm with a pulse duration of 300 fs and repetition rates of 1 kHz through a barium boron oxide crystal. The pump spot was modulated by placing an adjustable rectangular aperture followed by a lens of 300 mm focal length before entering the Fourier system. In addition, two pump sources with adjustable delay time were realized by a beam splitter and retroreflectors coupled with a delay stage. A schematic of the optical system setup is provided in Supplementary Fig. S4.
Time-resolved PL spectrum was conducted by a time-correlated single photon counting (TCSPC, SPC-150) system (below condensed threshold) and a home-built ultrafast optical Kerr gating (OKG) system (above condensed threshold). In detail, the TCSPC system was carried by an excitation source of a 400 nm, 80 MHz laser from Ti: sapphire oscillator (Chameleon Ultra, Coherent) and focused onto the sample by a ×100 objective (numerical aperture of 0.9) with a temporal resolution of ~40 ps. The time and angle-resolved PL is developed based on an optical Kerr gate coupled with the Fourier imaging system. The femtosecond pulses of 35 fs @ 800 nm, as mentioned previously, are divided into two parts. One part is frequency doubled to generate femtosecond pulses at the central wavelength of 400 nm. The sample is excited non-resonantly by focusing excitation pulses at 400 nm onto it, which leads to the excitation of polariton condensates. The remaining femtosecond pulses at 800 nm are precisely delayed relative to the UV excitation pulses and focused onto the Kerr medium. By varying the delay time of the OKG, the angle-resolved distribution of polariton condensate emission can be visualized frame by frame with sub-picosecond resolution.
Coherence and self-interference measurements were carried out by a Michelson interferometer attached to the Fourier imaging system. The emission pattern was split into two arms, and the image in one of the arms was inverted upside-down with a retro-reflector while the image in the other arm was kept constant. Then the two images with mirror symmetry were superposed again with a beam splitter and overlapped at the image plane simultaneously. The interference patterns were collected by a CMOS camera (Sunny Optics) for further analysis.
Polarization measurement was carried out by the combination of a half-waveplate (linear polarization) or a quarter-waveplate (circular polarization) with a linear polarizer before the entrance of the imaging camera. In this way, maps of four linear polarizations (horizontal IH, vertical IV, diagonal ID, antidiagonal IA) and two circular polarizations (right IR and left IL) were obtained. Three Stokes parameters can be calculated as: \({S}_{1}=\frac{{I}_{H}-{I}_{V}}{{I}_{H}+{I}_{V}}\), \({S}_{2}=\frac{{I}_{D}-{I}_{A}}{{I}_{D}+{I}_{A}}\), and \({S}_{3}=\frac{{I}_{R}-{I}_{L}}{{I}_{R}+{I}_{L}}\).
Numerical simulation methods
We used the commercial software Lumerical FDTD to perform the mode dispersions and Fourier space distribution. All the geometric parameters in the simulations were taken from the SEM images. The wavelength-dependent complex permittivity of CsPbBr3 was obtained from the literature39. Numerical simulation of angle-resolved reflection and far-field distribution are described in detail in Supplementary Notes 2 and 5.
Data availability
All data to evaluate the conclusions are present in the manuscript, and the Supplementary Material. Raw data are available from the corresponding authors on request.
References
Kasprzak, J. et al. Bose–Einstein condensation of exciton polaritons. Nature 443, 409–414 (2006).
Deng, H., Haug, H. & Yamamoto, Y. Exciton-polariton Bose-Einstein condensation. Rev. Mod. Phys. 82, 1489–1537 (2010).
Byrnes, T., Kim, N. Y. & Yamamoto, Y. Exciton–polariton condensates. Nat. Phys. 10, 803–813 (2014).
Zhen, B., Hsu, C. W., Lu, L., Stone, A. D. & Soljacic, M. Topological nature of optical bound states in the continuum. Phys. Rev. Lett. 113, 257401 (2014).
Hsu, C. W., Zhen, B., Stone, A. D., Joannopoulos, J. D. & Soljačić, M. Bound states in the continuum. Nat. Rev. Mater. 1, 1–13 (2016).
Ardizzone, V. et al. Polariton Bose-Einstein condensate from a bound state in the continuum. Nature 605, 447–452 (2022).
Riminucci, F. et al. Nanostructured GaAs/(Al, Ga)As waveguide for low-density polariton condensation from a bound state in the continuum. Phys. Rev. Appl. 18, 024039 (2022).
Imamoglu, A., Ram, R. J., Pau, S. & Yamamoto, Y. Nonequilibrium condensates and lasers without inversion: Exciton-polariton lasers. Phys. Rev. A 53, 4250–4253 (1996).
Sanvitto, D. & Kena-Cohen, S. The road towards polaritonic devices. Nat. Mater. 15, 1061–1073 (2016).
Amo, A. et al. Exciton–polariton spin switches. Nat. Photonics 4, 361–366 (2010).
Feng, J. et al. All-optical switching based on interacting exciton polaritons in self-assembled perovskite microwires. Sci. Adv. 7, eabj6627 (2021).
Struck, J. et al. Quantum simulation of frustrated classical magnetism in triangular optical lattices. Science 333, 996–999 (2011).
Tao, R. et al. Halide perovskites enable polaritonic XY spin Hamiltonian at room temperature. Nat. Mater. 21, 761–766 (2022).
Shi, T. et al. Planar chiral metasurfaces with maximal and tunable chiroptical response driven by bound states in the continuum. Nat. Commun. 13, 4111 (2022).
Kang, M. et al. Coherent full polarization control based on bound states in the continuum. Nat. Commun. 13, 4536 (2022).
Hwang, M.-S. et al. Ultralow-threshold laser using super-bound states in the continuum. Nat. Commun. 12, 4135 (2021).
Hwang, M.-S., Jeong, K.-Y., So, J.-P., Kim, K.-H. & Park, H.-G. Nanophotonic nonlinear and laser devices exploiting bound states in the continuum. Commun. Phys. 5, 106 (2022).
Kodigala, A. et al. Lasing action from photonic bound states in continuum. Nature 541, 196–199 (2017).
Kravtsov, V. et al. Nonlinear polaritons in a monolayer semiconductor coupled to optical bound states in the continuum. Light Sci. Appl. 9, 56 (2020).
Lu, L. et al. Engineering a light–matter strong coupling regime in perovskite-based plasmonic metasurface: quasi-bound state in the continuum and exceptional points. Photonics Res. 8, 91–100 (2020).
Xie, P. et al. Strong coupling between excitons in a two-dimensional atomic crystal and quasibound states in the continuum in a two-dimensional all-dielectric asymmetric metasurface. Phys. Rev. B 104, 125446 (2021).
Al-Ani, I. A. M., As’Ham, K., Huang, L., Miroshnichenko, A. E. & Hattori, H. T. Enhanced strong coupling of WSe2 monolayer by bound state in the continuum. J. Phys. Conf. Ser. 2172, 012009 (2022).
Dang, N. H. M. et al. Realization of polaritonic topological charge at room temperature using polariton bound states in the continuum from perovskite metasurface. Adv. Opt. Mater. 10, 2102386 (2022).
Grudinina, A. et al. Collective excitations of a bound-in-the-continuum condensate. Nat. Commun. 14, 3464 (2023).
Su, R. et al. Room-temperature polariton lasing in all-inorganic perovskite nanoplatelets. Nano Lett. 17, 3982–3988 (2017).
Su, R. et al. Observation of exciton polariton condensation in a perovskite lattice at room temperature. Nat. Phys. 16, 301–306 (2020).
Du, W., Zhang, S., Zhang, Q. & Liu, X. Recent progress of strong exciton–photon coupling in lead halide perovskites. Adv. Mater. 31, 1804894 (2019).
Su, R. et al. Perovskite semiconductors for room-temperature exciton-polaritonics. Nat. Mater. 20, 1315–1324 (2021).
Spencer, M. S. et al. Spin-orbit–coupled exciton-polariton condensates in lead halide perovskites. Sci. Adv. 7, eabj7667 (2021).
Su, R. et al. Direct measurement of a non-Hermitian topological invariant in a hybrid light-matter system. Sci. Adv. 7, eabj8905 (2021).
Su, R., Ghosh, S., Liew, T. C. H. & Xiong, Q. Optical switching of topological phase in a perovskite polariton lattice. Sci. Adv. 7, eabf8049 (2021).
Zhang, S. et al. All-optical control of rotational exciton polaritons condensate in perovskite microcavities. ACS Photonics 10, 2414–2422 (2023).
Peng, K. et al. Room-temperature polariton quantum fluids in halide perovskites. Nat. Commun. 13, 7388 (2022).
Masharin, M. A. et al. Room-temperature exceptional-point-driven polariton lasing from perovskite metasurface. Adv. Funct. Mater. 33, 2215007 (2023).
Zhang, Q. et al. High-quality whispering-gallery-mode lasing from cesium lead halide perovskite nanoplatelets. Adv. Funct. Mater. 26, 6238–6245 (2016).
Kang, J. & Wang, L.-W. High defect tolerance in lead halide perovskite CsPbBr3. J. Phys. Chem. Lett. 8, 489–493 (2017).
Du, W. et al. All optical switching through anistropic gain of CsPbBr3 single crystal microplatelet. Nano Lett. 22, 4049–4057 (2022).
Zhong, Y. et al. Large-scale thin CsPbBr3 single-crystal film grown on sapphire via chemical vapor deposition: toward laser array application. ACS Nano 14, 15605–15615 (2020).
Huang, C. et al. Ultrafast control of vortex microlasers. Science 367, 1018–1021 (2020).
Wang, Y. et al. Highly controllable etchless perovskite microlasers based on bound states in the continuum. ACS Nano 15, 7386–7391 (2021).
Su, R. et al. Room temperature long-range coherent exciton polariton condensate flow in lead halide perovskites. Sci. Adv. 4, eaau0244 (2018).
Guillet, T. & Brimont, C. Polariton condensates at room temperature. C. R. Phys. 17, 946–956 (2016).
Kim, S. et al. Coherent polariton laser. Phys. Rev. X 6, 011026 (2016).
Pitaevskii, L. & Stringari, S. Bose-Einstein condensation, Vol. 5. (Oxford University Press, 2003).
Haug, H., Doan, T. D., Cao, H. T. & Thoai, D. B. T. Temporal first- and second-order correlations in a polariton condensate. Phys. Rev. B 85, 205310 (2012).
Berghuis, A. M. et al. Room temperature exciton–polariton condensation in silicon metasurfaces emerging from bound states in the continuum. Nano Lett. 23, 5603–5609 (2023).
Zhai, Z. et al. Multimode vortex lasing from dye–TiO2 lattices via bound states in the continuum. ACS Photonics 10, 437–446 (2023).
Lan, B. et al. The topological charge measurement of the vortex beam based on dislocation self-reference interferometry. Phys. Scr. 94, 055502 (2019).
Chen, Z. et al. Observation of miniaturized bound states in the continuum with ultra-high quality factors. Sci. Bull. 67, 359–366 (2022).
Ren, Y. et al. Low-threshold nanolasers based on miniaturized bound states in the continuum. Sci. Adv. 8, eade8817 (2022).
Du, W. et al. Unveiling lasing mechanism in CsPbBr3 microsphere cavities. Nanoscale 11, 3145–3153 (2019).
Acknowledgements
Q.Z. acknowledges funding support from the Natural Science Foundation of Beijing Municipality (JQ21004), and the National Natural Science Foundation of China (52072006 and U23A2076). X.L. acknowledges funding support from the National Key Research and Development Program (2023YFA1507002), the National Science Foundation for Distinguished Young Scholars of China (22325301), the National Natural Science Foundation of China (22073022, 11874130, 12074086, 12204123, and 22173025), China Postdoctoral Science Foundation (2022M710925), the Strategic Priority Research Program of Chinese Academy of Sciences (XDB36000000), Beijing Municipal Natural Science Foundation (1222030), and the CAS Instrument Development Project (Y950291). Q.X. acknowledges funding support from the National Natural Science Foundation of China (12020101003) and strong support from the State Key Laboratory of Low-Dimensional Quantum Physics at Tsinghua University.
Author information
Authors and Affiliations
Contributions
X.L. and Q.Z. conceived the idea and led the project. X.L., Q.Z., X.W. S.Z., and J.S. designed experiments. X.W., W.D. and Y.Z. produced the samples. X.W., S.Z., J.S., X.Z., Z.Zhu., C.J., Y.C. and Y.W. performed the optical spectroscopy. X.W., X.D., X.Z. and S.Z. conducted the numerical simulations. X.L., Q.Z., Y.Z., B.W., Q.X., X.W., S.Z., J.S., Y.Z., Z.Zhang, and Y.L. analyzed the data. X.L., Q.Z., Q.X., X.W., S.Z., J.S., X.Z. and X.D. prepared the manuscript. X.L., Q.Z., Q.X. supervised the studies. All the authors discussed the results and revised the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Peer review
Peer review information
Nature Communications thanks the anonymous reviewers for their contribution to the peer review of this work. A peer review file is available.
Additional information
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Wu, X., Zhang, S., Song, J. et al. Exciton polariton condensation from bound states in the continuum at room temperature. Nat Commun 15, 3345 (2024). https://doi.org/10.1038/s41467-024-47669-8
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41467-024-47669-8
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.