Introduction

Phase engineering of group-VI transition metal dichalcogenides (TMDs) such as MoS2 and WS2 is important for acquiring novel physical and chemical properties1,2,3,4,5,6. Depending on coordination modes between the transition metal and chalcogen atoms, these TMDs present in either trigonal prismatic coordinated semiconductive phases (2H and 3R) or octahedral coordinated metallic phases (2M, Td, 1T, 1T′, etc.)1,2,3,6. Bulk 2M and the monolayered (ML) 1T′ WS2 have shown plenty of unique appealing properties, such as superconductivity7,8,9,10, Weyl semimetal states11,12, optical nonlinearity13,14 and enhanced electrochemical activities15,16,17,18,19. However, the 1T′ TMDs are metastable and tend to transform to the thermodynamically stable 2H phase1,3,4, hence their practical applications are significantly limited1,3. Although various strategies such as electron doping20,21, strain effect22,23, and heterostructural interaction24,25 can be adopted to stabilize the metallic phase TMDs, their intrinsic stabilities under practical operation conditions (e.g., temperature and atmosphere) remain unclear.

As van der Waals (vdW)-bonded layered materials, physicochemical properties of TMDs can dramatically change when their structures evolve from three-dimensional bulk to ultrathin two-dimensional (2D) regime. For example, band gaps of 2H MoS2 and WS2 turn from indirect to direct after thickness is reduced to monolayer26. Dielectric screening in gapped 2D crystals significantly reduces with decrease of layer number, leading to greatly enhanced Coulomb interactions27. The high Coulombic interactions have resulted in tightly bonded exitons and trions in ML MoS227, as well as anormal thickness-dependent Raman shifts28. Atomically thin TMDs exhibit much higher electrocatalytic activities than bulk matrixes, because of “self-gating effect” induced high carrier densities29,30. The in-plane inversion symmetry of bulk TMDs is broken after they are reduced to ML, which produces out-of-plane spin polarization depending on the valley (K or −K point) in momentum space2. Thermal conductivity is found to decrease with increase of layer number for thin 2H MoS2 and Td WTe231,32, while the opposite trend is seen for thin indium selenides33,34. In a word, the layer number plays critical roles in both the electronic and phonon properties of 2D TMDs. As 2M to 2H phase transition of WS2 (or 1T′ to 1H for ML WS2) involves changes in energy band structures of both electron and phonon, the layer number could greatly affect the stability of 2M WS2. However, the relation between layer number and stability of 2M WS2 is barely known to the best of our knowledge.

Here, we investigate the thickness-dependent intrinsic phase stability of mechanically exfoliated 2M WS2 and find that thinner samples have higher thermal stabilities. 2M to 2H phase transition temperature increases from 120 °C to 210 °C in the air as thickness of WS2 is reduced from bulk to bilayer (2L). ML WS2 can maintain 1T′ structure in the air and argon (Ar) atmosphere until temperature reaches 350 °C and 450 °C, respectively, which are about two and three times higher than phase transition temperatures of bulk 2M WS2. Raman spectroscopy reveals thinner 2M WS2 has more stiffened intralayer W‒W and W‒S bonds and higher thermal conductance, which enables thinner samples with higher resistance to lattice deformation and higher efficiency heat dissipation under elevated temperature. Theoretical simulation and calculation further confirm thinner WS2 has a higher average energy barrier per layer during the layer(s)-by-layer(s) phase transition process.

Results

Thickness-dependent phase stability of 2M WS2

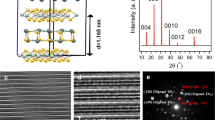
2M WS2 is synthesized through solid-state chemical method as reported previously7. The as-synthesized 2M WS2 shows distinctive Raman peaks at 110.9, 117.1, 177.2, 241.7, 268.6, 316.3, and 406.5 cm−1 (black line in Fig. 1d), the same as previously reported results3,7. Rietveld refinement based on powder X-ray diffraction (XRD) (Supplementary Fig. 1 and Supplementary Table 1) reveals the synthesized WS2 has monoclinic structure in C2/m space group with cell parameters a = 12.8417 Å, b = 3.2177 Å, c = 5.6912 Å and β = 112.8368°, which is in excellent agreement with the previously reported 2M WS2 structure3,7. X-ray photoelectron spectroscopy (XPS) (Supplementary Fig. 2) further evidences the synthesized sample is composed of only W and S elements. All these results confirm the high purity of the synthesized 2M WS2. Once 2M to 2H phase change is triggered by heat or oxidation, two additional Raman peaks appear at around 350.2 and 420.0 cm−1 (blue line in Fig. 1d) that are attributed to the A1g and \({E}_{2{{\mbox{g}}}}^{1}\) vibration modes of 2H WS23. After the WS2 flake is completely converted to 2H phase, all the 2M characteristic vibration modes disappear and only the A1g and \({E}_{2{{\mbox{g}}}}^{1}\) peaks can be seen (red line in Fig. 1d). Accordingly, the intensity of \({E}_{2{{\mbox{g}}}}^{1}\) mode can be used as an indicator for the extent of phase change within a 2M WS2 flake.

Fig. 1: Thickness-dependent phase stability of 2M WS2 on Si/SiO2 substrate.
figure 1

a Optical and b atomic force microscope (AFM) images and mappings of E12g Raman mode and photoluminescence (PL) intensities of a piece of exfoliated 2M WS2 flake with different thicknesses areas at different temperatures. AFM image is measured at room temperature, and the corresponding height profile is shown in Supplementary Fig. 3f. c Optical images, AFM image, and mappings of E12g Raman mode and PL intensities of a piece of exfoliated monolayered (ML) 1T′ WS2 flake at room temperature in the air and after heated at 450 °C in Ar atmosphere. Mappings measured area is outlined by dotted lines in the optical image. All the scale bars in (a–c) correspond to 2 μm. d Raman spectra of a 2M WS2 and an intermediate phase and 2H WS2 that were obtained by heating multilayered 2M WS2 at 120 °C for 5 min and at 250 °C for 20 min, respectively. e 2M to 2H (or 1T′ to 1H) phase transition temperatures (circle points) and air oxidation temperature (triangle point) as functions of WS2 layer thickness, measured in the air or Ar atmosphere with temperature elevated by 5 °C and held for 1 min or 15 min in each step. Solid lines are guides to the eye. f The power of laser required to activate 2M to 2H phase transition as a function of WS2 layer thickness measured in the air. A solid line is a guide to the eye.

Full size image

Here, we define phase transition temperature as the one at which the 2M or 1T′ WS2 starts to lose phase purity, i.e., the \({E}_{2{{\mbox{g}}}}^{1}\) Raman mode emerges, or the WS2 sample begins to be oxidized by the air. To investigate the thickness-dependent phase stability, 2M WS2 flakes are mechanically exfoliated into thin layers. Figure 1a,  b shows optical and atomic force microscope (AFM) images of a piece of exfoliated 2M WS2 flake with thickness varying from ML to 4L. This sample was treated with stepped heating in the air with temperature elevated by 5 °C and held for 1 min in each stage, during which optical images and mappings of \({E}_{2{{\mbox{g}}}}^{1}\) Raman mode and photoluminescence (PL) intensities are acquired to address the location of 2M to 2H (or 1T′ to 1H) phase change, as shown in Fig. 1a, b. It is found that the \({E}_{2{{\mbox{g}}}}^{1}\) mode can be observed at the 4L area when temperature goes up to 180 °C, whereas it does not appear at the 3L and 2L areas until the temperature goes to 190 °C, 210 °C, respectively. With temperature increasing from 180 °C to 325 °C, the \({E}_{2{{\mbox{g}}}}^{1}\) mode intensities of 3–4L areas keep increasing and the transparency is obviously decreasing, while the 2L area is too thin to tell transparency change. It is known that 2H WS2 has higher refractive index and lower transparency than 2M WS235. PL peaks (Supplementary Fig. 3a–c) at ~640 nm emerge in 2–4L areas as temperature reaches 325 °C, further confirming the 2M to 2H phase transitions36. The PL intensities are significantly enhanced after heating to 350 °C, as shown in Supplementary Fig. 3a–c, consistent with the increase of \({E}_{2{{\mbox{g}}}}^{1}\) mode intensity at elevated temperature. These results indicate 2M to 2H phase transition is a slow process, and the extent of phase transition augments at elevated temperatures or after longer time of heating. PL intensity of WS2 is sensitive to thickness26, and the distribution of PL intensity (Fig. 1b) agrees to the AFM defined areas of various thicknesses from 2L to 4L. Comparatively, the ML WS2 area preserves the typical 1T′ Raman pattern without emergence of \({E}_{2{{\mbox{g}}}}^{1}\) mode or PL until temperature reaches 350 °C (Fig. 1b and Supplementary Fig. 3d, e), evidencing the highest thermal stability among the four WS2 areas. In agreement with this result, chemically exfoliated ML 1T′ WS2 is also demonstrated to have good air-stability at room temperature37,38. Heated at 350 °C in the air, Raman signal of the ML 1T′ WS2 fades away and the ML 1T′ WS2 flake disappears, seen from Fig. 1a, which can be attributed to decomposition due to air oxidation. In our experiments of heating in the air, we never observe oxidation of 2L or multilayered 2M WS2 flakes before 2M to 2H phase transition, while we never observe 1T′ to 1H phase transition on a ML 1T′ WS2 flake before it was oxidized and decomposed. This means 1T′ to 1H phase transition temperature of ML 1T′ WS2 is higher than the air oxidation and decomposition temperature. After moving to an inert Ar atmosphere, 1T′ to 1H phase transition temperature of ML WS2 (Fig. 1c) is measured to be as high as 450 °C, confirmed by the reduced transparency and emergences of \({E}_{2{{\mbox{g}}}}^{1}\) mode and PL (Fig. 1c). These results strongly indicate thinner 2M WS2 has higher phase stability.

To further confirm the thickness-dependent stability of 2M WS2, we have prepared exfoliated samples with thickness covering a wide range (from 23L to ML) and systematically investigated their tolerance to heat in the air or Ar atmosphere. The optical, AFM images and Raman spectra of these samples at representative conditions can be found in Supplementary Figs. 4–6. Figure 1e summarizes phase transition temperatures of the investigated 2M WS2 samples during stepped heating programs. When heating in the air with temperature held for 1 min in each stage as aforementioned, the measured phase transition temperature continuously increases from 120 °C to 350 °C as WS2 is thinned from 20L to ML (blue line in Fig. 1e). When temperature is held for 15 min in each stage, the phase transition temperatures of thicker samples (≥6L) converge to a same level of 120 °C, while the phase transition temperatures of thinner samples (1‒5L) still maintain the thickness dependence (red line in Fig. 1e). Regardless of temperature holding time, ultrathin samples (ML and 2L) exhibit significantly high phase transition temperatures that are over 200 °C. When heating in Ar atmosphere, the same trend of phase transition temperature as function of sample thickness is also observed, except all 2M (or 1T′) WS2 samples show increased phase transition temperatures compared with heating in the air (yellow line in Fig. 1e). Previous X-ray absorption fine structure measurements have confirmed enrichment of electrons on 2M or 1T′ WS2 surface3,6. It has also been demonstrated that donation of electrons helps to stabilize 1T′ TMDs, while extraction of electrons promotes 1T′ to 1H phase transition20,21,39,40,41. Thus, higher 2M to 2H (or 1T′ to 1H) phase transition temperature measured in the inert atmosphere can be attributed to getting rid of electron acceptors, such as O2 and H2O molecules in the air. High-resolution XPS (Supplementary Fig. 7) shows that W 4f, S 2p and valance band edge spectra of 2M WS2 shift to higher energies after 2M to 2H phase transition is activated by heating in the air, and higher extent of phase transition leads to larger range of shifting. Meanwhile, no peak attributed to W‒O or S‒O chemical bond can be found in the W 4 f and S 2p spectra and O 1s spectra (probably contributed by adsorbed or intercalated H2O and O2 molecules) barely change during the 2M to 2H phase transition. These results indicate air speeds up 2M to 2H phase transition during heating processes by extracting electrons from 2M WS2 without chemically oxidizing it. The thinner 2M WS2 can also withstand higher intensity incident laser during Raman measurements (Fig. 1f and Supplementary Fig. 8). From 25L to 5L WS2, the intensity of laser required to activate the 2M to 2H phase transition increases from 2.8 mW to 14.6 mW. A 48.3 mW laser, which is the maximum output of the used instrument, can turn a 4L WS2 to 2H phase, but is not able to activate the phase transition for 1‒3L 2M WS2 samples.

2M to 2H phase transition mechanism

To understand how WS2 transforms from 2M to 2H phase, we closely investigate crystal structures of the two phases. Each layer of 2M (or a layer of 1T′) WS2 contains distorted [WS6]8− octahedrons sharing edges along bc plane, and W atoms form W–W zigzag chains along b direction, resulting in nonuniform distances between W-atom lines, d1 = 2.28 Å and d2 = 3.43 Å (Fig. 2a and Supplementary Fig. 9a)7. Viewing from the b direction, W and S atoms reside in six different planes and stack in an A/A’-B/B’-C/C’ model (Fig. 2a)7. Every two neighbored S atoms located in different planes (A and C or A’ and C’) share two W atoms (Supplementary Fig. 9a). Comparatively, each layer of 2H (or a layer of 1H) WS2 is composed of standard [WS6]8− trigonal prisms with uniform W-atom line distance of d3 = 2.70 Å (Fig. 2b and Supplementary Fig. 9b)42. Viewing from the b direction, W and S atoms stack in a simple A-B-A sandwiched model (Fig. 2b), and every two neighbored S atoms located in different planes share three W atoms (Supplementary Fig. 9b)42.

Fig. 2: 2M to 2H phase transition mechanism of WS2.
figure 2

a Crystal structures of 1T′ WS2, where W and S atoms coordinate in an octahedral configuration and stack in an A/A’-B/B’-C/C’ mode. b Crystal structures of 1H WS2, where W and S atoms coordinate in a trigonal prismatic configuration and stack in an A-B-A mode. c Top view (upper) and side view (lower) of crystal lattices when overlapping of a layer of 1T′ WS2 on top of a layer of 1H WS2. Color code: blue and orange spheres represent W and S, respectively. The broken W‒W and W‒S bonds are labeled by dash lines, and the directions of W and S atoms displacements are indicated by orange arrows. Each S atom that dissociates from a W atom after breaking the W‒S bond moves to a new position (marked by a dotted line circle) and forms a new W‒S bond.

Full size image

Because of the structural differences between 2M and 2H WS2, the 2M to 2H phase change involves reforming of intralayer bond network with displacements of W and S atoms and changing of interlayer spacing. As shown in Fig. 2c, during 2M to 2H phase change of WS2, the bonded W‒W chains break up and W atoms in these chains gradually separate along c direction. The gliding of W atoms finally gives uniform W-atom line distance, approaching that in 2H WS2. In the meantime, S atoms residing in C and C’ planes glide along the bc direction to lay on top of adjacent S atoms in A and A’ planes, respectively. Subsequently, each S atom dissociates from the initially bonded W atom and forms a new covalent bond with another adjacent W atom, resulting in alteration of W–S coordination from octahedron to trigonal prism, as shown in Fig. 2c. Along with out-of-plane displacements of W and S atoms and adjusting of W–S bond lengths and W–S–W angles, each [WS6]8− layer changes from the six-plane stack (2M) to the three-plane sandwiched stack (2H) (Fig. 2c). Since 2H WS2 has smaller average W‒S bond length, W–S–W bond angle and W-atom line distance but larger interlayer spacing than the 2M WS2 (Supplementary Fig. 9), 2M to 2H phase transition experiences slight in-plane contraction and vertical expansion.

The slowness of 2M to 2H phase transition of WS2 allows us to trace this process by investigating structures of intermediate phases after varied extents of 2M to 2H phase change. Intermediate phases WS2 can be obtained by heating the 2M WS2 above phase transition temperature within controlled time. As shown in Supplementary Figs. 4–6, all intermediate phases WS2 flakes show superpositions of 2M and 2H characteristic Raman modes, indicating the intermediate phases of WS2 are probably 1T′/1H heterostructures that can involve in-plane heterostructured areas and hybrid stacked layers. Figure 3a–c show high-resolution transmission electron microscopy (HRTEM) images of W-atom configurations in 2M and an intermediate phase. Zigzag chains of W atoms are observed in Fig. 3a, in consistency with the reported structure of 2M WS23. Area [1] of the intermediate phase WS2 (Fig. 3b) reveals W-atom lines distances ranging from 0.26 to 0.31 nm, which are in between d1 (0.23 nm) and d2 (0.34 nm) of 2M WS2 (Fig. 3a). Fourier transform of Area [1] displays irregular quadrilaterals (inset of Fig. 3b) that are distorted from the rectangular pattern of 2M WS2 (inset of Fig. 3a). Area [2] of the intermediate phase WS2 has a W-atom lines distance of 0.27 nm (Fig. 3c) and hexagonal pattern Fourier transform (inset of Fig. 3c), which are the same as 2H WS242. These results demonstrate that an intermediate phase WS2 has an in-plane heterostructure of distorted 1T′ and 1H, which is formed due to gliding of W atoms.

Fig. 3: Structural characterization of WS2 during 2M to 2H phase transition.
figure 3

High-resolution transmission electron microscopy (HRTEM) images and the corresponding Fourier transforms of (a) 2M and (b) an intermediate phase WS2. c A zoomed-in view and Fourier transform of area [2] in (b). d Powder X-ray diffraction (XRD) patterns of 2M, intermediate phases and 2H WS2. 2H and intermediate phases WS2 were obtained by heating 2M WS2 in the air at 250 °C for 20 min and at 130 °C for various times, respectively. e A zoomed-in view of the XRD patterns in the range of 13.5° ~ 16.0°. AFM images with height profiles and optical images (insets at top-right corners) of a piece of multilayered WS2 flake in (f) 2M, (g) an intermediate and (h) 2H phases. Edge of the WS2 flake is denoted by white arrow, where interlayer sliding can be seen. i Raman spectra of an intermediate phase multilayered WS2 flake measured from the surface layers (black line) and the exposed inner layers (red lines) after punching with a tungsten probe. Insets show the corresponding optical images of the pristine WS2 flake and the one after punching (left) and Raman \({E}_{2{{\mbox{g}}}}^{1}\) mode intensity mapping of the probe-punched WS2 flake (right). Raman-measured areas are defined by squares.

Full size image

To investigate layer stacking configurations of intermediate phases WS2, AFM images are taken for different phases WS2. It is observed that the thickness of a WS2 flake (Fig. 3d–f) increases from 25.461 nm to 25.967 nm and 27.074 nm as it changes from 2M to an intermediate and 2H phase, revealing the continuous change of interlayer spacing. Moreover, there is slight sliding at edge of the WS2 flake from 2M to the intermediate and 2H phase (Fig. 3d–f and Supplementary Fig. 12), indicating generation of interlayer dislocation during the phase change. Since transforming from a 1T′ to 1H layer results in in-plane lattice contraction, the interlayer dislocation can be attributed to non-concerted 1T′ to 1H phase transition among different layers and the followed reconfiguration of layer stacking. XRD has been carried out on bulk 2M WS2 powder during heating at 130 °C. With prolongation of heating time, XRD peaks attributed to 2M (200) diffractions are weakening and broadening, while the ones attributed to 2H (002) diffractions are intensifying and sharpening, and all these peaks are shifting to lower degrees but locating in between that of standard 2M (200) and 2H (002) diffractions, as shown in Fig. 3g, h. These results indicate ordered stacks of 1T′ and 1H layers are broken and built, respectively, with increase of phase change extent. An intermediate phase WS2 has hybrid low-order stacks of 1T′ and 1H layers with average interlayer spacing in between that of pure 2M and 2H phase. In agreement with this result, Raman spectra (Supplementary Fig. 10) of intermediate phases WS2 manifests that A1g mode (ascribed to out-of-plane vibration of 1H layer stacks) locates at lower wave number compared to 2H phase and keeps to blue-shift as the extent of 2M to 2H phase change increases. High-index XRD diffractions including 2M \((11\bar{1})\) (002) \((31\bar{1})\) (601) (311) are also disappearing with \((11\bar{1})\) diffraction shifting to higher degrees as heating time increases, as shown in Supplementary Fig. 11. This can be attributed to in-plane gliding and out-of-plane displacements of W and S atoms during the 2M to 2H phase transition process. The above XRD, Raman and AFM results hint that 2M to 2H phase transition of multilayered WS2 probably takes place layer(s) by layer(s). To further confirm this mechanism, we tested Raman spectra on an intermediate phase multilayered WS2 flake before and after punching with a tungsten probe. As shown in Fig. 3i, the exposed inner layers after punching (areas marked by red squares) exhibit significantly higher intensities of 2H A1g and \({E}_{2{{\mbox{g}}}}^{1}\) modes than top layers of the WS2 flake, which strongly evidences non-concerted phase transition among different layers.

Thickness-dependent thermal properties of 2M WS2

As the 2M to 2H phase change of WS2 occurs along with dissociations of W–W bonds and reconstructions of W–S bonds, strengths of these intralayer chemical bonds play a critical role in phase stability of 2M WS2. Characteristic vibration frequency (\(\sigma\)) of a chemical bond is in proportion to square root of the bond force constant (\(k\)), according to \(\sigma=({N}_{A}^{0.5}/2\pi ){(k/M)}^{0.5}\), where NA is Avogadro’s constant, and M is average atomic mass. Therefore, frequencies of Raman vibration modes of 2M WS2 manifest strengths of the intralayer chemical bonds. Figure 4a shows Raman spectra of 2M WS2 flakes with various thicknesses. All samples exhibit the same Raman pattern, indicating the exfoliated few-layered 2M WS2 flakes maintain the same distorted octahedral coordination as that of bulk one. Previous theoretical calculation has demonstrated 2M WS2 has nine active Raman vibration modes, including one Bg mode, one Bu mode and seven Ag modes (\({A}_{{{\mbox{g}}}}^{6}\) at 327.5 cm−1 is not detectable in our results), as denoted in Fig. 4a3. Interestingly, Bg, \({A}_{{{\mbox{g}}}}^{1}\) and Bu modes are found to distinctly stiffen (blue shift) with decrease of thickness. As shown in Fig. 4b, from 6L to ML 1T′ WS2, Bg mode shifts from 110.9 to 121.1 cm−1, with \({A}_{{{\mbox{g}}}}^{1}\) mode shifting from 117.1 to 125.5 cm−1 and Bu mode shifting from 177.2 to 184.7 cm−1. For flakes of 6 or more layers, the frequencies of these modes converge to the bulk values. Other Raman modes of ML 1T′ WS2 were also reported to blue shift compared with that of bulk 2M one3, but we are not able to see these shifts in our results, probably because the shifts are too small to be observed. As shown in Fig. 4c, Bg mode corresponds to in-plane gliding of W and S atoms, while \({A}_{{{\mbox{g}}}}^{1}\) mode corresponds to distortion of W‒S bonds and Bu mode corresponds to stretching W–W bonds, along with out-of-plane displacements of S atoms3. Stiffening of Raman vibration modes with decrease of layer number indicates increases in W–W and W‒S bond force constants, leading to more rigid S–W–S lattices in thinner 2M WS2. This accounts for the thickness-dependent stability of 2M WS2. It should be noted that the high stability of ultrathin 2M WS2 cannot be ascribed to substrate effect. The same trends of thickness-dependent Raman mode frequencies and phase stability of 2M WS2 are also observed when the substrate is changed from Si/SiO2 to polydimethylsiloxane (PDMS) that has minimum interaction with TMD flakes, as shown in Supplementary Fig. 13.

Fig. 4: Thickness-dependent intralayer bond strength and thermal conductance of 2M WS2 on Si/SiO2 substrate.
figure 4

a Raman spectra of exfoliated thin (1 ~ 6L) and bulk 2M WS2. The observed active Raman modes are labeled beside the corresponding peaks. b Frequencies of Bg, \({A}_{{{\mbox{g}}}}^{1}\), Bu and \({A}_{{{\mbox{g}}}}^{7}\) Raman modes and the difference between frequencies of Bu and \({A}_{{{\mbox{g}}}}^{7}\) modes (red dotted line) as a function of layer thickness of 2M WS2. c Atomic displacements of the Bg, \({A}_{{{\mbox{g}}}}^{1}\) and Bu Raman modes. Bg mode is viewed in the bc plane, while \({A}_{{{\mbox{g}}}}^{1}\) and Bu modes are viewed in the ac plane. d \({A}_{{{\mbox{g}}}}^{4}\) and \({A}_{{{\mbox{g}}}}^{5}\) Raman peaks of exfoliated thin (1–6L) and bulk 2M WS2. e Raman spectra of a 3L 2M WS2 acquired with different power laser excitation. Frequency of Bu mode as functions of f laser power and g temperature for 3L, 8L and 22L 2M WS2. Laser-power coefficient (\({\chi }_{{{\mbox{P}}}}\)) and temperature coefficient (\({\chi }_{{{\mbox{T}}}}\)) of Bu mode frequencies are extracted from slopes of the corresponding plots. h Thermal conductivities of 3L, 8L and 22L 2M WS2 estimated by \({\chi }_{{{\mbox{T}}}}\) divided by \({\chi }_{{{\mbox{P}}}}\). For each sample, an average thermal conductance is obtained based on three values calculated from three sets of \({\chi }_{{{\mbox{T}}}}\) and \({\chi }_{{{\mbox{P}}}}\) data that are extracted from Bu mode plots in (f, g) and \({A}_{{{\mbox{g}}}}^{5}\) and \({A}_{{{\mbox{g}}}}^{7}\) modes plots in Supplementary Figs. 14, 15.

Full size image

Additionally, the different thickness dependence of different Raman modes allows Raman frequency to be an indicator of the layer thickness. We have depicted the difference (Δω) between frequencies of Bu and \({A}_{{{\mbox{g}}}}^{7}\) modes in Fig. 4b. For ML WS2, Δω is 220.5 cm−1, which is smaller than the value of 221.5 cm−1 for 2L and 224.5 cm−1 for 3L WS2. From ML to bulk WS2, the Δω increases from 220.5 cm−1 to 229.2 cm−1. This statistic of Raman mode frequencies provides a powerful tool for rapidly identifying thickness of 2M TMD, similar to that used for analyzing thickness of 2H TMD28. Another useful measure is the \({A}_{{{\mbox{g}}}}^{4}\) mode that turns to be detectable when 2M WS2 is thinned to 4L as shown in Fig. 4d. \({A}_{{{\mbox{g}}}}^{4}\) mode has an intensity comparable with \({A}_{{{\mbox{g}}}}^{5}\) mode in 3L 2M WS2 and exhibits higher intensity than \({A}_{{{\mbox{g}}}}^{5}\) mode in 2L 2M WS2. Moreover, \({A}_{{{\mbox{g}}}}^{4}\) intensity is two times that of \({A}_{{{\mbox{g}}}}^{5}\) mode in ML 1T′ WS2 (Fig. 4d). The enhancing of \({A}_{{{\mbox{g}}}}^{4}\) mode with decreasing of layer number probably associates with change of vibration symmetries in ultrathin WS22.

As 2M to 2H phase transition of multilayered WS2 is probably a layer(s)-by-layer(s) slow process, thermal conductance plays an important role in phase stability of 2M WS2. We then evaluate the thermal conductivities of 2M WS2 flakes on SiO2/Si substrates using temperature- and laser-power-dependent Raman spectroscopy43. With increase of temperature or laser power, the Raman vibration modes of all WS2 flakes with various thicknesses are observed to soften (red shift), as shown in Fig. 4e and Supplementary Fig. 14. We have selected Bu, \({A}_{{{\mbox{g}}}}^{5}\) and \({A}_{{{\mbox{g}}}}^{7}\) modes to plot the peak frequency as functions of temperature or laser power, as shown in Fig. 4f, g and Supplementary Fig. 15a–d. The extracted temperature coefficients (\({\chi }_{{{\mbox{T}}}}\)) of peak frequencies for different thicknesses are similar, in a range of 0.030–0.060 cm−1/K. However, the extracted laser-power coefficients (\({\chi }_{{{\mbox{P}}}}\)) of peak frequencies exhibit obviously thickness dependence, as shown in Fig. 4f and Supplementary Fig. 15c, d. The \({\chi }_{{{\mbox{P}}}}\) of Bu mode for 3L, 8L and 22L 2M WS2 are 0.585, 1.085, and 1.596 cm−1/mW, respectively. Thermal conductance can be estimated by \({\chi }_{{{\mbox{T}}}}\) divided by \({\chi }_{{{\mbox{P}}}}\)33,34,43,44. Accordingly, the thermal conductance of 3L, 8L, and 22L 2M WS2 are calculated to be 0.092, 0.044, and 0.023 mW/K (Fig. 4h), respectively, which clearly evidences thinner sample has higher thermal conductance. To deconvolute thermal conductance of the SiO2/Si substrate from our measurements, we also tested Raman spectra of WS2 at holey substrates (Supplementary Fig. 16). The as-obtained results also confirm thinner 2M WS2 has higher thermal conductance, as shown in Supplementary Fig. 16, except that the calculated thermal conductivities at holey substrates are lower than the corresponding values at conventional SiO2/Si substrates. Such reduction of thermal conductance with increase of thickness can be attributed to stronger phonon scattering and enhanced anharmonicity in thicker 2M WS231. The high thermal conductance of ultrathin 2M WS2 can also be connected with the enhanced intralayer bonding that facilitates intralayer heat conduction. High thermal conductance enables high-efficiency heat dissipation during heating, leading to improved tolerance to elevated temperature for thinner 2M WS2.

The different thermal conductivities between thick and thin WS2 flakes can also be associated with their distinct phase distributions during the 2M to 2H phase change (Supplementary Fig. 17). Low thermal conductance of a thick 2M WS2 flake results in nonuniform heat distribution during continuous heating process, and the 2M to 2H phase change occurs first at regions where heat is concentrated. Moreover, the non-concerted structural evolution among different layers can contribute to high interlayer phonon scattering and cause further low thermal conductance for the intermediate phase WS2. Consequently, a sequential phase change at different regions leads to a 2M/2H stripes pattern on the WS2 flake (Supplementary Fig. 17). In contrast, high thermal conductance of an ultrathin 2M WS2 flake has uniform heat distribution, so the phase change homogeneously occurs at entire region of the flake when phase change temperature is reached (Supplementary Fig. 17).

Phase transition simulation of different thicknesses WS2

To study thickness-dependent phase transition kinetics, we have simulated the 2M to 2H phase change processes for 1L, 2L, and 3L WS2 supercells (as shown in supplementary Fig. 18) by employing Vienna ab initio simulation package (VASP) and calculated energies of WS2 lattices with different configurations by using climbing image nudged elastic band (CI-NEB) method. Like the transition state theory applied for chemical reactions, these calculations can find the transition state WS2 lattice configuration during phase transition and identify the path passing through the transition state and connecting the initial and the final phase states. Such a path consists of a series of phase transition coordinates, including initial phase state (composed by 1T′ layers), final phase state (composed by 1H layers), transition state, and other intermediate lattice configurations. Method and principle used to find these coordinates are discussed in Part III of supporting information with Supplementary Fig. 19. The calculation output electronic files are attached as Supplementary Software 1. Configurations of all WS2 lattices at the as-calculated coordinates (coord. I to coord. VII) are shown in Supplementary Figs. 20–26. During the simulated phase transition process, the octahedral coordinated lattices gradually deform until reach the transition states, which further evolve and relax to the trigonal prismatic coordinated lattices. Meanwhile, the WS2 unit cell shrinks in a and b directions (Supplementary Fig. 27), which agrees to the afore-discussed phase transition mechanism.

During lattice deformation process of 1L 1T′ WS2, W-atom line distance d1 increases from 2.280 Å (coord. I) to 2.317 Å (coord. III), while W-atom line distance d2 decreases from 3.340 Å (coord. I) to 3.342 Å (coord. III), as shown in Fig. 5a and Supplementary Fig. 20. These results reveal W-atom lines distances tend to be equalized along b direction, in consistency with observations from TEM images (Fig. 5a–c) of WS2 during 2M to 2H phase transition. With changing of W-atom line distances, S1 and S2 atoms are found to glide along bc plane by large ranges from coord. I to III, rendering severe elongation of W2‒S1 bonds to 2.558 Å and breakage of W4‒S2 bonds (Fig. 5a). Meanwhile, the W1‒S1 bond elongates from 2.404 Å (coord. I) to 2.517 Å (coord. III), while W3‒S1 shortens from 2.438 Å (coord. I) to 2.319 Å (coord. III) and W3‒S2 and W5‒S2 bonds also shorten in coord. III. Similarly, W4‒S3 and W4‒S4 bond lengths are found to respectively increase and decrease from coord. I to III. Altering of W‒S bonds lengths also results in out-of-plane displacements of S atoms, which makes distance between different S atomic planes reducing from 0.418 Å at coord. I to 0.273 Å at coord. III (Fig. 5b). The 1L WS2 supercell reaches transition state at coord. III, with the highest lattice energy due to the significant lattice deformation. With further atomic gliding and reforming of W‒S bonds, the lattice of 1L WS2 turns to 1H type but with stretched W–S bonds in coord. IV (Fig. 5a). After further shortening of W–S bonds in the following three steps (Supplementary Fig. 20), the lattice of 1L WS2 finally relaxes to a typical 1H structure in coord. VII (Fig. 5a).

Fig. 5: Simulation of 2M to 2H phase transition of WS2 using rectangular supercells.
figure 5

Representative molecular geometries of the 1L WS2 supercell in the initial 1T′ phase (coord. I), transition state (coord. III), an intermediate configuration (coord. IV) and the final 1H phase (coord. VII), viewing from (a) the c direction and (b) the a direction. Process from coord. I to coord. III corresponds to deformation of 1T′-type lattice and process from coord. IV to coord. VII corresponds to relaxation of 1H-type lattice. c Molecular geometries of the 2L WS2 supercell at the initial phase state (coord. I), transition state (coord. IV), an intermediate configuration (coord. V) and the final phase state (coord. VII), viewing from the a direction. The highly deformed layer at the transition state is marked by a red dotted square. The layer turned from 1T′ to 1H type of structures is marked by a blue dotted square. Interlayer spacings are denoted in different states, showing interlayer expansion from coord. I to coord. VII. Lattice edges are depicted in different states, referring to which interlayer dislocation between top and bottom layers can be seen. Color code: blue and orange spheres represent W and S, respectively.

Full size image

The simulations of 2L and 3L WS2 supercells reveal layer-by-layer phase transition mechanism, in good agreement with the XRD and Raman spectra of intermediate phases WS2 (Fig. 3g–i). For 2L WS2, the top layer changes from the initial 1T′ structure (coord. I) to a deformed structure at coord. IV, where the transition state is reached, and turns to 1H-type structure at coord. V that then relaxes to coord. VII. The bottom layer is gradually distorted from the initial 1T′ structure until coord. IV and changes to 1H structure from coord. VI to VII, as shown in Fig. 5c and Supplementary Figs. 21, 22. Similarly, the bottom layer of 3L WS2 first transforms to 1H structure, followed by the mid layer and top layer, seen from Supplementary Figs. 23–26. 1T′/1H heterostructures are seen at coord. V for 2L (Fig. 5c) and 3L WS2 (Supplementary Fig. 26). At transition states, both 2L and 3L WS2 contain a highly deformed layer, while other layers are also distorted from the initial 1T’ phase, as shown in Supplementary Table 2. The deformed layers in 2L and 3L WS2 have larger structural variations from the initial 1T′ lattice compared to that in 1L WS2. The two W-atom line distances get closer in transition state 2L and 3L WS2 than 1L WS2 (Supplementary Table 2). In addition, the deformed layer in 3L WS2 have two broken W‒S bonds, while deformed layers in 1L and 2L WS2 include only one (Supplementary Table 2).

The total phase transition barrier of a WS2 supercell is defined as the energy difference between the corresponding transition and initial states. As thicker WS2 supercell involves larger structural difference between the corresponding transition and initial states, the total phase transition barrier increases from 1.83 eV to 1.95 eV and 2.10 eV, as the number of layers increases from 1L to 2L and 3L, as shown in Fig. 6a. After normalizing the total phase transition barriers by the number of layers, average transition barriers per layer of 1L, 2L, and 3L WS2 supercells are calculated to be 1.83 eV, 0.97 eV and 0.70 eV (Fig. 6b), respectively, exhibiting the average transition barrier decreases with the increase of layer number. This result theoretically confirms thinner 2M WS2 has higher phase stability. It should be noted that the average transition barrier per layer decreases more and more slowly as the number of layers increases from 1L to 2L and 3L (Fig. 6c), implying the average transition barriers per layer for thick WS2 flakes would converge to a certain value. This agrees to the fact that phase transition temperatures of WS2 flakes thicker than 5L converge to 120 °C (Fig. 1e).

Fig. 6: Calculated energy profiles of 2M to 2H phase transition.
figure 6

a Total energies and b average energies of 1L, 2L, and 3L WS2 supercells at the initial state (coord. I), transition states (coord. III for 1L WS2 and coord. IV for 2L and 3L WS2), final states (coord. VII) and other intermediate configurations. Solid lines are guides to the eye. Energies in (b) are normalized by the corresponding numbers of WS2 layers. Ea1L, Ea2L, and Ea3L are total phase transition energy barriers for the 1L, 2L, and 3L WS2 supercells, respectively. \({\bar{{{\mbox{Ea}}}}}^{1{{{{{\rm{L}}}}}}}\), \({\bar{{{\mbox{Ea}}}}}^{2{{{{{\rm{L}}}}}}}\) and \({\bar{{{\mbox{Ea}}}}}^{3{{{{{\rm{L}}}}}}}\) are average transition barriers per layer for the 1L, 2L, and 3L WS2 supercells, respectively. c The average transition barrier per layer as function of the number of layers. A dashed line is a guide to the eye.

Full size image

Discussion

The higher intralayer bond strength in thinner 2M WS2 can be associated with reduction of interlayer vdW interactions. As is known, higher stability of 1T′ TMD than the corresponding 1T TMD originates from the distorted lattice that generates strong intralayer metal–metal bonds and prominent intralayer Coulombic interactions between metal and chalcogen atoms4. An interlayer Coulombic interaction, such as vdW force, brings screening effect to the intralayer interactions and weaken the intralayer bond strength26,27,28. As the interlayer vdW interaction decreases with reduction of thickness from bulk to a few layers, the intralayer bond strength accordingly increases and reaches maximum in ML 1T′ TMD, meaning that ML 1T′ TMD has the highest intrinsic phase stability.

VdW interaction also plays an important role in the phase transition process of multilayered WS2. One the one hand, vdW-interaction linked layers tend to deform simultaneously during phase transition, resulting in increasing of total transition barrier with the number of layers. One the other hand, the existence of vdW interaction can constrain the freedom of lattice deformation, to minimize the layer stacking energy. Consequently, phase transition of multilayered WS2 occurs layer(s) by layer(s). In fact, WS2 lattices always tend to minimize the vdW-interaction-associated stacking energy by optimizing the stack configuration. Interlayer expansion and dislocation during phase transition have been demonstrated by the simulated molecular geometries (Fig. 5c and Supplementary Fig. 26) and physical characterizations (Fig. 3d–f and Supplementary Fig. 12) of multilayered WS2. For a relatively thick WS2 (≥5L), transition state lattice might include two or more highly deformed layers that are widely separated since vdW interactions between them are small. Once the number of deformed layers existing at the transition state turns to be proportionate to the total number of WS2 layers, the average phase transition barrier per layer will converge to a constant value for thick and bulk WS2 flakes.

In summary, we have demonstrated ultrathin 2M WS2 has significantly higher thermal stabilities than the bulk counterparts. Both phase transition temperature and durability of 2M WS2 remarkably increase with thickness decreasing from bulk to ML. By analyzing 2M to 2H phase transition mechanism and Raman spectra of 2M WS2 with different thicknesses, the higher stability of thinner 2M WS2 is associated with stiffened intralayer bonds and enhanced thermal conductance, due to reduction of interlayer interactions. Theoretical calculation indicates average transition barrier increases with reduction of WS2 layer number, since the phase transition occurs through a layer(s)-by-layer(s) mechanism. The high intrinsic phase stabilities of ultrathin 2M TMDs can inspire their tempting applications in various fields, including superconductor, electronics and energy conversion and storage.

Methods

Fabrication of different layered 2M WS2

K0.7WS2 crystals were synthesized as previously reported7. Specifically, K2S2 (prepared via liquid ammonia), W (99.9%, Alfa Aesar), and S (99.99%, Alfa Aesar) were mixed by the stoichiometric ratios and ground in an argon-filled glovebox. The mixtures were pressed into a pellet and sealed in the evacuated quartz tube. The tube was heated at 850 °C for 2000 min and slowly cooled to 550 °C at a rate of 0.1 °C min−1. 2M WS2 single crystals were obtained by oxidizing K0.7WS2 in an aqueous solution containing 0.01 M K2Cr2O7 and 0.02M H2SO4 at room temperature for 1 h. Different layered WS2 flakes were mechanically exfoliated from the synthesized bulk 2M WS2 onto a SiO2/Si substrate or a holey SiO2/Si substrate. To transfer the exfoliated WS2 from SiO2/Si to PDMS, the SiO2/Si substrate was spin-coated with PMMA film and etched by KOH solution. A piece of PDMS film was used to pick up the WS2/PMMA and the PMMA film was removed by soaking in acetone for an hour. More details about sample preparation are available in Supplementary Note 1.

Temperature- and laser-power-dependent Raman measurements

Micro-Raman spectra were taken on the 2M WS2 using a confocal microscope Raman system (WITec, Alpha300R) with an optical microscope (Nikon). For temperature-dependent Raman measurement, the exfoliated 2M WS2 on a Si/SiO2 or holey Si/SiO2 or PDMS substrate was put on a hot plate, and temperature was increased by 5 °C and held for 1 min or 15 min in each heating step. The heating treatment was carried out either in the air or in a glovebox with an Ar atmosphere. After heating, the sample was transferred to the Raman instrument for Raman spectra acquisition. For laser-power-dependent Raman measurement, the powers of laser irradiating on the WS2 flakes were quantified by a power meter (Thorlabs). More details about Raman measurements are available in Supplementary Note 2.

Physical characterizations

The morphology and microstructure of the 2M WS2 flakes were transferred to microgrid and examined by transmission electron microscopy (TEM, FEI Verios G4). The thickness and morphology were analyzed by using AFM images, which were captured with a Bruker Dimension Icon AFM under tapping mode. The samples were characterized by XRD pattern (Bruker D8 Advanced).

Computational method

All the calculations were performed by the employee of the VASP45. The Perdew–Burke–Ernzerhof form of the generalized gradient approximation was employed to describe electronic exchange and correlation46. Lattice constants and atom positions of WS2 in 2H and 2M phases, respectively, were optimized by using the conjugate gradient algorithm until the maximum force on a single atom is less than 0.02 eV/Å. The thickness of the vacuum layer exceeded 15 Å to eliminate the fictitious interaction caused by periodic cells. The van der Waals interactions were depicted in terms of the D3 method of Grimme47. The CI-NEB method was adopted to locate the transition state from 2H to 2M48. More details about computational methods are available in Supplementary Note 3.