Abstract
Despite their technological relevance, a full microscopic understanding of glasses is still lacking. This applies even more to their surfaces whose properties largely differ from that of the bulk material. Here, we experimentally investigate the surface of a two-dimensional glass as a function of the effective temperature. To yield a free surface, we use an attractive colloidal suspension of micron-sized particles interacting via tunable critical Casimir forces. Similar to crystals, we observe surface melting of the glass, i.e., the formation of a liquid film at the surface well below the glass temperature. Underneath, however, we find an unexpected region with bulk density but much faster particle dynamics. It results from connected clusters of highly mobile particles which are formed near the surface and deeply percolate into the underlying material. Because its thickness can reach several tens of particle diameters, this layer may elucidate the poorly understood properties of thin glassy films which find use in many technical applications.
Similar content being viewed by others
Introduction
Solids typically begin to melt far below their bulk melting temperature by the formation of a liquid layer at their surface1,2. Such surface melting which originally has been observed by Faraday in 1842 in noting a quasi-liquid layer on ice has been reported for many crystalline materials2,3,4,5. Unlike crystals where the presence of a fluid on top of an ordered solid is detected, e.g., by neutron or X-ray scattering experiments1,2,6, the demonstration of surface melting in glasses is more challenging due to the lack of appropriate order parameters distinguishing a glass from a liquid7,8,9,10,11,12. Although the transition of a liquid into a glass qualitatively differs from how a liquid turns into a crystal, surface melting is also predicted for amorphous materials13,14,15,16. Apart from basic scientific interest, surface melting of glassy systems is expected not only to influence its surface properties but may also explain the unusual behavior of thin polymeric and metallic glassy films whose reduced glass-transition temperature and strongly enhanced surface mobility is exploited in technical applications17,18,19,20,21,22. Despite considerable effort, however, the microscopic changes taking place near a glass surface during surface melting have not yet been resolved.
Here, we present real-space experiments of the surface melting of a two-dimensional (2D) colloidal glass where the motion of particles is fully resolved in space and time. We find that the glass melts starting from the surface by forming a broad transient region composed of a liquid and supercooled liquid in coexistence with an underlying bulk glass (BG). Surprisingly, adjacent to the BG, we observe a region with bulk density but a faster particle dynamics, the latter resulting from connected cooperative clusters of highly mobile particles which are formed at the surface and proliferate deep into the system. The thickness of this unexpected region varies non-monotonically with the effective temperature and becomes largest near the bulk glass transition point.
Results
To yield a quasi-equilibrated gas-solid interface in a 2D colloidal system, an attractive particle interaction is required. In our experiments this is achieved by critical Casimir forces which arise due to fluctuations of the solvent’s composition near its critical temperature Tc23. Upon variations of the temperature ΔT = Tc−T, one can control the attraction between colloids suspended in the critical mixture in a fully reversible manner24. Note that higher ΔT corresponds to a weaker attraction strength in our system, yielding a higher effective temperature. The solvent is an aqueous micellar solution of non-ionic surfactant C12E5 with a lower critical point at Tc ≈ 32 °C and 1.2% surfactant weight25,26. A binary mixture of silica particles (ratio 0.55:0.45) with diameters σs = 2.4 μm and σl = 3.34 μm was added to the solvent which was contained in a sample cell with 100 μm in height. Due to gravity the particles sediment towards the bottom of the cell where they form a disordered monolayer. The Debye screening length of the system is about 30 nm 26, leading to rather short-ranged particle repulsion. To create a free surface between a low density gaseous and a high density glass phase, the sample cell was first tilted by 1.15° leading to a lateral density gradient across the sample. During this step the temperature was kept at ΔT = 11 K where critical Casimir forces are negligible (Supplementary Fig. 1). Afterwards the sample was aligned horizontally with the temperature slowly (0.2 K/h) increased to ΔT = 2.5 K. As a result, a free and equilibrated surface perpendicular to the original tilting direction develops (Fig. 1). Starting from such conditions, we slowly varied the temperature to yield thermally equilibrated states at different ΔT. Prior to each measurement, samples were kept at the corresponding temperature for at least three hours. For further details regarding the sample equilibration, we refer to Supplementary Note 2.
a Snapshots of a glass surface at temperatures ΔT = 4.5 K. The color code represents the local Voronoi area. The dashed horizontal line indicates the location of \({z}_{{{{{{{{\rm{sat}}}}}}}}}^{\varphi }\) as defined in (f). The scale bar is 50 μm. The origin of the z-axis has been defined by \({z}_{{{{{{{{\rm{sat}}}}}}}}}^{\varphi }\) for 4.5 K. b–e Typical zoom-in snapshots of the glass surface area (see dashed rectangular area in (a)) at different temperatures: ΔT = 2.5 K (b), 3.5 K (c), 4.5 K (d), 5.5 K (e). The scale bar is 15 μm. f Depth-resolved particle area fraction for different ΔT near the saturation and over the entire z range (inset). Dashed lines indicate \({z}_{{{{{{{{\rm{sat}}}}}}}}}^{\varphi }(\Delta T)\) where the corresponding area fractions reach 99.5% of the corresponding φsat. To compare profiles with different temperatures, \({z}_{{{{{{{{\rm{sat}}}}}}}}}^{\varphi }(\Delta T=2.5\,{{{{{{{\rm{K}}}}}}}})\) was chosen as the origin of the z-axis.
Figure 1 a shows a typical snapshot for ΔT = 4.5 K following the above protocol. The particles are colored according to their Voronoi cell area, highlighting their local area fraction φ. From the top to the bottom (i.e. in the direction of the z-axis) we observe a smooth transition from a highly diluted gas phase (red) to a densely packed (blue) disordered state. Figure 1b–e shows enlarged snapshots of the dashed region in Fig. 1a for ΔT between 2.5 and 5.5 K (Supplementary Video 1). Due to the temperature-dependent critical Casimir attraction, the interface becomes increasingly broadened with increasing ΔT which hallmarks the surface melting (see area fraction profiles in Fig. 1f). In contrast to the strong temperature dependence near the surface, the profiles almost perfectly overlap at large z where they converge to φsat which only slightly (<5%) varies with ΔT. The depths \({z}_{{{{{{{{\rm{sat}}}}}}}}}^{\varphi }(\Delta T)\) where the profiles saturate are shown as vertical dashed lines in Fig. 1f. Since the properties of glasses strongly depend on their density, we have also determined the variance of the z-resolved local area fraction. Both quantities saturate at the same depth which confirms that \({z}_{{{{{{{{\rm{sat}}}}}}}}}^{\varphi }\) is a reliable measure for the depth below which the structural properties of the system become constant (Supplementary Note 3). Notably, we find a rather continuous increase of φ between the gas and the liquid, which is due to the averaging parallel to the rough surface of the disordered system.
To investigate how the material’s properties change with increasing distance to the surface, we evaluated the z-resolved mean-squared displacement (MSD) and intermediate scattering function Fs(q, t) (Supplementary Note 3). Exemplarily, this is shown for ΔT = 4.5 K in Fig. 2 but the same qualitative behavior is also found for the other temperatures considered in this work. Near the surface (φ < 0.2, particles shown in red in Fig. 1a) the dynamics is diffusive with the diffusion coefficient being almost identical to that of isolated particles. We note that small deviations from a strictly linear MSD are observed at larger times due to the attractive particle interaction which can lead to the temporary formation of small particle clusters. We refer to this region as a gas phase. With increasing z, the dynamics first remains diffusive but with a gradually decreasing diffusion coefficient (Fig. 2a). In this range, the corresponding Fs(q, t) rapidly decays to zero (Fig. 2b) suggesting a liquid layer. At even larger depths (i.e. larger φ) particle displacements require increasingly cooperative rearrangements, leading to a sub-diffusive behavior27. Such cooperative behavior is supported by the strong increase of the decay time of Fs(q, t) (supercooled liquid). At depths z > 35σs the MSD and Fs(q, t) exhibit a plateau-like structure which is characteristic when the dynamics of particles is dominated by the cages formed by their neighbors as being characteristic for bulk glasses28.
a Mean squared displacement (MSD) and (b) intermediate scattering function Fs(q, t) for different z values (in units of σs): −25, −15, −5, 5, 15, 25, 35, 45, 55, 65, 75, 85, 95 (in the direction of the arrow) where q = 1.45 μm−1 corresponds to the first peak in the structure factor at φsat. Each bin is averaged over a width of z ± 5σs. The data is taken at ΔT = 4.5 K. The black solid line corresponds to the slope equal to 1.
Since the particle area fraction gradually increases from the surface towards the bulk, the observation of a smooth transition (liquid - supercooled liquid–glass) may simply reflect the density dependence of the phase behavior of a disordered colloidal system. This, however, is not in agreement with our results. Opposed to the area fraction which saturates for ΔT = 4.5 K at z ≈ 18σs (Fig. 1f), pronounced variations in Fs(q, t) are clearly visible even below z ≈ 65σs (Fig. 2b). Such decoupling of the intermediate scattering function from the area fraction is not observed in bulk glasses and must therefore originate from the presence of the surface. We note, that a decoupling of static and dynamic properties at different depths has been also reported in numerical simulations of thin glassy films of attractive polymers29.
Because Fs(q, t) decays rather slowly at large depths, a quantitative analysis of its characteristic decay time is difficult on our experimental time scales. Therefore, we have also calculated the self-part of the overlap function qs(t, z) which measures how similar particle configurations remain after time t over distance z30,31,32. This quantity displays a similar behavior as Fs(q, t) but decays considerably faster. For a definition of the self-part of the overlap function we refer to Supplementary Note 3.
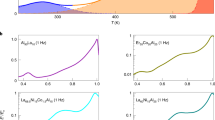
Figure 3a shows the temporal decay of qs(t) for increasing depth at a temperature ΔT = 4.5 K (qualitative similar results are observed over the entire temperature range considered in this work). We define the corresponding relaxation times τs(z) as the time to reach qs(t) = 0.4. As seen in Fig. 3b, τs(z) increases with z and eventually saturates at the temperature-dependent depth \({z}_{{{{{{\rm{sat}}}}}}}^{{\tau }_{s}}(\Delta T)\) which marks the transition towards the bulk glass. Similar to Fs(q, t), τs(z) only saturates considerably below the depth \({z}_{{{{{{{{\rm{sat}}}}}}}}}^{\varphi }\) where the area fraction becomes constant (Fig. 3c). In the following we are referring to the region \({z}_{{{{{{{{\rm{sat}}}}}}}}}^{\varphi }\le z\le {z}_{{{{{{{{\rm{sat}}}}}}}}}^{\tau }\) as a surface glassy layer (SGL). Remarkably, the thickness of the SGL, i.e., \({l}_{{{{{{{{\rm{SGL}}}}}}}}}={z}_{{{{{{{{\rm{sat}}}}}}}}}^{\varphi }-{z}_{{{{{{{{\rm{sat}}}}}}}}}^{\tau }\) varies non-monotonically as a function of the temperature with a maximum at ΔT ≈ 4.5 K (Fig. 3d). This maximum is found to be close to the bulk glass transition point (dashed vertical line in Fig. 3d and Supplementary Fig. 4d).
a Time-dependence of the overlap function for ΔT = 4.5 K for the following z values (in units of σs and in the direction of the arrow): −20, −15, −5, 0, 10, 20, 30, 45, 80. b Measured (symbols) and averaged (lines) values of the depth-dependent relaxation time τs(z) for different temperatures. The solid lines correspond to smoothed experimental data obtained from averaging over 10 data points, each. Vertical dashed lines denote \({z}_{{{{{{\rm{sat}}}}}}}^{{\tau }_{s}}\) where τs(z) saturates. c Temperature-dependence of \({z}_{{{{{{{{\rm{sat}}}}}}}}}^{\varphi }\) and \({z}_{{{{{{\rm{sat}}}}}}}^{{\tau }_{s}}\) with a surface glassy layer (SGL) in between. For \(z\ge {z}_{{{{{{\rm{sat}}}}}}}^{{\tau }_{s}}\) we observe a bulk glass (BG) while for \(z\le {z}_{{{{{{{{\rm{sat}}}}}}}}}^{\varphi }\) a gas/fluid state is found. Error bars correspond to the standard deviation of the corresponding mean values. d Thickness lSGL of the surface glassy layer as a function of the temperature with the transition point according to mode coupling theory shown as a vertical line. The error bars correspond to the total error due to the error bars shown in c.
To provide a microscopic understanding of the SGL, we have analyzed how particles with different mobility are distributed across the sample at different temperatures. Figure 4a–c shows the result for ΔT = 3.5, 4.5, and 5.5 K where the particles are color-coded according to their displacement Δr within 333 s (this timescale has been chosen since it is between the β- and the α-relaxation time of the bulk glass (Supplementary Note 5) and thus captures the cage-escape dynamics). As expected, highly mobile particles (red) are frequently found near the surface (gas, liquid) but they also proliferate by several tens of particle diameters below even beyond \({z}_{{{{{{{{\rm{sat}}}}}}}}}^{\varphi }\) (dashed horizontal lines) where the density area fraction saturates. To visualize the penetration depth of clusters comprised of fast particles in more detail, we highlighted in Fig. 4d–f connected clusters comprised of particles whose displacement Δr is larger than 0.3σs (this displacement roughly corresponds to the cage size near the glass transition temperature (Supplementary Fig. 4b). The time-dependence of such regions is shown in the Supplementary Video 2. Notably, the penetration depth of these clusters beyond \({z}_{{{{{{{{\rm{sat}}}}}}}}}^{\varphi }\) (horizontal dashed lines) exhibits a very similar non-monotonic temperature dependence as the thickness of the SGL (see Fig. 3d) which provides an important clue to understand its unusual properties.
a–c Typical snapshots with particles colored according to their displacements within 333 s (being much larger than the beta relaxation time τβ ( < 30 s) for three different temperatures (from left to right ΔT = 3.5 K (a), 4.5 K (b), 5.5 K (c)). The horizontal dashed and solid horizontal lines correspond to \({z}_{{{{{{{{\rm{sat}}}}}}}}}^{\varphi }\) and \({z}_{{{{{{\rm{sat}}}}}}}^{{\tau }_{s}}\), respectively. The scale bar is 35 μm. d–f Morphology of clusters comprised of connected particles whose displacement is larger than 0.3σs for ΔT = 3.5 K (d), 4.5 K (e), 5.5 K (f). Different (unconnected) clusters are shown in different colors. Dashed and solid horizontal lines correspond to \({z}_{{{{{{{{\rm{sat}}}}}}}}}^{\varphi }\) and \({z}_{{{{{{\rm{sat}}}}}}}^{{\tau }_{s}}\), respectively.
For a more quantitative support of a relationship between the SGL and clusters of highly mobile particles reaching down from the surface, we have analyzed the temperature-dependent distribution of the 10% fastest particles in the region \(z > {z}_{{{{{{\rm{sat}}}}}}}^{\varphi }\) (Supplementary Fig. 6 and Supplementary Video 3). They show a very similar behavior as seen in Fig. 4d–f in particular with regard to the non-monotonic temperature dependence of the extension below \({z}_{{{{{{\rm{sat}}}}}}}^{\varphi }\). From the decay of the measured z-dependent probability distribution of 10% fastest particles, we obtained their decay length which is in remarkable agreement with lSGL (Supplementary Fig. 6d, e). As shown in Supplementary Note 7, the 10% fastest particles form connected clusters and exhibit a cooperative dynamics, in resemblance to cooperative rearrangement regions (CRRs) in bulk glasses33,34. Unlike CRRs in bulk glasses whose size monotonically increases when approaching the glass transition point, however, in our experiments these clusters display a non-monotonic temperature dependence. This difference is due to the presence of the free surface which promotes the formation of fast particle clusters which then expand deep into the sample. At small ΔT, i.e. large particle attractions, the strongly reduced particle motility near the surface limits the formation of such clusters. On the other hand, at large ΔT the size of these clusters becomes smaller since dynamical correlations in glassy systems generally decrease with increasing effective temperature35,36,37. In combination, this leads to the non-monotonic dependence of lSGL as observed in our experiments.
Discussion
Our results demonstrate that surface melting of glasses is qualitatively different compared to crystals and leads to the formation of a surface glassy layer. This layer contains cooperative clusters of highly mobile particles which are formed at the surface and which proliferate deep into the material by several tens of particle diameters and well beyond the region where the particle density saturates. This might explain why the properties of thin glassy films considerably deviate from their corresponding bulk properties38,39,40. In addition, we found that the thickness of the surface glassy layer exhibits a non-monotonic dependence of the particle attraction, i.e. the effective temperature. Such behavior bears some interesting resemblance to recent observations of the non-monotonic properties of the dynamic correlation length ξdyn in atomic and colloidal glasses30,31,32, which is awaiting for further theoretical studies. Similar to ξdyn which is determined in the presence of a frozen interface and which characterizes the properties of the bulk glass30, the formation of a surface glassy layer during surface melting also reflects the properties of the underlying bulk material. Very recently, we became aware of another colloidal study which also observed that static and dynamic properties saturate at different depths near the surface of a glass41. Because in this study the attraction mechanism is different from ours, this strongly supports the idea the formation of surface glassy layers is expected to be a common phenomenon near the surface of glassy materials.
Data availability
The data that support our findings of this study are available from the corresponding author upon request.
References
Frenken, JoostW. M. & Van der Veen, J. F. Observation of surface melting. Phys. Rev. Lett. 54, 134 (1985).
Dash, J. G., Rempel, A. W. & Wettlaufer, J. S. The physics of premelted ice and its geophysical consequences. Rev. Mod. Phys. 78, 695 (2006).
Faraday, M. R. On certain conditions of freezing water. J. Frankl. Inst. 20, 283 (1850).
Slater, B. & Michaelides, A. Surface premelting of water ice. Nat. Rev. Chem. 3, 172–188 (2019).
Li, B. et al. Modes of surface premelting in colloidal crystals composed of attractive particles. Nature 531, 485–488 (2016).
Lied, A., Dosch, H. & Bilgram, J. H. Surface melting of ice ih single crystals revealed by glancing angle x-ray scattering. Phys. Rev. Lett. 72, 3554 (1994).
K., Binder & W., Kob, Glassy Materials and Disordered Solids: An Introduction to their Statistical Mechanics (World Scientific, 2011).
Kob, W. & Andersen, H. C. Testing mode-coupling theory for a supercooled binary lennard-jones mixture. ii. intermediate scattering function and dynamic susceptibility. Phys. Rev. E 52, 4134 (1995).
Berthier, L. & Biroli, G. Theoretical perspective on the glass transition and amorphous materials. Rev. Mod. Phys. 83, 587 (2011).
Weeks, E. R. Introduction to the colloidal glass transition. ACS Macro Lett. 6, 27–34 (2017).
Lu, P. J. & Weitz, D. A. Colloidal particles: crystals, glasses, and gels. Annu. Rev. Condens. Matter Phys. 4, 217–233 (2013).
Stillinger, F. H. & Debenedetti, P. G. Glass transition thermodynamics and kinetics. Annu. Rev. Condens. Matter Phys. 4, 263–285 (2013).
Tartaglino, U., Zykova-Timan, T., Ercolessi, F. & Tosatti, E. Melting and nonmelting of solid surfaces and nanosystems. Phys. Rep. 411, 291–321 (2005).
Van Hoang, V. & Quy Dong, T. Melting of monatomic glass with free surfaces. J. Chem. Phys. 136, 104506 (2012).
Jagla, E. A. & Tosatti, E. Surface defreezing of glasses. EPL (Europhys. Lett.) 51, 648 (2000).
Hoang, V. V. Melting of simple monatomic amorphous nanoparticles. J. Phys. Chem. C 116, 14728–14735 (2012).
Fakhraai, Z. & Forrest, J. A. Measuring the surface dynamics of glassy polymers. Science 319, 600–604 (2008).
Ediger, M. D. & Forrest, J. A. Dynamics near free surfaces and the glass transition in thin polymer films: a view to the future. Macromolecules 47, 471–478 (2014).
Cao, C. R., Lu, Y. M., Bai, H. Y. & Wang, W. H. High surface mobility and fast surface enhanced crystallization of metallic glass. Appl. Phys. Lett. 107, 141606 (2015).
Swallen, S. F., Traynor, K., McMahon, R. J., Ediger, M. D. & Mates, T. E. Stable glass transformation to supercooled liquid via surface-initiated growth front. Phys. Rev. Lett. 102, 065503 (2009).
Zhang, Y. & Fakhraai, Z. Decoupling of surface diffusion and relaxation dynamics of molecular glasses. Proc. Natl Acad. Sci. 114, 4915–4919 (2017).
Flenner, E., Berthier, L., Charbonneau, P. & Fullerton, C. J. Front-mediated melting of isotropic ultrastable glasses. Phys. Rev. Lett. 123, 175501 (2019).
Fisher, M. E. & Gennes, PierreG. D. Wall phenomena in a critical binary mixture. Comptes Rendus Hebd. Des. Seances De. L Academie Des. Sci. Ser. B 287, 207–209 (1978).
Hertlein, C., Helden, L., Gambassi, A., Dietrich, S. & Bechinger, C. Direct measurement of critical Casimir forces. Nature 451, 172–175 (2008).
Einaga, Y. Wormlike micelles of polyoxyethylene alkyl ethers CiEj. Polym. J. 41, 157–173 (2009).
Helden, L. et al. Critical Casimir interactions of colloids in micellar critical solutions. Soft Matter 17, 2737–2741 (2021).
Weeks, E. R. & Weitz, D. A. Subdiffusion and the cage effect studied near the colloidal glass transition. Chem. Phys. 284, 361–367 (2002).
Li, B., Lou, K., Kob, W. & Granick, S. Anatomy of cage formation in a two-dimensional glass-forming liquid. Nature 587, 225–229 (2020).
Illing, B. et al. Mermin–Wagner fluctuations in 2d amorphous solids. Proc. Natl Acad. Sci. 114, 1856–1861 (2017).
Kob, W., Roldán-Vargas, S. ándalo & Berthier, L. Non-monotonic temperature evolution of dynamic correlations in glass-forming liquids. Nat. Phys. 8, 164–167 (2012).
Nagamanasa, K. H., Gokhale, S., Sood, A. K. & Ganapathy, R. Direct measurements of growing amorphous order and non-monotonic dynamic correlations in a colloidal glass-former. Nat. Phys. 11, 403–408 (2015).
Ganapathi, D., Nagamanasa, K. H., Sood, A. K. & Ganapathy, R. Measurements of growing surface tension of amorphous–amorphous interfaces on approaching the colloidal glass transition. Nat. Commun. 9, 1–8 (2018).
Stevenson, J. D., Schmalian, J. örg & Wolynes, P. G. The shapes of cooperatively rearranging regions in glass-forming liquids. Nat. Phys. 2, 268–274 (2006).
Zhang, Z., Yunker, P. J., Habdas, P. & Yodh, A. G. Cooperative rearrangement regions and dynamical heterogeneities in colloidal glasses with attractive versus repulsive interactions. Phys. Rev. Lett. 107, 208303 (2011).
Bennemann, C., Donati, C., Baschnagel, J. örg & Glotzer, S. C. Growing range of correlated motion in a polymer melt on cooling towards the glass transition. Nature 399, 246–249 (1999).
Berthier, L. et al. Direct experimental evidence of a growing length scale accompanying the glass transition. Science 310, 1797–1800 (2005).
Donati, C., Glotzer, S. C. & Poole, P. H. Growing spatial correlations of particle displacements in a simulated liquid on cooling toward the glass transition. Phys. Rev. Lett. 82, 5064 (1999).
De Gennes, P. G. Glass transitions in thin polymer films. Eur. Phys. J. E 2, 201–205 (2000).
Yang, Z., Fujii, Y., Lee, FukKay, Lam, Chi-Hang & Tsui, OpheliaK. C. Glass transition dynamics and surface layer mobility in unentangled polystyrene films. Science 328, 1676–1679 (2010).
Torres, J. M., Stafford, C. M. & Vogt, B. D. Elastic modulus of amorphous polymer thin films: relationship to the glass transition temperature. Acs Nano 3, 2677–2685 (2009).
Zhang, Q., Li, W., Qiao, K. & Han, Y. Surface premelting and melting of colloidal glasses. https://doi.org/10.21203/rs.3.rs-1633832/v1.
Acknowledgements
The authors acknowledge stimulating discussions with Thomas Voigtman, Hailong Peng, Matthias Fuchs and Bo Li and financial support from the CRC1214 Anisotropic particles as building blocks, project B7 which is funded by the Deutsche Forschungsgemeinschaft.
Funding
Open Access funding enabled and organized by Projekt DEAL.
Author information
Authors and Affiliations
Contributions
C.B. and L.T. designed the research and discussed the results. L.T. carried out the experiments, analyzed the data and wrote the paper. C.B. supervised the project.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Peer review
Peer review information
Nature Communications thanks the anonymous reviewer(s) for their contribution to the peer review of this work.
Additional information
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Tian, L., Bechinger, C. Surface melting of a colloidal glass. Nat Commun 13, 6605 (2022). https://doi.org/10.1038/s41467-022-34317-2
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41467-022-34317-2
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.