Introduction

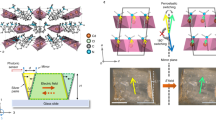
Various ferroelectric device types exist. However, the same basic mechanism occurs in all devices: spontaneous polarization (PS) changes under external stimuli and then converts mechanical to electrical energy, or vice versa; here, the polarization rotation, extension, and switch are critical1,2,3. Although ferroelectric materials differ, the core task for applications is to always make the above sequence of events possible, favorable, and stable, under both small and large drives4,5. Consequently, high piezoelectric response and a large coercive field (EC) are of fundamental importance, enabling both high operation efficiency and a wide operational field range in numerous electromechanical applications, such as high-power transducers and high-field actuators6,7. In the past 30 years, ultrahigh piezoelectric perovskites, relaxor-PbTiO3 (relaxor-PT) single crystals, have been discovered and greatly developed; they are the driving force for emerging electromechanical applications8. However, compositional modification for the simultaneous enhancement of piezoelectricity and EC is challenging. For example, inferior piezoelectricity d33 (~1100 pC/N) is afforded and consequently considerable degradation of electromechanical response occurs upon the hard doping by manganese (Mn) in Pb(Mg1/3Nb2/3)O3–PbTiO3 (PMN–PT) crystals9,10; further, EC with a low magnitude (~2.4 kV/cm), which is unsuitable for high-power and high-field applications, is afforded when soft doping strategies are employed11. Figure 1a summarizes the relation between the coercive field EC and piezoelectric coefficient d33 for various relaxor-PT ferroelectric crystals, demonstrating that high piezoelectric activity is generally associated with a low coercive field. Thus, while ultrahigh-piezoelectric relaxor-PT crystals are revolutionizing the electromechanical community, the crucial question to naturally arise is “is there a possibility to highly enhance the coercive field without sacrificing their ultrahigh piezoelectricity?”

Fig. 1: Dilemma in ferroelectrics: enhanced EC is usually achieved at the expense of piezoelectricity.
figure 1

a d33 vs. EC for various relaxor-PT single crystals. The red dashed line denotes the tendency of most relaxor-PT crystals. Generally, EC enhancement is accompanied by inferior piezoelectricity. Alternatively, our 0.06PSN–0.61PMN–0.33PT (red star) affords highly remarkable results. Data from refs. 9, 11, 17, 22, 31, 50,51,52,53,54,55,56,57,58,59,60,61,62,63,64,65 and this work. b Schematic of the different free energy landscapes and the corresponding macroscopic performances.

Full size image

Over the past decade, remarkable progress has been made toward achieving ultrahigh piezoelectricity in relaxor-PT by introducing an additional structural heterogeneity and a slush-like polar state to manipulate interfacial energies and/or expand the phase coexistence region12,13,14, which can further flatten the energy landscape and consequently favor an easy polarization rotation (Fig. 1b). However, a remarkable high EC has still not been achieved, which requires a high potential barrier to make the dipole switch difficult (Fig. 1b)15. For example, although recent studies have achieved piezoelectric activity of over 4000 pC/N in Sm-doped PMN–PT single crystals11, the crystals afford a low EC (~2.4 kV/cm). Furthermore, some studies demonstrated that EC of over 10 kV/cm can be achieved by doping relaxor-PT with Yb and Ho, but a weak piezoelectric response (~1100 pC/N) is inevitably afforded10,16. To date, to our knowledge, no study has reported a method for simultaneously achieving ultrahigh piezoelectricity and large EC for relaxor-PT crystals, and the fundamental mechanism of this issue is not yet fully understood.

Herein, the thermodynamics and microstructure of the relaxor-PT system are re-scrutinized. As shown in Fig. 1b, the piezoelectric activity and EC could be simultaneously improved by making the potential wells flatter and enhancing the barrier between adjunct polar states; the former could be realized via nanoscale inhomogeneity and the latter is usually correlated to large tetragonality in the perovskite lattice8. Thus, if a high-piezoelectric parent matrix comprises strongly tetragonal polar nano-regions (PNRs), large EC enhancement with improved piezoelectricity could be achieved. One can see an obvious difference exists in the lattice constants between PMN and another relaxor Pb(Sc1/2Nb1/2)O3 (PSN), (Supplementary Note 1 and Table S1), which is critical for creating a highly anisotropic microstructure with large tetragonality in a relaxor-PT system. Thus, we studied the effect of scandium (Sc) substitution for B-site cations in a model ultrahigh-piezoelectric relaxor-PT perovskite, PMN–PT. The resulting ternary 0.06PSN–0.61PMN–0.33PT crystals demonstrate excellent piezoelectric activity and electromechanical coupling response (d33 = 2630 pC/N, k33 = 90.8%; d15 = 490 pC/N, k15 = 54.7%), where the shear activity is twice that of the binary PMN–PT counterpart, and the ultrahigh longitudinal performance is maintained. Notably, EC was successfully improved by over three times to 8.2 kV/cm. Such an enhancement of the coercive field is the largest magnitude among all giant piezoelectric materials, far exceeding all experimentally observed results. Echoing to the proposed free energy landscape design, this work provid not only effective experimental routes but also vital theoretical guidelines for designing better ferroelectric materials.

Results

Materials properties

The composition selection of the PSN–PMN–PT solid solution is based on two considerations. First, the morphotropic phase boundary (MPB) compositions are definitely required to optimize the piezoelectric properties8,17. From the composition-dependent phase diagram of binary PMN–PT and PSN–PT, we deduced the MPB regions of the ternary PSN–PMN–PT system, as shown in the blue region in Fig. 2a. Additionally, a low Sc content doping strategy was employed in the crystal design because the piezoelectric activity of PSN–PT is significantly weaker than that of PMN–PT18,19. Subsequently, high-quality crack-free 0.06PSN–(0.94-x)PMN–xPT (x = 0.31-0.35) crystals with a diameter of 25 mm were successfully grown. Figure 2b displays the photograph of the as-grown crystals. All samples were cut from the same thin crystal wafer with identified PT contents and then poled along [001]C for domain-engineered configurations. The quantitative compositions of the samples were demonstrated to be 0.06PSN–0.61PMN–0.33PT via energy dispersive spectrometry, satisfying the material design requirements.

Fig. 2: Functional characterizations of PSN–PMN–PT single crystals.
figure 2

a Phase diagram of the PSN–PMN–PT ternary system. The blue area indicates the MPB region of the PSN–PMN–PT system. The orange point indicates the composition of our sample, 0.06PSN–0.61PMN–0.33PT, which is intensively investigated herein. b Photograph of the as-grown PSN–PMN–PT crystals, showing a large dimension of Φ25 × 35 mm3. c P–E loop of 0.06PSN–0.61PMN–0.33PT in comparison with that of 0.67PMN–0.33PT. d Temperature dependence of the relativity dielectric permittivity of the two crystals. 0.06PSN–0.61PMN–0.33PT exhibits a comparable Curie temperature TC but a markedly improved TF–F than 0.67PMN–0.33PT. e Shear piezoelectric performance of various [001]C-poled relaxor-PT crystals with MPB composition, demonstrating that 0.06PSN–0.61PMN–0.33PT exhibits significantly superior d15 and k15. f Comparison of the essential parameters for various [001]C-oriented PbTiO3-based relaxor ferroelectric single crystals with MPB composition. The 0.06PSN–0.61PMN–0.33PT crystals cover the largest area, denoting a superior overall performance. Data from refs. 22, 66, 67, and this work.

Full size image

Compared to their PMN–PT binary counterpart, 0.06PSN–0.61PMN–0.33PT crystals exhibit high longitudinal piezoelectric activity as well as obviously higher shear piezoelectric activity, higher ferroelectric–ferroelectric phase transition temperature TF–F, and a larger coercive field EC. As shown in Fig. 2c, the polarization–electric hysteresis loop (P–E loop) indicates that the 0.67PMN–0.33PT crystals afford a low EC of 2.6 kV/cm, severely hampering its potential applications. Notably, after a little Sc substitution, the coercive field of the resulting 0.06PSN–0.61PMN–0.33PT crystals is improved to 8.2 kV/cm, which is three times larger than that of PMN–PT. To the best of our knowledge, such an enhancement represents the most advanced enhancement reported to date for almost all investigated ferroelectrics with ultrahigh piezoelectric coefficients of over 2000 pC/N. To verify the repeatability of the extraordinary EC values, we analyzed several different samples; all samples afforded EC values of around 8 kV/cm (Fig. S1). Note that EC is not an intrinsic property of ferroelectrics20,21. Poling/de-poling is related to voltage-induced domain switching, including nucleation of new domains at a defect site (normally near domain walls) and domain growth. Thus, we considered the dynamics of domain switching by measuring the EC at various frequencies. As is well known, domain switching is considerably easy at low frequencies, which is related to domain switching under a very low field and long holding time of the applied fields. For the 0.06PSN–0.61PMN–0.33PT crystals, a large EC of ~7.5 kV/cm is maintained at frequencies as low as 0.1 Hz, and 11.8 kV/cm is afforded at 100 Hz (Supplementary Note 2 and Fig. S2). Moreover, we observed a low conductive current during the entire poling process, confirming the high quality of the crystals (Supplementary Note 3 and Fig. S3).

The TF-F observed in 0.06PSN–0.61PMN–0.33PT crystals is particularly interesting, ~85 °C, which are 13 °C higher than that observed for the PMN–PT crystals with similar PT contents (Fig. 2d), promising for a wide temperature usage range and drive field stability. Figure S4 displays the temperature dependence of the piezoelectric response of the 0.06PSN–0.61PMN–0.33PT crystals, exhibiting a variation of 140% in d33, which is considerably lower than that of PMN–PT crystals (200–300%)8. Furthermore, a relatively high EC of over 6.2 kV/cm can be maintained till the occurrence of the phase transition (Supplementary Note 4 and Fig. S5).

Remarkably, the piezoelectric coefficients d15 and electromechanical coupling constants k15 of the 0.06PSN–0.61PMN–0.33PT crystals were found to be 490 pC/N and 54.7%, respectively (Fig. 2e), featuring a significantly larger shear piezoelectric response than those of other [001]C-poled relaxor-PT systems. Moreover, 0.06PSN–0.61PMN–0.33PT crystals exhibit almost the same ultrahigh longitudinal property (d33 = 2630 pC/N) as the 0.67PMN–0.33PT crystals22. We also conducted detailed piezoelectric force microscopy (PFM) characterizations to investigate the polarization switching behavior and the local piezoelectric deformations of the PSN–PMN–PT crystals; the results further support their superior piezoelectric response from a microscopic perspective (Supplementary Note 5 and Fig. S6). Below TF–F, no obvious changes were observed in the domain morphology with increasing temperature (Fig. S7), which well agrees with the weak variations of the piezoelectric response and electromechanical properties, namely, a relatively strong thermal stability of functionality (Fig. S4). Figure 2f presents a radar chart summarizing the critical properties, including d33, k33, d15, k15, EC, and TF–F of various [001]C-poled relaxor-PT crystals with MPB compositions. The figure shows that the 0.06PSN–0.61PMN–0.33PT crystals cover an extremely large area, thereby demonstrating their superior overall performance and greater efficiency for potential device applications20,21,22.

Relaxor behavior

A key feature of relaxor-ferroelectric solid solutions is the existence of local heterogeneity, such as PNRs, which contribute over 50% to the dielectric/piezoelectric response according to the recent cryogenic experimental measurements13,23. Therefore, we investigated the relaxor behavior of the ternary 0.06PSN–0.61PMN–0.33PT crystals and explored the possible differences from their binary 0.67PMN–0.33PT counterparts, with the aim to determine why 0.06PSN–0.61PMN–0.33PT crystals simultaneously exhibit ultrahigh piezoelectric activity and extremely large EC, which seems unusual in most ferroelectric solid solution systems, as summarized in Fig. 1a.

Figure 3a shows the temperature-dependent reciprocal of the dielectric response of the 0.06PSN–0.61PMN–0.33PT and 0.67PMN–0.33PT crystals, indicating that the phase transitions proceed gradually rather than sharply with temperature. Such a diffuseness characteristic is a relaxor feature, causing the deviation from the Curie–Weiss law, where the Burns temperatures (TB) of around 268 °C can be derived for 0.06PSN–0.61PMN–0.33PT, 40 °C higher than that of 0.67PMN–0.33PT (228 °C). Thus, we reasonably deduce that during paraelectric-to-ferroelectric transitions, PNRs appear earlier (at a higher temperature) in 0.06PSN–0.61PMN–0.33PT than in 0.67PMN–0.33PT, presenting polarized precursor clusters. Such a diffused characteristic is further supported by quantitative analysis via the modified Curie–Weiss law24:

$$\frac{1}{\varepsilon }-\frac{1}{{\varepsilon }_{{\rm {m}}}}=\frac{{(T-{T}_{{\rm {m}}})}^{\gamma }}{C}$$
(1)

where εm is the maximum dielectric constant at Tm, C is the Curie-like constant, and γ describes the degree of diffuseness. Linear fitting of ln(1/ε33–1/εm) versus ln(T–Tm) data yields γ values of 1.96 and 1.74 for 0.06PSN–0.61PMN–0.33PT and 0.67PMN–0.33PT, respectively (Fig. 3b), suggesting a stronger relaxor nature in the ternary system. Further autocorrelation function analysis based on PFM characterizations demonstrates that it is much more difficult for the ternary crystal to establish a homogeneous polarization long-range order between neighboring clusters (Supplementary Note 6 and Figs. S8 and S9).

Fig. 3: Relaxor behavior.
figure 3

a Temperature dependence of the reciprocal of dielectric permittivity, from which TB is obtained by fitting with the modified Curie–Weiss law. b Modified Curie–Weiss law fitting results, from which γ can be obtained. c High-temperature dielectric property of 0.06PSN–0.61PMN–0.33PT crystals measured at various frequencies from 1 kHz to 1 MHz. d Vogel–Fulcher fitting results on the data shown in Fig. 3c and Fig. S7, from which the activation energy Ea and static freezing temperature TVF can be determined. e Summary of Ea for various pure PMN, PMN–PT, and PSN–PMN–PT. Data are from refs. 25, 68, 69. and this work. f TVF/Tm value as a function of frequency for 0.67PMN–0.33PT and 0.06PSN–0.61PMN–0.33PT.

Full size image

Figure 3c shows the frequency dependence of high-temperature dielectric properties of the ternary 0.06PSN–0.61PMN–0.33PT crystals, where the magnitude of the dielectric permittivity decreases and the dielectric maximum shifts to higher temperatures with increasing frequency, again demonstrating strong relaxor behavior. Notably, 0.06PSN–0.61PMN–0.33PT exhibits a “Tm shift” (shift of the dielectric maxima temperature with frequency over the range of 1 kHz–1 MHz) that is twice that of the 0.67PMN–0.33PT crystals (5 vs. 2.5 K, Fig. 3c and Fig. S10 in Supplementary Note 7), signifying strong interactions between PNRs and the development of local correlations23. Furthermore, the frequency dependence of the high-temperature dielectric data can be well fitted using the Vogel–Fulcher relation25:

$$f={f}_{0}\exp [-{E}_{\rm {{a}}}/({k}_{\rm {{B}}}({T}_{{{{{{\rm{m}}}}}}}-{T}_{{{{{{\rm{VF}}}}}}}))]$$
(2)

where f0 is the Debye frequency, Tm is the temperature of the permittivity maximum, and TVF is the static freezing temperature, which can be deemed as Tm at 0 Hz. TVF/Tm is a semi-quantitative parameter employed for evaluating PNR interactions25. Ea represents the activation energy of the polarization fluctuation in an isolated cluster that stems from the development of a short-range order; thus, a larger Ea suggests stronger interactions between neighboring PNRs. The fitted results are given in Fig. 3d, e, which show that the Ea of the 0.06PSN–0.61PMN–0.33PT crystals is considerably higher than that of 0.67PMN–0.33PT crystals (~0.024 vs. 0.008 eV), signifying stronger interactions among PNRs in the ternary crystals. Considering the higher TB, larger γ, shorter range order, higher Ea, and lower TVF/Tm (Fig. 3f), we reasonably deduce that 0.06PSN–0.61PMN–0.33PT exhibits more relaxor and diffused characteristics with much significantly stronger interactions between adjacent PNRs than 0.67PMN–0.33PT.

Highly dispersed local heterogeneous structure with considerable tetragonal phase

The strong polar cluster interaction is directly related to the structural instability and finally contributes to the material functionality26,27. This motivated us to further resolve the local microstructure of the 0.06PSN–0.61PMN–0.33PT crystals, and investigate its possible phase coexistence and complex crystallographic symmetry, which are crucial for understanding why PSN–PMN–PT crystals simultaneously afford ultrahigh possesses giant piezoelectricity and a high coercive field.

Figure 4a shows an aberration-corrected high-angle annular dark-field scanning transmission electric microscopy (HAADF-STEM) image. From the image we determined the polarization vector PS of each unit cell column based on the atomic positions. Note that a dispersed polar state with multiphase, including rhombohedral (R) and/or orthorhombic (O), tetragonal (T), and monoclinic (M), was detected. Moreover, 0.06PSN–0.61PMN–0.33PT exhibited considerably smaller (2–4 nm) than 0.67PMN–0.33PT (8–20 nm), suggesting a higher density of domain walls/phase interfaces and abundant local heterogeneous structure (Supplementary Note 8 and Figs. S11 and S12).

Fig. 4: Microstructure.
figure 4

a Atomic-resolution TEM images of the [001]C-oriented 0.06PSN–0.61PMN–0.33PT crystals, where the PS directions are given for each unit-cell column. The possible phase structures can be deduced using the PS directions (R rhombohedra, O orthorhombic, MA/MC monoclinic, and T tetragonal). b High-resolution XRD pattern and the optimal refinement results for 0.06PSN–0.61PMN–0.33PT. c Unit cell c/a ratios for 0.06PSN–0.61PMN–0.33PT and 0.67PMN–0.33PT derived from the TEM characterizations. d Local strain Sxx mapping extracted from the HAADF-STEM lattice image of 0.06PSN–0.61PMN–0.33PT in (a) and 0.67PMN–0.33PT in Fig. S9 via GPA, and the data along the white dotted lines are extracted and shown in (e). f Phase-field simulations of the domain structures of pure PMN–PT and that doped with tetragonal phase. PMN–PT exhibits pure R characteristics; after the introduction of the tetragonal phase, it exhibits multi-phase including R, O, and T characteristic with a reduced domain size. Different phases and various PS directions in the same phase are denoted by different colors.

Full size image

The abundant tetragonal phase in 0.06PSN–0.61PMN–0.33PT determined via HAADF-STEM is notable. This behavior was further evidenced in our high-resolution X-ray diffraction (XRD) characterizations, from which a detailed analysis of the peak positions and intensities was conducted and the volume fraction of the tetragonal component was estimated to be 34.5% (Fig. 4b), which is significantly higher than that of 0.67PMN–0.33PT, 13.7% (Supplementary Note 9, Table S2 and Fig. S13). Electric-field- and temperature-dependent structural evolutions were also conducted, and the relationship of structure–dielectric/piezoelectric properties were studied (Supplementary Note 10, Table S3 and Figs. S14–S17).

Furthermore, we calculated the distances between A-site cations on a per-unit cell basis via HAADF-STEM and estimated the local lattice anisotropy by determining the local c/a ratio. As shown in Fig. 4c, the standard deviation of the lattice parameter is significantly larger for 0.06PSN–0.61PMN–0.33PT than that for 0.67PMN–0.33PT, demonstrating a higher fluctuation in the sublattice parameter. Additionally, the local c/a ratios for PSN–PMN–PT varied more than those for PMN–PT, indicating a much larger tetragonality and a more dispersive behavior. These observations are consistent with the XRD data (Table S2).

Then, we conducted geometric phase analysis (GPA) on the HAADF-STEM images of 0.06PSN–0.61PMN–0.33PT and 0.67PMN–0.33PT crystals, from which we derived the variations of local strain Sxx along [001]C were (Fig. 4d, e). Notably, the ternary crystals possess significantly higher local strain (~3%) than their binary counterparts (1.5%), suggesting a large tetragonal lattice deformation c/a ratio.

This microstructure of the PSN–PMN–PT crystals has not been previously reported for any other ultrahigh-piezoelectric materials. It features a highly dispersed local heterogeneous structure with abundant tetragonal phases, markedly differing from the usual behavior of binary relaxor-PT crystals, where the high piezoelectric activity is only found in the rhombohedral-side MPB compositions. The phase-field calculations well match our experimental discoveries, verifying the experimentally observed microstructure and functionality. As shown in Fig. 4f, pure PMN–PT exhibits rhombohedral characteristics with a large domain size. When some tetragonal nanosized phases are introduced into this matrix (similar to the PSN–PMN–PT case), a dispersed domain structure with decreased domain size forms, and multiphase coexistence becomes inevitable, well agreeing with the transmission electron microscopy (TEM) results shown in Fig. 4a. We calculated the magnitudes of EC and d33 of these two systems, from which we observed significant EC enhancement without piezoelectricity reduction due to appropriate doping of the tetragonal phase (Supplementary Note 11, Table S4 and Fig. S18).

Discussion

Based on the experimental and phase-field simulation results, the ultrahigh piezoelectricity and extremely large EC in the PSN–PMN–PT system can be explained in the mesoscale. Previous studies have demonstrated that the introduction of cations into the B-site of PMN–PT can afford a high level of charge inhomogeneity28,29,30, consequently yielding strong relaxor behavior, as confirmed in this study (Fig. 3 and Supplementary Note 12). Compared to PMN–PT, it is considerably more difficult for PSN–PMN–PT to establish a ferroelectric long-range order with only short-range ordering between neighboring clusters, accounting for symmetry breaking; thus, we observed a highly dispersed local heterogeneous structure (Fig. 4). The dispersed micropolar state with multiphase coexistence is strongly correlated with abundant local heterogeneity (Fig. 4a), which can significantly flatten the free energy profile, significantly contributing to the ultrahigh piezoelectric activity12. In addition to the highly dispersed characteristic, 0.06PSN–0.61PMN–0.33PT crystal also features a considerable tetragonal phase component with a relatively large c/a ratio (Fig. 4b–d). Previous studies on the structure–property relation of relaxor-PT showed that tetragonal-rich crystals usually exhibit large EC31, where the large c/a ratio is directly related to the high potential barrier between different polar states. After Sc doping into PMN–PT, nanosized tetragonal domains are highly dispersed into the matrix (Fig. 4a). These tetragonal polar regions strongly interact with their neighboring clusters (Fig. 3e, f), acting as “frozen seeds” in the entire matrix and pining the PS switch via a cooperative effect, consequently yielding unparalleled coercive field enhancement. The pinning effect of the tetragonal polar regions on domain switch is associated with the difficult domain nucleation and growth process32,33, which is supported by the large activation electric field in 0.06PSN–0.61PMN–0.33PT (Supplementary Note 13 and Figs. S19 and S20).

Although an ultrahigh piezoelectric response and a large EC are generally exclusive in a single ferroelectric material (Fig. 1a), the enhancement of EC in our PSN–PMN–PT crystal is notably not at the expense of the piezoelectric activity, which can be explained from its particular microstructure. The dispersed microstructure is strongly correlated to a slush-like polar state with coexisting multiphases, including O/R, T, and M, signifying the instability of the ferroelectric phases, and intrinsically contributing substantially to the ultrahigh piezoelectric activity. Moreover, the interfacial energies can be manipulated using the abundant local structure heterogeneity arising from the tortuous interfaces between adjacent clusters, and the small domain size12, further improving the piezoelectric response. Note that due to the existence of the tetragonal phase, fully (001) poled crystals may contain considerable single-domain components34 and possess large shear piezoelectric activity that stems from the easy polarization rotation (Supplementary Note 14 and Figs. S21 and S22), which partially explains the two times larger d15 in 0.06PSN–0.61PMN–0.33PT than that in 0.67PMN–0.33PT.

Based on the above discussion, we propose a thermodynamic understanding of the inherent correlation between the macrostructure and materials properties: as regulated by Landau theory, the dispersed heterogeneous structure with multiphase coexistence makes the free energy extremely flat; meanwhile the highly tetragonal polar regions pin the domain switch, indicating the enhancement the potential barriers, resulting in a “flat and deep” potential well Fig. 5. This peculiar free energy profile causes a difficult polarization switch and an easy polarization rotation; consequently, an ultrahigh piezoelectric response and extremely large EC are simultaneously achieved. Both hard (large EC) and soft (high piezoelectric response) doping properties are affording using this strategy, successfully addressing the longstanding challenge that excellent sensitivity and high stability of dipoles are generally exclusive, and achieving a striking enhancement of the overall performance.

Fig. 5: Simultaneous achievement of ultrahigh piezoelectricity and extremely large EC.
figure 5

The particular local structure and relatively large c/a ratio in the PSN–PMN–PT are used to manipulate the free energy profile in different ways, affording a “deep and flat” free energy landscape. The PSN–PMN–PT system exhibits various characteristics including high level of local structure heterogeneity, slush-like multiphase coexistence, small domain size, and high density of PNRs, accounting for a flat potential well. Moreover, the lattice of PSN–PMN–PT system contains a considerable tetragonal phase component, demonstrating large anisotropy, which consequently contributes to a deep well, namely an enhanced ΔG. The flattened potential well facilitates the polarization (PS) rotation/elongation around the equilibrium position, enhancing the piezoelectric response, and the enhanced energy barrier makes the PS switch more difficult, contributing to a large EC.

Full size image

To verify the importance of the delicate optimization of the composition, our study is compared with previous studies based on similar material systems. In PSN–PMN–PT crystals with PSN:PMN ratio of 1:3, 1:1, or 3:1, Wang et al. 35 determined the piezoelectric constant as 1200–1600 pC/N and the coercive field as 4–6 kV/cm, which are significantly inferior to those of our 0.06PMN–0.61PMN–0.33PT sample (PSN:PMN ratio ~1:10). It has been demonstrated that the introduction of PSN into PMN–PT can yield a high level of lattice anisotropy (Supplementary Note 1)36,37, favoring a tetragonal phase. Thus, if a superfluous amount of Sc is introduced, the tetragonal clusters may become too large for dispersal into the entire ferroelectric matrix, destroying the desirable local heterogeneous microstructure; additionally, a phase separation could occur, causing functional degradation. Moreover, the importance of MPB composition in the piezoelectric response should be emphasized. Xi et al. 7,38 reported that in 0.06PSN–0.63PMN–0.31PT single crystals, although with a large coercive field of 8.17 kV/cm, the maximum piezoelectric constant is only ~1200 pC/N, which is similar to that of PZT ceramics or lead-free crystals. Guo et al. 39 reported that 0.05PSN–0.63PMN–0.32PT single crystals in R phase exhibit inferior performance with d33 = 1200 pC/N and ε33 ~ 3500. Compared to previous studies, our 0.06PSN–0.61PMN–0.33PT single crystals exhibit substantially superior overall performance (Supplementary Note 15, Table S5 and Fig. S23). Therefore, the appropriate balance between various effects, including relaxor and long-range order and tetragonal and other phases, need to be considered for materials-by-design. This strategy should be converted to an atomistic model to understand the contribution of each atom to the free energy profile in a complex material system, to ultimately realize high-performance and/or high-power applications.

The natural question arises that “why such simultaneous improvement has not been obtained in previous studies?” Currently, the most conventional strategy employed to enlarge the coercive field in relaxor-PT systems is hard doping8,9. In hard doping, a small amount (<2 mol%) of acceptor ions such as Mn2+/3+, is substituted into the B-sites of perovskite lattices, yielding acceptor–oxygen vacancy defect dipoles. These defect dipoles occupy energetically preferred sites in the lattice and align themselves along a preferential direction within a ferroelectric domain, and then, they move to the highly stressed areas of domain walls40. These defect dipoles pin the domain walls and stabilize the domain, establishing a parallel arrangement of defect dipole and local ferroelectric polarization, causing an offset of P–E behavior that is experimentally characterized as internal bias, which effectively increases the EC by 30% compared to that of undoped materials41. Such a significantly reduced degree of switchable polarization is accompanied by suppressed domain wall mobility, inevitably resulting in an inferior piezoelectric response. Alternatively, based on the P–E characterization results (Fig. 2c), an internal bias was not observed in the PSN–PMN–PT crystals, signifying the presence of a distinct mechanism associated with the intrinsically high lattice strain rather than the domain clamping effect observed for Mn-doped crystals. Therefore, the piezoelectric coefficients did not decrease with Sc doping, due to the no-loss or even enhanced extrinsic piezoelectric contribution. These easily removable domain walls are further suggested by Rayleigh analysis (Supplementary Note 16, and Figs. S24 and S25), showing that a large Rayleigh parameter α is afforded at both the room temperature and the temperature near TF–F, which is not favored in hard doping8,41.

In conclusion, by employing a precise microstructure-by-design, we successfully addressed the long-sought-after materials with simultaneous ultrahigh piezoelectricity and unparalleled enhancement of EC. Within the theoretical framework, we proposed a thermodynamic understanding of the inherent correlation between the free-energy landscape and material properties, where a “flat and deep” potential well is derived. Our dataset is the confirmation of the existence of extremely large EC in an ultrahigh piezoelectric material. Furthermore, although the relaxor–PT solid solution is employed herein, our proposed strategy is likely a universal and effective method for designing high-performance functional materials with both high tolerance and sensitivity to the external field.

Methods

Crystal growth

The PSN–PMN–PT single crystals were grown using the Bridgman technique. High-purity Sc2O3 (99.99%), Nb2O5 (99.95%), (MgCO3)4·Mg(OH)2·5H2O (>99.0%), PbO (>99.0%), and TiO2 (>99.0%) were used as raw materials. The precursors MgNb2O6 and ScNbO4 were synthesized in advance to avoid the impurity phase formation. Then, MgNb2O6, ScNbO4, TiO2 and PbO powders were mixed and placed in a Pt crucible wrapped with a sealed Al2O3 crucible. The crucible was placed in a computer-controlled Bridgman furnace, which was heated from 600 to 1400 °C at a rate of 10 °C/min and maintained at 1400 °C for 10 h, and a stable temperature gradient of 30–50 °C/cm was formed. The crucible was descended at a rate of 0.2–0.4 mm/h, and the PSN–PMN–PT single crystals gradually grew via spontaneous nucleation.

Sample preparation and electrical property measurements

All the samples used herein were [001]C-oriented with x//[100]C, y//[010]C and z//[100]C via XRD. After cutting and polishing, all the samples were annealed at 600 °C for 1 h to eliminate the stress generated during sample preparation. Gold electrodes were sputtered on the parallel (001)C faces of crystals. The temperature dependence of the dielectric constants was measured using an LCR meter (Agilent, 4284A) with a 2 °C/min step. P–E loops were obtained using a Precision Premier II tester (Radiant Technologies, Albuquerque). After being poled by a DC E-field of 10 kV/cm at room temperature, the longitudinal piezoelectric coefficient d33 was recorded using a quasi-static d33 meter (Institute of Acoustics, ZJ-4A) and shear coefficient d15 was measured using the resonance method. The resonance and anti-resonance frequencies were obtained using an Agilent 4294A impedance-phase gain analyzer, based on which the electromechanical coupling factors k33 and k15 were obtained.

PFM measurements and the autocorrelation function technique

For the PFM measurements employed in this work, the samples were ground to ensure a flat surface using the Al2O3 grinding powder and subsequently polished using polycrystalline diamond suspensions with abrasive particles of 9, 3, 1 μm, and 20 nm (MetaDi Supreme, Buehler). The PFM studies were performed using a Cypher ES (Asylum Research) in DART mode using Ir/Pt-coated conductive tips (Nanoworld, EFM). The autocorrelation images were obtained based on the PFM domain images via the following transformation42,43

$$C({r}_{1},{r}_{2})=\mathop{\sum}\limits _{x,y}D(x,y)D(x+{r}_{1},y+{r}_{2})$$
(3)

where D(x,y) is the piezoelectric signal and the autocorrelation function C(r1,r2) is the two-dimensional polarization–polarization correlation function. Furthermore, \(\langle C(r)\rangle ={\sigma }^{2}\exp [-{(r/\xi )}^{2h}]\) is the averaged autocorrelation function \(C({r}_{1},{r}_{2})\) over all in-plane directions.

Scanning transmission electron microscopy (STEM) experiments

The TEM samples were prepared using a Tescan LYRA-3 XUM Model focused ion beam instrument. The selected area electron diffraction patterns and morphology of the crystals in Fig. S8 were characterized using TEM FEI Talos F200. STEM images were acquired on a spherical aberration-corrected FEI Titan G2 microscope operated at 300 kV using a HAADF detector. All STEM images were Fourier-filtered using a lattice mask to remove noise. The strain analyses in HAADF-STEM images were obtained through GPA using the custom scripts in the Gatan DigitalMicrograph software44. The polar vector for each unit cell was determined as the B-site cation displacement relative to its four nearest A-site neighbor cations by fitting atom positions as two-dimensional Gaussian peaks45, which are mapped in the HADDF-STEM images of Figs. 4a and S9.

Phase-field simulations

In the phase-field simulations, the polarization Pi(r,t)(x,y,z) denotes the order parameter, which describes the ferroelectric polarization evolution. The temporal evolution of the polarization can be described by the time-dependent Ginzburg–Landau equation:

$$\frac{\partial {P}_{i}({{{{{\boldsymbol{r}}}}}},t)}{\partial t}=-L\frac{\delta {F}_{{\rm {P}}}}{\delta {P}_{i}({{{{{\boldsymbol{r}}}}}},t)},\,(i=x,y,z)$$
(4)

where t is the simulation time, L is the kinetic coefficient, r is the spatial position, and FP is the total free energy of the system that is denoted as follows46:

$${F}_{{\rm {P}}}=\iint \!\! \int({\,f}_{{\rm {bulk}}}({P}_{i})+{f}_{{\rm {elas}}}({P}_{i},\,{\varepsilon }_{ij})+{f}_{{\rm {elec}}}({P}_{i},\,{E}_{i})+{f}_{{\rm {grad}}}({P}_{i,j})){\rm {d}}V$$
(5)

where fbulk, felas, felec and fgrad represent the Landau bulk, elastic, electrostatic, and gradient energy densities, respectively. A stress-free boundary condition is adopted. The bulk energy density fbulk can be described as a six-order polynomial:

$${f}{_{\rm {bulk}}}= {\alpha }_{1}({P}_{x}^{2}+{P}_{y}^{2}+{P}_{z}^{2})+{\alpha }_{11}({P}_{x}^{4}+{P}_{y}^{4}+{P}_{z}^{4})\\ +{\alpha }_{12}({P}_{x}^{2}{P}_{y}^{2}+{P}_{x}^{2}{P}_{z}^{2}+{P}_{z}^{2}{P}_{y}^{2})+{\alpha }_{112}\left[{P}_{x}^{4}({P}_{y}^{2}+{P}_{z}^{2})\right.\\ \left.+{P}_{y}^{4}({P}_{y}^{2}+{P}_{x}^{2})+{P}_{z}^{4}({P}_{y}^{2}+{P}_{x}^{2})\right]+{\alpha }_{111}({P}_{x}^{6}+{P}_{y}^{6}+{P}_{z}^{6})\\ +{\alpha }_{123}{P}_{x}^{2}+{P}_{y}^{2}+{P}_{z}^{2}$$
(6)

where α1, α11, α12, α111, α112 and α123 are the Landau energy coefficients. Among which, only α1 is temperature-dependent, α1 = (T–TC)/(2ε0C0), where T is the temperature, TC is the Curie temperature, C0 is the Curie constant, and ε0 = 8.85 × 10−12 is the permittivity of vacuum47. The Landau coefficients of PMN–0.3PT and PMN–0.42PT were taken from ref. 48.

The gradient energy density can be expressed as

$${f}_{ \rm {{grad}}}=\frac{1}{2}{G}_{ijkl}\frac{\partial {P}_{i}}{\partial {r}_{j}}\frac{\partial {P}_{k}}{\partial {r}_{l}}$$
(7)

where Gijkl is the gradient energy coefficient. The electrostatic energy density can be written as

$${f}_{{ \rm {elec}}}=-\frac{1}{2}{\varepsilon }_{0}{K}_{ij}^{{\rm {b}}}{E}_{i}{E}_{j}-{E}_{i}{P}_{i}$$
(8)

where \({K}_{ij}^{{\rm {b}}}\) is the background relative permittivity and Ei is the electric field, which can be calculated as

$${E}_{i}=-\frac{\partial \varphi }{\partial {r}_{i}}$$
(9)

The electric potential φ can be obtained by solving the electrostatic equilibrium equation

$${\varepsilon }_{0}{K}_{ij}^{{\rm {b}}}\frac{{\partial }^{2}\varphi }{\partial {r}_{i}\partial {r}_{j}}=-\frac{\partial {P}_{i}}{\partial {r}_{i}}$$
(10)

Equations were numerically solved via the semi-implicit Fourier-spectral method49.