Introduction

Artificial circuit atoms interact with radiation much more strongly than conventional atoms1,2. In particular, a nearly unit efficiency scattering of single photons was observed with a superconducting flux qubit inductively coupled to a planar transmission line3, followed by a number of fundamental demonstrations involving resonant fluorescence of transmon qubits4,5,6,7. However, the lack of diversity among transition frequencies and selection rules in those two popular circuit atoms prevents their full-blown quantum control without invoking an extra cavity mode. In fact, indirectly interfacing superconducting qubits with radiation via far-detuned cavity resonators has been the sole option over the last decade to achieve both a state-of-the-art coherence time and a quantum non-demolition (QND) readout8. By contrast, a large portion of optical quantum technology relies on atoms that combine two types of transitions: a dark one with a long coherence time to store a qubit, and a bright one, which can fluoresce conditioned on the qubit state9. This effect of conditional fluorescence is known in atomic physics as electron shelving10 and it enables a direct interface between high-coherence qubits and propagating photons11. Inspired by this observation, we ask the following question: can we eliminate the cavity in a cQED setup by switching to three-level circuit atoms with appropriately designed transitions? Besides facilitating quantum communication and distributed quantum computing12,13,14, having a more direct qubit-photon interface may duly free up the on-chip space in quantum processor circuits, the majority of which is usually occupied by the readout resonators.

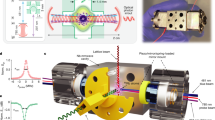
In this work we describe the electron shelving effect in a multilevel circuit atom—fluxonium15,16,17,18. Specifically, we demonstrate a conditional fluorescence readout of a qubit, which is directly coupled to a coaxial cable of the measurement apparatus, and yet has a relatively high coherence time. Fluxonium circuit consists of a Josephson junction shunted by a high-value inductance (superinductance) and a capacitance, here in the form of a bow-tie antenna (Fig. 1a). When cooled-down near 10 mK in a dilution refrigerator, the circuit dynamics can be characterized by a single collective degree of freedom, the superconducting phase-difference ϕ across the junction. At half-integer magnetic flux through the loop, ϕext = π, the variable ϕ behaves like the coordinate of a particle inside a symmetric double-well potential and the spectrum is first-order insensitive to flux noise (Fig. 1b). Tunneling across the barrier forms the qubit states \(\left|0\right\rangle\) and \(\left|1\right\rangle\), split by a relatively low-frequency ω01 (in the present device ω01 = 2π × 1.152 GHz). The non-computational states \(\left|2\right\rangle\), \(\left|3\right\rangle\), etc., are the orbitals at energy above the barrier. Higher-frequency transitions \(\left|1\right\rangle -\left|2\right\rangle\) and \(\left|0\right\rangle -\left|3\right\rangle\) are natural candidates for cycling, and for technical reasons, we use the latter one at frequency ω03 = 2π × 6.544 GHz. Note, transition \(\left|0\right\rangle -\left|3\right\rangle\) is allowed thanks to the absence of the harmonic ladder selection rule in fluxoniums.

Fig. 1: Cavityless cQED with fluxonium qubits.
figure 1

a Electron microscope image of fluxonium’s superconducting loop, made of one smaller-area Josephson junction and an array of larger-area junctions. Top inset: fluxonium’s effective three elements circuit model. Bottom inset: the smaller-area junction is connected to bow-tie antenna capacitance (optical image). b Effective potential seen by the phase-difference ϕ across the smaller-area junction (black), energy levels (horizontal black lines), and wavefunctions (gray) at the half-integer external flux through the loop, ϕext = π. The qubit levels are \(\left|0\right\rangle\) and \(\left|1\right\rangle\), the readout transition is \(\left|0\right\rangle -\left|3\right\rangle\), and even transitions are dipole-forbidden. c Setup for a broadband wireless coupling of fluxonium circuit to an external coaxial cable (not shown). d Transition frequencies and corresponding matrix elements of the Cooper pair number operator −i∂ϕ (colored stars). The solid line shows microwave transmission through the enclosure measured using a symmetric two-port configuration. Single-port configuration is used for all other measurements, as shown in Fig. 1c.

Full size image

Shelving requirements are met by the combination of (i) octaves of frequency separation between the qubit and cycling transitions, (ii) engineered frequency-dependent coupling of the atom circuit to external radiation, and (iii) parity selection rule at ϕext = π. We mount the fluxonium chip inside a copper enclosure (not a cavity resonator) with a specially designed input/output port. The port adapts a transverse-electromagnetic (TEM) field in the externally connected coaxial cable to a transverse electric (TE) field inside the enclosure, which in turn couples to the qubit antenna. Such a wireless link19 between an on-chip circuit and external radiation has a low-frequency cutoff associated with the TE-mode, which we designed to be near the frequency ω03. Therefore, the radiative lifetime of state \(\left|3\right\rangle\) can be orders of magnitude shorter than that of state \(\left|1\right\rangle\) even though transitions \(\left|0\right\rangle -\left|1\right\rangle\) and \(\left|0\right\rangle -\left|3\right\rangle\) have comparable matrix elements of the Cooper pair number operator −i∂ϕ in the present device (Fig. 1d). The radiative decay \(\left|3\right\rangle \to \left|2\right\rangle\) is similarly suppressed by the TE-mode cutoff, while the direct decay \(\left|3\right\rangle \to \left|1\right\rangle\) is dipole-forbidden by the parity selection rule. As a result, the driving of transition \(\left|0\right\rangle -\left|3\right\rangle\) would generate fluorescence if and only if the atom (and hence the qubit) starts in state \(\left|0\right\rangle\). Despite a periodic transit of population through state \(\left|3\right\rangle\), the circuit rapidly relaxes back to state \(\left|0\right\rangle\) on switching off the drive. In state \(\left|1\right\rangle\) the circuit is unresponsive to the drive (shelved). Thus, the conditional fluorescence readout has a built-in QND property, as, ideally, it does not switch the qubit state.

Following earlier work, we monitor fluorescence in the microwave domain using a phase-sensitive (homodyne) scheme3,4. To understand the measurement outcome in our single-port setup, let us neglect, for the time being, the possibility of transitions outside the cycling manifold \(\{\left|0\right\rangle ,\left|3\right\rangle \}\), and assume the environment to be at zero temperature. According to the input/output theory, the complex amplitude of the outgoing reflected wave αout is given by the sum of the incoming wave amplitude αin and the fluorescence field emitted by the atom20. For a drive near the readout frequency ω03, this relation takes the simple expression \({\alpha }_{{{{{{{{\rm{out}}}}}}}}}={\alpha }_{{{{{{{{\rm{in}}}}}}}}}-\sqrt{{{\Gamma }}}\langle | 0\rangle \langle 3| \rangle\), where Γ is the rate of direct radiative decay \(\left|3\right\rangle \to \left|0\right\rangle\), and the expectation value is taken over the dissipative driven steady state. Because the fluorescence contribution \(-\sqrt{{{\Gamma }}}\langle | 0\rangle \langle 3| \rangle\) is zero if the atom is not initially in state \(\left|0\right\rangle\), the complex reflection coefficient r = αout/αin can be related to the ground state population p0:

$$r=1-2{p}_{0}\times \frac{{{{\Gamma }}}^{2}/2-i(\omega -{\omega }_{03}){{\Gamma }}}{{{{\Gamma }}}^{2}/2+{{{\Omega }}}^{2}+2{(\omega -{\omega }_{03})}^{2}}.$$
(1)

Here the Rabi rate \({{\Omega }}=2\sqrt{{{\Gamma }}}{\alpha }_{{{{{{{{\rm{in}}}}}}}}}\) conveniently represents the readout drive strength in units of [Hz] and ω is the drive angular frequency. Information about the state of the atom is carried by the fluorescence signal conditionally generated during readout. Its power is proportional to the square of Ω∣1 − r(ω, Ω)∣. This quantity is maximal for \({{\Omega }}={{\Gamma }}/\sqrt{2}\) and ω = ω03, in which case Eq. (1) simplifies to r = 1 − p0. The maximal fluorescence power condition reflects the fact that a linear detection relies on the drive-induced coherence between states \(\left|0\right\rangle\) and \(\left|3\right\rangle\), rather than on the population of state \(\left|3\right\rangle\) in the more common case of photodetection.

Results

We begin our experiment by characterizing the fluorescence of transition \(\left|0\right\rangle -\left|3\right\rangle\) (Fig. 2a, b). The measured reflection amplitude r as a function of drive frequency ω and amplitude Ω fits well to the Eq. (1) model. Only four adjustable parameters are used in the global fit of over ten curves: ω03 = 2π × 6.544 GHz, Γ = 2π × 1.74 MHz, the thermal equilibrium ground state population \({p}_{0}^{{{{{{{{\rm{th}}}}}}}}}=0.78\), and the scaling factor converting the value of Ω to the RF-source amplitude. In order to ensure that the atom is in the driven steady-state while fluorescence is being integrated, we turn on the drive, wait for a time τw = 0.5 μs, then integrate the reflection signal during a time τm = 0.5 μs. The wait time is chosen so that it is much longer than the characteristic emission time 1/Γ = 91 ns but much shorter than the time scale of non-radiative processes in the system. At low power, Ω ≪ Γ, the reflection amplitude r as a function of frequency becomes a circle in the parametric IQ-plot, bounded by the values \({{{{{{{\rm{Re}}}}}}}}[r]=1\) for ∣ω − ω03∣ ≫ Γ and \({{{{{{{\rm{Re}}}}}}}}[r]=1-2{p}_{0}^{{{{{{{{\rm{th}}}}}}}}}\) at ω = ω03. As stronger driving saturates the \(\left|0\right\rangle -\left|3\right\rangle\) transition, the circle deforms into an ellipse and progressively shrinks into a point at r = 1 for Ω ≫ Γ. Agreement between spectroscopy data and the Eq. (1) model indicates that transition \(\left|0\right\rangle -\left|3\right\rangle\) is coupled to itinerant radiation with a nearly unit efficiency, and non-radiative processes are negligible.

Fig. 2: Conditional fluorescence readout.
figure 2

a Complex plane representation and b real part of the reflection coefficient r as a function of readout drive frequency ω at various powers represented by the \(\left|0\right\rangle -\left|3\right\rangle\) transition Rabi frequency Ω (inset). The experimental data (colored dots) are fitted by the theory of Eq. (1) (lines) with Γ = 2π × 1.74 MHz and p0 = 0.78 due to the thermal occupation of excited states. In the limit Ω → 0, the data in Fig. 2a becomes a circle with a radius p0 (gray circle, p0 = 1 and dashed circle, p0 = 0.78). The optimal readout settings are highlighted by the black star and arrow (see text below Eq. (1)). c Rabi oscillations (left panel), energy relaxation (right panel, yellow circles), and spin-echo coherence decay (green triangles) were measured using conditional fluorescence readout at optimal settings. Fitting to exponential decay yields (coincidentally) T1 = T2 = 52 μs.

Full size image

The readout characterization presented in Fig. 2a, b provides absolute calibration of the measurement records in terms of the fluxonium’s ground state population p0. This property is in contrast with a standard dispersive readout, where the measurement records are only known up to a scaling factor to the qubit population and have to be independently calibrated. In order to account for small thermal fluctuations over the course of the experiment, we repeated the previous calibration over 5 days. We find an average thermal equilibrium value of \({p}_{0}^{{{{{{{{\rm{th}}}}}}}}}=0.76\pm 0.03\), which corresponds to the atom being in thermal equilibrium at an effective temperature T = 45 ± 5 mK, which is indeed commonly encountered in superconducting qubits. In what follows, we operate the readout at the maximal signal point, where p0 = 1 − r (black star on Fig. 2a).

The protocol for time-domain qubit manipulations matches that of traditional circuit quantum electrodynamics. For example, applying a drive at the qubit frequency ω01 = 2π × 1.15 GHz prior to the readout pulse results in Rabi oscillations of p0 as a function of drive duration (Fig. 2c—left panel). Operating the circuit slightly off the sweet-spot at ϕext/2π = 0.507, i.e., weakly breaking the parity selection rule, we could produce Rabi oscillations between states \(\left|0\right\rangle\) and \(\left|2\right\rangle\) by compensating the vanishing transition dipole with a strong drive at frequency ω02 = 2π × 3.88 GHz (see Supplementary Note 5). Importantly, the nonzero decay rates of even transitions resulting from breaking the parity selection rule remain several orders of magnitude smaller than other decoherence processes (see Supplementary Table 1). The reflection coefficient measured during Rabi oscillations between states \(\left|0\right\rangle\) and either \(\left|1\right\rangle\) or \(\left|2\right\rangle\) yields equilibrium ratios \({p}_{1}^{{{{{{{{\rm{th}}}}}}}}}/{p}_{0}^{{{{{{{{\rm{th}}}}}}}}}=0.329\) and \({p}_{2}^{{{{{{{{\rm{th}}}}}}}}}/{p}_{0}^{{{{{{{{\rm{th}}}}}}}}}=0.007\) independently of the absolute calibration of \({p}_{0}^{{{{{{{{\rm{th}}}}}}}}}\). Assuming \({p}_{0}^{{{{{{{{\rm{th}}}}}}}}}+{p}_{1}^{{{{{{{{\rm{th}}}}}}}}}+{p}_{2}^{{{{{{{{\rm{th}}}}}}}}}=1\), we obtain the equilibrium value of \({p}_{0}^{{{{{{{{\rm{th}}}}}}}}}=0.75\), in close agreement with the spectroscopic calibration (see Supplementary Note 7). We checked that the qubit can be initialized with p0 ≈ 0.9 by strongly driving the \(\left|1\right\rangle -\left|3\right\rangle\) transition. During such a drive, entropy is removed from the on-chip circuit via spontaneous emission of photons \(\left|3\right\rangle \to \left|0\right\rangle\) into the measurement line (see Supplementary Note 6). However, the value \({p}_{0}^{{{{{{{{\rm{th}}}}}}}}}\) turns out to be sufficiently high for the purpose of our time-domain experiments and hence we performed them all starting from thermal equilibrium.

The energy relaxation signal following a π-pulse at frequency ω01 is exponential with a characteristic decay time T1 = 52 μs, which is nearly three orders of magnitude longer than the radiative decay time of state \(\left|3\right\rangle\) (Fig. 2c—right panel). Yet, given the highly suppressed port coupling at the qubit frequency, such value of T1 is still too short to be explained by radiative decay. In fact, the qubit decay is likely due to the dielectric loss in the circuit’s antenna capacitance with an effective quality factor Qdiel = 5 × 105. The spin-echo signal decay is also exponential with a characteristic coherence time T2 = 52 μs. The additional dephasing rate Γφ = 1/T2 − 1/2T1 can be explained by a thermal occupation of the propagating modes near the frequency ω03, which would induce upward jumps \(\left|0\right\rangle \to \left|3\right\rangle\). Assuming the rate of such jumps is Γφ, it is related to the effective photon temperature T at the microwave port via the detailed balance equation \({{{\Gamma }}}_{\varphi }={{\Gamma }}\exp (-\hslash {\omega }_{03}/{k}_{B}T)\) (see Supplementary Note 8). The value T = 45 ± 5 mK, previously extracted from the equilibrium population measurement, indeed accounts for the extracted value of Γφ. This dephasing channel can be exponentially suppressed in better-thermalized setups.

Finally, we explore the QND character of the conditional fluorescence measurement. An elementary test consists of measuring the atom population dynamics induced by the readout process. To do so, we drive transition \(\left|0\right\rangle -\left|3\right\rangle\) for a duration τ ≫ 1/Γ, pause for a brief period for state \(\left|3\right\rangle\) to relax, swap the state of interest with state \(\left|0\right\rangle\), and perform our conditional fluorescence readout (Fig. 3a—top panel). As a result, we acquire the population transients p0(t), p1(t), and p2(t) of states \(\left|0\right\rangle\), \(\left|1\right\rangle\), and \(\left|2\right\rangle\), respectively, during a drive at readout frequency. The fidelity of this population measurement is limited by the swap gate fidelities, which is above 99% for \(\left|0\right\rangle -\left|1\right\rangle\) and above 90% for \(\left|0\right\rangle -\left|2\right\rangle\), measured using randomized benchmarking (see Supplementary Note 3). The p0(t)-data indicates a gradual leakage of the population outside the cycling manifold \(\{\left|0\right\rangle ,\left|3\right\rangle \}\) on a characteristic time scale τcyc = 9.6 μs. Importantly, the leakage involves only states \(\left|1\right\rangle\) and \(\left|2\right\rangle\) as the data satisfies p0 + p1 + p2 = 1 within the measurement uncertainty (Fig. 3a— bottom panel). The average number of fluorescence cycles, in our case Ncyc = Γ × τcyc ≈ 105, is usually used in optical shelving to quantify the deviation of the readout from ideal QND behavior.

Fig. 3: Transient dynamics of fluorescence.
figure 3

a Time-domain evolution of populations p0 (down blue triangles), p1 (up red triangles), and p2 (yellow squares) induced by the cycling of the readout transition (pulse sequence on top). Note that p0 decays approximately exponentially in time with a characteristic time scale τcyc = 9.6 μs, after which the population is transferred from state \(\left|0\right\rangle\) to states \(\left|1\right\rangle\) and \(\left|2\right\rangle\). The black circles indicate p0 + p1 + p2 and the error bars represent the standard deviation coming from the repetition of the measurements over two days. b Free evolution of populations starting predominantly from state \(\left|2\right\rangle\) (pulse sequence on top). The data in both (a) and (b) is adequately explained by an optical pumping model (solid lines) (see text) where the dominant error mechanism is a non-radiative decay due to dielectric loss.

Full size image

Fluorescence lifetime τcyc can be accounted for by non-radiative transitions outside the cycling manifold. We further constrain the energy relaxation rates in our circuit by measuring populations of states \(\left|0\right\rangle\), \(\left|1\right\rangle\), and \(\left|2\right\rangle\) as a function of time following a \(\left|0\right\rangle -\left|2\right\rangle\) swap (Fig. 3b). Combining the two data sets shown in Fig. 3a and b, we construct a relaxation model based on two additional decay mechanisms, dielectric loss and quasiparticle tunneling across the junction. The model adequately matches the six experimental curves with only three adjustable parameters: the dielectric loss quality factor Qdiel = 5 × 105, previously introduced to account for decay \(\left|1\right\rangle \to \left|0\right\rangle\), the dimensionless quasiparticle density xqp = 10−6, and a fudge-factor of 2.5 in front of the \(\left|3\right\rangle \to \left|2\right\rangle\) decay rate. The latter could be due to a nearby two-level fluctuator21. This model highlights two specific fluorescence-blocking processes. A small role is played by the direct parity-breaking decay \(\left|3\right\rangle \to \left|1\right\rangle\) with a characteristic time 36 μs due to quasiparticle tunneling. The dominant readout error, though, comes from the decay \(\left|3\right\rangle \to \left|2\right\rangle\) with a characteristic time of 3.6 μs due to dielectric loss in the fluxonium capacitance (see Supplementary Note 8).

Discussion

In summary, we showed that a single circuit degree of freedom can combine both the qubit and the readout functions. The readout was made possible by the phenomenon of conditional fluorescence, analogous to electron shelving of atomic clocks. The required pair of dark and bright transitions arises naturally from fluxonium’s strong anharmonicity, in contrast to more complex circuit implementations of V- and Λ-type systems22,23,24. In future experiments, one can use transition \(\left|1\right\rangle -\left|2\right\rangle\) for cycling, in which case the fluorescence lifetime τcyc should increase to the T1-limit. The 3D coupling setup in Fig. 1c can be replaced by the more common capacitive connection to an on-chip transmission line. In that case, the qubit emission would still be strongly suppressed at low frequencies16, approximately as \(1/{\omega }_{01}^{3}\) for ℏω01 ≳ kBT. Finally, adding a parametric amplifier would enable a single-shot readout even at the single-photon power level25 (see Supplementary Note 4).

Going cavityless offers the most direct interface between superconducting qubits and propagating microwave photons without degrading the qubit coherence time. Such an interface can be useful for exploring quantum optics in the microwave range, with new applications, such as photon routing controlled by superposition of states26,27. In the context of waveguide QED, our setup implements a quantum node which can act simultaneously as an emitter and as a memory. This development may pave the way to the preparation of highly-entangled states over a quantum network14 and their use for distributed quantum computation protocols, such as teleported gates28. Of particular interest is to combine the shelving readout with recently developed single microwave photon counters29,30,31. Last but not least, eliminating readout resonators from large-scale quantum processor circuits may save precious on-chip space.

Methods

Derivation of Eq. 1

A drive at angular frequency ω near readout frequency induces damped Rabi oscillations of the readout transition with the Hamiltonian

$$H/\hslash =\frac{(\omega -{\omega }_{03})}{2}(\left|3\right\rangle \left\langle 3| -| 0\right\rangle \left\langle 0\right|)-i\frac{{{\Omega }}}{2}(\left|3\right\rangle \left\langle 0| -| 0\right\rangle \left\langle 3\right|)$$
(2)

and \({{\Omega }}=2\sqrt{{{\Gamma }}}{\alpha }_{{{{{{{{\rm{in}}}}}}}}}\). In all generality, one should consider all sources of loss and dephasing such as spontaneous emission in the line, non-radiative decay, and extra-dephasing due to flux noise, including transitions from the readout subspace to other states of the atom. However, the readout transition has been designed so that its emission rate in the line overcomes by many orders of magnitude the other decay and dephasing processes. Assuming an environment at zero temperature, the density matrix of the atom ρ evolves according to the following Lindblad equation

$$\dot{\rho }=-\frac{i}{\hslash }[H,\rho ]+{{\Gamma }}{{{{{{{\mathcal{D}}}}}}}}[\left|0\right\rangle \left\langle 3\right|](\rho )$$
(3)

where \({{{{{{{\mathcal{D}}}}}}}}\) is the Lindblad superoperator. The steady-state of this equation yields to the expectation value

$$\langle | 0\rangle \langle 3| \rangle =\frac{{{\Omega }}}{{{\Gamma }}}\frac{{{{\Gamma }}}^{2}/2-i(\omega -{\omega }_{03}){{\Gamma }}}{{{{\Gamma }}}^{2}/2+{{{\Omega }}}^{2}+2{(\omega -{\omega }_{03})}^{2}},$$
(4)

from which we derive Eq. (1). The good agreement between the theoretical model and the experimental data validates the assumptions made in the previous derivation.

Reflection coefficient calibration

The measured reflection coefficient is only known up to a scaling factor and has to be calibrated. In fact, the readout drive undergoes several stages of attenuation before reaching the artificial atom, while the fluorescence signal is amplified before digitization. Calibration is performed as follows. The circuit is flux biased at ϕext = 0 and is probed in reflection between 6.3 and 6.6 GHz. The external flux is chosen so that no circuit transition exists in the frequency band of interest. The acquired signal scal(ω) therefore accounts for the temperature-dependent attenuation and filtering of the lines as well as the whole acquisition setup amplification chain. Once the atom is biased at the wanted flux, the reflection coefficient r is deduced from the experimentally measured signal \({s}_{\exp }(\omega )\) by

$$r=\frac{{s}_{\exp }(\omega )}{{s}_{{{{{{{{\rm{cal}}}}}}}}}(\omega )}1{0}^{({P}_{{{{{{{{\rm{cal}}}}}}}}}-{P}_{\exp })/20}$$
(5)

with \({P}_{{{{{{{{\rm{cal}}}}}}}}/\exp }\) the room-temperature power of the readout drive during the calibration/experiment, expressed in dBm.

Relaxation model of transient dynamics

The relaxation model used to reproduce the data represented in Fig. 3 considers three distinct decay mechanisms. A jump from a state \(\left|i\right\rangle\) to \(\left|j\right\rangle\) can occur due to radiative decay in the line, dielectric loss, or quasiparticle tunneling in the junction, with the rates \({{{\Gamma }}}_{ij}^{{{{{{{{\rm{rad}}}}}}}}},\,{{{\Gamma }}}_{ij}^{{{{{{{{\rm{diel}}}}}}}}},\,{{{\Gamma }}}_{ij}^{{{{{{{{\rm{qp}}}}}}}}}\), respectively. All rates are computed assuming an equal bath temperature T = 50 mK for all decay mechanisms (see Supplementary Note 8).

We simulate the dynamics of the atom using the Python library QuTiP32. The spectrum of the fluxonium hamiltonian \({H}_{f}=4{E}_{C}{(-i\partial \phi )}^{2}+{E}_{L}{\phi }^{2}-{E}_{J}\cos (\phi -{\phi }_{{{{{{{{\rm{ext}}}}}}}}})\) is first computed in a Hilbert space of dimension 100 × 100, to ensure accuracy of the computed eigenfrequencies ωi and eigenstates \(\left|i\right\rangle ,\,i\le 99\). We then solve numerically the Lindblad master equation in the Fock state basis for a smaller Hilbert space containing the first ten eigenstates. This takes into account the fact that high-energy states do not contribute to the atom dynamics and allows for a faster computing time. The master equation contains all the jump operators \({\{\sqrt{{{{\Gamma }}}_{ij}^{{{{{{{{\rm{rad}}}}}}}}}+{{{\Gamma }}}_{ij}^{{{{{{{{\rm{diel}}}}}}}}}+{{{\Gamma }}}_{ij}^{{{{{{{{\rm{qp}}}}}}}}}}\left|j\right\rangle \left\langle i\right|\}}_{i,j\le 9}\) computed from the diagonalization of Hf. The initial state of the atom is the thermal equilibrium state ρth for Fig. 3a and a perfect swap between \(\left|0\right\rangle\) and \(\left|2\right\rangle\) on ρth for Fig. 3b.

The Hamiltonian for Fig. 3a is \(H=-i\frac{{{\Omega }}}{2}(\left|3\right\rangle \left\langle 0\right|-\left|0\right\rangle \left\langle 3\right|)\) and represents the readout drive, while it is 0 for Fig. 3b. They correspond to computing the evolution in the frame rotating at all the eigenfrequencies, i.e., applying the unitary \(U(t)=\exp (-i{H}_{f}t/\hslash )=\exp (-i{\sum }_{j}{\omega }_{j}\left|j\right\rangle \left\langle j\right|t)\) to the driven and undriven hamiltonians, respectively. We find that the transient dynamics of fluorescence is best reproduced for \({{\Omega }}=0.95{{\Gamma }}/\sqrt{2}\). This deviation from the ideal value can be due to small drifts between the measurements of Figs. 2 and 3.