Introduction

Oxide interfaces have acquired much attention in the last decade due to the emergence of multifunctionalities1,2,3,4. For example, LaAlO3 and SrTiO3 are both electrical insulators, but when they are grown on top of each other, highly conducting channels are formed at the interface1, accompanying ferromagnetism2,3,4,5 and gate-tunable superconductivity6,7. A key question that arises from such interfaces is whether bulk properties of a heterostructure can be controlled through the emergent states of matter at the interfaces. Interfacial effects arising from electrostatic and crystalline symmetry mismatches have been known to play important roles in determining the domain structures of ferroelectric thin films. Indeed, Yu et al. reported that atomically precise control of the interface could result in different polarization states in \((001)\)-oriented ferroelectric films8, having the polarization perpendicular to the surface. However, mechanisms underlying the interfacial symmetry mismatch on domain states and the ferroelectric response of in-plane polarized films are still not understood.

Single-domain in-plane polarized states are highly desirable for a number of potential functional device applications, such as high-performance electro-optic modulators9 and planar-type ferroelectric tunnel junctions10. However, epitaxial oxide thin films with in-plane polarization typically exhibit complicated multi-domain states which can severely degrade optical device performances11. For example, for ferroelectric waveguide applications, the waveguide loss and the electro-optic coefficient of ferroelectric materials are strongly influenced by the optical scattering by domain boundaries that exist in multidomain ferroelectric thin films and the crystalline quality of the films12,13,14,15.

In this work, we report the strategy for in-plane single-domain in BaTiO3 (BTO) ferroelectric thin films via interfacial symmetry and electrostatic potential mismatch using \({(110)}_{{{{{{\rm{O}}}}}}}\)-oriented PrScO3 (PSO) substrates. Density functional theory calculations and phase-field simulations predict the key roles of the interfacial environment between a film and the substrate, i.e., anisotropic strain, monoclinic distortion, and interfacial electrostatic potential, in stabilizing in-plane single-domain in BTO films on PrScO3 (PSO) \({(110)}_{{{{{{\rm{O}}}}}}}\) substrates. Scanning transmission electron microscopy (STEM), piezoresponse force microscopy (PFM), polarization hysteresis loop measurements, and optical second harmonic generation (SHG) results reveal that polarization direction of the BTO film is mainly along PSO \({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\) direction, while it has a small variable tilting along PSO \({\left\langle 002\right\rangle }_{{{{{{\rm{O}}}}}}}\) directions, leading to “quasi-single-domain” state. This work offers an approach to engineer in-plane ferroelectric epitaxial oxide thin films, enabling the development of device applications.

Results

Modeling of in-plane single-domain BTO

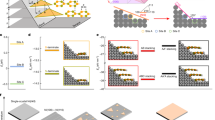
To design in-plane single-domain ferroelectric film, taking BTO—a canonical ferroelectric material—as a model system, we first discuss universal aspects of epitaxial strain for ferroelectric domain configurations. In general, in-plane polarization of ferroelectric oxide thin films is established under tensile strains16,17,18,19. However, under an isotropic biaxial strain, such films typically possess complicated multi-domain structures due to the presence of two energetically degenerate ferroelastic variants, resulting in four possible ferroelectric variants (Fig. 1a). Even though a proper anisotropic strain could give rise to single in-plane ferroelastic variant, 180° ferroelectric domains, corresponding to two possible ferroelectric variants, are still energetically equivalent (Fig. 1b).

Fig. 1: Schematic diagram depicting the strategy employed to achieve single in-plane ferroelectric film.
figure 1

a BTO films grown under an isotropic biaxial tensile strain. x, y, and z correspond to the pseudocubic (pc) [100], [010], and [001] axes, respectively. Due to tensile strain, c-axis of BTO lies along the in-plane direction of the substrate. However, the isotropic geometry gives rise to form a multi-domain configuration where four ferroelectric variant exists. b In the case of the BTO film grown under proper anisotropic biaxial strain, which is that the bpc and cpc of substrate are close to the a/b-axis and c-axis lattice parameters of tetragonal BTO, respectively, the BTO film is expected to have a polarization direction parallel to z directions, resulting in two ferroelectric variants. c BTO films grown on the substrate which possesses proper in-plane lattice match, monoclinic nature, and polar planes (e.g., BO2-terminated A3+B3+O3 substrates), one of two configurations can be stabilized. Note that the effect of electric field generated by polar planes is weaker and weaker as far away from the interface, which results in a single ferroelectric variant along in-plane direction.

Full size image

To energetically stabilize one of the two in-plane polarized states, we note that a symmetry lowering of the BTO film (i.e., monoclinic distortion) via an interfacial symmetry mismatch effect could play a central role (Fig. 1c). It is well known that interfacial oxygen octahedral coupling could initiate structures propagation over extensive distances and subsequently allows to tune the structure of epitaxial film20. For example, at the interface between CaTiO3 and NdGaO3, oxygen octahedral coupling breaks the mirror symmetry and stabilize a single domain structure with a single monoclinic tilt direction20. Once a monoclinic distortion is introduced near the interface region, an out-of-plane polarization will appear due to the symmetry lowering and the orientation will be determined by the polarity of the substrate surface termination. Such a monoclinic distortion of BTO is likely to gradually relax as the thickness of the film increases21,22, resulting in a single-domain in-plane ferroelectric state at the region far away from the interface (Fig. 1c).

To test feasibility of this approach, we consider orthorhombic rare-earth scandate (REScO3) substrates (Supplementary Table 1). It should be noted that the pseudocubic (pc) lattice parameter of REScO3 substrates, apc = 0.394 – 0.405 nm23,24, is similar to c-lattice parameter of bulk tetragonal BTO (4.036 Å)25. In addition, \({(110)}_{{{{{{\rm{O}}}}}}}\)-oriented REScO3 substrates possess a monoclinic nature since the out-of-plane direction (\([110]_{{{{{{\rm{O}}}}}}}\)) is not perpendicular to one of in-plane directions \(([1\bar{1}0]_{{{{{{\rm{O}}}}}}})\)26. Furthermore, a reliable method to obtain complete ScO2-terminated REScO3 has been reported27. Among them, \({(110)}_{{{{{{\rm{O}}}}}}}\)-oriented PSO substrate is a promising candidate for the following two reasons: first, its lattice parameters along bpc (i.e., \({\left[002\right]}_{{{{{{\rm{O}}}}}}}\)) and cpc (i.e., \({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\)) axis are similar to the b (=a) and c lattice parameters of bulk tetragonal BTO with misfit strains of +0.38% and −0.25%, respectively (Details in axis notations and the crystallographic relationship between BTO and PSO are represented in Supplementary Fig. 1). Second, PSO has a high in-plane anisotropic ratio (cpc/bpc) of 1.005, which is the largest among all scandates (Supplementary Table 1).

To evaluate the possibility of stabilizing an in-plane single-domain state in BTO/PSO heterostructures, we performed first-principles calculations. The BTO/PSO heterostructure was modeled using BTO lying on the top of ScO2-terminated PSO \({\left[110\right]}_{{{{{{\rm{O}}}}}}}\) (Fig. 2). Notably, we found that there is a monoclinic distortion in the BTO, and its tilt direction is opposite to that of PSO. Other possible structural configurations of BTO, where the tilt direction is the same as PSO was also calculated (Supplementary Fig. 2a). Interestingly, BTO/PSO heterostructures with opposite tilt direction turns out to be energetically favorable by 18.6 mJ/m2 (For more details, see the Supplementary Fig. 2 and Note 1). As expected, the out-of-plane polarization component of BTO is pointed “downward” to the interface due to negative charges associated with the ScO2 terminated PSO substrate, while the in-plane polarization component is preferably along PSO\({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\) direction, resulting in an overall polarization along the diagonal direction. On the other hand, to predict possible ferroelectric domain structures far away from the interface of the BTO thin films, we employed the phase-field method28. As suggested by the first-principles calculation, we assume that the initial state of the film has a uniform, in-plane polarization along PSO\({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\,\)direction. The interfacial effect associated with the negative charges is considered by introducing a layered charge distribution at the interface. A more detailed description of the model is given in the Method section. A representative two-dimensional section of the phase-field simulation results demonstrate a quasi-single-domain polarization state in the in-plane direction along PSO\({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\) as shown in Fig. 2b. There is also a spatial distribution of polarization which gradually rotates across the film thickness. Near the substrate-film interface an out-of-plane (toward the substrate) distorted polarization (Fig. 2d) is observed whereas near the film surface the polarization is entirely in-plane (along PSO \({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\) direction) (Fig. 2c). The out-of-plane polarization gradually decreases through the film thickness while the in-plane polarization magnitude remains nearly constant. This single domain state with in-plane polarization in the BTO/PSO heterostructures is consistent with our model as shown in Fig. 1c, suggesting the possibility to stabilize such a unidirectional in-plane polar state.

Fig. 2: Theoretical calculations of BTO/PSO heterostructure.
figure 2

a Calculated atomic structure of BTO/PSO \({(110)}_{{{{{{\rm{O}}}}}}}\) heterostructure. b The spatial polarization distribution of BTO film obtained from the phase field simulation. The film was assumed to be 50 nm thick with a fully coherent interface with respect to the substrate. Color bar reflects the polarization angle with respect to PSO \({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\). c, d The out-of-plane polarization gradually decreases through the film thickness (from (d) to (c)), becomes negligible near the film surface, predicting single domain in-plane BTO film.

Full size image

Experimental demonstration of in-plane single-domain BTO

For an experimental demonstration of BTO with single-domain in-plane polarization, BTO films with a thickness of 50 nm were grown on atomically smooth PSO \({(110)}_{{{{{{\rm{O}}}}}}}\) substrates by pulsed laser deposition (PLD) (see “Methods” for details). The crystallinity of the BTO films on PSO substrates was inferred from four-circle X-ray diffraction (XRD) measurements with a Cu Kα1 source (Supplementary Fig. 4). From reciprocal space maps of the BTO film around the PSO \({(33\bar{2})}_{{{{{{\rm{O}}}}}}}\) and PSO \({(240)}_{{{{{{\rm{O}}}}}}}\) reflections, we concluded that the BTO films are fully coherent with the PSO substrate. The lattice parameters of BTO were determined to be apc,BTO = 4.004 Å, bpc,BTO = 4.007 Å and cpc,BTO = 4.026 Å, using pseudocubic notation. Given the bulk lattice parameters, the BTO film is under a compressive strain along PSO \({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\) direction while under a tensile strain along PSO \({\left[002\right]}_{{{{{{\rm{O}}}}}}}\) direction (Supplementary Table 1).

To directly observe the polarization configuration of a BTO film, high-angle annular dark-field (HAADF) imaging using scanning transmission electron microscopy (STEM) was employed. Figure 3a shows low magnification HAADF-STEM images of the BTO film along the PSO \({\left[002\right]}_{{{{{{\rm{O}}}}}}}\,\)zone-axis. Ti displacements are represented by arrows in Fig. 3b, c, e, f, where the size of the arrows corresponds to the magnitude of atomic displacement in each unit cell. The average value of the out-of-plane component of the Ti displacement in the middle region of the film is negligibly small (−0.6 ± 0.9 pm), while strong downward polarization near the interface region (−8.3 ± 1.0 pm) is observed (Fig. 3d). The result of a decreasing out-of-plane polarization towards the middle region of the film is consistent to phase field simulation results as shown in Fig. 2b–d. Remarkably, the BTO film has a unidirectional in-plane Ti displacement (Fig. 3g) along PSO \({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\,\)direction in the middle region of the film (Fig. 3e) and BTO/PSO interface region (Fig. 3f). The average in-plane Ti-displacement for each region is estimated to be 6.0 ± 1.1 pm and 6.0 ± 1.0 pm, respectively, which is comparable to the Ti displacement along the polarization direction in bulk single crystal BTO29. The detailed overall polarization state of the BTO film is described in Supplementary Note 2. Furthermore, to verify the PSO \({\left(110\right)}_{{{{{{\rm{O}}}}}}}\) substrate termination, atomic-resolution energy dispersive spectroscopy (EDS) elemental mapping was performed together with HAADF-STEM imaging at the BTO/PSO interface. Supplementary Fig. 7 clearly shows that the interface configuration is ScO2-BaO. It should be noted that the TEM data distinctly demonstrate that the polarization directions near the interface are downward (Fig. 3c, d), which is originated from the negative polarity of the substrate surface. Consistent results are obtained from the multiple spots of the sample, indicating that the surface of the PSO substrate is uniformly ScO2 terminated. This is not surprising, because after high-temperature annealing of rare-earth scandate substrates for sufficient time, ScO2 is known to be more stable than the corresponding rare-earth oxide at the surface27,30.

Fig. 3: STEM imaging and polarization measurements of BTO/PSO heterostructure.
figure 3

a A low-magnification HAADF-STEM image of the BTO film with a zone axis of PSO \({[002]}_{{{{{{\rm{O}}}}}}}\). b, c High resolution images taken from selected areas (red and blue boxes in Fig. 3(a), respectively), shows out-of-plane component of Ti-displacement in the middle region (b), and near the interface region (c) of the BTO film, respectively. Note that the size of arrows corresponds to the amount of Ti-displacement. d Average value of out-of-plane Ti-displacement in each layer of BTO from the interface. e, f, High resolution images showing in-plane component of Ti-displacement in the middle region (e), and near the interface region (f) of the BTO film. g Average in-plane Ti-displacement in each layer of BTO from the interface. Both out-of-plane and in-plane Ti-displacements are extracted from the same regions in the BTO film. The error bars represent the 80% confidence intervals.

Full size image

By ferroelectric polarization hysteresis measurements, we explored the as-grown polarization state of the BTO/PSO heterostructures. The schematics of the electrode configurations for in-plane polarization hysteresis measurements are shown in Fig. 4a. The measurements were performed along two directions: PSO \({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\,\)and PSO \({\left[002\right]}_{{{{{{\rm{O}}}}}}}\). Notably, a well-defined hysteresis loop is observed only when applying electric field parallel to PSO \({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\), clearly showing the existence of ferroelectricity (Fig. 4b). The overall positive slope of the polarization in the field region from −30 to 30 kV cm−1 is attributed to the PSO substrate (Supplementary Fig. 8). The hysteresis loops are off-centered toward the negative field direction, pointing to a large polarization imprint31 mainly due to the asymmetric energy barrier between two polarization directions, i.e., PSO \({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\,\)and PSO\(\,{\left[\bar{1}10\right]}_{{{{{{\rm{O}}}}}}}\). The polarization signal obtained with electric field parallel to PSO \({\left[002\right]}_{{{{{{\rm{O}}}}}}}\) direction reveals that there is no remanent ferroelectric polarization along this direction (Fig. 4c). However, the non-linear and weak hysteresis behavior of the P-E loops imply a possible polarization rotation along the PSO \({\left\langle 002\right\rangle }_{{{{{{\rm{O}}}}}}}\) direction. The origin of this polarization rotation will be discussed later. From the STEM results, we found that the polarization direction of as-grown BTO is along PSO \({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\). This is in good agreement with the observation that there is no hysteresis when we apply a positive electric field toward PSO \({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\) since the polarization of BTO is already saturated in this direction in the as-grown state. Only under negative electric field a hysteresis loop is formed, indicating polarization reversal towards PSO \({\left[\bar{1}10\right]}_{{{{{{\rm{O}}}}}}}\). It should be noted that when the field sweep is completed, the BTO film has its original polarization state, that is, a polarization towards PSO \({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\) is still retained. This is a strong evidence for the in-plane polarization state toward PSO \(=\left[1\bar{1}0\right]_{{{{{{\rm{O}}}}}}}\) in our BTO samples is driven by the interfacial electrostatic potential which is not altered under bias conditions.

Fig. 4: In-plane polarization measurement versus electric field.
figure 4

a Schematics depicting parallel electrodes on the BTO/PSO sample. The 10-nm-thick SrRuO3 patterns which are oriented along two different directions with 50-μm gap are used as electrodes for the measurements. b, c Room temperature hysteresis loop measurements for two parallel electrodes arrangements where the electric field is applied parallel to PSO \({[1\bar{1}0]}_{{{{{{\rm{O}}}}}}}\) (b), and PSO \({[002]}_{{{{{{\rm{O}}}}}}}\) (c) directions, respectively.

Full size image

To better understand the microscopic domain structure, we performed angular-dependent lateral PFM measurements when the BTO film sample was rotated by a discreet angle with the respect to the surface normal (Fig. 5). As-grown BTO does not show any contrast in vertical PFM phase image mode (Supplementary Fig. 9). However, angular dependent lateral PFM measurements show that there are measurable domain features depending on the relative sample-cantilever orientation, where the lateral PFM signal is sensitive to the projected polarization along the axis perpendicular to the cantilever arm. Detailed analysis of the PFM images (Supplementary Fig. 10) reveals that the polarization is mainly along PSO \({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\), consistent with the STEM results (Fig. 3g) and the polarization hysteresis measurements (Fig. 4a). However, there are small periodical zigzag-type changes in the polarization orientation typically within ±15° relative to PSO [1-10]O) with respect to the PSO \({\left[002\right]}_{{{{{{\rm{O}}}}}}}\,\)and \({\left[00\bar{2}\right]}_{{{{{{\rm{O}}}}}}}\) directions resulting in a quasi-stripe domain structure with a lateral periodicity in the order of 50 nm and overall in-plane domain pattern (Fig. 5). The detailed analysis and interpretation on the PFM imaging of domain structure along PSO \({\left\langle 002\right\rangle }_{{{{{{\rm{O}}}}}}}\,\)are described in Supplementary Note 3.

Fig. 5: Angular dependent lateral PFM images and an overall in-plane polarization configuration.
figure 5

a–e Lateral PFM phase images with various relative sample-cantilever arm angles of 0° (a), 60° (b), 75° (c), 90° (d), and 105° (e). The inset shows the orientation of the cantilever with respect to the crystallographic directions of the PSO substrate. Note that the angle is defined by the angle between the polarization projection axis and PSO \({[1\bar{1}0]}_{{{{{{\rm{O}}}}}}}\) direction. f In-plane domain structure constructed from PFM images of a–e. The dashed arrows show two orthogonal in-plane components of in-plane polarization (solid arrows), g, Overall configuration of in-plane polarization in the BTO film. Designed single ferroelectric variant is achieved along PSO \({[1\bar{1}0]}_{{{{{{\rm{O}}}}}}}\) direction, while there are unavoidable extra variants along PSO \({\left\langle 002\right\rangle }_{{{{{{\rm{O}}}}}}}\,\)directions due to absence of suitable single crystal substrates.

Full size image

Discussion

The presented data highlights that in-plane polarized single-domain BTO thin film is achievable by control of anisotropic strain, monoclinic distortion due to structural mismatch at an interface, and interfacial valence mismatching. Near the BTO/PSO interface, presence of both in-plane and out-of-plane polarization components result in a diagonal direction of polarization whereas the polarization of BTO in the middle region of the film is entirely in-plane (along PSO \({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\)). The out-of-plane polarization gradually decreases through the film thickness (Fig. 3d), while the in-plane polarization magnitude remains almost constant (Fig. 3g). This is fully consistent with structural analysis showing that the BTO films have slight monoclinic distortions towards the BTO \({\left[00\bar{1}\right]}_{{{{{{\rm{pc}}}}}}}\) direction (Supplementary Fig. 17), while there is no tilting along BTO \({\left[0\bar{1}0\right]}_{{{{{{\rm{pc}}}}}}}\) direction (Supplementary Fig. 18). Such structural distortions are in accordance with the monoclinic symmetry probed by optical SHG measurements (Supplementary Figs. 20–22). From the local electron diffraction patterns, the monoclinic tilting angles between BTO \({\left[001\right]}_{{{{{{\rm{pc}}}}}}}\) and BTO \({\left[100\right]}_{{{{{{\rm{pc}}}}}}}\) near the interface region and film surface region are 91.11° and 90.58°, respectively (Supplementary Fig. 17e, f), indicating the structural relaxation far away from the interface, which is also confirmed by geometric phase analysis (GPA) from TEM data (Supplementary Note 4).

We now discuss the polarization rotation along the PSO \({\left\langle 002\right\rangle }_{{{{{{\rm{O}}}}}}}\) direction. This is mainly due to the absence of a suitable single crystal substrate which has the same b (=a) and c lattice parameters of bulk tetragonal BTO. Tensile strain along the PSO \({\left[002\right]}_{{{{{{\rm{O}}}}}}}\,\)as shown in Supplementary Table 1 could give rise to such a non-zero polarization state, leading to the zigzag-type as-grown domain structure (Fig. 5f). The polarization rotation in as-grown state results in non-linear and weak hysteresis behavior in P-E loop with applying electric field along the PSO \({\left[002\right]}_{{{{{{\rm{O}}}}}}}\)direction (Fig. 4c). The origin of non-zero polarization along the PSO \({\left[002\right]}_{{{{{{\rm{O}}}}}}}\)direction is further supported by phase field simulation where pure in-plane single-domain state is achieved by simply eliminating tensile strain along the PSO \({\left[002\right]}_{{{{{{\rm{O}}}}}}}\,\)direction (Supplementary Figs. 13–15). Therefore, we concluded that the strategy presented in this work effectively stabilizes single ferroelectric variant along the PSO \({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\) direction, even though unavoidable extra variants still exist (Fig. 5g).

The strategy we present here is to utilize a structural mismatch and the effect of an out-of-plane parameter (electric field) to manipulate the in-plane properties (polarization) by lowering the symmetry. We have experimentally demonstrated this strategy by preparation of BTO thin films on PSO \({(110)}_{{{{{{\rm{O}}}}}}}\) substrates where quasi-single-domain state along PSO \({[1\bar{1}0]}_{{{{{{\rm{O}}}}}}}\) direction is achieved at room temperature. We anticipate our strategy for reducing undesirable variants also to be generally used for tuning in-plane properties of correlated materials where their emergent states are subject to domain evolutions.

Methods

Sample growth and characterization

The BTO films were grown on \({(110)}_{{{{{{\rm{O}}}}}}}\)-oriented PSO substrates by PLD with in-situ reflection high-energy electron diffraction (RHEED) monitoring (Supplementary Fig. 4a). The BTO ceramic target was ablated using a KrF (248 nm) excimer laser at a repetition rate of 3 Hz with the laser fluence of ~2 J/cm2. The substrate temperature was kept at 680 °C with an oxygen partial pressure of 120 mTorr during the growth. The substrate-to-target distance was 60 mm. In all experiments, \({(110)}_{{{{{{\rm{O}}}}}}}\)-oriented PSO substrates provided by CrysTec with miscut angles of ~0.1° were used. The PSO substrates were soaked in deionized water for 30 min, and then annealed at 1100 °C in an oxygen atmosphere for 3 h.

DFT calculations

Density functional theory calculations were performed using the projector augmented plane-wave method and the Perdew-Burke-Ernzerhof exchange-correlation functional32 as implemented in the Vienna ab-initio simulation package (VASP)33,34. A bilayer structure was employed in the calculations with a ferroelectric (100) BTO layer lying on top of an orthorhombic (110) PSO layer. The theoretical lattice constants of bulk PSO: a = 5.73 Å, b = 5.75 Å, c = 8.13 Å were used. We fixed atomic coordinate of first three layers of PSO to their bulk values and relaxed the rest consisting of one and half layer of PSO and eight layers of BTO (Fig. S2) with the energy convergence limit of 1 \(\times {10}^{-4}\) eV. In the calculations, a kinetic energy cutoff of 340 eV was used for plane-wave expansion and a 4 × 4 × 1 Monkhorst-Pack grid35 of k points was used for Brillouin zone integration.

Phase-field simulations

In the phase-field model, imposed misfit strain is calculated to be anisotropic by using the pseudocubic lattice constants between BTO and PSO. The system is relaxed to reach an equilibrium state by evolving the polarization configuration with the time-dependent Ginzburg-Landau equation28.

The ferroelectric BTO is described by the spatial distribution of the spontaneous polarization \({{{{{\bf{P}}}}}}{(x,y,z)}\), the relaxational kinetics of which follows the time-dependent Ginzburg-Landau equation,

$$\frac{\delta {{{{{\boldsymbol{P}}}}}}}{\partial t}=-L\frac{\delta F}{\delta {{{{{\boldsymbol{P}}}}}}\,}.$$
(1)

Here, \(F\) is the free energy which consists of elastic (\({f}_{{{{{{\rm{elastic}}}}}}}\)), electrostatic (\({f}_{{{{{{\rm{electrostatic}}}}}}}\)), bulk (\({f}_{{{{{{\rm{bulk}}}}}}}\)), and gradient (\({f}_{{{{{{\rm{gradient}}}}}}}\)) energy contributions, and \(L\) is the kinetic coefficient. The total free energy can be described as,

$$F={\int }_{V}({f}_{{{{{{\rm{bulk}}}}}}}+{f}_{{{{{{\rm{elastic}}}}}}}+{f}_{{{{{{\rm{electrostatic}}}}}}}+{f}_{{{{{{\rm{gradient}}}}}}})dV.$$
(2)

More in-depth description of each term in the energy contributions can be found in the references36. The parameters of the model for BTO follows from Li et al.37.

The 3D system is discretized into a \(128\Delta x\times 128\Delta y\times 90\Delta z\) grid with \(\Delta x=\Delta y=\Delta z=1\,{{{{{\rm{nm}}}}}}\). The thickness of the substrate takes up \(30\Delta z\) to accommodate the relaxation of the displacement arising from the domain structure of the 50 nm film. The free surface of the film is described by the traction-free boundary condition on the top while the bottom interface is assumed to be fully coherent. As a result, the prescribed misfit strains between the film and the substrate can be calculated by using the pseudocubic lattice constant \({a}_{{{{{{\rm{BTO}}}}}}}^{{{{{{\rm{pc}}}}}}}={\left({a}_{{{{{{\rm{BTO}}}}}}}^{2}{c}_{{{{{{\rm{BTO}}}}}}}\right)}^{\frac{1}{3}}\,\)of BTO and that of the \({(110)}_{{{{{{\rm{O}}}}}}}\) plane of PSO, i.e., \({\varepsilon }_{{xx}}=\frac{{a}_{{{{{{\rm{PSO}}}}}}}^{\left[\bar{1}10\right]}-{a}_{{{{{{\rm{BTO}}}}}}}^{{{{{{\rm{pc}}}}}}}}{{a}_{{{{{{\rm{BTO}}}}}}}^{{{{{{\rm{pc}}}}}}}}=0.50 \%\) and \({\varepsilon }_{{yy}}=\frac{{a}_{{{{{{\rm{PSO}}}}}}}^{\left[002\right]}-{a}_{{{{{{\rm{BTO}}}}}}}^{{{{{{\rm{pc}}}}}}}}{{a}_{{{{{{\rm{BTO}}}}}}}^{{{{{{\rm{pc}}}}}}}}=0.01 \%\). As the lattices of the film and the substrate on the interface are orthogonal, there is no in-plane shear misfit strain, i.e., \({\varepsilon }_{{xy}}={\varepsilon }_{{yx}}=0.0 \%\). Considering the absence of top and bottom electrodes of the BTO film in experiments, we adopt the open-circuit boundary condition at both top and bottom of the film, i.e., \({{{{{\bf{D}}}}}}\cdot {{{{{\boldsymbol{n}}}}}}=0\), where \({{{{{\bf{D}}}}}}\) is the electric displacement and \({{{{{\boldsymbol{n}}}}}}\) is the normal of the interface plane. The elastic and electric equilibrium equations for the thin-film system are solved following the method developed by Li et al.38. To account for the interfacial effect as predicted by the first-principles calculations, we assume an effective charged layer at the bottom interface with a fixed, uniform charge density \(\rho =-0.5{{{{{\rm{C}}}}}}/{{{{{{\rm{m}}}}}}}^{2}\). The phase-field simulations were performed by using the commercial phase-field package (mupro.co).

STEM measurements

TEM specimens were prepared by mechanical polishing followed by argon ion milling using Gatan PIPS II. STEM HAADF imaging and EDS experiments were carried out on a JEOL JEM-300CF (Grand ARM) equipped with a cold field emission gun and double spherical aberration correctors with a spatial resolution of 0.6 Å operating at 300 KV in Irvine Materials Research Institute at the University of California, Irvine. STEM-HAADF images were taken with the convergence angle of the incident electrons at 32 mrad and the collection angle at 90–165 mrad. EDS mappings were acquired using dual silicon-drift detectors (SDDs). 50 scans (each with a 0.4 ms dwell time) in the same area across the interface were summed. The high resolution HAADF STEM imaging provides spatial resolution adequate to measure the atomic positions of the A and B site cations of BTO. The high frequency noise was removed by applying an annular mask in frequency space, and then the initial peak positions were determined by identifying local maxima and refined by fitting Gaussian curves to obtain the atom center positions. Displacements were calculated as the difference between the center of each cation and the center of mass of its for adjacent neighbors. Strain analysis is based on GPA39 that preinstalled in Gatan Digital Micrograph software.

PFM measurements

PFM measurements were performed on a commercial AFM system (MFP3D, Asylum Research). Conductive Pt/Ir coated probes (PPP-EFM, Nanosensors) were used for imaging, with an ac modulation amplitude of 0.6 V at a frequency around 350 kHz in the resonance enhanced PFM mode. Both in-plane and out-of-plane signal were collected to examine the possible domain structures.

P-E loop measurements

Polarization vs. Electric field (PE) hysteresis loops were measured using a Radiant Technologies Precision Premier II Ferroelectric Testing system with a 4 kV High-Voltage Interface and a 4 kV TREK amplifier. PE loops were performed using a 250–400 Hz frequency, sinusoidal waveform with an amplitude of 150 V. To make contact to the sample electrodes we used micromanipulator probe tips with a diameter of 10 μm.

SHG measurements

To probe the symmetry of BTO film, optical second harmonic polarimetry measurement was performed using a far-field setup as schematically shown in Supplementary Fig. 20. Linear polarized optical femtosecond pulses at \(\lambda\) = 800 nm (100 fs, 1 kHz) with polarization direction \(\varphi\) was focused onto the sample at an incident angle \(\theta\). The X-polarized (\({I}_{2\omega ,X}\)) and Y-polarized (\({I}_{2\omega ,Y}\)) components of second harmonic signal was collected by a photo-multiplier tube behind a band pass filter. Polarimetry measurement on BTO film was performed by rotating the incident polarization \(\varphi\) at each \(\theta\) for two different sample orientations (O1: (S1, S2, S3) = (\({\left[00\bar{1}\right]}_{{{{{{\rm{O}}}}}}}\), \({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\), \({\left[\bar{1}\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\)), O2: (S1, S2, S3) = (\({\left[1\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\), \({\left[001\right]}_{{{{{{\rm{O}}}}}}}\), \({\left[\bar{1}\bar{1}0\right]}_{{{{{{\rm{O}}}}}}}\))). During measurement, incident angles at \(\theta\) = −45°, −30°, −15°, 0°, 15°, 30° and 45° were used.

Symmetry analysis of the SHG polarimetry was performed using an analytical model as described in literatures40. Theoretical fits to all SHG polarimetry data were simultaneously performed by assuming a single ferroelastic domain with monoclinic symmetry of the BTO film, where the SHG coefficients can be written as:

$${d}^{m}=\left(\begin{array}{cc}\begin{array}{ccc}0 & 0 & 0\\ {d}_{21} & {d}_{22} & {d}_{23}\\ {d}_{31} & {d}_{32} & {d}_{33}\end{array} & \begin{array}{ccc}0 & {d}_{15} & {d}_{16}\\ {d}_{24} & 0 & 0\\ {d}_{34} & 0 & 0\end{array}\end{array}\right)$$
(3)