Abstract
The non-trivial topology of three-dimensional topological insulators dictates the appearance of gapless Dirac surface states. Intriguingly, when made into a nanowire, quantum confinement leads to a peculiar gapped Dirac sub-band structure. This gap is useful for, e.g., future Majorana qubits based on TIs. Furthermore, these sub-bands can be manipulated by a magnetic flux and are an ideal platform for generating stable Majorana zero modes, playing a key role in topological quantum computing. However, direct evidence for the Dirac sub-bands in TI nanowires has not been reported so far. Here, using devices fabricated from thin bulk-insulating (Bi1−xSbx)2Te3 nanowires we show that non-equidistant resistance peaks, observed upon gate-tuning the chemical potential across the Dirac point, are the unique signatures of the quantized sub-bands. These TI nanowires open the way to address the topological mesoscopic physics, and eventually the Majorana physics when proximitized by an s-wave superconductor.
Similar content being viewed by others
Introduction
In topological insulator (TI) nanowires1,2,3, the quantum confinement of the electron motion along the circumferential direction is described by the angular-momentum quantum number ℓ. In zero magnetic field, this quantization leads to the gap opening at the Dirac point, and the sub-bands become doubly-degenerate (see Fig. 1a). When a magnetic flux Φ threads along the wire, the energy spectrum is modified in a nontrivial way as described by the following formula (under the simplified assumption of a circular wire cross-section):
Here, vF is the Fermi velocity, Rw is the wire radius, and Φ0 = hc/e is the flux quantum; note that ℓ takes half-integer values \(\pm \!\frac{1}{2},\pm \!\frac{3}{2},\ldots\!\) due to a Berry phase arising from the spin-momentum locking of the TI surface states1. Interestingly, a spin-non-degenerate gapless spectrum is restored when Φ is a half-integer multiple of Φ0; the spin-momentum locking in this gapless sub-band leads to the appearance of Majorana zero modes (MZMs) when the wire is proximitized by an s-wave superconductor3,4. The tunability of the spin-momentum locking with Φ makes the sub-bands described by Eq. (1) a particularly interesting platform for topological mesoscopic physics.
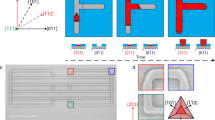
a The sub-band structure of quantum-confined TI surface states described by Eq. (1) (left) and corresponding density of states (right). b SEM image of nanowires (and other nanostructures) grown on a substrate. c TEM image revealing the single-crystalline nanowire body, the remainder of the 20 nm Au-nanoparticle growth catalyst, and a 4-nm thick oxide layer at the nanowire surface. The inset shows the SAED pattern taken in the c-axis direction. TEM image and SAED pattern were taken from different nanowires and directions. d false-color SEM micrograph of device 5 with the schematics of electrical wiring. Pt/Au leads are colored yellow, the nanowire is shown in green. The inset shows a magnified view of the nanowire. e Schematic 3D image of the device construction. Pt/Au leads are illustrated in yellow. The blue and red arrow illustrate the angular momentum ℓ. f R(T) curves of devices 1, 2, 4, and 5.
In experiments, to elucidate the peculiar quantization effects, the TI nanowire should be bulk-insulating and as narrow as possible, preferably less than ~ 100 nm. Past efforts for TI nanowires5,6,7,8,9,10,11,12,13,14,15,16 have only been able to indirectly probe the quantized Dirac sub-bands, although bulk-insulating TI nanowires have been occasionally reported12,16,17,18,19,20,21. In this work, we employed the vapor–liquid–solid (VLS) method using Au nanoparticles as catalysts5 and applied the concept of compensation, which has been useful for achieving bulk-insulation in bulk crystals22,23. Specifically, we tuned the Bi/Sb ratio of \({({{\rm{Bi}}}_{1-{\rm{x}}}{{\rm{Sb}}}_{{\rm{x}}})}_{2}{{\rm{Te}}}_{3}\) nanowires to a value that yields the most insulating properties. Fabrication of gate-tunable four-terminal devices allows us to bring the chemical potential across the Dirac point, upon which we discovered unusual oscillatory behavior in the resistance near the Dirac point in very thin wires. This feature turns out to be the signature of the quantized Dirac sub-bands in TI nanowires as our theoretical calculations show.
Results
Structural and chemical analysis
During the VLS growth, the catalysts form a constantly over-saturated liquid alloy with the absorbed source materials, which then precipitate and form a crystal underneath. Using nominally-20-nm-diameter Au nanoparticles as catalysts, we obtain nanowires with a constant diameter between 20 and 100 nm, with a length of up to several μm (Fig. 1b). By using transmission electron microscopy (TEM) and energy-dispersive X-ray (EDX) analysis (Fig. 1c), we identify the Au catalyst at the tip of most of the analyzed nanowires. The wires are found to be surrounded by a ~4-nm-thick amorphous oxide shell. The selected-area diffraction patterns (SAED, Fig. 1c inset) indicate a high crystalline quality. We found hexagonal symmetry for a direction perpendicular the nanowire axis, which allows us to identify the growth direction to be \(\langle 11\bar{2}0\rangle\)-type. The compositional analysis using EDX along the wire shows a constant stoichiometry (Bi0.68Sb0.32)2Te3 in the nanowire core and no incorporation of Au was detected (See Supplementary Note 2).
Temperature and gate-dependent device resistance
In the following, we report five representative devices 1–5. The scanning electron microscope (SEM) picture of device 5 is shown in Fig. 1d, with its schematic depicted in Fig. 1e. The single-crystalline nanowires are most likely of hexagonal shape (see Supplementary Note 1). The resistance R vs. temperature T curves shown in Fig. 1f present both insulating and metallic behavior; nevertheless, all these samples were bulk-insulating, which can be seen in their gate-voltage VG dependences of R (Fig. 2a–c and Supplementary Fig. 4) showing a clear maximum, indicating that the Dirac point is crossed. The difference in the R(T) dependence is most likely explained by a slightly different electron density n of the samples in the absence of gating (n ≈ 0.38, 0.14, 0.42, − 0.2, 0.5 nm−1 relative to the Dirac point, according to our analysis described later).
In panels a-c, lower curves show the VG dependence of R observed in devices 1-3 at 2 K; ΔVG = 0 corresponds to the Dirac point of the TI surface state, which was achieved with VG of 30.5, 10, and 18.5 V in devices 1, 2, and 3, respectively. For each device multiple consecutive sweeps (9, 54, and 40 for devices 1, 2, and 3, respectively) were performed. For clarity, only 9 of these sweeps are shown for each device as thin lines, together with a single exemplary curve highlighted in bold gray. From the average (colored bold lines) large oscillations of type I are identified (see main text) which arise from the sub-band structure and the corresponding maxima are labeled by arrows. Oscillations of type II are smaller and differ between individual sweeps. Upper curves in a–c are the theoretically calculated resistivity with a small density of impurities, assuming that electron density n is proportional to VG; we used Rw of 20, 16, and 20 nm, and CG of 2.0, 2.2, and 3.6 pF/m for devices 1, 2, and 3, respectively. Pronounced maxima arise at sub-band crossings (dashed lines). d Rescaled position ΔVG/V0 (see main text) of the resistivity maxima of the three devices (open circles) as a function of the quantum number ℓ compared to the theoretically calculated n in units of 2π/Rw (solid line). Crosses indicate the value of n at sub-band minima.
In the R(VG) traces, we found a hierarchy of fluctuation features. We observe semi-oscillatory features in the VG dependence with the amplitude AI ≈ 3, 2 and 1 kΩ for devices 1, 2, and 3, respectively. We will show these features (type I) to be the signature of sub-band crossings. They are not universal conductance fluctuations (UCF) whose main fingerprint would be a strong change in magnetic field and the lack of any clear periodicity and a random amplitude. In contrast, the present features of type I occur in a regular fashion, i.e., at regularly spaced gate voltages and with a largely uniform amplitude. Further, they are robust in small applied magnetic fields (for a detailed discussion on UCF see Supplementary Note 3). The other type of fluctuations have the amplitude AII ≈ 0.5 kΩ (type II) and were changing with time (Supplementary Fig. 3). We speculate that they arise from time-dependent conductance fluctuations due to charge traps or mobile scattering centers, similar to those observed in metallic nanowires of similar mesoscopic size24, but they may also be affected by the presence of electron-hole puddles25,26,27. Averaging over several gate-voltage sweeps suppresses type II fluctuations while type I fluctuations remain unaffected, see Supplementary Note 4.
Model of gate-voltage dependent surface conduction
We now discuss the main observation of this work, that is, the reproducible semi-oscillatory feature in the R(VG) curves. Due to the 1D nature of the energy bands in the nanowire, the density of states (DOS) diverges as \(1/\sqrt{E}\) at each of the sub-band’s edges as shown in Fig. 1a. This causes a sub-band crossing to have two contrasting effects on R: (i) The opening of a new conductance channel can decrease the resistivity as more charge can be transported. It can, however, also (ii) increase the resistivity by opening a new channel where electrons from other bands can scatter into. Thus, we have performed a straightforward theoretical calculation using an idealized model based on the surface state of a circular TI nanowire. (Small anisotropy effects arising both from the hexagonal shape of the wire and the anisotropic electrostatic environment are discussed in the Supplementary Notes 13–16). The effects of local impurities are taken into account using the T-matrix formalism and we find that the experimental data is best described by weak impurities (see Supplementary Note 11). In Fig. 3, we schematically show how different sub-bands contribute to the conductivity: When a new channel is added (Fig. 3b), all other channels scatter efficiently into the new channel and, as a result, the conductivity contribution of each channel drops. This is by far the dominant effect and leads to pronounced peaks in R even when several channels are present. The diverging density of states of the newly added channel (Fig. 1a) is the main reason why this effect is so large, but it is further enhanced by a matrix-element effect originating in the topological protection of the surface states (Supplementary Note 11).
a, b Scattering processes (arrows) between conduction channels for two positions of μ (dashed line). When μ is at the bottom of a sub-band, b all other sub-bands scatter at large rates (bold arrows) with the new sub-band due to its diverging density of states (illustrated by a large red dot), leading to a pronounced minimum in the conductivity. c Theoretically-calculated conductivity as function of μ (parameters as in Fig. 2). Thin black lines display the contribution of each sub-band labeled by \(\ell =\pm \!\frac{1}{2},\pm \!\frac{3}{2},\pm \!\frac{5}{2},\pm \!\frac{7}{2}\), which add up to give the total conductivity (thick blue line). The conductivity of all channels shows pronounced minima at μ = ℓℏvF/Rw, when the chemical potential touches the bottom of a new sub-band.
Hence, our calculations show that the resistance is expected to show a peak, each time a sub-band is crossed. This leads to equidistant peaks in Fig. 3, at μ = ℓℏvF/Rw, when the conductivity is plotted as function of the chemical potential μ. In the experiment, however, the gate voltage VG, rather than μ, is varied and we observe a super-linear dependence of the spacings of the main peaks (neglecting features of type II). This originates from the fact that the effective capacitance of the nanowire devices (which dictates the VG dependence of the accumulated charge) must be computed from their quantum capacitance CQ and geometric (or galvanic) capacitances CG in series where CQ is proportional to the DOS16. In our experiment, CG strongly dominates and the gate voltage directly controls the electron density n (n ≈ CGΔVG/e, with ΔVG measured from the Dirac point), rather than μ. This relation is used for the theory plots in Fig. 2. It also determines the peak positions indicated by dashed lines. We label the position of the peaks identified in the experimental data by the angular momentum quantum number ℓ of the added channel. The influence of the flat bottom gate geometry on the charge homogeneity around the wire is negligible, since it does not affect the position of peaks due to Klein-tunneling physics16 (see Supplementary Note 13). When the chemical potential reaches the bottom of the first electron or the top of the first hole band (\(\ell =\!\pm\! \frac{1}{2}\)), the charge density is approximately zero in both cases and therefore there is only a single peak in the center for \(\ell =\!\pm \!\frac{1}{2}\). For large ℓ, the peak position scales with ℓ2, which is peculiar to the sub-bands of Dirac origin, where the charge density grows as μ2 due to the 2D nature of the TI surface.
Sub-band crossings observed in experiment
It is striking that in Figs. 2a–c the theory can reproduce the essential features of our experiment, in particular the locations of the peaks in the averaged R(VG) curves. While for devices 1 and 2 every peak can be indexed, type II features arising from disorder are more pronounced in device 3, such that some of the sub-band crossing features are not discernible despite averaging. To visualize the agreement between theory and experiment, we plot in Fig. 2d the rescaled gate voltage values of the peaks, ΔVG/V0, vs the sub-band index ℓ, and compare it to the theoretically calculated electron density n at the peak position (in units of 2π/Rw). In these units the rescaling factor is given by \({V}_{0}=\frac{2\pi e}{{R}_{{\rm{w}}}C}\), where C is the capacitance per length of the wire. The super-linear behavior in the VG-dependent sub-band crossings and the excellent agreement of theory and experiment is a direct signature of the quantum-confined Dirac surface states, which is observed here for the first time.
It is prudent to mention that the quantum-confined sub-band structure of TI nanowires have been indirectly inferred5,6,7,8,9,10,11,12,13,14,15,16 from the Aharonov–Bohm (AB)-like oscillations of R as a function of the axial magnetic flux Φ, which is due1 to a periodic change in the number of occupied sub-bands at a given μ. In particular, the observation by Cho et al.12 that R at Φ = 0 takes a maximum when μ is near the Dirac point and changes to a minimum at some other μ was consistent with the gapped Dirac cone; however, the VG dependence was not very systematic nor convincing in ref. 12. A relatively systematic VG dependence of R was recently reported for HgTe nanowires and was carefully analyzed16; unfortunately, the Dirac point of HgTe is buried in the bulk valence band, hindering the characteristic super-linear behavior in the ΔVG vs ℓ relation from observation.
Discussion
The realization of very thin, bulk-insulating TI nanowires and the observation of the quantum-confined Dirac sub-band structure reported here is crucial for exploring the mesosocpic physics associated with the topological surface states, not to mention their potential for future studies of MZMs. For example, the dependence of the spin degeneracy on the magnetic flux along the nanowires will give us a new tuning knob for mesoscopic transport phenomena, in which the spin-momentum locking can be varied. Also, it is an interesting insight that the charge inhomogeneity induced by gating on TI nanowires will not affect the energy locations of the sub-band crossings due to Klein-tunneling physics. Therefore, the new-generation TI nanowires realized here will open vast opportunities for future studies of topological mesoscopic physics including MZMs.
Methods
Nanowire synthesis
The \({({{\rm{Bi}}}_{1-{\rm{x}}}{{\rm{Sb}}}_{{\rm{x}}})}_{2}{{\rm{Te}}}_{3}\) nanowires were synthesized by the VLS method using powders of Bi2Te3 and Sb2Te3 as starting materials in a two-zone 50-mm tube-furnace under a constant Ar flow. The Si/SiO2 substrates were first decorated with suspended 20-nm Au-nanoparticles with the help of Poly-L-Lysine solution and then placed between the two zones (set to temperatures T1 and T2) of the furnace. The temperature was first ramped to T1 = 500–510 ∘C and T2 = 280 ∘C within 60 min, kept at these values for 60 min, and finally reduced back to ambient temperature in roughly 4 h, while keeping a constant Ar flow of 600 SCCM.
Device fabrication
Our gate-tunable four-terminal devices were fabricated on degenerately-doped Si wafers covered by 280-nm thermally-grown SiO2 which acts as a gate dielectric. Gold contact pads and a coordinate system were pre-defined by optical lithography. The as-grown nanowires were transferred by gently bringing together the surfaces of the pre-patterned wafer and the growth substrate, and nanowires suitable for device fabrication were identified by optical microscopy. Per device, five to seven contacts with varying distances were defined by electron beam lithography, which was performed by exposing a PMMA A4 resist layer using a Raith PIONEER Two system. The contact area was cleaned using gentle oxygen plasma treatment and a dip in dilute hydrochloric acid shortly before metallization. Subsequently, 5-nm-thick Pt was sputter-deposited as a wetting layer and an additional 45-nm-thick Au layer was deposited by thermal evaporation (devices 4 & 5) or by sputtering (devices 1, 2, and 3), resulting in the structure schematically shown in Fig. 1e. The contact resistance was well below 1 kΩ for all of the devices. Following the transport measurements, SEM was used to determine the device geometry and the nanowire diameter. The distance between the centers of the voltage contacts were 0.5, 0.8, 1.0, 1.2, and 0.7 μm, and the diameter of the nanowires were 41, 32, 41, 43, and 29 nm for devices 1–5, respectively.
TEM analysis
TEM micrographs, as well as TEM diffraction patterns, were recorded by using a JEM 2200-FS (JEOL) microscope operated at an acceleration voltage of 200 kV. A carbon film supported by a standard copper grid was used as sample carrier for TEM characterization. Elemental chemical analysis of the samples was done by Energy-Dispersive X-ray Analysis (EDX) performed with a JEOL Dry SD100GV detector.
Measurements
Transport measurements were performed in a liquid-helium cryostat in the temperature range of 2–300 K. The wafers were glued onto copper sample holders and manually bonded with 50-μm gold wires using vacuum-cured silver paste. For fast measurements, we used a quantum transport measurement system (SPECS Nanonis Tramea) in the low-frequency lock-in mode with the ac current of 100 nA at the frequency f ≈ 17 Hz, while the device is configured in a conventional four-(device 3, 4, & 5) or three-(device 1 & 2) terminal geometry. Gate-voltage sweeps were performed at various rates from 0.0125 V/s to 0.25 V/s while monitoring the sample temperature with a dedicated thermometer using a low-power AC resistance bridge (Lakeshore Model 370).
Theoretical calculations
We consider the surface states of a quantum wire described by the 2D Dirac equation where antiperiodic boundary conditions in the transverse direction arise from curvature-induced Berry phase effect1. Disorder is modeled by a small density of randomly located local scattering potentials, which is treated within a (non-self-consistent) T-matrix approximation, which can be calculated in a fully analytic way, see Supplementary Note 9. Within our approximation, qualitative features are independent of the density of impurities; however, they do depend on the amplitude of the scattering potential. The Kubo formula was used to calculate the conductivity. Vertex corrections were ignored as a previous study showed that they have only a small, purely quantitative effect28. Plots of resistivities were obtained from ρ = 1/(σ0 + σ(μ)), where σ0 is mainly used to avoid the divergence of ρ when σ = 0. It describes the presence of conductance contributions (e.g., from impurity bands on the surface or in the bulk) not taken into account in our approximation. Note that, although the experimental data are shown in resistance R, the theory calculates the resistivity ρ, because the transport is assumed to be in the diffusive regime. The dependence of μ on ΔVG is computed from n(μ) = CGΔVG/e, where n(μ) is the electron density along the wire and CG is treated as a fitting parameter. Full details of our calculations including a discussion of effects arising from deviations of the circular shape of the wire are given in Supplementary Notes 13–16.
Data availability
The experimental data that support the findings of this study are available in figshare with the identifier doi:10.6084/m9.figshare.13524050 (ref. 29).
References
Zhang, Y. & Vishwanath, A. Anomalous Aharonov-Bohm conductance oscillations from topological insulator surface states. Phys. Rev. Lett. 105, 206601 (2010).
Bardarson, J. H., Brouwer, P. W. & Moore, J. E. Aharonov-Bohm oscillations in disordered topological insulator nanowires. Phys. Rev. Lett. 105, 156803 (2010).
Cook, A. & Franz, M. Majorana fermions in a topological-insulator nanowire proximity-coupled to an s-wave superconductor. Phys. Rev. B 84, 201105 (2011).
Alicea, J. New directions in the pursuit of majorana fermions in solid state systems. Rep. Prog. Phys. 75, 076501 (2012).
Peng, H. et al. Aharonov-Bohm interference in topological insulator nanoribbons. Nat. Mater. 9, 225 (2009).
Xiu, F. et al. Manipulating surface states in topological insulator nanoribbons. Nat. Nanotechnol. 6, 216–221 (2011).
Tian, M. et al. Dual evidence of surface dirac states in thin cylindrical topological insulator Bi2Te3 nanowires. Sci. Rep. 3, 1212 (2013).
Hamdou, B., Gooth, J., Dorn, A., Pippel, E. & Nielsch, K. Surface state dominated transport in topological insulator Bi2Te3 nanowires. Appl. Phys. Lett. 103, 193107 (2013).
Safdar, M. et al. Topological surface transport properties of single-crystalline SnTe nanowire. Nano Lett. 13, 5344–5349 (2013).
Hong, S. S., Zhang, Y., Cha, J. J., Qi, X.-L. & Cui, Y. One-dimensional helical transport in topological insulator nanowire interferometers. Nano Lett. 14, 2815–2821 (2014).
Bäßler, S. et al. One-dimensional edge transport on the surface of cylindrical BixTe3−ySey nanowires in transverse magnetic fields. Appl. Phys. Lett. 107, 181602 (2015).
Cho, S. et al. Aharonov-Bohm oscillations in a quasi-ballistic three-dimensional topological insulator nanowire. Nat. Commun. 6, 7634 (2015).
Arango, Y. C. et al. Quantum transport and nano angle-resolved photoemission spectroscopy on the topological surface states of single Sb2Te3 nanowires. Sci. Rep. 6, 29493 (2016).
Jauregui, L. A., Pettes, M. T., Rokhinson, L. P., Shi, L. & Chen, Y. P. Magnetic field-induced helical mode and topological transitions in a topological insulator nanoribbon. Nat. Nanotechnol. 11, 345–351 (2016).
Bhattacharyya, B., Sharma, A., Awana, V. P. S., Senguttuvan, T. D. & Husale, S. FIB synthesis of Bi2Se3 1D nanowires demonstrating the co-existence of Shubnikov-de Haas oscillations and linear magnetoresistance. J. Phys. Condens. Matter 29, 07LT01 (2017).
Ziegler, J. et al. Probing spin helical surface states in topological HgTe nanowires. Phys. Rev. B 97, 035157 (2018).
Hong, S. S., Cha, J. J., Kong, D. & Cui, Y. Ultra-low carrier concentration and surface-dominant transport in antimony-doped Bi2Se3 topological insulator nanoribbons. Nat. Commun. 3, 757 (2012).
Wang, Z., Qiu, R. L. J., Lee, C. H., Zhang, Z. & Gao, X. P. A. Ambipolar surface conduction in ternary topological insulator Bi2(Te1−xSex)3 nanoribbons. ACS Nano 7, 2126–2131 (2013).
Jauregui, L. A., Pettes, M. T., Rokhinson, L. P., Shi, L. & Chen, Y. P. Gate tunable relativistic mass and Berry’s phase in topological insulator nanoribbon field effect devices. Sci. Rep. 5, 8452 (2015).
Dufouleur, J. et al. Weakly-coupled quasi-1d helical modes in disordered 3d topological insulator quantum wires. Sci. Rep. 7, 45276 (2017).
Kunakova, G. et al. Bulk-free topological insulator Bi2Se3 nanoribbons with magnetotransport signatures of dirac surface states. Nanoscale 10, 19595–19602 (2018).
Taskin, A. A., Ren, Z., Sasaki, S., Segawa, K. & Ando, Y. Observation of Dirac holes and electrons in a topological insulator. Phys. Rev. Lett. 107, 016801 (2011).
Ando, Y. Topological insulator materials. J. Phys. Soc. Jpn. 82, 102001 (2013).
Beutler, D. E., Meisenheimer, T. L. & Giordano, N. Resistance fluctuations in thin Bi wires and films. Phys. Rev. Lett. 58, 1240–1243 (1987).
Borgwardt, N. et al. Self-organized charge puddles in a three-dimensional topological material. Phys. Rev. B 93, 245149 (2016).
Breunig, O. et al. Gigantic negative magnetoresistance in the bulk of a disordered topological insulator. Nat. Commun. 8, 15545 (2017).
Bömerich, T., Lux, J., Feng, Q. T. & Rosch, A. Length scale of puddle formation in compensation-doped semiconductors and topological insulators. Phys. Rev. B 96, 075204 (2017).
Taskin, A. et al. Planar hall effect from the surface of topological insulators. Nat. Commun. 8, 1340 (2017).
Münning, F. et al. Dataset for “Quantum confinement of the Dirac surface states in topological-insulator nanowires”. figshare. https://doi.org/10.6084/m9.figshare.13524050 (2021).
Acknowledgements
This project has received funding from the European Research Council (ERC) under the European Union’s Horizon 2020 research and innovation programme (grant agreement no. 741121) and was also funded by the Deutsche Forschungsgemeinschaft (DFG, German Research Foundation) under CRC 1238—277146847 (Subprojects A04, B01, and C02) as well as under Germany’s Excellence Strategy—Cluster of Excellence Matter and Light for Quantum Computing (ML4Q) EXC 2004/1 - 390534769. O.B. acknowledges the support from the Quantum Matter and Materials Program at the University of Cologne funded by the German Excellence Initiative.
Funding
Open Access funding enabled and organized by Projekt DEAL.
Author information
Authors and Affiliations
Contributions
Y.A. conceived the project. F.M., O.B., D.F., and M.R. performed the growth and device experiments. S.R. performed the TEM analysis. H.F.L., supported by A.R., developed the theory. Y.A., F.M., H.F.L., O.B., and A.R. wrote the manuscript with inputs from all authors.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Peer review information Nature Communications thanks the anonymous reviewer(s) for their contribution to the peer review of this work.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Münning, F., Breunig, O., Legg, H.F. et al. Quantum confinement of the Dirac surface states in topological-insulator nanowires. Nat Commun 12, 1038 (2021). https://doi.org/10.1038/s41467-021-21230-3
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41467-021-21230-3
This article is cited by
-
Quantum confinement detection using a coupled Schrödinger system
Nonlinear Dynamics (2024)
-
Electromagnetic response of the surface states of a topological insulator nanowire embedded within a resonator
Communications Physics (2023)
-
Proximity-induced superconductivity in (Bi1−xSbx)2Te3 topological-insulator nanowires
Communications Materials (2022)
-
Giant magnetochiral anisotropy from quantum-confined surface states of topological insulator nanowires
Nature Nanotechnology (2022)
-
Opportunities in topological insulator devices
Nature Reviews Physics (2021)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.