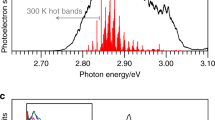
Abstract
Understanding the connection between the motion of the nuclei in a molecule and the rearrangement of its electrons lies at the heart of chemistry. While many experimental methods have been developed to probe either the electronic or the nuclear structure on the timescale of atomic motion, very few have been able to capture both these changes in concert. Here, we use time-resolved photoelectron imaging to probe the isomerisation coordinate on the excited state of an isolated model chromophore anion of the photoactive yellow protein. By probing both the electronic structure changes as well as nuclear dynamics, we are able to uniquely measure isomerisation about a specific bond. Our results demonstrate that the photoelectron signal dispersed in time, energy and angle combined with calculations can track the evolution of both electronic and geometric structure along the adiabatic state, which in turn defines that chemical transformation.
Similar content being viewed by others
Introduction
Chemistry can be broadly defined as the change in valence electronic structure as atoms in a molecule geometrically rearrange. The adiabatic picture that describes this delicate interplay between electrons and nuclei is a central pillar to chemical dynamics and leads to the concept of a potential energy surface within the Born-Oppenheimer approximation1. Consequently, developing experimental probes that are sensitive to both nuclear and electronic evolution in real-time has become a primary goal in chemical physics2,3,4,5,6. Time-resolved photoelectron spectroscopy is one such method as it maps an adiabatically evolving wavepacket onto ionised final states with no strict selection rules7,8. A variant of the method, time-resolved photoelectron imaging, offers an additional dimension through the photoelectron angular distributions (PADs) that are sensitive to the molecular orbital from which the electron is removed9. Adiabatic changes can be tracked through molecular-frame PADs, but such measurements require a connection between the laboratory and molecular frames of reference, either through coincidence measurements2,5 or molecular alignment10. This complexity inhibits the application of such experiments to complex polyatomic molecules for which the methods are ultimately designed. Overcoming these limitations will provide a platform to probe chemical dynamics in complex molecules.
The photoactive yellow protein, PYP, is a blue-light absorbing protein that has been an important testbed for novel structural probes in complex biological environments11,12. The absorption maximum for the S1 ← S0 transition in PYP is at ~450 nm and can be traced to a small chromophore that undergoes a light-activated trans–cis isomerisation which serves as a mechanical lever and triggers an extensive bio-cycle with numerous intermediates13,14,15. Derivatives of the PYP chromophore are commonly based on para-coumaric acid and have been studied extensively as a prototypical bio-chromophore16,17. Yet, there remains ambiguity about which specific bonds are involved in the initial excited state isomerisation and, hence, there is a desire to develop experimental probes that can distinguish subtly different reaction coordinates. For example, the anionic para-coumaric ketone chromophore (pCK−, Fig. 1a), studied by Zewail and coworkers using time-resolved photoelectron spectroscopy, can isomerise about the first (single), the second (double), or both bonds in the para-position; but the photoelectron spectra alone could not discern these differences16. Chemical derivatives in which rotation about specific bonds is inhibited have also been studied, but such modifications diverge further from the native chromophore18. Several computational studies have explored the potential energy surfaces of the S0 and S1 states and considered the dynamics on the S1 state following photoexcitation19,20,21,22,23. These have converged on the position that the initial isomerisation coordinate involves predominantly rotation about the single bond, but have not been clearly linked with experimental data. In the present study, pCK− is probed by time-resolved photoelectron imaging combined with electronic structure calculations. For specific photoelectron features, the temporal evolution of the spectra and laboratory-frame PADs, in unison with our calculations, enables the identification of the nuclear and electronic structural changes associated with the single-bond isomerisation coordinate on the excited state, thus demonstrating a direct probe for adiabatic dynamics, i.e. chemistry.
a Structure of pCK− with arrows indicating single (φSB) and double (φDB) bond rotation. b Time-resolved photoelectron spectra plotted as a normalised false-colour map with a t < 0 spectrum subtracted and where white indicates signal <0. c time-resolved β2 false-colour map, where values are omitted whenever the signal level is <0.1 in b. d Integrated photoelectron signal over spectral windows ε1, ε2, and ε3 indicated in b. e Photoelectron spectra at t = 0 and 1 ps, representative of spectral windows ε1 and ε2 in b, respectively. f β2 corresponding to spectra in e as indicated by shaded regions. Vertical arrows in d show the maximum kinetic energy expected for detachment from the Franck-Condon, single-bond, and double bond rotated minima, εFC, εSB, and εDB, respectively.
Results
Time-resolved photoelectron imaging
Our experiment involves excitation of mass-selected pCK− at 2.79 eV (444 nm) with femtosecond pulses to the bright S1 state. The excited state dynamics are subsequently probed at various delays using femtosecond pulses at 1.55 eV (800 nm) at the centre of a velocity map imaging spectrometer, yielding time-resolved photoelectron images. The temporal resolution (full width at half maximum) is 100 ± 10 fs and the spectral resolution ~5% of the electron kinetic energy, ε.
The time-resolved photoelectron spectra are shown in Fig. 1b over the first picosecond following excitation. Each spectrum has had the spectrum at t < 0 removed to leave only the time-evolving signals. Figure 1e displays spectra at a few specific delays. At very early times, the photoelectron spectrum exhibits a peak centred at an electron kinetic energy, ε ~ 1.4 eV. With increasing pump-probe delay, t, this initial peak shifts towards lower ε, leaving a peak centred at ε ~ 0.8 eV at longer times (t >> 1 ps). This peak then slowly decays with a lifetime of ~120 ps with no further spectral evolution (see Supplementary Fig. 1). Based on our photoelectron spectra (Supplementary Note 2 and Supplementary Fig. 2), the electron affinity of pCK is 2.87 ± 0.05 eV. Hence, excitation to the S1 state at 2.79 eV is just below the detachment threshold and ensures we are predominantly probing bound-state dynamics, although the S1 absorption profile is broad and extends into the continuum24.
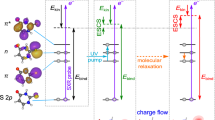
Calculated surface (XMCQDPT2) obtained from a linear interpolation in internal coordinates (LIIC) connecting: (left) the S1 planar minimum (PM) and the S1 minimum along the single-bond (SB) pathway; and (right) the S1 PM and the S1 minimum along the double-bond (DB) pathway. a Energetic analysis of excitation to the S1 state. Structures indicate critical points on the S1 potential energy surface, defined as the Franck-Condon structure (FC) and the S1(SB) and S1(DB) minima. Pump and probe photon energies are included and expected maximum kinetic energies for the resultant photoelectrons detached from the FC (εFC), PM (εPM), SB (εSB) and DB (εDB). b Analysis of the modelled photoelectron angular distributions corresponding to the same regions on the S1 potential energy surface. The Dyson orbitals, ΨD, for SB and DB shown, ΨD(SB) and ΨD(DB), respectively. The Dyson orbitals for FC and PM are very similar and only ΨD(FC) is included. In the structures, carbon atoms are dark grey, hydrogen atoms are light grey, and oxygen atoms are red.
Fig. 1d presents the integrated photoelectron signal over specific spectral windows that are indicated in Fig. 1b (ε1, ε2, and ε3) and are representative of the different spectral features. The high energy peak (represented by spectral region ε1 in Fig. 1b) shows a rapid initial decay with an apparent oscillation superimposed. The signal in the intermediate spectral range (ε2) rises as the high energy signal decays and similarly oscillates with a commensurate period, but with a π phase shift. These dynamics are also clearly visible in Fig. 1b.
In addition to the evolution of the photoelectron spectra, we also observe an evolution of the PADs. Laboratory-frame PADs are typically quantified by anisotropy parameters, β2n(ε)25. For a two-photon (pump-probe) process, n = 1 and 2, and the PADs are defined through9,26
where I(ε, θ) is the photoelectron signal as a function of the angle, θ, between the laser polarisation axis and the photoelectron emission velocity vector, σ is the detachment cross-section, and P2(cosθ) and P4(cosθ) are the second- and fourth-order Legendre polynomials. For large polyatomic molecules, only changes in β2 are often significant, which has limiting values +2 and −1 that correspond to electron emission predominantly parallel to and perpendicular to the polarisation axis, respectively. Figure 1c shows the measured β2(ε, t), with a 5-point moving average in ε. Figure 1c is directly comparable to the spectral evolution shown in Fig. 1b. Note that when the overall photoelectron signal is low, the determination of β2(ε, t) has a large uncertainty and we omit data for signal that is less than 0.1 of the normalised signal in Fig. 1b for clarity. The corresponding β4(ε, t) data are given in the Supplementary Note 3 and Supplementary Fig. 3 and have values very close to zero suggesting that β2(ε, t) is a good measure of the overall PADs.
β2 parameters at t = 0 (a) and t = 1 ps (b) with experimental (black and blue, respectively) and computed (red, orange, and green) values shown. For clarity, the region over which the experimental and computed β2 values are comparable is highlighted in the experimental data. Dyson orbitals corresponding to the critical points on the potential energy surface are shown in Fig. 2b.
Figure 1f shows the β2(ε) with no moving-average applied at two delays, t = 0 and t = 1 ps, with the corresponding spectra shown in Fig. 1e. To determine a specific anisotropy for a given feature, β2(ε) has been averaged over the spectral features as shown by the shaded regions in Fig. 1e. This yields values of β2 = −0.36 ± 0.09 and β2 = −0.11 ± 0.12 for the initial photoelectron peak at centred at ε ~1.4 eV (ε1) and the lower energy peak centred at ε ~0.8 eV (ε2), respectively.
Assignment of photoelectron features
There are two dominant pathways discussed for the initial S1 state dynamics of PYP chromophores19,20,22. The ground state of pCK− is planar because of the π-conjugation over the para-substituent on the phenolate anion. Upon excitation to the S1 state, an electron populates a molecular orbital with π* character, weakening the corresponding π-conjugation of the molecule and facilitating rotation about the bonds. Following S1 photoexcitation, the molecule first rapidly relaxes to a local planar minimum with a geometry that is very similar to the Franck-Condon geometry (i.e. S0 minimum). From this planar S1 minimum, rotation about either the single bond, φSB, or the double bond, φDB, can occur as shown in Fig. 1a.
Figure 2 shows the relevant potential energy surfaces that have been calculated using high-level multireference methods along two different pathways connecting the S1 planar minimum (PM) with the two minima on the S1 surface. These two minima arise from rotation around φSB or φDB and their geometries are denoted as SB and DB, respectively, as shown inset in Fig. 2a. The calculated pathways connect the different minima via a linear interpolation in internal coordinates (LIIC) and as such account for the geometrical changes along the different points of the S1 potential energy surface. While other nuclear displacements take place, the motion along either pathway is dominated by the rotations φSB or φDB. Motion along the φDB involves a barrier, while that along the φSB coordinate is essentially barrierless. Our calculations are in reasonable agreement with previous theoretical work, which suggested that φSB rotation is more probable and also found a barrier along φDB for related chromophores20,22. The main differences with previous theoretical works arise from the levels of theory used. Here, our main goal is to treat the S1 excited state on the same footing as the D0 final state to offer the most reliable energies to compare with the photoelectron spectra that are measured in the experiment. The vertical excitation energies of the S0, S1 and D0 states are obtained from the same XMCQDPT2 calculation (see Computational Details for more information).
The photoelectron spectrum is determined by the difference in energy between the anionic (S1) and neutral (D0) states, as shown in Fig. 2a. Based on the calculated values, detachment of the Franck-Condon geometry (denoted FC in Fig. 2a) with a hv = 1.55 eV probe will lead to electron signal extending to εFC = 1.40 eV. The rapid initial relaxation to the S1 planar minimum (PM) reduces this limit slightly to εPM = 1.34 eV. Rotation about φSB and φDB leading to the S1 minima SB and DB is expected to lead to photoelectron signal extending to εSB = 0.87 eV and εDB = 0.21 eV. These limits are shown in Fig. 1e. It is important to note that the estimated uncertainty in the calculations is ~0.2 eV and that only the molecular geometry at each critical point was used to determine these energies. Additionally, the maxima of the photoelectron peaks are expected to be shifted to slightly lower energy compared to the predicted maximum kinetic energy because the potential energy at the minima is lower than at the initial excitation energy. For example, the predicted maximum signal for rotation about φSB will occur in the range 0.43 < εSB < 0.87 eV. Hence, the calculated maximal values should be used as a guide only. Nevertheless, based on the potential energy surfaces in Fig. 2a, the agreement of the peak at t = 0 with the expected energy for the Franck-Condon (and S1 planar minimum) geometry is excellent. At the later time of t = 1 ps, the broad peak centred at ε ~ 0.8 eV is consistent with a twisted intermediate that has undergone rotation about φSB. This peak is not consistent with rotation about φDB as the spectral maximum of DB is expected at ε < 0.21 eV. Hence, based solely on energetic arguments, the dynamics involving the peaks at ε1 ~ 1.4 eV and ε2 ~ 0.8 eV correspond to dynamics involving rotation about the single bond.
Rotation about specific bonds also leads to differing electronic structures: adiabatically, a change in nuclear configuration is associated with an instantaneous adaptation of the underlying electronic structure. That is to say, the character of the valence orbitals at a given molecular geometry should be reflected in the laboratory-frame PADs and these may be expected to be different for the two different isomerisation pathways. Such changes can be quantitatively analysed by computing the Dyson orbital, ΨD, for the key structures along the reaction coordinate. The Dyson orbital can be thought of as the one-electron wavefunction describing the electron that is being photodetached. Krylov and coworkers have shown that PADs can be conveniently calculated from ΨD yielding computed β2(ε) trends27,28. We have previously shown that computed β2(ε) are in satisfactory agreement with experimental ones for several molecular anions in their ground state, including para-substituted phenolate anions, which pCK− is a derivative of29,30. Moreover, we showed that PADs are also sensitive to subtly differing electronic structure when a short alkyl chain (ethyl) lies either in the plane of the phenolate ring or perpendicular to it30. We have now extended these calculations to predict the β2(ε) for detachment from the S1 excited state of pCK−.
Figure 2b shows ΨD for key critical geometries: the Franck-Condon geometry, ΨD(FC), and the two S1 minima associated with a rotation about φSB and φDB: ΨD(SB) and ΨD(DB). Laboratory-frame PADs were calculated based on these ΨD, with the neutral D0 ground state as the final state. The computed β2 values can be directly compared to the measured values (Fig. 1f). The simplest comparison can be done by averaging the computed β2 values over the same energy range as for the experimental results. This yielded computed anisotropy parameters for key geometries of β2 = −0.48 (FC), β2 = −0.40 (PM), β2 = −0.19 (SB) and β2 = +0.04 (DB). These can be directly compared with experimental values of β2 = −0.36 and β2 = −0.11 for the initial peak at t = 0 and the peak at t = 1 ps. Such a comparison suggests that the signal in ε1 arises from FC and PM, while the signal in ε2 arises from SB. A more useful comparison is based on the trends of β2(ε). From Fig. 3a, the measured β2(ε) for the peak at t = 0 is in reasonable quantitative agreement with the β2(ε) computed from ΨD(FC) and ΨD(PM). From Fig. 3b, β2(ε) for the photoelectron peak at t = 1 ps is in reasonable quantitative agreement with β2(ε) computed from ΨD(SB). In contrast, the agreement for this feature with β2(ε) computed from ΨD(DB) is poor and qualitatively has the wrong sign and trend. Other points along the φDB coordinate, including at the barrier, yielded predominantly positive β2(ε) values, similar to that predicted from ΨD(DB), and thus also qualitatively different to the observed experimental trends. We conclude that the signal in the ε2 spectral range is a direct measure of the single bond pathway rather than the double bond one upon photoexcitation to S1 of pCK− and that the dynamical changes between ε1 and ε2 reflect adiabatic motion between the FC/PM region to the SB minimum on the S1 excited state. This conclusion is consistent with the energetic arguments made earlier.
Overall, the agreement between predicted and measured β2(ε) is almost quantitative, especially given that these are based on single geometries that do not account for other nuclear motions (either thermal or photoinduced), which will tend to make the PADs more isotropic. Moreover, the calculation of the PADs employs some key approximations. In particular, the outgoing wave is treated as a plane wave and thus assumes no interaction of the photoelectron with the neutral core. In the present case, this may be a poor approximation because the neutral pCK core has a large permanent dipole moment. Despite these limitations, the agreement is very good, especially in terms of the trends of β2 with ε as see in Fig. 3.
Inspection of ΨD in Fig. 2b provides intuitive chemical insight about how the PADs reflect the changes in electronic structure along the isomerisation coordinate. Specifically, we have previously used a simple Hückel model to interpret changes in the excited state energies and character for a series of para-substituted phenolate anions29. As pCK− belongs to this family, similar arguments apply here. The S1 and S2 states can be considered as linear combinations of molecular orbitals localised on the phenolate ring and the π-conjugated para-substituent. From Fig. 2b, rotation about φSB leads to a localisation of ΨD(SB) onto the π-conjugated substituent. Locally, ΨD(SB) is therefore associated with a planar π-conjugated system and this is expected to lead to β2 < 0, similar to that predicted for ΨD(FC)26. In contrast, following the rotation about φDB, ΨD(DB) becomes delocalised over a non-planar moiety. Such a molecular orbital is expected to yield β2 ~ 0, as previously seen in the ground electronic state of para-ethylphenolate30. Hence, despite the complex nature of lab-frame PADs, simple arguments provide an intuitive view of the electronic structure changes associated with the isomerisation coordinate. Hence, without the need to perform high-level calculations, the observed PADs can provide qualitative insight into the changes in valence-bonding along the isomerisation coordinate.
Discussion
Based on the spectral and angular distributions, the photoelectron signal at ε1 is assigned to the signature of FC and PM and that at ε2 to the twisted intermediate following rotation about the single bond, SB. The dynamics associated with this evolution is shown in Fig. 1d. The coherence observed shows a nuclear wavepacket moving on the excited state surface from the S1 planar minimum past the SB minimum and back again with a period of ~400 fs. Note that the vibrational modes that comprise this wavepacket are not necessarily the Franck-Condon active modes. The dominant FC modes are likely to stretch the C–C bonds as the excitation involves a π* ← π transition. These are high frequency modes that lead to very rapid dynamics from the FC towards the S1 minimum. This motion then evolves into the modes that lead to isomerisation. The observed oscillation is in agreement with excited state molecular dynamics simulations of pCK− that have predicted a similar oscillation20. Only a single oscillation is observed, presumably as a result of the dephasing to other modes (i.e. internal vibrational energy redistribution).
The time-resolved photoelectron spectroscopy experiment by Zewail and coworkers similarly noted energetic shifts and associated dynamics, following photoexcitation at hv = 3.10 eV16. Excitation at 3.10 eV is above the adiabatic detachment energy and probably also above the barrier to double bond rotation. In their experiments, autodetachment from the S1 state (characterised by electrons at low ε) was a prominent feature, which could swamp any signatures of the dynamics associated with double bond rotation that might have been occurring. We also observe a very small fraction of autodetachment (≲4%), enabled by the finite temperature (~300 K) and the spectral width of the pump pulse. Additionally, Zewail and coworkers observed an oscillation in the high kinetic energy window, similar to that observed in ε1, but not the out-of-phase oscillation at lower energy (ε2), probably because of contamination by autodetachment16. Dynamics involving isomerisation were also observed in a recent study on a closely related PYP chromophore anion in which the ketone is replaced by an ester group31. These dynamics were in competition with internal conversion to a non-valence state of the anion. Such dynamics are not observed here highlighting that even small chemical changes can have a marked impact on the excited state dynamics.
Finally, Fig. 1b and e shows a peak at very low ε (ε3) in the time-resolved photoelectron spectra. This spectral peak could arise from double bond rotation. The maximum expected energy for photodetachment from DB, εDB = 0.21 eV, which would be consistent with this peak. It is not informative to analyse the PADs for this channel because they are at too low kinetic energy, where the PADs are generally expected to be isotropic. However, a number of observations may suggest a different origin of the ε3 signal. Firstly, the formation of the DB minimum involves motion along the φDB coordinate (Fig. 2a) and should lead to photoelectron signals that evolve continuously from FC/PM to the DB minimum; but this is not observed. Secondly, the oscillation frequency of the integrated signal in ε2 and ε3 is essentially identical (Fig. 1d); one might expect that the period of motion to differ slightly between the two coordinates. Thirdly, if this signal was attributed to DB rotation, then the minimum of the photoelectron signal in ε3 would arise because the probe photon energy was insufficient to access the final neutral state (D0)32. In that case, the oscillation should be observable in the total photoelectron signal, but no such changes are seen (Supplementary Fig. 4). Instead, we suspect that the signal in ε3 comes about because the probe can access the first excited state of the neutral, D1. This excited state can be seen in the photoelectron spectrum at higher photon energies (see Supplementary Fig. 2). According to our calculations, the vertical energy difference between the SB intermediate on S1 and the D1 is 1.3 eV, suggesting that it could be accessed with the 1.55 eV probe. Nevertheless, the assignment of this feature remains somewhat uncertain and we cannot exclude that concomitant dynamics about φDB are taking place on the S1 state over the first picosecond. It would be useful to probe the dynamics with a higher energy photon. However, this comes with added complications of possible excitations from the S1 to higher-lying excited states of the anion.
In summary, we have probed the geometric and electronic structure of a polyatomic molecule using time-resolved photoelectron imaging. In combination with calculations beyond the Franck-Condon region, we can identify specific signals that arise from an isomerisation coordinate involving rotation about the single bond in pCK−. The photoelectron signal provides information about changes in the energies of potential energy surfaces along an intramolecular coordinate, while the photoelectron angular distributions capture the changes in electronic structure that arise from such an isomerisation. While we can conclusively identify single-bond rotation, we cannot exclude that double-bond rotation may be occurring also, because its photoelectron signatures are not captured well in the current experiments. To the best of our knowledge, this presents the first study in which lab-frame photoelectron angular distributions have been tracked along a non-dissociative adiabatic coordinate and that have been quantitatively modelled. These methods provide a basis for probing adiabatic dynamics in large molecular systems.
Methods
Experimental details
Experiments were performed on an anion photoelectron imaging spectrometer33. Anions were produced by negative-mode electrospray ionization of pCK in methanol at pH ~ 10 and transferred into vacuum where they were stored in a ring-electrode trap, thermalized to ~300 K, and unloaded into a time-of-flight mass spectrometer at 100 Hz. Mass-selected anions were intersected by a pair of delayed femtosecond pulses at the centre of a velocity-map imaging spectrometer, which monitored the velocity vectors of the emitted photoelectrons. Probe pulses used the fundamental of a Ti:Sapph (450 μJ pulse−1) and pump pulses were generated by 4th harmonic generation of idler of an OPA (5 μJ pulse−1) and interacted with the sample unfocussed (beam diameter ~ 3 mm). Pump and probe polarizations were set parallel to the detector. The temporal instrument response is 100 fs and times are accurate to better than ±10 fs. Raw photoelectron images were analysed using polar onion peeling34, which recovers the 3D electron velocity distribution from the 2D projection measured on the position sensitive detector (see Supplementary Methods and Supplementary Fig. 5). This analysis yields photoelectron spectra and PADs that were calibrated using the photoelectron spectrum of iodide.
Computational details
The energetic minima corresponding to FC(S0), the planar S1 state and SB(S1) and DB(S1) were first located at the SA2-CASSCF(12,11)/6-31G* level of theory (see Supplementary Fig. 6 and Supplementary Table 1)35. Linear interpolation in internal coordinates (LIIC) pathways were obtained to link the different critical points. An LIIC pathway gives the most straightforward path from a given molecular geometry to a different geometry by interpolating a series of geometries in between, using internal (not Cartesian) coordinates (see for example ref. 36). It is important to note that no reoptimisation of the molecular geometries is performed along these pathways, implying that LIIC pathways do not correspond to minimum energy paths, per se. In particular, the barriers observed along LIIC pathways are possibly higher than the actual barriers one would obtain by searching for proper transition states. LIICs, however, offer a clear picture of the possible pathways between critical points of potential energy surfaces and allow to predict photophysical and photochemical processes that a molecule can undergo. The electronic energy of the S1, S2 and D0 states were recalculated at all points along the LIICs using multi-state extended multi-configurational quasi-degenerate perturbation theory (MS-XMCQDPT2)37 to correct for the lack of dynamic correlation at the SA-CASSCF level. The (aug)-cc-pVTZ basis set was used where the augmented function was only affixed to the oxygen atoms38. The D0 was calculated through addition of an orbital characterized by an extremely diffuse p-function (α = 1E–10) in the active space and included in the 6 state averaging procedure to mimic detachment to the continuum39,40,41. A rigid shift was applied to match the S0–D0 energy to the experimentally determined vertical detachment energy of 2.94 ± 0.05 eV at the Franck-Condon geometry. A DFT/PBE0-based one-electron Fock-type matrix was used to obtain energies of MCSCF semi-canonical orbitals used in perturbation theory as done elsewhere39,40,41.
The Dyson orbitals for critical geometries were calculated using EOM-EE/IP-CCSD/6-31+G** 27,28,42,43 and the PADs were modelled using ezDyson v444. EOM-EE-CCSD calculations with the 6-31+G** basis set were also used to determine the vertical excitation energies of the first excited state of the neutral, D1, at the minimum energy geometries on the S1 surface.
The initial SA-CASSCF calculations were performed with Molpro 201245, XMCQDPT2 calculations were carried out using the Firefly quantum chemistry package46 and EOM-EE/IP-CCSD calculations used QChem 547.
Data availability
All data is available at https://doi.org/10.5281/zenodo.3750967.
Code availability
Polar onion peeling is available at https://www.github.com/adinatan/PolarOnionPeeling (Matlab version) or http://www.verlet.net/research.html (Labview version).
References
Born, M. & Oppenheimer, R. Zur Quantentheorie der Molekeln. Ann. Phys. 389, 457–484 (1927).
Geßner, O. et al. Femtosecond Multidimensional Imaging of a Molecular Dissociation. Science 311, 219–222 (2006).
Li, W. et al. Time-resolved dynamics in N2O4 probed using high harmonic generation. Science 322, 1207–1211 (2008).
Peng, P., Marceau, C. & Villeneuve, D. M. Attosecond imaging of molecules using high harmonic spectroscopy. Nat. Rev. Phys. 1, 144–155 (2019).
Hockett, P., Bisgaard, C. Z., Clarkin, O. J. & Stolow, A. Time-resolved imaging of purely valence-electron dynamics during a chemical reaction. Nat. Phys. 7, 612–615 (2011).
Wörner, H. J., Bertrand, J. B., Kartashov, D. V., Corkum, P. B. & Villeneuve, D. M. Following a chemical reaction using high-harmonic interferometry. Nature 466, 604–607 (2010).
Stolow, A., Bragg, A. E. & Neumark, D. M. Femtosecond time-resolved photoelectron spectroscopy. Chem. Rev. 104, 1719–1758 (2004).
Blanchet, V., Zgierski, M. Z., Seideman, T. & Stolow, A. Discerning vibronic molecular dynamics using time-resolved photoelectron spectroscopy. Nature 401, 52–54 (1999).
Reid, K. L. Photoelectron angular distributions. Annu. Rev. Phys. Chem. 54, 397–424 (2003).
Holmegaard, L. et al. Photoelectron angular distributions from strong-field ionization of oriented molecules. Nat. Phys. 6, 428–432 (2010).
Tenboer, J. et al. Time-resolved serial crystallography captures high-resolution intermediates of photoactive yellow protein. Science 346, 1242–1246 (2014).
Kuramochi, H., Takeuchi, S., Kamikubo, H., Kataoka, M. & Tahara, T. Fifth-order time-domain Raman spectroscopy of photoactive yellow protein for visualizing vibrational coupling in its excited state. Sci. Adv. 5, eaau4490 (2019).
Ujj, L. et al. New photocycle intermediates in the photoactive yellow protein from ectothiorhodospira halophila: picosecond transient absorption spectroscopy. Biophys. J. 75, 406–412 (1998).
Wilderen, L. J. G. W. van et al. Ultrafast infrared spectroscopy reveals a key step for successful entry into the photocycle for photoactive yellow protein. Proc. Natl Acad. Sci. USA 103, 15050–15055 (2006).
Hellingwerf, K. J., Hendriks, J. & Gensch, T. Photoactive yellow protein, a new type of photoreceptor protein: will this “yellow lab” bring us where we want to go? J. Phys. Chem. A 107, 1082–1094 (2003).
Lee, I.-R., Lee, W. & Zewail, A. H. Primary steps of the photoactive yellow protein: Isolated chromophore dynamics and protein directed function. Proc. Natl Acad. Sci. USA 103, 258–262 (2006).
Photophysics of Ionic Biochromophores. https://doi.org/10.1007/978-3-642-40190-9 (Springer-Verlag, 2013).
Mustalahti, S. et al. Photoactive yellow protein chromophore photoisomerizes around a single bond if the double bond is locked. J. Phys. Chem. Lett. 11, 2177–2181 (2020).
Groenhof, G. et al. Photoactivation of the photoactive yellow protein: why photon absorption triggers a trans-to-cis isomerization of the chromophore in the protein. J. Am. Chem. Soc. 126, 4228–4233 (2004).
Virshup, A. M., Chen, J. & Martínez, T. J. Nonlinear dimensionality reduction for nonadiabatic dynamics: The influence of conical intersection topography on population transfer rates. J. Chem. Phys. 137, 22A519 (2012).
Virshup, A. M. et al. Photodynamics in complex environments: ab initio multiple spawning quantum mechanical/molecular mechanical dynamics. J. Phys. Chem. B 113, 3280–3291 (2009).
Boggio-Pasqua, M., Burmeister, C. F., Robb, M. A. & Groenhof, G. Photochemical reactions in biological systems: probing the effect of the environment by means of hybrid quantum chemistry/molecular mechanics simulations. Phys. Chem. Chem. Phys. 14, 7912–7928 (2012).
Gromov, E. V., Burghardt, I., Hynes, J. T., Köppel, H. & Cederbaum, L. S. Electronic structure of the photoactive yellow protein chromophore: Ab initio study of the low-lying excited singlet states. J. Photochem. Photobiol. Chem. 190, 241–257 (2007).
Rocha-Rinza, T. et al. Gas phase absorption studies of photoactive yellow protein chromophore derivatives. J. Phys. Chem. A 113, 9442–9449 (2009).
Cooper, J. & Zare, R. N. Angular distribution of photoelectrons. J. Chem. Phys. 48, 942–943 (1968).
Sanov, A. Laboratory-frame photoelectron angular distributions in anion photodetachment: insight into electronic structure and intermolecular interactions. Annu. Rev. Phys. Chem. 65, 341–363 (2014).
Oana, C. M. & Krylov, A. I. Cross sections and photoelectron angular distributions in photodetachment from negative ions using equation-of-motion coupled-cluster Dyson orbitals. J. Chem. Phys. 131, 124114 (2009).
Melania Oana, C. & Krylov, A. I. Dyson orbitals for ionization from the ground and electronically excited states within equation-of-motion coupled-cluster formalism: Theory, implementation, and examples. J. Chem. Phys. 127, 234106 (2007).
Anstöter, C. S., Dean, C. R. & Verlet, J. R. R. Chromophores of chromophores: a bottom-up Hückel picture of the excited states of photoactive proteins. Phys. Chem. Chem. Phys. 19, 29772–29779 (2017).
Anstöter, C. S., Dean, C. R. & Verlet, J. R. R. Sensitivity of photoelectron angular distributions to molecular conformations of anions. J. Phys. Chem. Lett. 8, 2268–2273 (2017).
Bull, J. N., Anstöter, C. S. & Verlet, J. R. R. Ultrafast valence to non-valence excited state dynamics in a common anionic chromophore. Nat. Commun. 10, 5820 (2019).
Rogers, J. P., Anstöter, C. S. & Verlet, J. R. R. Ultrafast dynamics of low-energy electron attachment via a non-valence correlation-bound state. Nat. Chem. 10, 341–346 (2018).
Lecointre, J., Roberts, G. M., Horke, D. A. & Verlet, J. R. R. Ultrafast relaxation dynamics observed through time-resolved photoelectron angular distributions. J. Phys. Chem. A 114, 11216–11224 (2010).
Roberts, G. M., Nixon, J. L., Lecointre, J., Wrede, E. & Verlet, J. R. R. Toward real-time charged-particle image reconstruction using polar onion-peeling. Rev. Sci. Instrum. 80, 053104 (2009).
Boggio-Pasqua, M. & Groenhof, G. On the use of reduced active space in CASSCF calculations. Comput. Theor. Chem. 1040–1041, 6–13 (2014).
Hudock, H. R. et al. Ab initio molecular dynamics and time-resolved photoelectron spectroscopy of electronically excited uracil and thymine. J. Phys. Chem. A 111, 8500–8508 (2007).
Granovsky, A. A. Extended multi-configuration quasi-degenerate perturbation theory: the new approach to multi-state multi-reference perturbation theory. J. Chem. Phys. 134, 214113 (2011).
Dunning, T. H. Gaussian basis sets for use in correlated molecular calculations. I. The atoms boron through neon and hydrogen. J. Chem. Phys. 90, 1007–1023 (1989).
Anstöter, C. S., Gartmann, T. E., Stanley, L. H., Bochenkova, A. V. & Verlet, J. R. R. Electronic structure of the para-dinitrobenzene radical anion: a combined 2D photoelectron imaging and computational study. Phys. Chem. Chem. Phys. 20, 24019–24026 (2018).
Bochenkova, A. V. et al. UV excited-state photoresponse of biochromophore negative ions. Angew. Chem. Int. Ed. 53, 9797–9801 (2014).
Bochenkova, A. V. et al. Mechanism of resonant electron emission from the deprotonated GFP chromophore and its biomimetics. Chem. Sci. 8, 3154–3163 (2017).
Frisch, M. J., Pople, J. A. & Binkley, J. S. Self‐consistent molecular orbital methods 25. Supplementary functions for Gaussian basis sets. J. Chem. Phys. 80, 3265–3269 (1984).
Krishnan, R., Binkley, J. S., Seeger, R. & Pople, J. A. Self‐consistent molecular orbital methods. XX. A basis set for correlated wave functions. J. Chem. Phys. 72, 650–654 (1980).
Gozem, S. & Krylov, A. I. ezDyson, http://iopenshell.usc.edu/downloads/ezdyson.
Werner, H.-J., Knowles, P. J., Knizia, G., Manby, F. R. & Schütz, M. Molpro: a general-purpose quantum chemistry program package. WIREs Comput Mol. Sci. 2, 242–253 (2012).
Granovsky, A. A. Firefly version 8, http://classic.chem.msu.su/gran/firefly/index.html.
Shao, Y. et al. Advances in molecular quantum chemistry contained in the Q-Chem 4 program package. Mol. Phys. 113, 184–215 (2015).
Acknowledgements
We thank James Bull and Anastasia Bochenkova for allowing access to computing facilities and Anna Krylov for helpful discussions regarding Dyson orbital calculations. This work has been funded by the European Research Council under Starting Grants 306536 and 803718.
Author information
Authors and Affiliations
Contributions
J.R.R.V. conceived the project. C.S.A. performed most of the experiments and calculations with help from J.R.R.V. and B.F.E.C., respectively. C.S.A. analysed the data and all discussed the results. J.R.R.V. wrote the paper with input from C.S.A. and B.F.E.C.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Peer review information Nature Communications thanks the anonymous reviewers for their contribution to the peer review of this work. Peer reviewer reports are available.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Anstöter, C.S., Curchod, B.F.E. & Verlet, J.R.R. Geometric and electronic structure probed along the isomerisation coordinate of a photoactive yellow protein chromophore. Nat Commun 11, 2827 (2020). https://doi.org/10.1038/s41467-020-16667-x
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41467-020-16667-x
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.