Abstract
The electrochemical N2 fixation to produce ammonia is attractive but significantly challenging with low yield and poor selectivity. Herein, we first used density function theory calculations to reveal adjacent bi-Ti3+ pairs formed on anatase TiO2 as the most active electrocatalytic centers for efficient N2 lying-down chemisorption and activation. Then, by doping of anatase TiO2 with Zr4+ that has similar d-electron configuration and oxide structure but relatively larger ionic size, the adjacent bi-Ti3+ sites were induced and enriched via a strained effect, which in turn enhanced the formation of oxygen vacancies. The Zr4+-doped anatase TiO2 exhibited excellent electrocatalytic N2 fixation performances, with an ammonia production rate (8.90 µg·h−1·cm−2) and a Faradaic efficiency of 17.3% at −0.45 V versus reversible hydrogen electrode under ambient aqueous conditions. Moreover, our work suggests a viewpoint to understand and apply the same-valance dopants in heterogeneous catalysis, which is generally useful but still poorly understood.
Similar content being viewed by others
Introduction
The production of ammonia (NH3) by the well-known Haber‒Bosch process from N2 and H2 has marked over a century of success for providing > 80% nitrogen source for fertilizer and an alternative energy carrier with large energy density1. Despite the natural abundance of N2, the high bond energy of the N≡N triple bond (941 kJ mol−1) prevents it as a reactive form and thus demands a significant amount of the global energy cost annually2. In addition, the use of fossil fuels to produce H2 reactant also leads to a significant level of CO2 release3. The electrochemical N2 fixation (also known as N2 reduction reaction, N2RR) can be processed in ambient conditions and use inexpensive aqueous electrolytes as the proton source, and thus is regarded as a promising alternative approach4. The direct electron transfer from electrode surface to N ≡ N requires overcoming substantially high energy barriers5, so the key to achieve efficient N2 fixation is to develop active catalytic centers that can efficiently reduce the large activation barrier of N ≡ N and promote its dissociation. As an overwhelming level of water molecules exists than solvated N2, the other key to achieve this goal is to enrich the electrocatalytic centers that can favorably proceed with the N2RR over the hydrogen evolution reaction (HER).
Theoretical calculations have suggested that metal sites with low chemical valence are potential to enhance the electron-donating ability to the π* antibonding orbitals of N2 molecule6, which weakens the N≡N bond and drives the eventual cleavage of N2. Some noble metal-based catalysts such as Ru7 and Au8 with stepped surfaces can strongly bind to N2 and the intermediates, and thus can lower the overpotential and increase the rate of the N2RR in an aqueous electrolyte. Earth-abundant compounds4,9, including metal oxides, nitrides and carbides, have also been investigated as electrocatalysts10, with tailorable activities by specific facets11, defects12, vacancies13, or hybrid material interfaces14. Nonetheless, to date, the electrocatalytic N2 fixation is still limited by its low yields and slow kinetics15. The critical understanding and rational tuning the active centers of N2RR electrocatalysts remain as a highly challenging but imperative issue.
Previously, the roles of oxygen vacancies (Vo’s) in transition metal oxides have been extensively discussed16,17,18, and the low-valance dopants have also been suggested to facilitate the formation of Vo’s19, but the knowledge of their contributions to the N2RR is still limited. For example, Li et al. reported that TiO2 with Vo’s can chemisorb and activate N2 molecules16, and the formation of each Vo’s is, in turn, related to the formation of a pair of Ti3+. Hirakawa et al.17 suggested that two Ti3+ ions in adjacent positions, which are inherently created on the surface defects of rutile TiO2 (110) surfaces, behave as active sites for photocatalytic conversion of N2 to ammonia with water. However, more-recent calculations provide different results and indicate that such rutile TiO2 (110) surfaces are unlikely to be the relevant surface for the N2RR20. To date, all the theoretical and experimental studies have not been able to reconcile the discrepancies in which types of TiO2 are the best for the N2RR, or what is the bonding nature of the active centers.
Herein, by means of density function theory (DFT) calculations, we first reveal that two adjacent Ti3+ sites (designated as a bi-Ti3+ pair) on anatase TiO2 (101) can chemically adsorb and activate N2 molecules in a lying-down manner, whereas single or isolated Ti3+ sites cannot. On the other hand, similar bi-Ti3+ pair on rutile (110) surface cannot adsorb and activate N2, unless the strong repulsion between one of its nearest lattice oxygens at the bridge sites and the negatively charged N2 upon activation can be eliminated. Thus, the formation of two adjacent Ti3+ sites on anatase (101) should be the most-effective electrocatalytic centers for N2 fixation.
Accordingly, we develop an experimental strategy for inducing such adjacent bi-Ti3+ sites on anatase (101) surfaces as N2RR active centers, using a dopant-induced Vo formation strategy. Zr4+ is selected to dope in the TiO2 framework, owing to its similar d-electron configuration and oxide structure, as well as its suitable size. As shown in Fig. 1, doping of Zr4+, which has a relatively larger radius of 72 pm (compared with 52 pm of Ti4+)21 into anatase TiO2, can still retain its anatase crystal structure but also exert a tension on the TiO2 framework, which can enhance the formation of Vo. As the oxidation number of Zr4+ is fixed, the newly formed Vo must be associated with the formation of two adjacent Ti3+ sites, which are beneficial to enrich active centers and enhance the N2RR over the HER. In contrast, Ce4+ with a much larger ion radius (106 pm) cannot be incorporated into the TiO2 framework without breaking the original crystal structure, whereas Ce3+ can also be associated with the newly formed Vo’s, thus not contributing to the formation of the bi-Ti3+ pairs as active centers. Electrochemical measurements demonstrate that the Zr4+-doped anatase TiO2 exhibit significant enhanced N2RR performances, including an ammonia formation rate of 8.90 ± 0.17 µg h−1 cm−2 catalyst and a corresponding Faradaic efficiency (FENH3) of 17.3%, significantly surpassing those of undoped TiO2 or Ce4+-doped TiO2, whose efficiencies were limited by their lower Ti3+ densities.
Results and discussion
Theoretical calculations
In order to tune Ti3+-based electrocatalysts for the N2RR, DFT calculations were first carried out to rationally screen different types of Ti3+ for a better understanding of bonding structures of these active centers. The calculations for screening an ideal N2RR electrocatalyst are often based on the following criteria:22,23,24 first, strong chemisorption of N2 molecules; second, effective stabilization of N2H*; and third, destabilization of NH2*. In the present study, we also investigated the capability of forming Vo’s efficiently, as the fourth criterion.
According to the four criteria above, several types of Ti3+ sites were screened for the N2RR, including adjacent bi-Ti3+ pairs on anatase (101) surfaces (designated as A(101)-Vo), rutile (110) surfaces with 1 or 2Vo’s (designated as R(110)-Vo, R(110)-2Vo, respectively), as well as single Ti3+ with four coordination sites on anatase (101) surfaces (Fig. 2). As shown below, the number of the associated Vo’s is critical to discriminate against the active and non-active bi-Ti3+ sites. We utilized density functional theory (DFT) and the computational hydrogen electrode (CHE) approach25 that have been proven useful in understanding various electrocatalytic reactions20,22,26,27,28. More details about how to determine the active sites and the atomic configurations of different Ti3+ sites are further illustrated in the Methods section, and the corresponding results are presented in Fig. 3, Supplementary Fig. 1 and Supplementary Table 1.
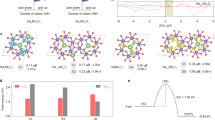
DFT calculations of four types of Ti3+ sites. a The adjacent bi-Ti3+ on anatase (101) surfaces with one oxygen vacancy (i.e., A(101)-Vo); b the single Ti3+ with four coordination on anatase (101) surfaces with one oxygen vacancy; c the adjacent bi-Ti3+ on rutile (110) surfaces with one oxygen vacancy (i.e., R(110)-Vo); d the adjacent bi-Ti3+ on rutile (110) surfaces with a pair of oxygen vacancies together, (i.e., R(110)-2Vo’s). The light-blue spheres stand for the lattice oxygens at the bridge sites where the surface oxygen vacancies are formed most easily. The red spheres stand for the other lattice oxygens on the surfaces and the gray spheres stand for the titanium cations. All four types of the active site models are highlighted by using the green circles. As compared c and d, the bridge lattice oxygen (circled in blue) can exert a large repulsion with the chemisorbed N2 if it adopts a lying-down mode
DFT predicted activity for different types of Ti3+ site. a adjacent bi-Ti3+ on anatase (101) surfaces with oxygen vacancy, b single Ti3+ on anatase (101) surfaces with oxygen vacancy. The light-blue spheres stand for the lattice oxygens at the bridge sites where the surface oxygen vacancies are formed most easily. The red spheres stand for the other lattice oxygens on the surfaces and the gray spheres stand for the titanium cations. ΔG refers to the free energy, and ΔE refers to the electronic energy. The Vo formation energy, ΔE(Vo), was calculated in related to the 1/2 O2 formation as described in the Methods section
The adsorption of N2 molecule is the first step to initialize the N2RR22,23,29. Both the adsorption free energy (ΔG) and the adsorption electronic energy (ΔE) are presented in Fig. 3 and Supplementary Fig. 1. As free N2 has a very stable triple bond, whereas the charge transfer and bond elongation are associated with high energy cost, these quantities are used as a strong evidence to show whether or not N2 is chemisorbed and activated. The A(101)-Vo (i.e., Vo on anatase (101) planes) presents adjacent bi-Ti3+ pairs, which can effectively induce the chemisorption of N2 in a lying-down manner and subsequent activation (Fig. 3a). This is illustrated by the fact that N2 molecule is 0.56 |e| charged (Bader charge30), which makes the N−N bond length elongated from 1.12 Å in an original N2 molecule to 1.18 Å in the chemisorbed state. Similar chemisorption of N2 was also reported on the Vo’s of anatase (010)17. On the contrary, the adjacent bi-Ti3+ pairs on R(110)-Vo (Supplementary Fig. 1a) and single Ti3+ sites on the A(101)-Vo (Fig. 3b) can only adsorb N2 in a standing-up manner, with much less charge transfer and negligible N−N bond length elongation. Furthermore, by comparing the geometry structures between the adsorbed N2 on R(110)-Vo and that on A(101)-Vo, it can be seen that there exists a large repulsion in the former between the adsorbed N2 and one of the adjacent lattice oxygens at the bridge site, owing to a close distance between negatively charged N and the lattice O2‒ (Fig. 2 and Supplementary Fig. 1a). Thus, it is only when such lattice O2‒ is removed and an adjacent bi-Ti3+ pair on R(110)-2Vo is formed, the efficient activation of N2 can occur as shown by a significant amount of negative charge on N2 and an elongated N−N bond length (Supplementary Fig. 1b).
Based on the aforementioned Criterion 2, the first hydrogenation step was further investigated. The results show that the initial N2 adsorption has a large impact on the subsequent hydrogenation. As illustrated in Fig. 3a and Supplementary Fig. 1b, the calculated reaction free energies (ΔG) for the first hydrogenation step on A(101)-Vo and R(110)-2Vo are 0.24 and 0.25 eV, respectively, much lower than those on the single Ti3+ sites (0.50 eV, Fig. 3b) and the adjacent bi-Ti3+ sites on R(110)-Vo (0.75 eV, Supplementary Fig. 1a), whereas N2 is also inactivated in the latter two cases. Our calculation results agree with the previous hypothesis20, and show that R(110)-Vo is unlikely to be the relevant surface for the N2RR. Here, we can now rule out the two configurations of single Ti3+ sites and the adjacent bi-Ti3+ sites on R(110)-Vo, which cannot activate N2 and are unfavorable for the first hydrogenation step.
By examining the Criterion 3, we found that the reaction free energies for the hydrogenation of NH2* on A(101)-Vo (Fig. 3a) and R(110)-2Vo (Supplementary Fig. 1b) are 0.18 and 0.23 eV, respectively. These numbers can be favorably compared with those for the first hydrogenation step. Hence, following the first three criteria, both the adjacent bi-Ti3+ pair sites on A(101)-Vo and R(110)-2Vo are active for the N2RR. However, as shown in Supplementary Fig. 1a and b, the formation of two adjacent Vo’s on rutile (110) surfaces is 0.36 eV higher than the formation of two separated Vo’s (see more discussion in the Methods section). This downplays the role of the adjacent bi-Ti3+ sites on R(110)-2Vo. Hence, the anatase (101) surface is the more suitable support for the development and enrichment of the active bi-Ti3+ pair sites. The feasibility of the N2RR on the adjacent bi-Ti3+ on A(101)-Vo is also confirmed by the whole free energy pathway calculated at 0 and −0.24 V as presented in Supplementary Fig. 2.
Low-valance dopants have often been utilized to facilitate the formation of Vo’s19. However, their incorporation into TiO2 does not guarantee the formation and enrichment of the active bi-Ti3+ sites. On the other hand, the same-valance dopants are generally useful in heterogeneous catalysis19, whereas their roles are less understood. Assuming that the same-valance dopants could introduce strain into the original lattice, we examined how lattice expansion and contraction would change the formation energy of Vo’s. As shown in Supplementary Fig. 3, both the tensile strain and the compressive strain can lower the formation energy of Vo’s in the anatase lattice (see the Methods for more computational details). Considering Ti4+ is the smallest cation for the oxidation state of M4+ (e.g., 72 pm of Zr4+, or 106 pm of Ce4+, as compared with 52 pm of Ti4+)21, introducing the tensile strain is practically feasible. Note that Zr4+ has a similar d-electron configuration and oxide structure, it is thus an ideal replacement of Ti4+. Furthermore, as the oxidation number of Zr4+ is fixed, the newly formed Vo’s are expected to be associated with the formation of two adjacent Ti3+, which are beneficial to the enrichment of the active sites. In contrast, Ce4+ has a much larger ion radius (106 pm), presumably lowering the formation energy of Vo’s to a larger extent. Nevertheless, it is more likely to break the original crystal structure of TiO2 upon Ce4+ doping. Furthermore, Ce4+ can be reduced to Ce3+ during the formation of Vo’s21, and thus does not contribute to the formation of the bi-Ti3+ active centers upon doping.
Synthesis and structural characterizations
TiO2 anatase nanotubes were first synthesized by a hydrothermal method, followed by incubation of Zr4+ or Ce4+ dopants with subsequent annealing (see Methods section). The crystal structures of different samples were investigated by X-ray diffraction (XRD, Fig. 4a). For the undoped and Zr4+-doped TiO2 (designated as Zr-TiO2) nanotubes, all the diffraction peaks correspond to an anatase phase (JCPDS# 21‒1272). The TiO2 peak intensity decreases with the Zr4+ doping, suggesting the slight decrease of TiO2 crystallinity, but no peaks associated with ZrO2 are observed. Close examination of the spectra shows that with the increasing Zr4+ content, the XRD peaks gradually shift toward lower diffraction angles (Fig. 4b), indicating the increase of TiO2 lattice constants upon Zr4+ doping. The corresponding interplanar spacing values of the (101) planes change from 0.351 nm for undoped TiO2 to 0.359 nm for Zr-TiO2. This observation of anatase TiO2 lattice expansion upon the Zr4+ doping is supported by the DFT calculations (Supplementary Table 2 and Supplementary Table 3). In contrast, the Ce-doped TiO2 does not exhibit characteristic peaks of TiO2, but presents peaks that are associated with CeO2 (JCPDS# 43‒1002), suggesting the loss of TiO2 anatase structure and the formation of CeO2 crystals upon the Ce4+ doping. As a comparison, the Zr4+ doping on rutile TiO2 (designated as Zr-rutile-TiO2) was also conducted to illustrate the effect of different active sites. The XRD peaks (Supplementary Fig. 4) confirm the typical rutile phase (JCPDS# 21‒1276).
Structural and compositional characterizations. a X-ray diffraction (XRD) patterns of ZrO2, CeO2, undoped TiO2, Ce-TiO2, and Zr-TiO2. b The enlarged view of XRD pattern at 22o−28o range. c–e High-resolution transmission electron microscopy (HRTEM) images of c undoped TiO2, d Ce-TiO2, and e Zr-TiO2 samples. Insets: Transmission electron microscopy (TEM) images of the corresponding nanotubes. f High-angle annular dark-field scanning transmission electron microscopy (HAADF-STEM) image with corresponding element mappings of Zr-TiO2, showing the distribution of Ti (green), O (blue), and Zr (red). (Source data are provided as a Source Data file.)
High-resolution transmission electron microscopy images show that the undoped (pristine) TiO2 anatase nanotubes have a tubular structure, with the outer and inner diameters of 7–9 and ~ 4 nm, respectively (Fig. 4c). The resolved lattice fringes are measured as 0.351 nm, consistent with the interplanar distance of (101) planes of anatase TiO2. After doping with 5% Ce4+ (i.e., Ce-TiO2, Fig. 4d), small CeO2 nanoparticles are observed on the exteriors of original TiO2 nanotubes, suggesting an inhomogeneous behavior of phase separation. In contrast, for TiO2 nanotubes doped with 5% Zr4+ (i.e., Zr-TiO2, Fig. 4e), no nanoparticles are observed outside the nanotubes. The resolved lattice fringes of 0.359 nm are similar but slightly larger than those of (101) planes of anatase TiO2, also in good accord with the XRD results. The energy-dispersive X-ray spectroscopy (EDS) and mapping confirm the existence and uniform distribution of Ti, O, and Zr elements in the nanotubes (Fig. 4f and Supplementary Fig. 5).
High-angle annular dark-field scanning transmission electron microscopy (HAADF-STEM) was then used to characterize the dopant distribution in different types of TiO2 nanotubes. Compared with the undoped TiO2 nanotubes (Fig. 5a), individual Zr4+ ions are observed to occupy the original positions of Ti4+, exhibiting a distribution of single atoms (highlighted by red circles in Fig. 5b). The electron energy loss spectroscopy (EELS) analysis of Ti-edge was conducted to probe the phase and chemical states of titanium for the undoped, Zr- and Ce-doped TiO2 nanotubes, respectively (Fig. 5c). The undoped TiO2 nanotubes present Ti4+ features with two peaks at 458.4 (Ti-L3) and 463.7 eV (Ti-L2), respectively. For Zr-TiO2, the centers of these two peaks shift to lower energy near 457.5 and 462.7 eV, respectively, indicating that the cation incorporation elevates the content of the unoccupied Ti 3d state as well as the distorted Ti3+ coordination31. Furthermore, a shift of 0.6 eV to lower energy is observed for the Ti-L3 edge in the EELS spectra of Ce-TiO2, associating with the existence of a distorted structure in Ce-TiO2 samples31. Based on the intensity of L2 and L3 from the Zr-TiO2 sample (Supplementary Fig. 6a), the ratio of Ti3+/∑Ti (∑Ti = Ti3+ + Ti4+) is estimated to be 29.1%32. Similarly, the Ti3+/∑Ti values of undoped TiO2 and Ce-TiO2 are calculated as 8.5% and 21.0%, respectively (Supplementary Fig. 6b, c).
Structural and compositional characterizations. High-angle annular dark-field scanning transmission electron microscopy (HAADF-STEM) of a undoped TiO2 and b Zr-TiO2 samples. Single Zr4+ ions were highlighted by red circles. c Electron energy loss spectroscopy (EELS) profiles of the Ti-L2, 3 edge recorded across the undoped TiO2, Ce-TiO2, and Zr-TiO2 samples. d Ti 2p X-ray photoelectron spectroscopy (XPS) spectra of the Zr-TiO2, Ce-TiO2, and undoped TiO2, samples. (Source data are provided as a Source Data file.)
X-ray photoelectron spectroscopy (XPS) was further conducted to study the oxidation states of metal ions on the catalyst surfaces. For the undoped TiO2 nanotubes (Fig. 5d, bottom panel), two main peaks centered at 458.9 and 464.6 eV are observed, corresponding to the Ti 2p3/2 and Ti 2p1/2 peaks, respectively. These two peaks can be further deconvoluted into four sub-peaks, assigned to Ti3+ 2p3/2 (457.4 eV), Ti4+ 2p3/2 (458.9 eV), Ti3+ 2p1/2 (463.1 eV), and Ti4+ 2p1/2 (464.6 eV), respectively, confirming the coexistence of Ti3+ and Ti4+ species33. The ratio between Ti3+ and ∑Ti for undoped TiO2 is calculated as 10%. The incorporation of Zr4+ into TiO2 frameworks leads to a clear shift to lower binding energy direction and widening both peaks (Fig. 5d, top panel), suggesting the increase of the Ti3+ content. The Ti3+/∑Ti ratio is calculated as 31% for Zr-TiO2 nanotubes, in which the increase of Ti3+ percentage is a clear indication of oxygen vacancy increase34. In comparison, the Ti3+/∑Ti ratio for Ce-TiO2 nanotubes is measured as 21% (Fig. 5d, middle panel), which can be attributed to the loss of TiO2 anatase structure, in good accord with XRD results. Accordingly, the analyses of both the Zr 3d peaks (Supplementary Fig. 7a) and Ce 3d peaks (Supplementary Fig. 7b) confirm the existence of Zr4+ and Ce4+/Ce3+ in the Zr-TiO2 and Ce-TiO2 nanotubes, respectively. The defects of unpaired electrons in materials were also probed by electron paramagnetic resonance spectra. Among the three samples (Supplementary Fig. 8), the Zr-TiO2 sample presents the largest signal at g = 2.003, further confirming its largest concentration of Ti3+ ions35.
To further verify their electronic structures, these samples were characterized by X-ray Absorption Near-edge Fine Structure (XANES) spectroscopy. The XANES spectra of Ti K-edge in Zr- or Ce-doped TiO2 samples are similar to that in undoped TiO2 (Supplementary Fig. 9), confirming similar local structure modification of Ti cations36. The main Ti pre-edge peak at 4970.9 eV (indicated by the black arrow) in the three samples (Fig. 6a) is ascribed to the weak symmetry of the surrounding Ti cations in these catalysts37. The slight increase of the pre-edge intensity in both Zr-TiO2 and Ce-TiO2 indicates the existence of more distorted structures and defective Ti environment38. The main peak of Ti K-edge at 4987.6 eV in Zr- or Ce-doped TiO2 samples (Fig. 6b, indicated by the black arrow) is lower than that in pure TiO2 (4987.3 eV), indicating that the Ti species are partially reduced after doping. The bond length information of different samples was further investigated by the Fourier transformed (FT) k3-weighted of Ti K-edge Extended X-ray Absorption Fine Structure (EXAFS) spectra (Fig. 6c). The undoped TiO2 nanotubes show two peaks at 1.39 and 2.41 Å, corresponding to the Ti–O and Ti–Ti bonds, respectively39. Interestingly, the doping of relatively larger Zr4+ in the TiO2 framework results in a contracted Ti–O bond length (1.34 Å) with a larger distribution, suggesting that Zr4+ cations are interstitially incorporated in TiO2 lattice and result in abundant surface defect sites40. This observation is consistent with a previous report that the contracted Ti–O bonds associated with coordinately unsaturated Ti cations acted as Lewis acid sites41.
X-ray absorption spectroscopy characterizations. a, b Enlarged Ti K-edge X-ray absorption near-edge structure (XANES) spectra, and c Fourier transformed (FT) k3-weighted of Ti K-edge Extended X-ray Absorption Fine Structure (EXAFS) spectra of the undoped TiO2, Zr-TiO2, and Ce-TiO2 samples. d Zr K-edge XANES spectra and e Fourier-transformed k3-weighted of EXAFS spectra of pure ZrO2 and Zr-TiO2 samples. (Source data are provided as a Source Data file.)
The Zr K-edge spectra of Zr4+-doped TiO2 nanotubes were also investigated to probe the local structure surrounding Zr cations (Fig. 6d). The main Zr K-edge in the range of 18,020–18,040 eV is split into two peaks at 18,018.5 eV (Peak A) and 18,030.0 eV (Peak B), indicating six-coordinated Zr4+ cations that are consistent with the coordination of Ti4+ in anatase TiO2 structure42. These features are distinctively different from those of pure tetragonal ZrO2 with 7- or 8-coordinated Zr4+ cations43. The EXAFS spectra of Zr-TiO2 (Fig. 6e) only present the bond length of Zr–O (1.52 Å) but not Zr–Zr (3.20 Å), in agreement with the HAADF-STEM results that the Zr4+ cations in the TiO2 framework exhibit a single-atomic distribution.
Electrochemical N2 fixation
The nitrogen temperature-programmed desorption (N2-TPD) was first carried out to evaluate the capability of N2 adsorption by these samples (Supplementary Fig. 10). The two broad peaks centered at 170 and 500 °C are attributed to physisorption and chemisorption of N2, respectively44. Both the undoped TiO2 and Ce-TiO2 show very weak chemisorption peaks. In contrast, the Zr-TiO2 sample presents a strong N2 chemisorption, suggesting that the incorporation of Zr4+ in the anatase TiO2 lattice leads to a significant increase of active sites for N2 adsorption.
The aqueous electrocatalytic N2 reduction was then conducted in an electrochemical cell at room temperature and pressure. N2 gas was supplied in a feed gas stream to the cathode, while 0.1 m KOH aqueous solution was used as the electrolyte (Methods section). All the voltages reported in this work were converted into values versus reversible hydrogen electrode (vs. RHE), as shown in Supplementary Fig. 11. The linear sweep voltammetric (LSV) curves of the Zr-TiO2 nanotubes were first measured in both N2-saturated and Ar-saturated electrolytes, respectively, in the same voltage range (Fig. 7a). A clear current density increase is observed for the N2-saturated electrolyte, suggesting the occurrence of the N2RR11. The thermodynamic equilibrium potential of N2 to NH3 in 0.1 m KOH is calculated as 0.056 V vs. RHE (detailed calculation shown in the Supplementary Note 1), based on the free energies tabulated in literature45. Here, the onset potential of the overall electrochemical reactions is defined as the total current density gets over 50 μA cm‒2. In order to achieve this current density, the onset potentials of the undoped TiO2, Ce-TiO2, Zr-rutile-TiO2, and Zr-TiO2 catalysts are ‒ 0.246, ‒ 0.178, ‒ 0.497, and ‒ 0.141 V vs. RHE, respectively (Supplementary Fig. 12 and Supplementary Fig. 13). For a current density of 1 mA cm‒2 achieved, the undoped TiO2, the Ce-TiO2, Zr-rutile-TiO2, and Zr-TiO2 require ‒ 0.643, ‒ 0.578, ‒ 0.715, and ‒ 0.538 V vs. RHE, respectively. This comparison suggests that the incorporation of Zr4+ should be the main contributor of active sites for catalyzing N2RR. The partial current densities for ammonia production were calculated by multiplying the total current density with the FENH3 at selected potentials (Supplementary Fig. 14). By defining the current density level toward NH3 production as 25 μA cm‒2, the N2RR onset potential for the Zr-TiO2 is calculated as ‒ 0.4 V vs. RHE, corresponding to the overpotential of 456 mV.
Electrochemical N2 fixation. a Linear sweep voltammetric curves in N2-saturated (red line) and Ar-saturated (black line) electrolytes. b Yield of NH3 production (red bars, left y axis) and Faradaic efficiency (green dots, right y axis) of Zr-TiO2 at each given potentials. c 1H nuclear magnetic resonance (NMR) analysis of the electrolyte fed by 15N2 (upper panel) and 14N2 (lower panel) after the electrolytic reactions. d Comparison of the ammonia yield rate (blue bars) and NH3 Faradaic efficiencies (FENH3, green dots) using different feeding gases for the N2RR at − 0.45 V vs. RHE. e Yield of NH3 (blue bars, left y axis) and FENH3 (green dots, right y axis) of undoped TiO2 at each given potentials. f Yields of NH3 with different catalysts at − 0.45 V vs. RHE. (Source data are provided as a Source Data file.)
The average yields of ammonia and the corresponding FENH3 of those electrocatalysts were measured using the sodium salicylate-sodium hypochlorite method13 (Methods section). The corresponding calibration plots were displayed (Supplementary Fig. 15). All samples were measured with over three times to get the average values. The quantification of ammonia was carefully controlled to avoid possible contamination sources46. To rule out the possible contamination of ammonia from the air or the solution, several control experiments were carried out, as specified in Supplementary Fig. 16. Very little ammonia was detected in those controls, and the photographs of their colorimetric assays showed no color difference. In addition, as a control, the electrochemical tests were also conducted for all the electrocatalysts under Ar controls47. The corresponding ultraviolet-visible (UV–Vis) spectra of electrolyte after 3 h electrolysis and chromogenic reaction show the maximum values are comparable to the spectrum backgrounds (Supplementary Fig. 17), suggesting almost no ammonia was produced for all samples in an Ar-saturated electrolyte.
For the electrochemical tests of Zr-TiO2 conducted in N2-saturated electrolytes, the UV–Vis spectra show a significant enhancement of the peak centered around 660 nm, suggesting that the Zr-TiO2 nanotubes catalyze N2 reduction (Supplementary Fig. 18). No N2H4 product is detected in the electrolyte for the Zr-TiO2 catalysts after 3 h N2RR test (Supplementary Fig. 19). For the Zr-TiO2 nanotubes, both the ammonia production rate and the corresponding FENH3 reach their peak values at − 0.45 V vs. RHE, which are calculated as 8.90 ± 0.17 µg h−1 cm−2 catalyst and 17.3% (Fig. 7b), respectively. Further increase the negative potential leads to the decrease of the ammonia production rate and FENH3, which can be attributed to the increase of the competitive HER on the electrode surfaces. The quantitative measurement of the ammonia production was further verified by two other methods, including the ion chromatography (IC) and the nuclear magnetic resonance (NMR)48. The 1H NMR spectra show a triplet coupling (~ 52 Hz) for 14NH4+ and a doublet coupling (~ 72 Hz) for 15NH4+ (Fig. 7c). The obtained ammonia production rate and the corresponding FENH3 for 15N2 as the feeding gas are comparable to those used 14N2 (Fig. 7d and Supplementary Fig. 20), confirming that the ammonia detected is attributed to the electroreduction of N2. The total ammonia produced during the 3 h electrochemical reaction time was calculated as 0.538 μmol (detailed calculation shown in the Supplementary Note 2).
In contrast, all the undoped TiO2 (Fig. 7e), Zr-rutile-TiO2 (Supplementary Fig. 21), and Ce-TiO2 (Supplementary Fig. 22) electrocatalysts exhibit much lower ammonia production rates, with the peak values of 1.48, 3.22, and 5.79 µg h−1 cm−2 catalyst at a higher negative potential of − 0.65 V, respectively. Furthermore, the N2RR tests were also conducted on ZrO2 and CeO2 nanoparticles under the same catalytic potential of − 0.45 V vs. RHE to probe the effect of Zr-incorporation (Fig. 7f). Both the pure CeO2 and ZrO2 nanoparticles exhibit lower or even negligible NH3 production rates. This comparison suggests that the bi-Ti3+ pairs induced by Zr4+-doped TiO2 function as excellent electrocatalytic centers for the N2 fixation at ambient conditions.
Finally, the chronoamperometry tests at selected applied potentials of − 0.5 and − 0.45 V (where the ammonia yields were the highest) show that the N2RR performance of the Zr-TiO2 nanotubes catalyst was stable after several hours of continuous electrolysis (Supplementary Fig. 23). This cycling stability test was further repeated for a total of six runs (Supplementary Fig. 24), showing no obvious change in the NH3 yield rate and current efficiency. After the electrochemical test, the Zr-TiO2 catalyst was re-measured with XPS (Supplementary Fig. 25). No obvious difference is observed compared with that before the electrochemical test, further confirming the good stability of the Zr-TiO2 nanotubes in N2RR electrocatalysis.
In this work, we have first screened several types of Ti3+ sites by means of DFT calculations. The adjacent bi-Ti3+ pairs formed on the most-stable surface of anatase TiO2 (i.e., A(101)-Vo) are identified as the active electrocatalytic centers, which can lead to a lying-down manner as efficient N2 chemisorption and subsequent activation. However, similar bi-Ti3+ pair sites formed on the most stable surface of rutile TiO2 (i.e., R(110)-Vo), as well as the single Ti3+ site, are concluded as the inactive sites.
By further removing a second O2‒ to form R(110)-2Vo, the adjacent bi-Ti3+ pairs on rutile TiO2 can now induce a lying-down chemisorption manner for N2 (Supplementary Fig. 1b), exhibiting a significant amount of negative charge on N2 and an elongated N−N bond length. Nevertheless, as shown in Supplementary Fig. 1a and b, the formation of two adjacent Vo’s on rutile (110) surfaces is 0.36 eV higher than the formation of two separated Vo’s, downplaying the role of the adjacent bi-Ti3+ sites on R(110)-2Vo. Hence the anatase (101) surface is the more suitable host for the development and enrichment of the active bi-Ti3+ pair sites.
The competitive adsorption of H2O and activation of HER on these bi-Ti3+ catalytic sites are also estimated (Supplementary Table 4). For convenience, here we assume the chemical potential of the water in solution is equal to 3.169 kPa as pure liquid water at room temperature. Then, the calculated adsorption free energy changes is only 0.04 eV, when the adsorbed H2O on the bi-Ti3+ sites is replaced by the N2 under working condition (the corresponding structures are presented in Supplementary Fig. 26). For HER, the adsorption free energy of the first hydrogen is calculated to be 0.19 eV on the oxygen vacancy, which is only slightly more advantageous than that of N2RR of 0.24 eV. Meanwhile, one has to take into account the fact that the high pH of the electrolyte (pH 13) has an inhibiting effect on the HER46. Thus, N2 in our situation is able to compete with H2O and H for adsorption and activation as shown in our experiments.
In addition, previous literatures show that if the anatase nanoparticles are exposed under reduction situation with hydrogen, it results in reduced nanoparticles comprising a crystalline TiO2 core and a disordered shell with abundant oxygen vacancies49. With respect to the corresponding pristine surface O*, the calculated free energy diagrams for the further reduced surface OH* and H2O* of pristine A(101) surface, with and without Zr-doping under different potentials, are shown in Supplementary Fig. 27. The result suggests that the surface with O* totally hydrogenated is the most stable under working conditions for URHE < −0.40 eV, such that the oxygen vacancies should remain thermodynamically stable, which should not be annihilated by the O** or OH* species.
As Zr4+ is not a reducible cation, it is not expected to directly form Vo’s next to Zr4+, but our work shows that the doping of Zr4+ into the anatase TiO2 framework is a useful strategy to induce and enrich the specific adjacent bi-Ti3+ pairs on the anatase surfaces. Owing to its similar d-electron configuration and oxide structure but relatively larger ionic size as compared with Ti4+, the doped single Zr4+ ion induces a strained effect without breaking the original TiO2 structure, which, in turn, enhances the formation of oxygen vacancy and subsequently bi-Ti3+ sites on the anatase surfaces. Control experiments reveal that Ce4+ doping does not have the same role as Zr4+ doping, for Ce4+ has a too larger size and a variable oxidation state.
Based on an expanded anatase lattice with lattice constants that are 1.023 times larger than the optimized one by DFT, various anatase A(101) surfaces were built without or with different Zr4+ concentrations in surfaces or subsurfaces. The calculated results for some representatives of the strained A(101) surfaces are shown in Supplementary Table 3. The Vo formation energy without Zr4+ doping is calculated to be 0.27 eV lower than the A(101) surface based on the optimized lattice. With various contents of Zr4+ dopants in surfaces or subsurfaces, the calculated Vo formation energies are within 0.22 and 0.33 eV smaller than that of the optimized lattice. The DFT results support the experiment observation that Zr4+ enhances the Vo formation on A(101) surface, which is owing to the tensile strain induced by the Zr4+ doping.
Owing to the efficient chemisorption and activation of the N2 molecules by the Zr4+ doping-induced bi-Ti3+ pairs on anatase TiO2, the Zr-TiO2 exhibits an outstanding ammonia production rate, a high FENH3, and excellent electrochemical stability, significantly exceeding those of the undoped TiO2 or Ce-TiO2 samples under similar testing conditions. Compared with other N2RR electrocatalysts in aqueous solutions at ambient conditions reported to date (Supplementary Table 5), our Zr-TiO2 sample demonstrated one of the highest NH3 production rates and FENH3 values.
In conclusion, our study demonstrates the bonding nature of the active centers and a unique approach of optimizing electrocatalytically active sites by rational design of dopant size and charge, enabling new opportunities for efficient electrochemical N2 reduction. Our work not only reveals bi-Ti3+ pairs on anatase TiO2 as effective N2RR active centers, but also suggests an attractive viewpoint to understand and apply the same-valance dopants in heterogeneous catalysis, which is generally useful but still poorly understood. Further development of similar multiple active sites with cooperative binding and activation effects on N2 may lead to a vast variety of opportunities of enhancing the N2RR capabilities and potential large-scale utilization toward direct atmospheric N2 fixation.
Methods
Chemicals and materials
Titanium (IV) oxide (P25, Sinopharm Chemical Reagent Co., Ltd, analytically pure), zirconium nitrate pentahydrate (Aladdin, Z190748, 99.5%), cerium nitrate hexahydrate (Aladdin, C105378, 99.5%), sodium hydroxide (Macklin, S817977, ≥ 98%), salicylic acid (Macklin, S817529, 99.5%), potassium sodium tartrate tetrahydrate (Macklin, P816438, 99.5%), sodium nitroferricyanide(III) dehydrate (Macklin, S818341, 99.98% metals basis), sodium hypochlorite solution (Macklin, S828471, available chlorine 4.0%), ammonium chloride (Maclin, A801305, 99.8%), hydrazine monohydrate (Alfa Aesar, A14005, 98%), hydrochloric acid (Sinopharm Chemical Reagent Co., Ltd, 10011018, 36.0−38.0%), potassium hydroxid (Aladdin, P112287, 99.99% metals basis), sulfuric acid (Sinopharm Chemical Reagent Co., Ltd, 10021618, 95.0−98.0%), ethyl alcohol (Sinopharm Chemical Reagent Co., Ltd, 10009218, ≥ 99.7%), 4-(dimethylamino) benzaldehyde (Sigma-Aldrich, 156477, 99%), Nafion solution (Dupont), 211 Nafion membrane (Dupont), deionized (DI) water (Millipore, 18.2 MΩ cm), N2 gas (99.99%), Ar gas (99.99%). Ammonium sulfate ((NH4)2SO4, 99%), (15NH4)2SO4, 98 atom% 15N), and nitrogen-15N2 (98 atom% 15N) were purchased from Sigma-Aldrich. All chemical reagents were used as received without further purification.
Characterization
The XRD data were characterized by Bruker SMART APEX (II)-CCD (Germany). X-ray photoelectron spectroscopy was recorded on a Perkin Elmer PHI 5000 C ESCA system (Perkin Elmer, USA). The high-resolution transmission electron microscopy images and the EDX spectroscopy spectra were recorded by a JEM 2100 F (JEOL, Japan) and a Tecnai T20 (FEI, USA) transmission electron microscope. The HAADF-STEM, EELS, and the EDX mapping experiments were performed using Titan Cubed Themis G2 300 (FEI) microscope equipped with Super-X detectors at 200 kV. N2-TPD measurements were performed on a Micrometrics Autochem II 2920 system. Electron-spin resonance signals were recorded on a Bruker ESR A300 spectrometer at room temperature. XANES and EXAFS data were collected on beamline 14 W at the Shanghai Synchrotron Radiation Facility (SSRF). The UV–Vis absorption spectrum was recorded by an ultraviolet-visible spectrometer (U-3900H, Hitachi, Japan). 1H-NMR (nuclear magnetic resonance) measurements were performed on a Bruker NMR600. IC analysis was performed on an ICS-2000 (Thermo Fisher Scientific) equipped with an isocratic pump.
Synthesis of Zr-TiO2
In a typical synthesis, 2.0 g of TiO2 (P25) was mixed with 60 mL of (10 m) NaOH solution in a Teflon-lined stainless autoclave at 150 °C for 20 h. The slurry was washed with 0.1 m HCl solution for several times until the pH value reached 1.6, and then with DI water until pH was close to 7, before being filtrated to obtain TiO2 nanotubes. The introduction of Zr4+ to TiO2 was conducted by wet impregnating the TiO2 nanotubes with 20 mL of 0.35 m zirconium nitrate solution. The mixture was stirred at room temperature for 4 h. After the reaction, the substrate was washed with DI water and ethanol for several times, followed by drying at 60 °C and then annealed in Ar at 400 °C, with a ramping rate of 2 °C min‒1 for 2.5 h.
Electrochemical measurements
A total of 5 mg of catalyst was dispersed in 0.5 mL of ethanol followed by the addition of 50 µL of Nafion solution. The mixture was sonicated thoroughly to form a homogeneous ink. The working electrodes were then prepared by drop-casting the catalyst inks onto carbon paper to achieve a loading of 1.0 mg cm‒2. All the electrochemical performance measurements were performed with an Autolab electrochemical workstation (Autolab PGSTAT204) at room temperature (25 ± 2 °C) using 0.1 m KOH as the electrolyte. For the electrocatalytic N2RR, a saturated calomel electrode (SCE) and a Pt wire were used as reference and counter electrodes, respectively. The potentials were all converted to the RHE scale according to Nernst equation (1):
where ERHE is the converted potential vs. RHE, ESCE is the experimental potential measured vs. SCE, E0SCE is the standard potential of SCE at 25 °C. The potential of SCE was calibrated to the RHE in 0.1 m KOH electrolyte saturated with high-purity H2 (Supplementary Fig. 11), consistent with the Equation (1). The scan rate for LSV was kept at 1.0 mV s‒1.
For the potentiostatic measurement, the KOH electrolyte (pH 13, 30 mL) was purged with pure N2 for 30 min before the measurement. For comparison, the same electrochemical test was also conducted in an Ar-saturated KOH solution. Constant potential electrolysis was conducted at various potentials for 3 h. The electrochemical experiments were repeated for three times to obtain the averaged measured values.
Determination of ammonia
The concentration of produced ammonia was spectroscopically determined by the indophenol blue method50 with some modification. Sodium salicylate (5 g), sodium hydroxide (1.47 g), and potassium sodium tartrate tetrahydrate (5 g) were dissolved in DI water and diluted to 100 mL was used as the color reagent A. In brief, 8 mL of the testing electrolyte was taken from the electrochemical cell, followed by adding 1 mL of color reagent A, 100 μL of (10 mg/mL) sodium nitroferricyanide (C5FeN6Na2O), and 100 μL of 0.05 m NaClO. The UV-Vis absorption spectra were then measured. The concentration of indophenol blue was determined using the absorbance at 660 nm. The concentration-dependence absorption curves were calibrated using standard ammonia chloride solutions with different concentrations. All the spectroscopic measurements were repeated for three times to obtain the averaged measured values. The fitting curve (y = 0.1066x + 0.0109, R2 = 0.9991) showed good linear relation of absorbance value with NH3 concentrations.
Determination of NH3 yield rate and FE NH3
The electrochemical ammonia yield rate (rNH3 (electrochemical)) was calculated by subtracting the background signal of non-electrochemical ammonia, using Equation (2):
where ΔcNH3 (yield) = cNH3 (yield, N2)−cNH3 (yield, Ar).cNH3 (yield, N2) is the measured NH4+ mass concentration in an N2-saturated electrolyte, cNH3 (yield, Ar) is the measured NH4+ mass concentration in an Ar-saturated electrolyte as a control. Vaq is the volume of electrolyte, t is the electrochemical reaction time, and A is the geometric area of the cathode.
The electrochemical ammonia faradaic efficiency (FENH3 (electrochemical)) was calculated by subtracting the background signal of non-electrochemical ammonia47, using Equation (3):
where n is the number of transferred electrons (3), F is the Faraday constant, and Q is the quantity of applied electric charges.
In order to identify the source of ammonia, 15N2 enriched gas was employed to serve as the feeding gas. After N2 reduction reaction, the NH4+-containing electrolyte was characterized by 1H NMR measurement, using 1 mm maleic acid as an internal standard. 10% dimethyl sulphoxide (DMSO)-d6 was used as the solvent. A doublet coupling (~ 72 Hz) for 15NH4+ and a triplet coupling (~ 52 Hz) for 14NH4+ were observed for different testing solutions.
For the IC method, 3 mL of electrolyte was transferred into the chromatograph. The sample loop was 20 μL, with methanesulfonic acid as the eluent. The concentration-dependence plot by the IC method was characterized with different standard NH4+ concentrations.
Note that specific precautions were necessary with rigorous control experiments to minimize possible ammonia contamination during the N2RR test46. (1) The chemicals including water were purchased or prepared with high purity. (2) All the electrochemical measurements including control experiments were conducted at fume hoods to provide a clean atmosphere. (3) Considering the effect of human respiration and skin contaminates, masks and latex gloves (pre-soaked in 100 mL of 0.01 m HCl for 3 h) were used during the N2RR tests and ammonia quantification measurement.
Determination of hydrazine
The hydrazine presented in the electrolyte was estimated by the method of Watt and Chrisp51. A mixture of para-(dimethylamino) benzaldehyde (5.99 g), HCl (concentrated, 30 mL) and ethanol (300 mL) was used as a color reagent B. In brief, 5 mL of testing electrolyte after the electrocatalytic reaction was taken out from the electrochemical cell, followed by adding 5 mL of color reagent B. After 20 min for color development, the UV-Vis absorption spectra were measured at 455 nm. The concentration-dependence plot was obtained by using standard hydrazine monohydrate solutions with different concentrations. The fitting curve showed good linear relation of absorbance with N2H4·H2O concentration (y = 0.7624x + 0.0161, R2 = 0.9992) by three times independent calibrations.
Computational details
The Vienna ab initio simulation package was utilized to perform all DFT calculations52,53,54. The 2 s, 2p electrons in oxygen and nitrogen, the 3d, 4 s electrons in titanium and the 5 s, 4d, 5p electrons in zirconium were treated as valence electrons, whereas the kinetic energy cutoff for the plane wave basis sets was set to be 400 eV. The remaining core electrons were described by the projector augmented-wave method55. The surface Monkhorst–Pack meshes56 of 2 × 2 × 1 and 5 × 5 × 5 k-point sampling in the surface Brillouin zone were employed for slab model and bulk, respectively. For bulk optimization, all atoms as well as lattice constants were allowed to fully relax. For systems involving anatase (101), a 1 × 3 supercell of 12 atomic layers was used, where the bottom five layers of atoms were fixed in their optimized bulk positions, whereas the top seven layers, as well as the adsorbate, were allowed to fully relax. For systems involving rutile (110), a 3 × 2 supercell of 12 atomic layers was used, where the bottom five layers of atoms were fixed in their optimized bulk positions, whereas the top seven layers, as well as the adsorbate, were allowed to fully relax. After the convergence criteria for optimizations were met, the largest remaining force on each atom was less than 0.02 eV Å‒1. For all calculations, the spin polarized generalized gradient approximation of the Perdew–Burke–Ernzerhof functional57 was used. As the standard DFT functions tended to over-delocalize electrons, DFT + U was employed58 with an effective U value of 3.3 eV for Ti 3d-orbitals, as obtained from linear response59. For the dopants, we used standard DFT on Zr, as there were no issues with describing the electronic structure21. For surface reactions, the contributions of dispersive interactions were accounted for by using the DFT + D3 method with Becke-Jonson damping60,61. Bader’s theory of atoms in molecules was used for charge analysis30,62,63.
Oxygen vacancy formation energies were calculated as:
where Et(TixO2x-1) and Et(TixO2x) are the total energies of the optimized supercell with and without VO vacancy, respectively, and Et(O2) is the total energy of a gas phase O2. The second Vo formation energies, from TixO2x-1 were calculated as:
where Et(TixO2x-2) is the total energies of the optimized supercell with the first and second VO vacancy, respectively. This type of oxygen vacancy formation energy is commonly used in theoretical heterogeneous catalysis, as it is convenient to compare different oxides (doped or undoped) under different reaction conditions18. Indeed, the formation of oxygen vacancies in reducible oxides like TiO2 and CeO2 is relatively easy18, in particular, under the reduction conditions such as H2 or CO atmosphere, or on the anode surfaces as in the present work. The formation energy of oxygen vacancies should be related with H2O or CO2 formation, thus providing the thermodynamic driving force.
The adsorption free energies of gases on the surfaces were calculated as:
where Gt(gas/surface), Gt(surface), and Gt(gas) are the total free energies of the adsorption systems of surfaces with or without vacancies, and adsorbate species in the gas phase, respectively. Here, we assumed that in addition to the total electronic energies, only the translation and rotation contributions of the gas phase species are significant and the other parts can be ignored. Assuming the gas phase species as ideal gases, the partition functions of translation Qtrans and rotation Qrot were calculated as:64
where P (1 atm) and m are the partial pressure and molecular mass, respectively, kB is the Boltzmann constant, T (298.15 K) is the absolute temperature, \(V = \frac{{k_{\mathrm{B}}T}}{{P_{\mathrm{A}}}}\) is the volume of the system, σ is the symmetry factor, Arot, Brot, Crot are rotational constants, and h is the Plank’s constant.
In our work, the DFT calculations were carried out to rationally screen four types of Ti3+ for a better understanding of bonding structures of the active centers (Fig. 2): (a) the adjacent bi-Ti3+ on anatase (101) surfaces with one oxygen vacancy (i.e., A(101)-Vo); (b) the single Ti3+ with four coordination on anatase (101) surfaces with one oxygen vacancy; (c) the adjacent bi-Ti3+ on rutile (110) surfaces with one oxygen vacancy, (i.e, R(110)-Vo); (d) the adjacent bi-Ti3+ on rutile (110) surfaces with a pair of oxygen vacancies together, (i.e., R(110)-2Vo’s). Cases (a) and (c) were chosen because anatase and rutile are the most common crystal phases for TiO2, and anatase (101) and rutile (110) surfaces are, respectively, the most exposed surfaces. Case (b) was chosen for its low coordination. Case (d) was formed after the removal of one nearby lattice O2‒ in Case (c). This nearby lattice O2‒ presents a large repulsion with the chemisorbed N2 in a lying-down manner, which precludes the bi-Ti3+ site of R(110)-Vo from effective N2 activation.
The overall N2RR process (N2 + 6 H+ + 6e‒ → 2NH3) involves several proton-coupled electron transfer steps. The Gibbs free energy change (ΔG) of each elementary step was calculated by using the standard hydrogen electrode model27,28,65, which uses one-half of the chemical potential of hydrogen as the chemical potential of the proton-electron pair. According to this method, the ΔG value can be determined as:
where ΔH and ΔS are the enthalpy change and entropy change, respectively. ΔGU is the free energy contribution related to electrode potential U. ΔGpH is the correction of the H+ free energy by the concentration, which can be calculated as
References
Soloveichik, G. Electrochemical synthesis of ammonia as a potential alternative to the Haber–Bosch process. Nat. Catal. 2, 377–380 (2019).
Deng, J., Iñiguez, J. A. & Liu, C. Electrocatalytic nitrogen reduction at low temperature. Joule 2, 846–856 (2018).
Chen, X., Li, N., Kong, Z., Ong, W. J. & Zhao, X. Photocatalytic fixation of nitrogen to ammonia: state-of-the-art advancements and future prospects. Mater. Horiz. 5, 9–27 (2018).
Cui, X., Tang, C. & Zhang, Q. A review of electrocatalytic reduction of dinitrogen to ammonia under ambient conditions. Adv. Energy Mater. 8, 1800369 (2018).
Mehta, P. et al. Overcoming ammonia synthesis scaling relations with plasma-enabled catalysis. Nat. Catal. 1, 269–275 (2018).
Ma, X. L., Liu, J. C., Xiao, H. & Li, J. Surface cluster catalyst for N2-to-NH3 thermal conversion. J. Am. Chem. Soc. 140, 46–49 (2017).
Ishikawa, A., Doi, T. & Nakai, H. Catalytic performance of Ru, Os, and Rh nanoparticles for ammonia synthesis: a density functional theory analysis. J. Catal. 357, 213–222 (2018).
Bao, D. et al. Electrochemical reduction of N2 under ambient conditions for artificial N2 fixation and renewable energy storage using N2/NH3 cycle. Adv. Mater. 29, 1604799 (2016).
Cao, N. & Zheng, G. Aqueous electrocatalytic N2 reduction under ambient conditions. Nano Res. 11, 2992–3008 (2018).
McPherson, I. J., Sudmeier, T., Fellowes, J. & Tsang, S. C. E. Materials for electrochemical ammonia synthesis. Dalton Trans. 48, 1562–1568 (2019).
Yang, D., Chen, T. & Wang, Z. Electrochemical reduction of aqueous nitrogen (N2) at a low overpotential on (110)-oriented Mo nanofilm. J. Mater. Chem. A 5, 18967–18971 (2017).
Lv, C. et al. Defect engineering metal-free polymeric carbon nitride electrocatalyst for effective nitrogen fixation under ambient conditions. Angew. Chem. Int. Ed. 57, 10246–10250 (2018).
Zhang, Y. et al. High-performance electrohydrogenation of N2 to NH3 catalyzed by multishelled hollow Cr2O3 microspheres under ambient conditions. ACS Catal. 8, 8540–8544 (2018).
Shi, M. M. et al. Au sub-nanoclusters on TiO2 toward highly efficient and selective electrocatalyst for N2 conversion to NH3 at ambient conditions. Adv. Mater. 29, 1606550 (2017).
Chen, G. F. et al. Ammonia electrosynthesis with high selectivity under ambient conditions via a Li+ incorporation strategy. J. Am. Chem. Soc. 139, 9771–9774 (2017).
Li, C. et al. Promoted fixation of molecular nitrogen with surface oxygen vacancies on plasmon-enhanced TiO2 photoelectrodes. Angew. Chem. Int. Ed. 57, 5278–5282 (2018).
Hirakawa, H., Hashimoto, M., Shiraishi, Y. & Hirai, T. Photocatalytic conversion of nitrogen to ammonia with water on surface oxygen vacancies of titanium dioxide. J. Am. Chem. Soc. 139, 10929–10936 (2017).
Yang, J. et al. High-efficiency “working-in-tandem” nitrogen photofixation achieved by assembling plasmonic gold nanocrystals on ultrathin titania nanosheets. J. Am. Chem. Soc. 140, 8497–8508 (2018).
McFarland, E. W. & Metiu, H. Catalysis by doped oxides. Chem. Rev. 113, 4391–4427 (2013).
Comer, B. M. & Medford, A. J. Analysis of photocatalytic nitrogen fixation on rutile TiO2 (110). ACS Sustain. Chem. Eng. 6, 4648–4660 (2018).
Iwaszuk, A. & Nolan, M. Electronic structure and reactivity of Ce- and Zr-doped TiO2: assessing the reliability of density functional theory approaches. J. Phys. Chem. C. 115, 12995–13007 (2011).
Zhao, J. & Chen, Z. Single Mo atom supported on defective boron nitride monolayer as an efficient electrocatalyst for nitrogen fixation: a computational study. J. Am. Chem. Soc. 139, 12480–12487 (2017).
Skúlason, E. et al. A theoretical evaluation of possible transition metal electro-catalysts for N2 reduction. Phys. Chem. Chem. Phys. 14, 1235–1245 (2012).
Höskuldsson, Á. B., Abghoui, Y., Gunnarsdóttir, A. B. & Skúlason, E. Computational screening of rutile oxides for electrochemical ammonia formation. ACS Sustain. Chem. Eng. 5, 10327–10333 (2017).
Calle-Vallejo, F. & Koper, M. T. M. First-principles computational electrochemistry: achievements and challenges. Electrochim. Acta 84, 3–11 (2012).
Man, I. C. et al. Universality in oxygen evolution electrocatalysis on oxide surfaces. ChemCatChem 3, 1159–1165 (2011).
Peterson, A. A., Abild-Pedersen, F., Studt, F., Rossmeisl, J. & Nørskov, J. K. How copper catalyzes the electroreduction of carbon dioxide into hydrocarbon fuels. Energy Environ. Sci. 3, 1311 (2010).
Nørskov, J. K. et al. Origin of the overpotential for oxygen reduction at a fuel-cell cathode. J. Phys. Chem. B 108, 17886–17892 (2004).
Montoya, J. H., Tsai, C., Vojvodic, A. & Nørskov, J. K. The challenge of electrochemical ammonia synthesis: a new perspective on the role of nitrogen scaling relations. ChemSusChem 8, 2180–2186 (2015).
Sanville, E., Kenny, S. D., Smith, R. & Henkelman, G. Improved grid-based algorithm for bader charge allocation. J. Comput. Chem. 28, 899–908 (2007).
Lu, X. et al. Conducting interface in oxide homojunction: understanding of superior properties in black TiO2. Nano Lett. 16, 5751–5755 (2016).
Stoyanov, E., Langenhorst, F. & Steinle-Neumann, G. The effect of valence state and site geometry on Ti L3,2 and O K electron energy-loss spectra of TixOy phases. Am. Mineral. 92, 577–586 (2007).
Lucky, R. A. & Charpentier, P. A. N-doped ZrO2/TiO2 bimetallic materials synthesized in supercritical CO2: morphology and photocatalytic activity. Appl. Catal. B 96, 516–523 (2010).
Ou, G. et al. Tuning defects in oxides at room temperature by lithium reduction. Nat. Commun. 9, 1302 (2018).
Wan, J. et al. Defect effects on TiO2 nanosheets: stabilizing single atomic site Au and promoting catalytic properties. Adv. Mater. 30, 1705369 (2018).
Li, J. et al. Tracking the effect of sodium insertion/extraction in amorphous and anatase TiO2 nanotubes. J. Phys. Chem. C. 121, 11773–11782 (2017).
Hernández-Alonso, M. D., Coronado, J. M., Bachiller-Baeza, B., Fernández-García, M. & Soria, J. Influence of structural and surface characteristics of Ti1‒xZrxO2 nanoparticles on the photocatalytic degradation of methylcyclohexane in the gas phase. Chem. Mater. 19, 4283–4291 (2007).
Wang, D., Liu, L., Sun, X. & Sham, T. K. Observation of lithiation-induced structural variations in TiO2 nanotube arrays by X-ray absorption fine structure. J. Mater. Chem. A 3, 412–419 (2015).
Ma, R., Fukuda, K., Sasaki, T., Osada, M. & Bando, Y. Structural features of titanate nanotubes/nanobelts revealed by Raman, X-ray absorption fine structure and electron diffraction characterizations. J. Phys. Chem. B 109, 6210–6214 (2005).
Zhao, Y. et al. Layered-double-hydroxide nanosheets as efficient visible-light-driven photocatalysts for dinitrogen fixation. Adv. Mater. 29, 1703828 (2017).
Luca, V., Djajanti, S. & Howe, R. F. Structural and electronic properties of sol-gel titanium oxides studied by X-ray absorption spectroscopy. J. Phys. Chem. B 102, 10650–10657 (1998).
Dargaud, O. et al. Structural role of Zr4+ as a nucleating agent in a MgO-Al2O3-SiO2 glass-ceramics: a combined XAS and HRTEM approach. J. Non−Cryst. Solids 356, 2928–2934 (2010).
Galoisy, L., Pélegrin, E. L., Arrio, M. A., Ildefonse, P. & Calas, G. Evidence for 6-coordinated zirconium in inactive nuclear waste glasses. J. Am. Ceram. Soc. 82, 2219–2224 (1999).
Sun, S. et al. Efficient photocatalytic reduction of dinitrogen to ammonia on bismuth monoxide quantum dots. J. Mater. Chem. A 5, 201–209 (2017).
Lide, D. R. et al. CRC Handbook of Chemistry and Physics, (85th ed.). CRC Press. pp. 6–8. ISBN 978-0-8493-0485-9 (2004).
Chen, G. F. et al. Advances in electrocatalytic N2 reduction-strategies to tackle the selectivity challenge. Small Methods 2, 1800337 (2018).
Greenlee, L. F., Renner, J. N. & Foster, S. L. The use of controls for consistent and accurate measurements of electrocatalytic ammonia synthesis from dinitrogen. ACS Catal. 8, 7820–7827 (2018).
Wang, M. et al. Over 56.55% faradaic efficiency of ambient ammonia synthesis enabled by positively shifting the reaction potential. Nat. Commun. 10, 341 (2019).
Naldoni, A. et al. Effect of nature and location of defects on bandgap narrowing in black TiO2 nanoparticles. J. Am. Chem. Soc. 134, 7600–7603 (2012).
Zhu, D., Zhang, L., Ruther, R. E. & Hamers, R. J. Photo-illuminated diamond as a solid-state source of solvated electrons in water for nitrogen reduction. Nat. Mater. 12, 836–841 (2013).
Watt, G. W. & Chrisp, J. D. Spectrophotometric method for determination of hydrazine. Anal. Chem. 24, 2006–2008 (1952).
Kresse, G. & Hafner, J. Ab initio molecular dynamics for open-shell transition metals. Phys. Rev. B 48, 13115–13118 (1993).
Kresse, G. & Furthmüller, J. Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set. Comput. Mater. Sci. 6, 15–50 (1996).
Kresse, G. & Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 54, 11169–11186 (1996).
Blöchl, P. E. Projector augmented-wave method. Phys. Rev. B 50, 17953–17979 (1994).
Methfessel, M. & Paxton, A. T. High-precision sampling for Brillouin-zone integration in metals. Phys. Rev. B 40, 3616–3621 (1989).
Perdew, J. P., Burke, K. & Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 77, 3865–3868 (1996).
Dudarev, S. L., Savrasov, S. Y., Humphreys, C. J. & Sutton, A. P. Electron-energy-loss spectra and the structural stability of nickel oxide: an LSDA + U study. Phys. Rev. B 57, 1505–1509 (1998).
Cococcioni, M. & de Gironcoli, S. Linear response approach to the calculation of the effective interaction parameters in the LDA + U method. Phys. Rev. B 71, 035105 (2005).
Grimme, S., Antony, J., Ehrlich, S. & Krieg, H. A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 132, 154104 (2010).
Grimme, S., Ehrlich, S. & Goerigk, L. Effect of the damping function in dispersion corrected density functional theory. J. Comput. Chem. 32, 1456–1465 (2011).
Tang, W., Sanville, E. & Henkelman, G. A grid-based Bader analysis algorithm without lattice bias. J. Phys. Condens. Matter 21, 084204 (2009).
Henkelman, G., Arnaldsson, A. & Jónsson, H. A fast and robust algorithm for Bader decomposition of charge density. Comput. Mater. Sci. 36, 354–360 (2006).
Chorkendorff, I. & Niemantsverdriet, J. W. Concepts of modern catalysis and kinetics. Angew. Chem. Int. Ed. 117, 2375–2375 (2005).
Rossmeisl, J., Logadottir, A. & Nørskov, J. K. Electrolysis of water on (oxidized) metal surfaces. Chem. Phys. 319, 178–184 (2005).
Acknowledgements
We thank the following funding agencies for supporting this work: the National Key Research and Development Program of China (2017YFA0206901, 2018YFA0209401), the Natural Science Foundation of China (21773036, 21688102, 51761165012), the Science and Technology Commission of Shanghai Municipality (17JC1400100, 19XD1420400), the Innovation Program of Shanghai Municipal Education Commission (2019-01-07-00-07-E00045), and the Collaborative Innovation Center of Chemistry for Energy Materials (2011-iChem). We thank the support from Shanghai Synchrotron Radiation Facility (SSRF).
Author information
Authors and Affiliations
Contributions
G.Z. and X.X. proposed, designed, and supervised the project. G.Z., X.X., J.L., N.C., and Z.C. wrote the manuscript. N.C., K.Z., J.X., J.Z., and J.L. performed the experiments and analyzed the data. Z.C. and X.X. performed the theoretical calculations. All the authors discussed, commented on, and revised the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Peer Review Information: Nature Communications thanks Zhongfang Chen, and the other, anonymous, reviewer(s) for their contribution to the peer review of this work.
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Source Data
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Cao, N., Chen, Z., Zang, K. et al. Doping strain induced bi-Ti3+ pairs for efficient N2 activation and electrocatalytic fixation. Nat Commun 10, 2877 (2019). https://doi.org/10.1038/s41467-019-10888-5
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41467-019-10888-5
This article is cited by
-
Synthesis, characterization, and mechanistic study involved in the self-doping of TiO2 nanotubes simultaneously to the impedimetric detection of methylene blue
Journal of Solid State Electrochemistry (2024)
-
Electrocatalytic reduction of NO to NH3 in ionic liquids by P-doped TiO2 nanotubes
Frontiers of Chemical Science and Engineering (2023)
-
Cu-based MOF-derivatived catalyst for efficient electrocatalytic reduction of nitrogen and nitrate to produce ammonia
Ionics (2023)
-
Crystal defect engineering of Bi2Te3 nanosheets by Ce doping for efficient electrocatalytic nitrogen reduction
Nano Research (2023)
-
Wettability control of defective TiO2 with alkyl acid for highly efficient photocatalytic ammonia synthesis
Nano Research (2023)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.