Abstract
Layered transition-metal dichalcogenides have emerged as exciting material systems with atomically thin geometries and unique electronic properties. Pressure is a powerful tool for continuously tuning their crystal and electronic structures away from the pristine states. Here, we systematically investigated the pressurized behavior of MoSe2 up to ∼60 GPa using multiple experimental techniques and ab-initio calculations. MoSe2 evolves from an anisotropic two-dimensional layered network to a three-dimensional structure without a structural transition, which is a complete contrast to MoS2. The role of the chalcogenide anions in stabilizing different layered patterns is underscored by our layer sliding calculations. MoSe2 possesses highly tunable transport properties under pressure, determined by the gradual narrowing of its band-gap followed by metallization. The continuous tuning of its electronic structure and band-gap in the range of visible light to infrared suggest possible energy-variable optoelectronics applications in pressurized transition-metal dichalcogenides.
Similar content being viewed by others
Introduction
Transition-metal dichalcogenides (TMDs) 2H-MX2 (M=Mo, W, and etc, X=S, Se, and Te) have recently attracted intense scientific and engineering interest because of their ease of fabrication and unique electronic structure1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16. TMDs have strong chemical bonding within each X-M-X trilayer and weak van der Waals (vdW) interaction between neighbor trilayers in their crystal structures. This quasi two-dimensional (2D) nature grants TMDs facile three-dimensional (3D) to 2D crossover through methods like exfoliation1,2,4,5,8,9,12. Band structure engineering on TMDs allows ones to explore exotic condensed matter phenomena and develop many potential applications. For example, the modification of their band structures from indirect-band-gap to direct-band-gap provides insights into opto-electronics and valley electronics11,14,15,16,17,18. So far, the electronic structure engineering of TMDs has mainly been achieved through the following experimental methods: (i) applying electrical field to control the spin splitting and freedom of electrons14,16, (ii) utilizing quantum confinement with samples thinning down into monolayers of MX211,15,17,18, and (iii) employing stress or strain (by bending or stretching the thin films or employing substrates with different lattice constants)19,20, as suggested by calculations18,21,22,23,24,25,26.
Compared with the three methods mentioned above, high pressure is a powerful way to induce dramatic changes in their crystal structures and electronic structures27,28. This qualifies high pressure as a desirable approach to explore the tunability of TMDs. In particular, the ability to continuously tune the crystal and electronic structures away from the pristine states is crucial to a wide array of applications, e.g., electromechanical devices, energy-variable opto-electronics, and energy-variable photovoltaics. Various pressure-induced electronic evolutions such as semiconductor to metal transitions have been observed in different materials29,30,31. However, many of these electronic transitions were accompanied by first-order structural transitions. A pressure-induced first-order structural transition, by definition, involves a discontinuous change in the volume (of the unit cell). And the corresponding discontinuity in its electronic structure could limit the energy tunability for potential opto-electronic or photovoltaic applications. To overcome this challenge, we need to discover TMDs with continuous structural and electronic response.
Previous high pressure studies on MoS2 clearly demonstrated that a first-order structural transition (2Hc-MoS2 to 2Ha-MoS2) occurred close to metallization32,33,34,35,36. This transition may relate to the vdW interactions in between neighbor S-Mo-S trilayers36. To prevent the 2Hc to 2Ha transition, the substitution of chalcogenide anions in MoS2 is a potential route. Because Se2− has broader electron orbitals than S2−’s that lead to stronger interlayer interactions, MoSe2 may have totally different high pressure behavior. Experimentally, the structural and electronic behavior of compressed MoSe2 remains to be fully explored37,38,39. Interestingly, recent calculations have predicted that MoSe2 metallizes between 28 to 40 GPa while preserving the 2Hc structure40.
In our work, high pressure up to ∼60 GPa was generated by a diamond anvil cell (DAC). X-ray diffraction (XRD) data and Raman spectra data indicate that MoSe2 preserves the 2Hc structure without any structural transition. Ab-initio calculations for modeling the layer sliding process are presented to understand the contrasting behavior between MoSe2 and MoS2, and further predict the structural stability of MoTe2. Infrared (IR) spectra data and temperature-dependent electrical resistivity data demonstrate the highly tunable transport properties of MoSe2. Electronically, both experiments and calculations show that pressure strongly modulates its band structure from semiconducting to metallic.
Results
XRD and Raman spectroscopy
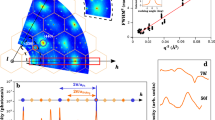
The experimental set-up of the DAC is shown in Fig. 1a. Under compression, all XRD peaks for MoSe2 continuously shift to larger 2θ (smaller d-spacing) and no new peak is observed (Supplementary Fig. 1 and Supplementary Note 1). Decompression of the sample shows the shifts of all peaks are reversible. All patterns are consistent with the 2Hc structure and representative Rietveld refinements are shown in Supplementary Fig. 2 and Supplementary Table 1. The absence of a first-order structural transition is further supported by equation of state (EOS) data in Fig. 1b and normalized cell parameters versus pressure data in Fig. 1c, as neither of them exhibits any discontinuity. To fit the pressure-volume relation, a third-order Birch-Murnaghan (BM) EOS is employed. With unit-cell volume V0 fixed at the experimental value of 120.8 Å3, the fitting yields a bulk modulus B0=62(1) GPa and a derivative of bulk modulus B’=5.6(1). The relatively large value of B’ suggests a strong change of volume compressibility under pressure.
(a) Schematic of the high pressure DAC set up. (b) Pressure-volume data from XRD measurement and the curve represents a third-order BM-EOS fitting. (c) Normalized cell parameters a/a0 and c/c0 versus pressure. The error bars given by EXPGUI-GSAS are smaller than the size of the markers. (d) Evolution of vibrational modes A1g and E2g and their difference (E2g−A1g) under pressure, measured by Raman spectroscopy.
At ambient conditions, the structure of 2Hc-MoSe2 features the X-M-X triple layers linked via vdW forces (Fig. 2a,b)41,42. During the initial compression, cell parameter c is much more compressible than a due to the weak vdW interactions in between Se-Se layers along c (Fig. 1c), while higher pressure allows them to have nearly isotropic contractions. At the highest pressure ∼60 GPa, a and c reduce by ∼10 and ∼15% respectively. The gradual closure of the vdW gap is suggested by tracking the ratio of Se-Mo layer distance to Se-Se layer distance, where it drops fast at low pressure but decreases much slower at high pressure (Supplementary Fig. 3). In addition, we measured the Raman spectra under pressure (Supplementary Fig. 4 and Supplementary Note 2). See Fig. 1d, the vibrational modes A1g and E2g, and the spacing between them shift successively under pressure. These observations indicate that the crystal structure of MoSe2 continuously evolves from a quasi 2D structure to an isotropic 3D one without a structural transition.
(a) The side view (projected on ac plane) of 2Hc structure in MoS2, MoSe2 and MoTe2. X represents S, Se and Te. The red arrows represent one sliding path for the 2Hc to 2Ha transition, where one unit of X-Mo-X triple layers (marked by a blue box) shifts systematically in ab plane. (b) The top view (projected on ab plane) of 2Hc structure. (d) The side view of 2Ha structure. (c) The top view of 2Ha structure. (e) The total energy of MoS2, MoSe2 and MoTe2 as a function of relative sliding from 2Hc to 2Ha. The total energies of 2Hc structures are set to be zero as references, marked by a broken line. For MoS2, the unit cell volume was fixed at experimental data under 20 GPa35. After electronic relaxation of the atomic positions, the S-Mo-S layer distance of structure was set to be a constant during the layer sliding. The same procedures were performed on MoSe2 under 23 GPa (our experimental data) and MoTe2 under 20 GPa (theoretical data40).
Structural calculations
Our XRD and Raman results appear to be surprising—At ambient conditions, MoS2 and MoSe2 are iso-structural in crystal structures and possess highly similar electronic structures, and it is therefore natural to assume that the 2Hc to 2Ha transition32,33,34,35 would also occur in MoSe2. Nonetheless, 2Ha structure does not fit the XRD patterns of MoSe2 in the entire pressure region studied in this work. Though bearing highly similar Mo-Se chemical environments and the same space group (P63/mmc), 2Ha structure and 2Hc structure have distinct structural topologies. In 2Ha structure Mo occupies a 2b Wyckoff position while in 2Hc structure it occupies a 2c Wyckoff position. Also, the two adjacent units of X-Mo-X triple layers have contrasting stacking patterns in 2Hc and 2Ha (shown in Fig. 2a–d). To seek theoretical support of the structural stability of 2Hc-MoSe2, we performed two sets of ab-initio calculations. We first confirmed that 2Hc-MoSe2 is more stable than 2Ha-MoSe2, based on the experimental unit cell at the highest pressure ∼60 GPa. This is consistent with recent calculations showing the enthalpy difference between 2Ha and 2Hc of MoSe2 keeps increasing from ambient pressure up to at least 130 GPa40. We then calculated the cell parameters at different volumes based on 2Hc-MoSe2 and the results agree well with our experimental data (Supplementary Fig. 5 and Supplementary Note 3). Note that the small discrepancy at low pressure comes from the limitations of ab-initio calculations in describing the vdW interaction.
In order to understand the contrasting structural behavior between MoS232,33,34,35 and MoSe240, and see whether there is a predictable trend in TMDs, we further carried out layer sliding simulations for MoS2, MoSe2, and MoTe2 at ∼20 GPa. The side and top views of 2Hc-type and 2Ha-type structures are shown in Fig. 2a–d. The transition from 2Hc to 2Ha can be realized by systematically shifting half of the atoms (one unit of X-Mo-X triple layers) in a unit cell. As illustrated in Fig. 2a, we defined one sliding path by the red arrows for this transition. For MoS2, the unit-cell volume is fixed at the experimental value at 20 GPa35. After initial relaxation of the atomic positions within the 2Hc-type structure, the S-Mo-S layer distances are fixed during the layer sliding. The same procedures are performed for MoSe2 at 23 GPa (using our experimental data) and MoTe2 at 20 GPa (based on previous theoretical data40). The total energies of 2Hc are set to be zero as the references. Fig. 2e shows the relative energies as a function of the relative layer sliding, i.e., 0 represents 2Hc and 1 represents 2Ha. MoX2 needs to overcome an energy barrier in order to undergo the 2Hc to 2Ha transition. The maximum energy barrier is ∼0.3 eV for MoSe2 and ∼0.5 eV for MoTe2, which are significantly higher than ∼0.15 eV for MoS2. More importantly, 2Ha-MoSe2 and 2Ha-MoTe2 bear higher energies than the initial 2Hc structures, which would make this transition unfavorable. However, in the case of MoS2, the 2Ha structure becomes energetically favorable. Note that the X-Mo-X layer distance is fixed in this set of calculations. Realistically in compressed MoS2, the S-Mo-S distance and unit cell volume drop during the 2Hc to 2Ha transition32,33,34,35, which allows the total change in enthalpy to be continuous at zero temperature.
IR spectroscopy
The lattice response of MoSe2 at high pressure will inevitably change its electronic structure, and thus its optical properties which strongly depend on the electronic structure. Our data shows that MoSe2 undergoes a large electronic evolution where band-gap narrowing is followed by metallization of MoSe2. Fig. 3 shows the measured synchrotron IR spectra and its analysis (details are shown in Supplementary Note 4). Below 16.3 GPa, the transmittance spectra (Fig. 3a) look similar, where a transmission window extends from 0.06 to 1.0 eV. At pressure above 20.2 GPa, the 0.3–1.0 eV region develops into a tilted curve and keeps collapsing into lower energy region, indicating the band-gap’s narrowing. At above 40.7 GPa to the highest pressure, nearly zero transmission is observed in between 0.15 to 1.0 eV, suggesting the metallization of MoSe2. Another way to interpret the IR data is from the viewpoint of the optical density (OD) Aλ (see Supplementary Fig. 6 for the plot of OD versus energy). OD or Aλ is defined as −logT (T as the transmittance). It can be easily seen from the energy-pressure-OD map in Fig. 3b that a clear changeover of low OD (in semiconducting state) to high OD (in metallic state) occurs between 20 to 35 GPa.
(a) Representative IR transmittance spectra at high pressures, numbers show pressures in unit of GPa. The 0.23–0.28 eV region is obscured by diamond phonon absorptions from the DAC. (b) Pressure−energy−optical density (OD) contour, OD is defined as −log(T) while T is the transmittance. (c) Evolution of band gap under pressure. Circles are extrapolated indirect band gaps and the curve shows a parabolic fit of the band gap versus pressure. The band gap closure is observed at ∼40 GPa.
For an indirect-band-gap semiconductor, the absorption coefficient is proportional to the square of the energy difference of the photon energy and band-gap43. Using this empirical model for semiconductors, we obtained the indirect-band-gap Eg via linear extrapolations of (hνAλ)1/2 where hν is the photon energy. A representative extrapolation is shown in Supplementary Fig. 7. The fitted Eg value at 20.2 GPa is 0.4 eV. From 20.2 to 35.1 GPa, Eg keeps decreasing (see Fig. 3c). From 40.7 GPa to the highest pressure, Eg is nominally zero. We notice that the trend of band-gap decrease at below 41 GPa could not be well described by a linear fitting. The non-linearity is also shown in previous calculations on band-gap’s dependence on applied strain23. The lack of data points and inaccuracies in optical measurement prevent us from determining the best function for the band-gap−pressure relation. However, as a simple approach to guide eyes, we fit the data with a parabolic curve, which yields Eg=−0.08(2) P+0.0010(3) P2, indirect-band-gap (Eg) in unit of eV and pressure (P) in unit of GPa. The extrapolated band-gap at ambient pressure is 1.6(3) eV, which is in good agreement with previous reports18,44,45.
Electrical resistivity
We also measured the temperature-dependent resistivity up to ∼42 GPa (Supplementary Note 5 and Supplementary Fig. 8). At low pressures (Fig. 4a), the temperature (T)−resistivity (ρ) curves at below 23.4 GPa exhibit negative dρ/dT throughout all temperatures, indicating the presence of a semiconducting state. From 27.0 to 37.0 GPa, the high-temperature region shows positive dρ/dT whereas the low-temperature region has negative dρ/dT (see Fig. 4b). At above 41.0 GPa, positive dρ/dT is observed in all temperatures, implying the metallization of MoSe2. A comparison of our room temperature resistivity data on MoSe2 (Fig. 4c) with previous data on MoS2 shows that there are dissimilar trends in between them34,35. For MoS2, the room temperature resistivity dropped strongly at below ∼15 GPa and then reached a plateau at higher pressure34,35, which was related to a first-order structural transition. In sharp contrast, for MoSe2, the decrease of its room temperature resistivity is nearly exponential, fit by log (ρ)=1.9(1)—0.134(5) P, resistivity (ρ) in unit of Ω cm−1 and pressure (P) in unit of GPa. Pressure allows the room temperature electrical resistivity of MoSe2 to decrease more than six orders of magnitude from ambient to 41.6 GPa.
(a) Temperature-resistivity curves at different pressure, numbers show pressures in GPa. (b) Temperature-pressure-resistivity contour map. (c) Room temperature resistivity versus pressure, the line shows a linear fitting of log ρ versus pressure (equivalent for an exponential fitting of ρ versus pressure).
Electronic structure
To better understand the electronic evolution of MoSe2 that determines its highly tunable optical and electrical transport properties, we performed ab-initio calculations on the electronic structure of MoSe2 at four representative pressures. The results undoubtedly demonstrate the pressure-induced band-gap narrowing and metallization. At ambient pressure, seen from Fig. 5a, the band structure is consistent with previous results18,44,45. It has a direct-band-gap EK-K (∼1.8 eV) at K and an indirect-band-gap (∼1.3 eV) that locates in between Γ and Γ-K conduction band (CB) valley. The bottom of CB between Γ and K mainly origins from the Mo dxy and dx2−y2 orbitals, and the top of valence bands (VBs) at Γ comes from the Mo dz2 orbital. Meanwhile, the dxz and dyz dominated CBs are further above from the Fermi-level (EF). Higher pressure results in a strong decrease of its indirect-band-gap and induces large movements of the orbitals towards the EF. At 23 GPa, shown in Fig. 5b, the indirect-band-gap becomes as small as 0.5 eV. Albert decreasing, the direct-band-gaps remain at large values, e.g., EK-K is ∼1.4 eV. Remarkably, pressure allows the dxz and dyz orbitals to gain more overlap with Se p orbitals and thus largely widen their band dispersions. In comparison, the dxy and dx2−y2 orbitals are less impacted due to smaller overlap with Se p orbitals. As a consequence (see Supplementary Note 6), one dxz and dyz dominated CB quickly goes down at K point and forms two CB valley minimum together with the previous dxy and dx2−y2 dominated CB.
Metallic band structures are obtained by further increasing the pressure. For example, seen from Fig. 5c at 41 GPa, there lies density of states across the EF. The dxy and dx2−y2 dominated CB valley minimum crosses below the EF, while the other CB valley minimum is still slightly above the EF (see Supplementary Fig. 9). At 58 GPa, shown by Fig. 5d, both CB valley minima cross below the EF. It is worth mentioning that even at as high as 58 GPa, the ‘indirect’ feature of the band structure is still well maintained: although the CBs and VBs overlap in energy range, no direct cross is seen. To be specific, the energy separation at K is as large as ∼0.6 eV. Meanwhile, the relative shifts of CBs and VBs generate a number of hole pockets (e.g., at Γ and A) and electron pockets (e.g., at K). These pockets may largely affect the low-temperature electrical and optical transport properties of MoSe2.
Discussions
Our experiments and calculations clearly demonstrate the absence of structural transition in MoSe2. One empirical understanding of the contrasting behavior in MoS232,33,34,35,36 and MoSe2 involves the different localizations of p orbitals among chalcogenide anions S2−, Se2−, and Te2−. The 3p orbitals of S2− dominate the electronic structure in MoS2 while the 4p and 5p orbitals are primary for MoSe2 and MoTe2 correspondingly. 4p and 5p orbitals are much more delocalized than 3p orbitals, which would give rise to strong interaction within the vdW gaps of MoSe2 and MoTe2 to prevent this sliding process, vice versa for MoS2. We can safely conclude that it is easier for 2Hc-MoS2 to experience a structural transition through sliding in between neighbor S-Mo-S layers, but this does not apply to MoSe2 or MoTe2. Beside from the effects of chalcogenides anions, the effects of transition metal cation should also be considered in determining the stabilities of layered structures. For example, recent calculations proposed that the interlayer Mo-Mo d-electron propagation should be considered in determining the stability of layered structures40. More importantly, size effect of different transition metal cations is also expected to change the interlayer interactions. Previous studies on WS2 and WSe246,47,48 showed that they experience continuous lattice contractions under pressure. W2+ has broader electron orbitals and may introduce stronger interlayer interactions than Mo2+, which results in the absence of layer sliding in WS2. In a more general perspective, stronger interlayer interactions help stabilize the structures of TMDs and are more likely to yield continuous lattice response under extreme environments such as pressure.
Previous studies have reported many electronic transitions such as insulator to metal or semiconductor to metal transitions in the group of binary chalcogenides, see for example, Bi2X331,49,50, Sb2X351,52,53, and Ag2X54,55,56. For structures starting with vdW gaps at ambient conditions, the closure of their vdW gaps is generally accompanied or followed by first-order structural transitions where large structural re-constructions or atomic movements take place43,44,45,46,47,48. However, in the case of MoSe2, the metallization process does not involve any sudden change in the crystal structure, which allows its electronic structure to be continuously tuned. Through multiple experimental techniques combined with ab-initio calculations, we demonstrate that the band-gap of MoSe2 (in the range of visible to IR region) exhibits a strong dependence on pressure. This may allow MoSe2, one representative TMD, to be applied in energy-variable opto-electronics and photovoltaics, although the limitation of sample size (0.05 to 0.1 mm) must be taken into account in future investigation.
Compared with others methods of band structure engineering approachable by experiments11,12,13,14,15,16,17,18, pressure is the only way to metalize MoSe2 and MoS234,35. This highlights pressure’s dramatic role in tuning the electronic properties of TMDs. Our different aspects of the experimental data show good agreement with the recent calculations on MoSe240. This further suggests 2Hc-MoSe2 a suitable material with a concise unit cell for testing and improving first-principles calculations that can be probed by experiments. If future experiments, i.e., by applying non-uniaxial compression, are able break the inversion symmetry of the crystal structure of pressurized MoSe2, the spin and valley electronics of MoSe2 would be largely different when we consider the large shifts of the CBs and VBs5,12,14,16. More importantly, the novel scenario of excitonic insulator may be experimentally realizable in MoSe240, while in MoS2 the complexity of the layer sliding structural transition may prevent the formation of this electronic state32,33,34,35,36. For the pressurized metallic MoSe2, its distinguished ‘indirect’ band structures and electronics state filled with electron holes and pockets await further exploitation in condensed matters physics, i.e., charge density wave or superconductivity may be found in TMDs at higher pressure35,36,40.
In conclusion, we comprehensively studied the high pressure behavior of MoSe2 up to ∼60 GP through a series of structural, vibrational, optical, and electrical measurements combined with ab-initio calculations. 2Hc-MoSe2 evolves from an anisotropic 2D layered structure to an isotropic 3D one without any sudden structural change under pressure. Our layer sliding calculations highlight the role of the chalcogenide anions in stabilizing either 2Ha or 2Hc layered patterns. Electronically, MoSe2 undergoes a semiconductor to metal transition, and correspondingly exhibits highly tunable optical and electrical properties. Upon compression, the ‘indirect’ feature of its electronic structure is robustly conserved with the appearance of two conduction band minima. The large and continuous tuning of its electronic structure may have potential applications in energy-variable (visible to IR) opto-electronics and photovoltaics.
Methods
Sample growth
High quality stoichiometric MoSe2 single crystals were grown by direct vapor transport technique39,44,57. Elemental Mo and Se (99.9% purity, purchased from Koch Light Ltd.) of the stoichiometric ratio were sealed in a quartz ampoule at pressure better than 10−5 Torr. The ampoule was placed in a two-zone horizontal furnace where the temperatures were slowly raised from room temperature to 1,060 and 1,080 °C for growth zone and source zone respectively. This temperature gradient was then maintained for ∼188 h to produce to single crystal platelets of MoSe2. The shiny and gray crystals have a typical thickness of ∼4 μm and area of ∼3 mm × 3 mm. The purity and homogeneity are checked by electron microprobe analysis.
High pressure experiments
Single crystals of MoSe2 were used for the high pressure IR, Raman, and resistivity measurements. Powders of MoSe2 were grounded from single crystals for the high pressure XRD measurements. Ruby spheres were used for determining pressure for all experiments. Neon was used as the pressure transmitting medium for the XRD and Raman experiments. Angle dispersive XRD experiments were performed at beamline 16-BMD of the Advanced Photon Source (APS), Argonne National Laboratory (ANL). The Rietveld fitting was performed using GSAS-EXPGUI package58. The Raman spectra were collected using a Renishaw inVia micro Raman system with a 514 nm laser excitation line. High-pressure IR measurements were conducted at beamline U2A of the National Synchrotron Light Source (NSLS), Brookhaven National Laboratory (BNL). A MoSe2 single crystal was sandwiched between the pressure transmitting medium (KBr) and one side of the culet. Infrared measurements were performed on a Bruker Vertex 80v FT-IR spectrometer coupled to a Hyperion-2000 microscope with a MCT mid-band detector. Fringes in raw IR data were removed by filtering high frequency terms after Fourier transformation. For temperature-dependent four-probe resistivity measurement, cubic BN was used as the insulating layer, and four electrodes were cut from Pt foils. The temperature-dependent sheet resistance of the sample was measured with Van der Paul geometry by cooling down to 10 K in a liquid helium cryostat. Pressures were measured at room temperature. More details are described in the supplementary information.
Ab-initio calculations
The Vienna ab-initio Simulation Package59,60 was employed to optimize crystal structures and calculate electronic structures with the framework of local density approximation density functional theory61. The projector augmented wave62 pseudo-potential was used and the kinetic energy cutoff was fixed to 450 eV for all the calculations. For structural calculations in comparison with experiments, the unit cell volume is fixed while the cell parameters and atomic positions are fully relaxed. For band structure calculations, the lattice constants were fixed to be experimental values, and then the atomic positions are fully relaxed. HSE06 hybrid function63 was employed to improve the band structure calculations.
Additional information
How to cite this article: Zhao, Z. et al. Pressure induced metallization with absence of structural transition in layered molybdenum diselenide. Nat. Commun. 6:7312 doi: 10.1038/ncomms8312 (2015).
References
Novoselov, K. S. et al. Two-dimensional atomic crystals. Proc. Natl. Acad. Sci. 102, 10451–10453 (2005).
Mak, K. F., Lee, C., Hone, J., Shan, J. & Heinz, T. F. Atomically Thin MoS2: A New Direct-Gap Semiconductor. Phys. Rev. Lett. 105, 136805 (2010).
Komsa, H.-P. et al. Two-Dimensional Transition Metal Dichalcogenides under Electron Irradiation: Defect Production and Doping. Phys. Rev. Lett. 109, 035503 (2012).
Tongay, S. et al. Thermally driven crossover from indirect toward direct bandgap in 2D semiconductors: MoSe2 versus MoS2 . Nano Lett. 12, 5576–5578 (2012).
Wang, Q. H., Kalantar-Zadeh, K., Kis, A., Coleman, J. N. & Strano, M. S. Electronics and optoelectronics of two-dimensional transition metal dichalcogenides. Nat. Nanotechnol. 7, 699–712 (2012).
Xiao, D., Liu, G.-B., Feng, W., Xu, X. & Yao, W. Coupled Spin and Valley Physics in Monolayers of MoS2 and Other Group-VI Dichalcogenides. Phys. Rev. Lett. 108, 196802 (2012).
Han, S. W. et al. Controlling Ferromagnetic Easy Axis in a Layered MoS2 Single Crystal. Phys. Rev. Lett. 110, 247201 (2013).
Jin, W. et al. Direct Measurement of the Thickness-Dependent Electronic Band Structure of MoS2 Using Angle-Resolved Photoemission Spectroscopy. Phys. Rev. Lett. 111, 106801 (2013).
Li, X., Zhang, F. & Niu, Q. Unconventional Quantum Hall Effect and Tunable Spin Hall Effect in Dirac Materials: Application to an Isolated MoS2 Trilayer. Phys. Rev. Lett. 110, 066803 (2013).
Mkhonta, S. K., Elder, K. R. & Huang, Z.-F. Exploring the Complex World of Two-Dimensional Ordering with Three Modes. Phys. Rev. Lett. 111, 035501 (2013).
Qiu, D. Y., da Jornada, F. H. & Louie, S. G. Optical Spectrum of MoS2: Many-Body Effects and Diversity of Exciton States. Phys. Rev. Lett. 111, 216805 (2013).
Sun, L. et al. Spin-Orbit Splitting in Single-Layer MoS2 Revealed by Triply Resonant Raman Scattering. Phys. Rev. Lett. 111, 126801 (2013).
Wang, H., Lu, Z., Xu, S. & Kong, D. Electrochemical tuning of vertically aligned MoS2 nanofilms and its application in improving hydrogen evolution reaction. Proc. Natl. Acad. Sci. 110, 19701–19706 (2013).
Yuan, H. et al. Zeeman-type spin splitting controlled by an electric field. Nat. Phys. 9, 563–569 (2013).
Zhang, Y., Chang, T., Zhou, B. & Cui, Y. Direct observation of the transition from indirect to direct bandgap in atomically thin epitaxial MoSe2 . Nat. Nanotechnol. 9, 111–115 (2014).
Yuan, H. et al. Generation and electric control of spin–valley-coupled circular photogalvanic current in WSe2 . Nat. Nanotechnol. 9, 851–857 (2014).
Ramasubramaniam, A., Naveh, D. & Towe, E. Tunable band gaps in bilayer transition-metal dichalcogenides. Phys. Rev. B 84, 205325 (2011).
Yun, W. S., Han, S. W., Hong, S. C., Kim, I. G. & Lee, J. D. Thickness and strain effects on electronic structures of transition metal dichalcogenides: 2H-MX2 semiconductors (M=Mo, W; X=S, Se, Te). Phys. Rev. B 85, 033305 (2012).
Zhu, C. R. et al. Strain tuning of optical emission energy and polarization in monolayer and bilayer MoS2 . Phys. Rev. B 88, 121301(R) (2013).
Hui, Y. Y. et al. Exceptional tunability of band energy in a compressively strained trilayer MoS2 sheet. ACS Nano 7, 7126–7131 (2013).
Kaplan-Ashiri, I. et al. On the mechanical behavior of WS2 nanotubes under axial tension and compression. Proc. Natl. Acad. Sci. 103, 523–528 (2006).
Johari, P. & Shenoy, V. B. Tuning the Electronic Properties of Semiconducting Transition Metal Dichalcogenides by Applying Mechanical Strains. ACS Nano 6, 5449–5456 (2012).
Bhattacharyya, S. & Singh, A. K. Semiconductor-metal transition in semiconducting bilayer sheets of transition-metal dichalcogenides. Phys. Rev. B 86, 075454 (2012).
Peelaers, H. & Van de Walle, C. G. Effects of strain on band structure and effective masses in MoS2 . Phys. Rev. B 86, 241401(R) (2012).
Cheiwchanchamnangij, T., Lambrecht, W. R. L., Song, Y. & Dery, H. Strain effects on the spin-orbit-induced band structure splittings in monolayer MoS2 and graphene. Phys. Rev. B 88, 155404 (2013).
Chang, C.-H., Fan, X., Lin, S.-H. & Kuo, J.-L. Orbital analysis of electronic structure and phonon dispersion in MoS2, MoSe2, WS2, and WSe2 monolayers under strain. Phys. Rev. B 88, 195420 (2013).
Jayaraman, A. Diamond anvil cell and high-pressure physical investigations. Rev. Mod. Phys. 55, 65–108 (1983).
Mujica, A., Rubio, A., Muñoz, A. & Needs, R. J. High-pressure phases of group-IV, III—V, and II—VI compounds. Rev. Mod. Phys. 75, 863–912 (2003).
Arcangeletti, E. et al. Evidence of a Pressure-Induced Metallization Process in Monoclinic VO2 . Phys. Rev. Lett. 98, 196406 (2007).
Vilaplana, R. et al. High-pressure vibrational and optical study of Bi2Te3 . Phys. Rev. B 84, 104112 (2011).
Segura, A. et al. Trapping of three-dimensional electrons and transition to two-dimensional transport in the three-dimensional topological insulator Bi2Se3 under high pressure. Phys. Rev. B 85, 195139 (2012).
Aksoy, R. et al. X-ray diffraction study of molybdenum disulfide to 38.8 GPa. J. Phys. Chem. Solids 67, 1914–1917 (2006).
Livneh, T. & Sterer, E. Resonant Raman scattering at exciton states tuned by pressure and temperature in 2H-MoS2 . Phys. Rev. B 81, 195209 (2010).
Nayak, A. P. et al. Pressure-induced semiconducting to metallic transition in multilayered molybdenum disulphide. Nat. Commun. 5, 3731 (2014).
Chi, Z.-H. et al. Pressure-Induced Metallization of Molybdenum Disulfide. Phys. Rev. Lett. 113, 036802 (2014).
Hromadová, L., Martoňák, R. & Tosatti, E. Structure change, layer sliding, and metallization in high-pressure MoS2 . Phys. Rev. B 87, 144105 (2013).
Aksoy, R., Selvi, E. & Ma, Y. X-ray diffraction study of molybdenum diselenide to 35.9 GPa. J. Phys. Chem. Solids 69, 2138–2140 (2008).
Sugai, S. & Ueda, T. High-pressure Raman spectroscopy in the layered materials 2H-MoS2, 2H-MoSe2, and 2H-MoTe2 . Phys. Rev. B 26, 6554–6558 (1982).
Dave, M., Vaidya, R., Patel, S. G. & Jani, A. R. High pressure effect on MoS2 and MoSe2 single crystals grown by CVT method. Bull. Mater. Sci. 27, 213–216 (2004).
Rifliková, M., Martoňák, R. & Tosatti, E. Pressure-induced gap closing and metallization of MoSe2 and MoTe2 . Phys. Rev. B 90, 035108 (2014).
James, P. B. & Lavik, M. T. The crystal structure of MoSe2 . Acta Crystallogr. 16, 1183–1183 (1963).
Wilson, J. A. & Yoffe, A. D. The transition metal dichalcogenides discussion and interpretation of the observed optical, electrical and structural properties. Adv. Phys. 18, 193–335 (1969).
Fox, M. in Opt. Prop. Solids Oxford Univ. Press (2010).
Mahatha, S. K., Patel, K. D. & Menon, K. S. R. Electronic structure investigation of MoS2 and MoSe2 using angle-resolved photoemission spectroscopy and ab initio band structure studies. J. Phys. Condens. Matter 24, 475504 (2012).
Coehoorn, R. et al. Electronic structure of MoSe2, MoS2, and WSe2. I. Band-structure calculations and photoelectron spectroscopy. Phys. Rev. B 35, 6195 (1987).
Selvi, E., Aksoy, R., Knudson, R. & Ma, Y. High-pressure X-ray diffraction study of tungsten diselenide. J. Phys. Chem. Solids 69, 2311–2314 (2008).
Liu, B. et al. Pressure Induced Semiconductor-Semimetal Transition in WSe2 . J. Phys. Chem. C 114, 14251–14254 (2010).
Bandaru, N. et al. Structural stability of WS2 under high pressure. Int. J. Mod. Phys. B 28, 1450168–1–10 (2014).
Polian, A. et al. Two-dimensional pressure-induced electronic topological transition in Bi2Te3 . Phys. Rev. B 83, 113106 (2011).
Zhang, J. et al. Electronic topological transition and semiconductor-to-metal conversion of Bi2Te3 under high pressure. Appl. Phys. Lett. 103, 052102 (2013).
Gomis, O. et al. Lattice dynamics of Sb2Te3 at high pressures. Phys. Rev. B 84, 174305 (2011).
Zhao, J. et al. Pressure-induced disordered substitution alloy in Sb2Te3 . Inorg. Chem. 50, 11291–11293 (2011).
Bera, A. et al. Sharp Raman Anomalies and Broken Adiabaticity at a Pressure Induced Transition from Band to Topological Insulator in Sb2 Se3 . Phys. Rev. Lett. 110, 107401 (2013).
Zhang, J. et al. Impurity level evolution and majority carrier-type inversion of Ag2S under extreme compression: Experimental and theoretical approaches. Appl. Phys. Lett. 103, 082116 (2013).
Zhao, Z., Wang, S., Zhang, H. & Mao, W. L. Pressure induced structural transitions and metallization in Ag2Te. Phys. Rev. B 88, 024120 (2013).
Zhao, Z. et al. Tuning the crystal structure and electronic states of Ag2Se: Structural transitions and metallization under pressure. Phys. Rev. B 89, 180102(R) (2014).
Agarwal, M. K., Patel, P. D. & Gupta, S. K. Effect of doping MoSe2 single crystals with rhenium. J. Cryst. Growth 129, 559–562 (1993).
Toby, B. H. EXPGUI, a graphical user interface for GSAS. J. Appl. Cryst. 34, 210–213 (2001).
Kresse, G. & Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 59, 1758–1775 (1999).
Kresse, G. & Hafner, J. Ab initio molecular dynamics for liquid metals. Phys. Rev. B 47, 558–561 (1993).
Hohenberg, P. & Kohn, W. Inhomogeneous electron gas. Phys. Rev. 155, 864–871 (1964).
Blöchl, P. E. Projector augmented-wave method. Phys. Rev. B 50, 17953–17979 (1994).
Heyd, J., Scuseria, G. E. & Ernzerhof, M. Hybrid functionals based on a screened Coulomb potential. J. Chem. Phys. 124, 8207–8215 (2006).
Acknowledgements
We thank Y. Xu at Stanford University for useful discussions. We thank S. Tkachev, C. Park, S. Sinogeikin, H. Yan, and Y. Meng at APS for their technical assistance. Z.Z., H.T.Y., Y.L., H.Y.H., Y.C., and W.L.M. are supported by the Department of Energy (DOE), Basic Energy Sciences (BES), Materials Sciences and Engineering Division, under Contract DE-AC02-76SF00515. H.Z. is supported by ARO W911NF-09-1-0508. K.D.P. and G.K.S. are funded by a major research project from University Grants Commission, New Delhi, India. HPCAT operations are supported by DOE-NNSA, DE-NA0001974 and DOE-BES, DE-FG02-99ER45775, with partial instrumentation funding by NSF MRI-1126249. APS is supported by DOE-BES, DE-AC02-06CH11357. U2A is supported by COMPRES under NSF Cooperative Agreement EAR 11-57758 and DOE-NNSA, DE-FC03-03N00144, CDAC. NSLS is supported by DOE-BES, DE-AC02-98CH10886.
Author information
Authors and Affiliations
Contributions
Z.Z., H.Z., H.T.Y., and W.L.M. designed the project. G.K.S. and K.D.P. synthesized the samples. Z.Z., H.T.Y., S.W., Y.L., Q.Z., and Z. L. conducted the experiments. H.Z. performed the calculations. Y.C., H.Y.H, and W.L.M. supervised the project and all authors contributed to data discussions. Z.Z., H.Z., and H.T.Y., wrote the paper with inputs from all others.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
Supplementary Figures 1-9, Supplementary Table 1, Supplementary Notes 1-6 and Supplementary References (PDF 1059 kb)
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Zhao, Z., Zhang, H., Yuan, H. et al. Pressure induced metallization with absence of structural transition in layered molybdenum diselenide. Nat Commun 6, 7312 (2015). https://doi.org/10.1038/ncomms8312
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/ncomms8312
This article is cited by
-
The structural, stability, electronic, optical and thermodynamic properties of MoX2 (X= S, Se, and Te) under hydrostatic pressures: a plasmon approach and first-principle study
Journal of Molecular Modeling (2024)
-
Free-electron interactions with van der Waals heterostructures: a source of focused X-ray radiation
Light: Science & Applications (2023)
-
Giant piezoresistivity in a van der Waals material induced by intralayer atomic motions
Nature Communications (2023)
-
Broadband infrared study of pressure-tunable Fano resonance and metallization transition in 2H-\(\hbox {MoTe}_2\)
Scientific Reports (2022)
-
Two-dimensional diamonds from sp2-to-sp3 phase transitions
Nature Reviews Materials (2022)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.