Abstract
The development of spintronics devices relies on efficient generation of spin-polarized currents and their electric-field-controlled manipulation. While observation of exceptionally long spin relaxation lengths makes graphene an intriguing material for spintronics studies, electric field modulation of spin currents is almost impossible due to negligible intrinsic spin–orbit coupling of graphene. In this work, we create an artificial interface between monolayer graphene and few-layer semiconducting tungsten disulphide. In these devices, we observe that graphene acquires spin–orbit coupling up to 17 meV, three orders of magnitude higher than its intrinsic value, without modifying the structure of the graphene. The proximity spin–orbit coupling leads to the spin Hall effect even at room temperature, and opens the door to spin field effect transistors. We show that intrinsic defects in tungsten disulphide play an important role in this proximity effect and that graphene can act as a probe to detect defects in semiconducting surfaces.
Similar content being viewed by others
Introduction
Graphene is a promising material for both fundamental spin-based transport phenomena and low power-consuming spin-based device applications1,2. Among the first predictions for graphene was the topological insulator with spin-polarized edge states3. Furthermore, room temperature (RT) ballistic charge transport4 and gate-tunability make graphene an ideal material for spin field effect transistors (FETs)5. However, graphene’s extremely weak intrinsic spin–orbit coupling (SOC)6 makes the experimental observation of these phenomena very challenging. Previous studies have shown that the weak hydrogenation of graphene can significantly enhance the SOC7,8. However, chemical functionalization introduces disorder and severely limits charge mobility and hence, the spin relaxation length8,9. Equally important, the hybridization between hydrogen and carbon induces a Rashba type SOC by introducing sp3 defects thereby breaking the inversion symmetry of the lattice10. The decoration of the graphene surface with heavy-metal adatoms such as gold (Au), indium (In) or thallium (Tl) has also been proposed to increase the SOC11,12. However, also this approach introduces disorder and hence, equally limits both charge and spin transport properties13.
These challenges have recently brought to light other two-dimensional crystals such as transition metal dichalcogenides (TMDCs). TMDCs have band gaps in a technologically attractive energy range (~1–2 eV) and orders of magnitude higher SOC than graphene14. This, combined with spin split bands makes them an almost ideal material for spintronics. However, the presence of unavoidable defects in TMDCs typically limits their electron mobilities to μ~100 cm2 V−1 s−1 (ref. 15). Hence, we expect a spin relaxation length even lower than in weakly hydrogenated graphene.
In this work, we show that by utilizing the proximity effect between graphene and TMDCs one can keep the extraordinary high mobilities of crystalline graphene and at the same time enhance its SOC to allow electric field controllable spin currents at RT. Since graphene and WS2 have comparable workfunctions (~4.6 eV and ~4.7 eV, respectively)16,17,18, the charge neutrality point of graphene on WS2 is expected to be located approximately in the middle of the energy gap of WS2. Therefore, naively, one would expect WS2 to act just as another inert substrate, such as SiO2 or BN. Remarkably, the presence of intrinsic defects in WS2 creates electronic states that overlap in energy with the electronic states of graphene. Because of the finite thickness of the WS2 (~10 nm) substrate, the number of these defects is large. Thus, above a threshold back-gate voltage (VTH) they act as a sink for electronic charges, resulting in a gate bias (VBG)-independent conductivity. For VBG<VTH, our devices have electronic mobilities as high as 50,000 cm2 V−1 s−1, comparable to what has been reported on BN substrates19 with no signature of SOC enhancement. In contrast, for VBG>VTH, we found a giant enhancement of SOC (~17 meV). The combination of high charge mobility and large proximity induced SOC results in a gate-tunable spin Hall effect (SHE) even at RT, a crucial ingredient in realizing spintronics paradigms such as the spin FET5 and potentially even the quantum spin Hall insulator3.
Results
Device structure and measurement configurations
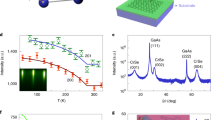
The fabrication of graphene/WS2 heterostructure (Fig. 1a) devices starts with the standard dry transfer method4 of single-layer graphene (SLG) onto atomically flat few layers of WS2 (see Supplementary Fig. 1). Subsequently, e-beam lithography is used to form electrodes and pattern graphene into a Hall bar. Typical channel lengths and widths are L=3 μm and w=1 μm, respectively. A pair of electrodes is also created directly on WS2. An optical image of a typical final device is shown in Fig. 1b. Experiments were carried out in two different measurement configurations. In the conventional local four-terminal measurement configuration (Hall bar), current I16 flows between contact 1 and contact 6 and a local voltage drop is measured between contact 2 and contact 4. In the non-local measurement configuration (H bar), the current I23 flows between the pair of contact 2 and contact 3, and a non-local voltage V45 is recorded across the neighbouring pair of contact 4 and contact 5 (Fig. 1c). All measurements were performed with standard a.c. lock-in technique at low frequencies in vacuum as a function of magnetic field (B), back-gate voltage (VBG) at both RT and liquid-Helium temperatures. In total, we have characterized 10 graphene/WS2 devices. Here we discuss two representative devices and unless otherwise stated, the results shown are from device B.
(a) Schematics representation of a multilayer WS2/graphene heterostructure device. The highest unoccupied state of the sulphur vacancy is depicted in yellow, highlighting on the W atoms closest to the vacancy. W, S and C atoms are represented by dark grey, orange and light-grey spheres, respectively. (b) Optical micrograph of a completed device with multiple Hall bar junctions on G/WS2 heterostructure and a two-terminal device on WS2. Scale bar, 2 μm. (c) Schematics for the local and non-local measurement configurations.
Local charge transport
Figure 2a shows the local conductivity σ of graphene on a WS2 substrate as a function of VBG at T=1.5 K (μh≈18,000 cm2 V−1 s−1). While on the hole side σ is linear in VBG, on the electron side σ saturates above a threshold voltage VTH ≥15 V. A similar behaviour has been observed in all graphene on WS2 devices and is absent, for example, in graphene on BN devices. We also performed two-terminal I–V measurements directly across WS2 alone. To minimize any shunting of the current through the WS2, we use Cr/Au contacts (see Supplementary Figs 2 and 3). In such measurements, only an insulating response over the same VBG range is observed. Next, we discuss perpendicular magnetic-field (B⊥)-dependent measurements as shown in Fig. 2b,c. From Hall effect measurements at low fields, we see that the origin of the conductance saturation on the electron side is due to the saturation of the charge density at ~7 × 1011 cm−2 (inset, Fig. 2c). The electron mobility above the saturation region is estimated to be μe≈24,750 cm2 V−1 s−1. The observation of such enhanced electronic mobility compared with graphene devices fabricated on SiO2 substrate is due to improved substrate roughness and reduced fabrication residues left on graphene (see Supplementary Fig. 4). In high B⊥ fields, this surprising saturation in conductivity result even alters the well-known Landau level (LL) fan diagram of graphene. This is clearly seen in the fan diagram where the longitudinal resistance, RXX, is recorded on a colour plot as a function of both VBG and the perpendicularly applied B⊥ (Fig. 2b). For example, at B⊥=4.5 T, RXX versus VBG shows the regular integer quantum hall effect on the hole side with filling factor v=2, 6, 10, 14, 18, 22. However, on the electron side only the quantization for the first two LLs is observed. Since the LLs in graphene are not equally spaced in energy, it is possible to lock the graphene resistance even at the first LL for |B⊥|>10 T (Fig. 2c), making such graphene/WS2 heterostructure potentially a suitable device for quantum resistance metrology20 (see Supplementary Fig. 5).
(a) Local resistivity (black lines) and conductivity (dark-yellow line) measurement as a function of back-gate voltage at 1.5 K. (b) Landau fan diagram of longitudinal resistance as a function of magnetic field and back-gate voltage. (c) Corresponding plots of longitudinal resistance as a function of back-gate voltage at constant magnetic fields. (Black and dark-yellow lines represent 4.5 T and 12 T, respectively.) Inset: carrier concentration as a function of applied back-gate voltage.
Non-local spin transport
We now turn our attention to non-local transport measurements. This measurement technique is a powerful tool to detect spin-dependent transport phenomena that otherwise escape notice. Recently, this configuration has been, for example, used to detect Zeeman interaction-induced spin currents in graphene in the presence of an externally applied perpendicular magnetic field21,22. Balakrishnan et al.8, also implemented this geometry to demonstrate an enhancement of SOC in hydrogenated graphene by measuring spin precession in an in-plane magnetic field. We first discuss the RT non-local measurement of a graphene on SiO2 device of comparable mobility (μ≈13.000 cm2 V−1 s−1) (Fig. 3a). For such devices, the non-local resistance is symmetric around the Dirac peak and its magnitude over the entire back-gate bias range can be fully accounted for by an Ohmic leakage contribution23,~ρe−πL/w (dashed dotted fit to the data). In contrast, graphene on WS2 substrates (devices B and C, Fig. 3b,c) shows a non-local signal, which cannot be accounted for by an Ohmic contribution neither quantitatively nor qualitatively. First, the magnitude of the observed non-local signal is almost an order of magnitude larger than in graphene on SiO2 samples. More importantly, we observe a strong electron–hole asymmetry in non-local resistance, which is completely absent in both graphene on SiO2 and even in graphene on BN substrates (not shown). For instance, device B and device C have a non-local signal difference ΔR between the hole side and electron side of ΔR~6.5 Ω and ΔR~20 Ω, respectively. We find that there is a sample-to-sample variation of the non-local signal most likely due to a variation in the amount of the disorder in each sample (see Supplementary Fig. 6; Supplementary Table 1; Supplementary Note 1). However, the electron–hole asymmetry in the non-local signal is observed in all our graphene/WS2 devices. Equally important, the onset of the asymmetry coincides with VTH at which the local conductivity saturates. Note that this asymmetry does rule out thermal effects as discussed in ref. 22 as the dominant effect.
(a) Non-local resistance measurement as a function of back-gate voltage at RT in a reference graphene/SiO2 device. Sample A: (black line) with its calculated Ohmic contribution (blue line). (b,c) Non-local measurement for sample B and sample C at 1.5 K and RT, respectively. Inset (b) represents the complete data range of data from (b). (d) Fan diagram of non-local resistance as a function of in-plane magnetic field and back-gate voltage. Colour scale bar is adjusted to show between 12 and 20 Ω for clarification. Corresponding plots of non-local resistance as a function of in-plane magnetic field at constant back-gate voltages.(Black, pink and dark-yellow lines represent 37 V, 2 V (Dirac point (DP)) and −37 V, respectively).
In-plane magnetic-field dependence of non-local signal
To determine the origin of the non-local signal, we applied a magnetic field B|| in the plane of the device parallel to the current flow direction. Such Hanle spin precession measurements for device B as a function of VBG are summarized in Fig. 3d. Also, here we see a clear electron–hole asymmetry. The critical gate voltage at which the field dependence of the non-local signal changes markedly coincides again with VTH at which the conductance saturation is observed. For all VBG<VTH, the non-local signal is magnetic field independent. Two representative RNL versus B|| traces at VBG=−37 V and VBG=2 V (Dirac point (DP)) are shown in Fig. 3d. On the other hand, for all VBG>VTH, the non-local signal shows a maximum at zero magnetic field and decreases with increasing |B||| with the onset of an oscillatory-type behaviour clearly visible for |B|||>8 T. Remarkably, the maximum observed change of the non-local signal ΔR~6 Ω as a function of field is comparable to the difference observed in the non-local signal ΔR as a function of VBG (Fig. 3b). It is important to note that in this measurement configuration only a spin current can give rise to a magnetic-field-dependent signal23,24. Thus, the observation of the oscillatory magnetic-field-dependent signal directly proves the spin origin of the non-local signal. To assure there is no contribution from Joule heating and other spurious sources, we have also performed d.c. measurements with bias currents of few μA at both current polarities (see Supplementary Fig. 7). Both the magnitude and asymmetry of the non-local signal is identical in d.c. and a.c. measurements (see Supplementary Fig. 8). We are now ready to extract the spin transport parameters of our devices. For this, we fit the field dependence of the non-local signal using the following equation23:

where γ is the spin Hall coefficient, w the width of the channel, ρ is the resistivity of the channel, ωB is the Larmor frequency, τs is the spin relaxation time and λs is the spin relaxation length. We obtain λs=2 μm, τs=5 ps. The strongly reduced τs when compared with τs of hydrogenated graphene devices8 (~90 ps) and graphene spin valve devices1 (~200 ps) is a direct consequence of the huge enhancement of SOC. However, having a high diffusion constant (D) in high-mobility graphene/WS2 devices results in long λs  ), which is comparable to those observed in graphene-based spin valve devices1.
), which is comparable to those observed in graphene-based spin valve devices1.
We finally calculate the proximity SOC strength ΔSO. Since the dominant dephasing mechanism in SLG is Elliott–Yafet type1,25, we have  (ref. 26) where εF is the Fermi energy and τP is the momentum relaxation time
(ref. 26) where εF is the Fermi energy and τP is the momentum relaxation time . Thus, we obtain a proximity SOC of 17.6 meV, which is even higher than that achieved by hydrogenating graphene8. With this, we identify the SHE and inverse SHE as the origin of the observed non-local signal. The latter is only possible because of the three orders of magnitude enhancement of the intrinsic SOC graphene when in proximity with WS2. SOC strength that is extracted from the temperature dependence of spin precession signal is at the same order with the value extracted above (see Supplementary Fig. 9). Low-field magnetoresistance measurements provided further evidence for a strong enhancement of the SOC for VBG>VTH. Here, we see at the same threshold voltage a transition from positive to negative magnetoresistance (see Supplementary Fig. 10). This transition from weak localization to weak anti-localization behaviour provides independent evidence for the proximity effect-induced SOC.
. Thus, we obtain a proximity SOC of 17.6 meV, which is even higher than that achieved by hydrogenating graphene8. With this, we identify the SHE and inverse SHE as the origin of the observed non-local signal. The latter is only possible because of the three orders of magnitude enhancement of the intrinsic SOC graphene when in proximity with WS2. SOC strength that is extracted from the temperature dependence of spin precession signal is at the same order with the value extracted above (see Supplementary Fig. 9). Low-field magnetoresistance measurements provided further evidence for a strong enhancement of the SOC for VBG>VTH. Here, we see at the same threshold voltage a transition from positive to negative magnetoresistance (see Supplementary Fig. 10). This transition from weak localization to weak anti-localization behaviour provides independent evidence for the proximity effect-induced SOC.
Discussion
Next, we discuss the origin of the proximity effect in more detail. Recently single sulphur vacancies have been observed in MoS2 flakes using an atomic resolution direct imaging technique27,28. Similar structural defects are also expected for WS2 crystals (see Supplementary Fig. 11). For this purpose, we characterized our chemical-vapour transport method-grown WS2 crystals with X-ray photoelectron spectroscopy. Figure 4a shows the X-ray photoelectron spectroscopy spectra of W4f and S2p from where we estimate a large concentration of sulphur vacancies of the order of 1013 cm−2 (see Supplementary Fig. 12; Supplementary Note 2). This result shows that there are localized states in the gap of WS2, originating from sulphur vacancies. As a next step, we performed ab initio fully relativistic density functional theory (DFT) calculations to discuss the origin of these states and its relevance for the observed phenomena (see Supplementary Fig. 13; Supplementary Note 3 for the models of other possible sources of disorder). Our calculations for the band structure of the graphene/WS2 interface show that the Dirac point of graphene is within the WS2 band gap (Fig. 4b). The band structure of the interface is nearly a superposition of the band structures of the two moieties. The lowest conduction band of WS2 is practically unchanged by the presence of graphene. Note that the proximity of WS2 with or without vacancies does not induce any significant SOC in the graphene electronic bands (see Supplementary Fig. 14).
(a) High-resolution XPS of W4f and S2p core levels. (b) Band structures of graphene and bulk WS2 with a sulphur vacancy in bulk WS2 (aligned with respect to the vacuum-level energy). In the latter, a rigid shift of 0.2 eV has been applied to the unoccupied states to correct the band gap to the experimental value32 (compare with Supplementary Fig. 14).
A sulphur vacancy (VS) on the other hand, introduces a double unoccupied state in the top half of the band gap of bulk WS2. As shown in Fig. 4b, the Fermi level crosses only the graphene states, so the longitudinal electron transport uses graphene π states; the transverse electron transport involves tunnelling from graphene to the vacancy acceptor states and back to graphene.
In fact, using  , where νF is the Fermi velocity (0.9 × 106 m s−1) calculated from the effective mass of charge carriers (see Supplementary Fig. 15) and n is the carrier concentration (7 × 1011 cm−2) at VTH, we obtain εF~0.09 eV at VTH in good agreement with our theoretical estimate. The mid gap states originate on the vacancy dangling bonds and are localized on W. They have an unusually large spin splitting of about ΔESO~0.2 eV to which we assign the origin of the proximity SOC observed. The graphene electrons move from graphene to the vacancy states in WS2 by quantum tunnelling. This tunnelling relies on the proximity of the wavefunction of the vacancy states to the graphene, which is characterized by hybridization energy VH. From the second-order perturbation theory (ΔESO≈VH2/ΔSO), the hybridization energy is estimated to be 0.059 eV.
, where νF is the Fermi velocity (0.9 × 106 m s−1) calculated from the effective mass of charge carriers (see Supplementary Fig. 15) and n is the carrier concentration (7 × 1011 cm−2) at VTH, we obtain εF~0.09 eV at VTH in good agreement with our theoretical estimate. The mid gap states originate on the vacancy dangling bonds and are localized on W. They have an unusually large spin splitting of about ΔESO~0.2 eV to which we assign the origin of the proximity SOC observed. The graphene electrons move from graphene to the vacancy states in WS2 by quantum tunnelling. This tunnelling relies on the proximity of the wavefunction of the vacancy states to the graphene, which is characterized by hybridization energy VH. From the second-order perturbation theory (ΔESO≈VH2/ΔSO), the hybridization energy is estimated to be 0.059 eV.
In conclusion, we have demonstrated the proximity-induced enhancement of otherwise weak SOC in graphene while simultaneously preserving its exceptionally high electronic quality. Our results in graphene/WS2 heterostructures show SOC as high as 17 meV, which resulted in the observation of SHE even at RT. The SHE is further supported by performing the spin precession measurements. Our detailed theoretical and experimental analyses show that the origin of the proximity effect is intrinsic, due to the unavoidable defects in the WS2 substrate. These defects not only strongly enhance the SOC but also act as a sink for electronic charges of graphene once the Fermi level aligns with these localized states, resulting in conductivity, which is independent of gate bias. Such enhancement of the SOC is crucial for the development of graphene-based spin FETs.
Methods
WS2 crystal growth
Bulk crystals of 2H-WS2 were grown by the chemical-vapour transport method using iodine as the transport agent. As-received WS2 powder (Alfa Aesar, 99.9 purity %) was sealed in an ampoule of fused quartz. The ampoule was kept in a temperature gradient from 875 to 935 °C in a dual zone furnace for 1 week. WS2 crystals grew on the cold end of the ampoule.
Dry transfer method
Transferring of graphene onto a WS2 substrate process starts with the micromechanical exfoliation of SLG flake on a bilayer polymer stack. The bottom layer polymer is dissolved with its developer so that the remaining resist (poly(methyl methacrylate) (PMMA)) and the graphene flake are isolated from the supporting substrate. The resulting PMMA/graphene film is transferred onto previously exfoliated WS2 crystal (the thickness of WS2 substrates varied from 5 to 15 nm). During this process, the graphene surface to be transferred onto the WS2 crystal surface never gets exposed to any solvent, resulting in ultra-clean interfaces. After the transfer process, the graphene surface that is contacted to the PMMA layer is annealed at 340 °C for 6 h in forming gas to remove the transfer residues. Hall bar structures are only fabricated in wrinkle, bubble-free regions. Electronic quality of graphene devices fabricated on different 2D crystals by employing this dry transfer method is discussed in ref. 29.
DFT calculations
The fully relativistic DFT calculations were performed using the method described in ref. 30. For the dispersion, we used the correction by Grimme31. Bulk WS2 was modelled using a 4 × 4 × 1 supercell, with the experimental c/a ratio. The graphene/WS2 monolayer interface was modelled in a supercell with 3 × 3 primitive cells of WS2 and 4 × 4 primitive cells of graphene, with the respective lattice vectors aligned.
Additional information
How to cite this article: Avsar, A. et al. Spin–orbit proximity effect in graphene. Nat. Commun. 5:4875 doi: 10.1038/ncomms5875 (2014).
References
Avsar, A. et al. Toward wafer scale fabrication of graphene based spin valve devices. Nano Lett. 11, 2363–2368 (2011).
Maassen, T. et al. Long spin relaxation times in wafer scale epitaxial graphene on SiC (0001). Nano Lett. 12, 1498–1502 (2012).
Kane, C. L. & Mele, E. J. Quantum spin Hall effect in graphene. Phys. Rev. Lett. 95, 226801 (2005).
Mayorov, A. S. et al. Micrometer-scale ballistic transport in encapsulated graphene at room temperature. Nano Lett. 11, 2396–2399 (2011).
Schliemann, J., Egues, J. C. & Loss, D. Nonballistic spin-field-effect transistor. Phys. Rev. Lett. 90, 146801 (2003).
Konschuh, S., Gmitra, M. & Fabian, J. Tight-binding theory of the spin-orbit coupling in graphene. Phys. Rev. B 82, 245412 (2010).
Castro Neto, A. H. & Guinea, F. Impurity-induced spin-orbit coupling in graphene. Phys. Rev. Lett. 103, 026804 (2009).
Balakrishnan, J., Koon, G. K. W., Jaiswal, M., Castro Neto, A. H. & Özyilmaz, B. Colossal enhancement of spin-orbit coupling in weakly hydrogenated graphene. Nat. Phys. 9, 284–287 (2013).
Wojtaszek, M., Vera-Marun, I. J., Maassen, T. & van Wees, B. J. Enhancement of spin relaxation time in hydrogenated graphene spin-valve devices. Phys. Rev. B 87, 081402(R) (2013).
Gmitra, M., Kochan, D. & Fabian, J. Spin-orbit coupling in hydrogenated graphene. Phys. Rev. Lett. 110, 246602 (2013).
Weeks, C., Hu, J., Alicea, J., Franz, M. & Wu, R. Engineering a robust quantum spin Hall state in graphene via adatom deposition. Phys. Rev. X 1, 021001 (2011).
Ma, D., Li, Z. & Yang, Z. Strong spin-orbit splitting in graphene with adsorbed Au adatoms. Carbon NY 50, 297–305 (2012).
Pi, K. et al. Manipulation of spin transport in graphene by surface chemical doping. Phys. Rev. Lett. 104, 187201 (2010).
Wang, Q. H., Kalantar-Zadeh, K., Kis, A., Coleman, J. N. & Strano, M. S. Electronics and optoelectronics of two-dimensional transition metal dichalcogenides. Nat. Nanotechnol. 7, 699–712 (2012).
Radisavljevic, B., Radenovic, A., Brivio, J., Giacometti, V. & Kis, A. Single-layer MoS2 transistor. Nat. Nanotechnol. 6, 147–150 (2011).
Yu, J. Y. et al. Tuning the graphene work function by electric field effect. Nano Lett. 9, 3430–3434 (2009).
Gong, C. et al. Band alignment of two-dimensional transition metal dichalcogenides: Application in tunnel field effect transistors. Appl. Phys. Lett. 103, 053513 (2013).
Britnell, L. et al. Strong light-matter interactions in heterostructures of atomically thin films. Science 340, 1311–1314 (2013).
Dean, C. R. et al. Boron nitride substrates for high-quality graphene electronics. Nat. Nanotechnol. 5, 722–726 (2010).
Janssen, T. J. B. M., Tzalenchuk, A., Lara-Avila, S., Kubatkin, S. & Fal’ko, V. I. Quantum resistance metrology using graphene. Rep. Prog. Phys. 76, 104501 (2013).
Abanin, D. A. et al. Giant nonlocality near the Dirac point in graphene. Science 332, 328–330 (2011).
Renard, J., Studer, M. & Folk, J. A. Origins of nonlocality near the neutrality point in graphene. Phys. Rev. Lett. 112, 116601 (2014).
Abanin, D. A., Shytov, A. V., Levitov, L. S. & Halperin, B. I. Nonlocal charge transport mediated by spin diffusion in the spin Hall effect regime. Phys. Rev. B 79, 035304 (2009).
Jedema, F. J., Heersche, H. B., Filip, A. T., Baselmans, J. J. A. & van Wees, B. J. Electrical detection of spin precession in a metallic mesoscopic spin valve. Nature 416, 713–716 (2002).
Józsa, C. et al. Linear scaling between momentum and spin scattering in graphene. Phys. Rev. B 80, 241403 (R) (2009).
Ochoa, H., Castro Neto, A. H. & Guinea, F. Elliot-Yafet mechanism in graphene. Phys. Rev. Lett. 108, 206808 (2012).
Zhou, W. et al. Intrinsic structural defects in monolayer molybdenum disulfide. Nano Lett. 13, 2615–2622 (2013).
Qiu, H. et al. Hopping transport through defect-induced localized states in molybdenum disfulide. Nat. Commun. 4, 2642 (2013).
Tan, J. Y. et al. Electronic transport in graphene-based heterostructures. Appl. Phys. Lett. 104, 183504 (2014).
Carvalho, A., Ribeiro, R. M. & Castro Neto, A. H. Band nesting and the optical response of two-dimensional semiconducting transition metal dichalcogenides. Phys. Rev. B 88, 115205 (2013).
Grimme, S. Semiempirical GGA-type density functional constructed with a long-range dispersion correction. J. Comput. Chem. 27, 1787–1799 (2006).
Yen, P. C., Huang, Y. S. & Tiong, K. K. The growth and characterization of rhenium-doped WS2 single crystals. J. Phys. Condens. Matter. 16, 2171 (2004).
Acknowledgements
We thank A. Hamilton, R. Ribeiro and S. Natarajan for their help and useful discussions. B. Ö. would like to acknowledge support by the National Research Foundation, Prime Minister's Office, the Singapore under its Competitive Research Programme (CRP Award No. NRF-CRP9-2011-3), the Singapore National Research Foundation Fellowship award (RF2008-07), and the SMF-NUS Research Horizons Award 2009-Phase II. A.H.C.N. would like to acknowledge support by the National Research Foundation, Prime Minister's Office, Singapore under its Competitive Research Programme (CRP Award No. NRF-CRP6-2010-5).
Author information
Authors and Affiliations
Contributions
B.Ö. and A.H.C.N. devised and supervised the project. A.A. and B.Ö. designed the experiments. A.A., J.Y.T. and Y.Y. did the device fabrication. A.A., J.B., G.K.W.K., T.T. and E.C.T.O’F. carried out the transport measurements. A.H.C.N., A.C. and A.S.R. developed the theoretical aspects of this work. G.E. and J.L. grew WS2 crystals and performed XPS measurements. A.A., A.C., A.H.C.N. and B.Ö. co-wrote the manuscript. All authors discussed the results and commented on the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
Supplementary Figures 1-15, Supplementary Tables 1, Supplementary Notes 1-3 and Supplementary References (PDF 1331 kb)
Rights and permissions
About this article
Cite this article
Avsar, A., Tan, J., Taychatanapat, T. et al. Spin–orbit proximity effect in graphene. Nat Commun 5, 4875 (2014). https://doi.org/10.1038/ncomms5875
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/ncomms5875
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.