Abstract
Understanding glass structure is still challenging due to the result of disorder, although novel materials design on the basis of atomistic structure has been strongly demanded. Here we report on the atomic structures of the zinc phosphate glass determined by reverse Monte Carlo modelling based on diffraction and spectroscopic data. The zinc-rich glass exhibits the network formed by ZnOx (averaged x<4) polyhedra. Although the elastic modulus, refractive index and glass transition temperature of the zinc phosphate glass monotonically increase with the amount of ZnO, we find for the first time that the thermal expansion coefficient is very sensitive to the substitution of the phosphate chain network by a network consisting of Zn-O units in zinc-rich glass. Our results imply that the control of the structure of intermediate groups may enable new functionalities in the design of oxide glass materials.
Similar content being viewed by others
Introduction
Oxide glass prepared by melt-quenching is usually composed of network former (NWF) and network modifier (NWM) groups1. SiO2 is a typical NWF oxide that constitutes the ring structures, which are formed from SiO4 tetrahedra2. Alkali silicate glass consisting of an alkali oxide as NWM and SiO2 as NWF is a representative oxide glass and it is widely used in industrial applications such as photonic and electronic devices. Furthermore, structural modification of the SiO4 tetrahedral network in alkali silicate glasses has been extensively studied both experimentally and theoretically3,4,5,6 to clarify structure–property relations of glasses.
P2O5 is generally classified as NWF groups along with SiO2, B2O3 and GeO2 from the viewpoint of glass-forming ability. However, P2O5 differs from other NWF oxides since the P=O bond allows the delocalized electrons in phosphate glasses7,8,9,10. On the other hand, several studies have shown that the main glass network11,12 of non-conventional phosphate glass, that is, so-called invert glass, is composed of a counter metal oxide. Previous studies have suggested that the local structure of the PO4 unit does not always act as a NWF, and that its structure depends on the chemical composition of the glass. However, the detailed structure of the glass network has not been fully understood due to the lack of experimental information, which, in turn, is the result of disorder. Phosphate glass is often considered for practical applications because of its durability13, but has significant potential for application to several other uses, owing to its unique physical and structural properties14,15,16,17,18. Understanding the network structure of a phosphate glass system is therefore one of the most important unresolved issues facing glass science.
In contrast to the conventional NWF group, several metal oxides, which are classified as intermediate groups, can act as either NWF or NWM groups1, depending on the number of NWF units, that is, the glass composition. This suggests that the conventional glass-forming theory cannot explain the behaviour of the intermediate group of oxide glass. Zinc oxide is classified as being part of the intermediate group. In the case of zinc phosphate (ZP), monolithic bulk glass can be obtained although the P2O5 content is <33.3 mol% (that is, less than that of pyro-ZP). ZP exhibits low-melting-point properties and does not contain any hazardous cations, such as lead. Therefore, ZP glass is a promising material for use as lead-free sealing glass19,20. Recently, ZP glass has also been shown to be a good host for emitting centres21,22,23,24 and is, therefore, a potential candidate for next-generation optical materials. In fact, these types of glass exhibit high transparency in the ultraviolet region; for example, the 60ZnO–40P2O5 glass has an optical absorption edge of over 6 eV, which stems from the O–Zn charge transfer transition25. The bandgap of ZP glass is, however, much wider than expected especially when that (at 3.4 eV) of the wurtzite ZnO crystal with Zn–O four coordination is considered26. The four-coordinated state of Zn is believed to result from its having a similar coordination to that of the ZnO crystal. The structure of ZP glass, particularly that of 60ZnO–40P2O5, has been extensively analysed using X-ray photoelectron spectroscopy27,28, 31P nuclear magnetic resonance (NMR)29,30,31, X-ray or neutron diffraction32,33,34, high-performance liquid chromatography analysis35, Raman and infrared spectroscopy36, as well as molecular dynamics simulation37. Several groups have also performed structural analyses using neutron and X-ray diffraction with the aid of reverse Monte Carlo (RMC) modelling34,38,39. Those studies revealed the zinc in the ZP glass to be in the four-coordinated state.
However, previous investigations have shown that metal oxide has a small oxygen coordination in glass40,41,42,43, since the rigid glass network enables the metastable species of metal oxide to be sustained in the glass matrix. Therefore, the modelling of a reliable atomic arrangement in glass based on metal–cation-specific experimental data and conventional diffraction data is essential. As such, in this article, we report on the reliable atomic configuration of ZP glass from the viewpoint of three-dimensional (3D) network linkage, that is, the connectivity of each oxide. We use a combination of 31P magic angle spinning (MAS) NMR, Zn K-edge extended X-ray absorption fine structure (EXAFS), as well as X-ray and neutron diffraction data38 to determine the dependence of this connectivity on the chemical composition and on the zinc coordination. Moreover, we discuss the relationship between several properties and glass structure and find that the thermal expansion coefficient is sensitive to the substitution of the phosphate chain network by a network consisting of Zn–O units in zinc-rich glass.
Results
Sample characterization and physicochemical properties
First of all, it is ensured that nanocrystallites are not precipitated in transparent xZnO-(100-x)P2O5 (xZP) glass prepared by the melt-quench method. The chemical compositions of ZP glasses were determined by using inductively coupled plasma-atomic emission spectrometry analysis (Supplementary Fig. 1 and Supplementary Table 1). It is confirmed that the compositions of our samples are precise. Supplementary Table 2 summarizes the glass transition temperature Tg, density, molar volume, refractive indices and the longitudinal sound velocity VL of ZP glass. As the table indicates, the Tg, density and refractive index increase with the amount of ZnO. Supplementary Fig. 2 shows that the longitudinal modulus c11 also increases with the amount of ZnO, while the molar volume decreases.
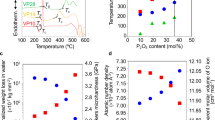
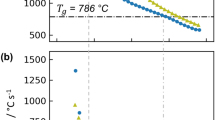
Figure 1a shows the thermal expansion curves for 58ZP, 60ZP, 65ZP and 70ZP glass. The bending points of the curves correspond to the Tg listed in Supplementary Table 2. Figure 1b shows linear thermal expansion coefficients of these glasses as a function of ZnO amount. The thermal expansion coefficients increase with increasing ZnO fraction. If the glass network is similar, that is, if the glass has the same NWF, the lower Tg glass generally exhibits a higher thermal expansion coefficient44,45. However, the obtained results show that the higher Tg glass exhibits a higher thermal expansion coefficient. The anomaly of thermal expansion coefficients suggests that the glass networks might be changed, although the Tg and c11 values changed linearly depending on the chemical composition (Supplementary Table 2).
(a) Thermal expansion curves of 58ZP, 60ZP, 65ZP and 70ZP glasses. (b) Thermal expansion coefficients below the Tg as a function of ZnO. The 70ZP glass exhibits higher Tg as well as higher thermal expansion coefficient below and above the Tg, compared to those of 60ZP glass. Closed squares indicate each mean value.
NMR spectroscopy and EXAFS
NMR spectroscopic measurements were used to obtain the information on the local structure in glasses. The 31P MAS NMR spectra of xZP glass (Fig. 2a) exhibit peaks that correspond to the Q0 (3 p.p.m.), Q1 (−11 p.p.m.) and Q2 (−30 p.p.m.) units, which are commonly observed in ZP glass29,30,31,46. However, peaks corresponding to the Q3 unit are absent from the spectra. The ratio of Qn units, as calculated from the area of each peak, shows (Fig. 2b) that the number of Q2 chains decreases significantly as the amount of ZnO increases. The results of our NMR are in line with the results of high-performance liquid chromatography analysis35, indicating that the PO4 units no longer form a glass network, especially for 70ZP glass, as was recently suggested by Hoppe et al.39.
We also determined the Zn–O coordination number and subsequently the local oxygen coordination of zinc. The EXAFS spectra, k3χ(k), of the different types of ZP glass are shown in Fig. 3a. As the figure shows, the oscillation and the period of the oscillation are slightly attenuated at high k values and shift towards the low k region as the amount of ZnO increases. The Fourier-transformed (FT) EXAFS spectra of the xZP glass, shown in Fig. 3b, exhibit one prominent peak, that is, the peak corresponding to the first coordination of the glass. Supplementary Table 3 summarizes the atomic distance and Debye–Waller factor, coordination number, NZnO, of the Zn–O in the glass. We estimated the values by fitting the first coordination shell in the EXAFS spectra and obtained an atomic distance and NZnO of ∼1.96–1.97 Å and <4, respectively. This small oxygen coordination is different from those obtained from X-ray and neutron diffraction with the aid of RMC modelling34,38,39.
Structure modelling
We performed RMC modelling using X-ray diffraction, neutron diffraction38 and EXAFS data with several coordination number constraints to identify several chemical coordination states around phosphorus, based on the results of 31P MAS NMR, to reveal the behaviour of the oxygen coordination around zinc. Figure 4 shows that there is good agreement between the RMC-modelled and experimentally determined X-ray and neutron structure factors, S(Q) and EXAFS k3χ(k), of the 60ZP and the 70ZP glasses. The partial structure factors, Sij(Q), calculated from the RMC models are shown in Supplementary Fig. 3. The 60ZP and the 70ZP glasses have almost identical SPO(Q), SOO(Q) and SZnO(Q), indicating that the different types of ZP glass have the same short-range structure. This similarity can also be clearly observed in the partial-pair correlation functions, gij(r), shown in Supplementary Fig. 4. On the other hand, the SPP(Q), SPZn(Q) and SZnZn(Q) differ significantly, indicating the substantial difference between the connectivity of the short-range structural units, PO4 tetrahedra and Zn–O polyhedra in the 60ZP and the 70ZP glasses. This behaviour is consistent with the NMR data, which reveal a significant reduction of Q2 species in the 70ZP glass. In addition, NZnO of 3.6 and 3.8 were calculated at atomic distances of up to 2.4 Å for 60ZP and 70ZP glasses, respectively. The Zn–O coordination numbers obtained from RMC modelling agree with the data determined experimentally by EXAFS, which is very sensitive to the Zn–O coordination, but are smaller than some of the values reported in previous studies34,37,38. To confirm the reduced Zn–O coordination in the glass, the FT EXAFS spectra and neutron total correlation functions T(r) for the different types of ZP glass were compared with those of ZnO and β-Zn2P2O7 crystals. As can be seen in Supplementary Fig. 5a, the Zn–O atomic distance in FT EXAFS spectra of the ZP glass is slightly shorter than that of ZnO crystal. In addition, the T(r) of β-Zn2P2O7 crystal shown in Supplementary Fig. 5b has two Zn–O atomic distances at 2.03 Å (Zn–O(I)) and at 2.30 Å (Zn–O(II)). The NZnO calculated up to the first and the second coordination distances are 4 and 2, respectively. Thus, the correlation peak at 2.03 Å of T(r) for β-Zn2P2O7 crystal is assigned to tetrahedral coordinated zinc. On the other hand, the Zn–O atomic distance is shorter and the peak area in the ZP glass is smaller than those in ZnO and β-Zn2P2O7 crystal. These observations above suggest that the Zn–O coordination in ZP glass is different from that in ZnO and β-Zn2P2O7 crystal, although fourfold zinc is dominant in glasses as Walter et al. concluded30.
Discussion
Thus, we have succeeded in modelling atomic configurations, which reproduce the X-ray, neutron, EXAFS and NMR data. To understand network formation in the RMC models, the Qn distribution was calculated on the basis of the atomic configurations obtained from said models. Q0:Q1:Q2:Q3:Q4 ratios of 0.8:49.8:49.0:0.4:0 and 33.8:65.8:0.4:0:0, obtained for the 60ZP and the 70ZP, respectively, concurred with the results of NMR shown in Fig. 2b and those reported in previous studies29,30,31,35. However, although Hoppe et al. reported on the structure of ZP glass derived from RMC modelling using X-ray and neutron diffraction data, we are not aware of any structure models that reproduce diffraction, EXAFS and NMR data. Accordingly, it is demonstrated that our model is reliable not at only short-range but also at intermediate range, including the connectivity of polyhedra, because our obtained Qn distribution was calculated by analysing the –O–P–O– connectivity (not simply estimating on the basis of the coordination numbers reported in ref. 39 (see Methods for details)). The results of Qn analysis and connectivity of atoms together with coordination numbers are listed in Supplementary Table 4. To understand the connectivity of the network consisting of PO4 tetrahedra and Zn–O polyhedra in detail, oxygen–cation coordination number distributions were calculated, as shown in Fig. 5. As can be seen in Fig. 5a,b, it is found that OP2 is more dominant than OZn2 in 60ZP glass, while OZn2 is more dominant than OP2 in 70ZP glass, demonstrating that the role of network formation changes from PO4 tetrahedra to Zn–O polyhedra between 60ZP and 70ZP, although Fig. 5c suggests that twofold oxygen is taken by an interplay between the PO4 tetrahedra and Zn–O polyhedra. The change in the role of the network formation from PO4 tetrahedra to Zn–O polyhedra between 60ZP and 70ZP can explain the behaviour of the thermal expansion coefficient. It is also found that 33% of the oxygen is coordinated by three cations (9% is P2–O–Zn1 and 24% is P1–O–Zn2) in 70ZP glass. Another important feature in oxygen–cation coordination is that the cation coordination numbers are increased in 70ZP glass. To understand such connectivity in 3D atomic configuration, the connectivity of PO4 and Zn–O polyhedra were analysed. Figure 6 shows the 3D linkage of the phosphate network in terms of the chain length, as calculated from the total number of atoms in each phosphate; Fig. 6a shows the fraction of Q1 units and Q2 chains of PO4 polyhedra in the 60ZP glass and a typical RMC-modelled Q2 chain. As can be seen in Fig. 6b, the 70ZP glass consists of only isolated PO4 tetrahedra (Q0) and P2O7 dimers (Q1). Thus, our RMC models reproduce the modification of the 3D phosphate network as observed by 31P MAS NMR. Similar behaviour is observed in binary silicate and aluminate glass with low glass-forming ability40,41,42. This observation is significant since low-melting-point phosphate glass is essential to many commercial applications.
The probabilities of the formation of polyhedral connections between PO4 tetrahedra and Zn–O polyhedra were calculated (Supplementary Table 5) to elucidate the mechanism of glass formation in binary oxide glass with low amounts of NWF. Corner-sharing between PO4–PO4, PO4–Zn–O polyhedra and Zn–O polyhedra–Zn–O polyhedra occurred predominantly, as stipulated by Zachariasen’s rule1. Both types of ZP glass exhibit the same polyhedral connections, to which their similar Tg and longitudinal modulus can be attributed. Furthermore, the total number of atoms constituting the ZnxOy units (Fig. 7) was estimated to determine the origin of the glass network in the glass. As the figure shows, the ZnxOy units do not form a network in 60ZP glass, and the size of the fragment (consisting of up to 41 atoms, as shown in the inset of Fig. 7a) is <20 Å. In contrast, 10% of the ZnxOy units in the 70ZP glass form networks consisting of more than 40 atoms, as manifested by their atomic configurations consisting of up to 1,300 atoms, as shown in the inset of Fig. 7b. The unusual network structure units formed by the ZnxOy polyhedra can be attributed to the smaller oxygen coordination of the zinc compared to that of the ZnO crystal; the high glass-forming ability of the 70ZP glass stems from this small oxygen coordination. Although the Zn–O coordination number is larger in the RMC model recently reported by Hoppe et al.34, they attributed the increased oxygen coordination around the zinc to the increased rigidity of the ZP in the 75ZP and 80ZP glass. On the other hand, it is suggested from our RMC model that the small Zn–O coordination is a signature of the NWF, according to Zachariasen’s rule1, in our model for 70ZP glass. This suggestion is supported by the small oxygen–cation coordinations (2.06 in 60ZP and 2.29 in 70ZP) listed in Supplementary Table 4, although the oxygen–cation coordination number is increased in 70ZP glass.
In this study, several types of binary ZnO–P2O5 glass are prepared with a wide range of chemical compositions. The present findings regarding the 3D network formation of ZnxOy units suggest that interplay between the PO4 tetrahedra and Zn–O polyhedra is important for tuning the physical properties of the glass. A small Zn–O coordination number might be important for improving the glass-forming ability and to increase the elastic modulus owing to this interplay. On the other hand, the addition of ZnO results in an increased oxygen–cation coordination number, which indicates an increased glass transition temperature, refractive index and packing fraction, and hence longitudinal modulus. Furthermore, we find that the thermal expansion coefficient sensitively reflects the substitution of the phosphate chain network by a network consisting of ZnxOy units in zinc-rich glass. In other words, we succeed in revealing, for the first time, the relationship between the atomic structure of glass and its functionality by using structural modelling based on a combination of an advanced quantum beam technique and spectroscopic measurement. Since phosphate glass exhibits a low melting point, then both the phosphate and glass networks consist of counter metal oxides, which give rise to this low-melting-point behaviour. The results obtained in this study, via several structural analysis methods, are significant since they clearly reveal the fundamental properties that determine the functionality of these types of glasses and may be useful in the design of phosphate glasses for practical applications.
Methods
Preparation of binary ZP glass
The different types of binary ZnO–P2O5 (xZP) glass, namely, 58ZnO–42P2O5 (58ZP), 60ZnO–40P2O5 (60ZP), 65ZnO–35P2O5 (65ZP) and 70ZnO–30P2O5 (70ZP), were prepared by a conventional melt-quenching method using a platinum crucible47. Batches consisting of ZnO (99.99%) and (NH4)2HPO4 (99%) were initially calcined at 800 °C for 3 h in an ambient atmosphere. The calcined solid was then melted at 1,100 °C for 30 min in an ambient atmosphere. The glass melt was quenched on a stainless plate maintained at 200 °C and then annealed at the glass transition temperature, Tg, for 1 h. The samples were then mechanically polished to produce a mirror surface.
Compositional analysis
Chemical compositions of ZP glasses were determined by using inductively coupled plasma-atomic emission spectrometry analysis using a SPECTRO BLUE (SPECTRO, Germany). The standard solutions (1,5,10 and 15 p.p.m.) of each element for the calibration curves were prepared by mixing each 1,000 p.p.m. standard solution with 0.1 M HNO3 solution. The calibration curves at different wavelengths of each element are shown in Supplementary Fig. 1. It was found that the calibration curves show a good linearly for both Zn and P. The concentrations of zinc and phosphorus are calculated by using averaged values of these three emission bands shown in Supplementary Fig. 1. The measured concentration values are summarized in Supplementary Table 1. The ZnO fraction fZnO of the glasses, that is, chemical composition of ZnO–P2O5 glasses, can be obtained from both concentration of Zn, cZn, and that of P, cP, by using the following equation:

From two samples of each composition, average ZnO fraction can be calculated.
Physicochemical analysis
The Tg was determined from thermomechanical analysis (TMA) at a heating rate of 10 °C min−1 and under a 1.0 g loading using a TMA 8310 (Rigaku, Japan). The linear thermal expansion coefficient of the samples, measuring around 4 mm × 4 mm × 15 mm, was also evaluated using the same equipment at a temperature range of 200–350 °C. Moreover, the local coordination state of phosphorus was determined by measuring the 31P MAS NMR spectra using a CMX-400 NMR spectrometer (JEOL, Japan). A frequency, spin rate and pulse delay of 161.80 MHz, 10 kHz and 5 s, respectively, were used in the measurements. The chemical shifts were estimated with respect to H3PO4 in a D2O solution (0 p.p.m.) and the conventional notation for phosphorus sites, Qn, was used for the analysis. The n value denotes the number of bridging oxygens per PO4 tetrahedron. Furthermore, the densities were measured by applying the Archimedes method using water at room temperature. We measured the refractive index of the samples using a prism coupler with a 473, 633, 1,319 and 1,553 nm light source (Metericon, NJ, USA); the error in the measurement was 10−4.
Elastic modulus measurement
The Brillouin shifts νB of the different types of glass were measured using a high-resolution modification of a Sandercock Fabry–Perot system48. A frequency-doubled diode-pumped solid state neodymium:yttrium–aluminium–garnet laser oscillating in a single longitudinal mode at 532 nm (Oxxius SLIM-532 300 mW) was used as the excitation source. In addition to the excitation laser source, a second weak reference laser was also used to act as an independent reference signal that was used for stabilization of the Fabry–Perot. The reference laser was a small diode-pumped solid state neodymium:yttrium–vanadate (Nd:YVO4) laser module (Photonic Products 300-0088-01, 4 mW) oscillating in single transverse mode (TEM00), which had two to three longitudinal modes separated by 120 GHz. The longitudinal sound velocity VL, shown in Supplementary Table 2, was calculated from VL=νBλ/ 2n532, where νB, λ and n532 are the Brillouin shift, the wavelength of incident light (=532 nm) and the refractive index at 532 nm, respectively. The n532 values were calculated from the Cauchy relationship between the refractive indices at different wavelengths.
EXAFS measurement
The Zn K-edge (9.66 keV) EXAFS spectra were measured at the BL01B1 beamline of SPring-8 synchrotron radiation facility (Hyogo, Japan). The measurements were performed using a Si (111) double-crystal monochromator in the transmission mode (Quick Scan method) at room temperature. Pellet samples for the measurements were prepared by mixing the granular sample with boron nitride. The corresponding analyses were performed by using REX2000 software (Rigaku)49.
High-energy X-ray diffraction measurement
The high-energy X-ray diffraction experiment was performed at the BL04B2 beamline at the SPring-8 synchrotron radiation facility, using a two-axis diffractometer dedicated to the study of disordered materials50. The energy of the incident X-rays was 61.4 keV. The raw data were corrected for polarization, absorption and the background, and the contribution of Compton scattering was subtracted by using standard data analysis software50.
RMC modelling
RMC modelling of the 60ZP and the 70ZP glass was performed using RMC++ code51. The start configurations, which contained 5,000 and 5,250 particles for the 60ZP and the 70ZP, respectively, were created using hard-sphere Monte Carlo simulations with constraints applied to avoid physically unrealistic structures. The r-spacing for the calculations of partial-pair correlation functions was set to be 0.05 Å. Two kinds of constraints were used: a closest atom–atom approach and connectivity. The closest atom–atom approach was chosen based on the need to avoid unreasonable spikes in the partial-pair correlation functions. The constraint on the P–O connectivity was that all of the phosphorus atoms were coordinated to four oxygen atoms for atomic distances of up to 1.7 Å. In addition, fixed neighbour constraints52 were applied for P–O at 1.55–1.7 Å and P=O at 1.4–1.55 Å to reproduce the Q0:Q1:Q2:Q3 ratio based on the results of the NMR in which the Q0:Q1:Q2:Q3 ratio is 0:50:50:0 for 60ZP and 33.3:66.7:0:0 for 70ZP. After the hard-sphere Monte Carlo simulations, RMC simulations containing X-ray S(Q), neutron S(Q) and k3χ(k) EXAFS data, measured at the Zn K-edge, were performed. The EXAFS back scattering tables were obtained from FEFF calculations53.
Data availability
The authors declare that all relevant data supporting the findings of this study are available from the corresponding authors on request.
Additional information
How to cite this article: Onodera, Y. et al. Formation of metallic cation-oxygen network for anomalous thermal expansion coefficients in binary phosphate glass. Nat. Commun. 8, 15449 doi: 10.1038/ncomms15449 (2017).
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
References
Zachariasen, W. H. The atomic arrangement in glass. J. Am. Chem. Soc. 54, 3841–3851 (1932).
Warren, B. E. & Biscoe, J. The structure of silica glass by X-ray diffraction studies. J. Am. Ceram. Soc. 21, 49–54 (1938).
Du, J. & Cormack, A. N. The medium range structure of sodium silicate glasses: a molecular dynamics simulation. J. Non-Cryst. Solids 349, 66–79 (2004).
Du, J. & Corrales, L. R. First sharp diffraction peak in silicate glasses: structure and scattering length dependence. Phys. Rev. B 72, 092201 (2005).
Du, J. & Corrales, L. R. Compositional dependence of the first sharp diffraction peaks in alkali silicate glasses: a molecular dynamics study. J. Non-Cryst. Solids 352, 3255–3269 (2006).
Pedone, A., Malavasi, G., Cormack, A. N., Segre, U. & Menziani, M. C. Insight into elastic properties of binary alkali silicate glasses; prediction and interpretation through atomistic simulation techniques. Chem. Mater. 19, 3144–3154 (2007).
Brow, R. K. Review: the structure of simple phosphate glasses. J. Non-Cryst. Solids 263–264, 1–28 (2000).
Martin, S. W. Review of the structure of phosphate glasses. Euro. J. Solid State Inorg. Chem. 28, 163–205 (1991).
Hoppe, U. A structural model for phosphate glasses. J. Non-Cryst. Solids 195, 138–147 (1996).
Sayer, M. & Mansingh, A. Transport properties of semiconducting phosphate glasses. Phys. Rev. B 6, 4629–4643 (1972).
Masai, H. et al. 31P NMR and IR study of highly water-stable SrO-BaO-Nb2O5-P2O5 glass and glass-ceramics. Chem. Lett. 42, 1305–1307 (2013).
Takamatsu, Y. et al. The state of P=Onb non-bridging oxygen and proton incorporation in binary MO·P2O5 (M = Ca, Mg) phosphate glasses. Solid State Ionics 245–246, 19–23 (2013).
Bunker, B. C., Arnold, G. W. & Wilder, J. A. Phosphate glass dissolution in aqueous solutions. J. Non-Cryst. Solids 64, 291–316 (1984).
Kokubo, T. & Takadama, H. How useful is SBF in predicting in vivo bone bioactivity? Biomaterials 27, 2907–2915 (2006).
Paulose, P. I., Jose, G., Thomas, V., Unnikrishnan, N. V. & Warrier, M. K. R. Sensitized fluorescence of Ce3+/Mn2+ system in phosphate glass. J. Phys. Chem. Solids 64, 841–846 (2003).
Campbell, J. H. & Suratwala, T. I. Nd-doped phosphate glasses for high-energy/high-peak-power lasers. J. Non-Cryst. Solids 263, 318–341 (2000).
Yan, Y. C., Faber, A. J., de Waal, H., Kik, P. G. & Polman, A. Erbium-doped phosphate glass waveguide on silicon with 4.1 dB/cm gain at 1.535 μm. Appl. Phys. Lett. 71, 2922–2924 (1997).
Inaba, S., Hosono, H. & Ito, S. Entropic shrinkage of an oxide glass. Nat. Mater. 14, 312–317 (2015).
Brow, R. K. & Tallant, D. R. Structural design of sealing glasses. J. Non-Cryst. Solids 222, 396–406 (1997).
Morena, R. Phosphate glasses as alternatives to Pb-based sealing frits. J. Non-Cryst. Solids 263–264, 382–387 (2000).
Joshi, B. C. Enhanced Eu3+ emission by nonradiative energy-transfer from Tb3+ in zinc phosphate glass. J. Non-Cryst. Solids 180, 217–220 (1995).
Caldino, U., Hernandez-Pozos, J. L., Flores, C., Speghini, A. & Bettinelli, M. Photoluminescence of Ce3+ and Mn2+ in zinc metaphosphate glasses. J. Phys.: Condens. Matter 17, 7297–7305 (2005).
Masai, H., Takahashi, Y., Fujiwara, T., Matsumoto, S. & Yoko, T. High photoluminescent property of low-melting Sn-doped phosphate glass. Appl. Phys. Express 3, 082102 (2010).
Masai, H. et al. White light emission of Mn-doped SnO-ZnO-P2O5 glass containing no rare earth cation. Opt. Lett. 36, 2868–2870 (2011).
Masai, H. et al. Correlation between emission property and concentration of Sn2+ centre in the SnO-ZnO-P2O5 glass. Opt. Express 20, 27319–27326 (2012).
Özgür, Ü. A comprehensive review of ZnO materials and devices. J. Appl. Phys. 98, 041301 (2005).
Onyiriuka, E. C. Zinc phosphate glass surfaces studied by XPS. J. Non-Cryst. Solids 163, 268–273 (1993).
Brow, R. K. An XPS study of oxygen bonding in zinc phosphate and zinc borophosphate glasses. J. Non-Cryst. Solids 194, 267–273 (1996).
Brow, R. K., Tallant, D. R., Myers, S. T. & Phifer, C. C. The short-range structure of zinc polyphosphate glass. J. Non-Cryst. Solids 191, 45–55 (1995).
Walter, G., Hoppe, U., Vogel, J., Carl, G. & Hartmann, P. The structure of zinc polyphosphate glass studied by diffraction methods and 31P NMR. J. Non-Cryst. Solids 333, 252–262 (2004).
Wiench, J. W., Pruski, M., Tischendorf, B., Otaigbe, J. U. & Sales, B. C. Structural studies of zinc polyphosphate glasses by nuclear magnetic resonance. J. Non-Cryst. Solids 263–264, 101–110 (2000).
Hoppe, U. et al. Combined neutron and X-ray scattering study of phosphate glasses. J. Non-Cryst. Solids 293–295, 158–168 (2001).
Walter, G., Hoppe, U., Baade, T., Kranold, R. & Stachel, D. Intermediate range order in MeO-P2O5 glasses. J. Non-Cryst. Solids 217, 299–307 (1997).
Hoppe, U., Dimitriev, Y. & Jóvári, P. Structure of zinc phosphate glasses of 75 and 80 mole% ZnO content studied by X-ray diffraction and reverse Monte Carlo simulations. Z. Naturforsch. A 60, 517–526 (2005).
Tischendorf, B., Otaigbe, J. U., Wiench, J. W., Pruski, M. & Sales, B. C. A study of short and intermediate range order in zinc phosphate glasses. J. Non-Cryst. Solids 282, 147–158 (2001).
Saout, G. L. et al. A multispectroscopic study of PbOxZnO0.6-x(P2O5)0.4 glasses. J. Non-Cryst. Solids 293–295, 657–662 (2001).
Tischendorf, B., Alam, T. M., Cygan, R. T. & Otaigbe, J. U. The structure and properties of binary zinc phosphate glasses studied by molecular dynamics simulations. J. Non-Cryst. Solids 316, 261–272 (2003).
Suzuya, K., Itoh, K., Kajinami, A. & Loong, C.-K. The structure of binary zinc phosphate glasses. J. Non-Cryst. Solids 345, 80–87 (2004).
Hoppe, U., Walter, G., Carl, G., Neuefeind, J. & Hannon, A. C. Structure of zinc phosphate glasses probed by neutron and X-ray diffraction of high resolving power and by reverse Monte Carlo simulations. J. Non-Cryst. Solids 351, 1020–1031 (2005).
Kohara, S. et al. Glass formation at the limit of insufficient network formers. Science 303, 1649–1652 (2004).
Kohara, S. et al. Relationship between topological order and glass forming ability in densely packed enstatite and forsterite composition glasses. Proc. Natl Acad. Sci. USA 108, 14780–14785 (2011).
Akola, J. et al. Network topology for the formation of solvated electrons in binary CaO–Al2O3 composition glasses. Proc. Natl Acad. Sci. USA 110, 10129–10134 (2013).
Kohara, S. et al. Atomic and electronic structures of an extremely fragile liquid. Nat. Commun. 5, 5892 (2014).
Tomozawa, M., Takata, M., Acocella, J., Watson, E. B. & Takamori, T. Thermal properties of Na2O-3SiO2 glasses with high water content. J. Non-Cryst. Solids 56, 343–348 (1983).
Shelby, J. E. Thermal expansion of alkali borate glasses. J. Am. Ceram. Soc. 66, 225–227 (1983).
Kirkpatrick, R. J. & Brow, R. K. Nuclear magnetic resonance investigation of the structures of phosphate and phosphate-containing glasses: a review. Solid State Nucl. Mag. 5, 9–21 (1995).
Masai, H. et al. Fabrication of Sn-doped zinc phosphate glass using a platinum crucible. J. Non-Cryst. Solids 358, 265–269 (2012).
Koreeda, A. & Saikan, S. Note: higher resolution Brillouin spectroscopy by offset stabilization of a tandem Fabry-Pérot interferometer. Rev. Sci. Instrum 82, 126103 (2011).
Taguchi, T., Ozawa, T. & Yashiro, H. REX2000: yet another XAFS analysis package. Phys. Scripta T115, 205–206 (2005).
Kohara, S. et al. Structural studies of disordered materials using high-energy x-ray diffraction from ambient to extreme conditions. J. Phys. Condens. Matter 19, 506101 (2007).
Gereben, O., Jóvári, P., Temleitner, L. & Pusztai, L. A new version of the RMC++, reverse Monte Carlo programme, aimed at investigating the structure of covalent glasses. J. Optoelectron. Adv. Mater. 9, 3021–3027 (2007).
Evrard, G. & Pusztai, L. Reverse Monte Carlo modelling of the structure of disordered materials with RMC++: a new implementation of the algorithm in C++. J. Phys. Condens. Matter 17, S1–S13 (2005).
Ankudinov, A. L., Ravel, B., Rehr, J. J. & Conradson, S. D. Real-space multiple-scattering calculation and interpretation of X-ray-absorption near-edge structure. Phys. Rev. B 58, 7565–7576 (1998).
Acknowledgements
This work was partially supported by the JSPS KAKENHI Grant-in-Aid for Young Scientists (A) Number 26709048, the Izumi Science and Technology Foundation and the Collaborative Research Program of I.C.R., Kyoto University (grant Nos. 2015-69 and 2016-47). We thank Prof Y. Sohrin, Dr T. Minami and Mr M. Tsujisaka (I.C.R., Kyoto University) for supporting the inductively coupled plasma-atomic emission spectrometry measurement. The synchrotron radiation experiments were performed at the BL01B1 and the BL04B2 of SPring-8 with the approval of the Japan Synchrotron Radiation Research Institute (JASRI; Proposal Nos. 2013A1412, 2014A1134, 2015A1311, 2016A0130 and 2016A0134). We thank Dr T. Ina for supporting the EXAFS measurements at the BL01B1. This work was also supported by JST-PRESTO ‘Advanced Materials Informatics through Comprehensive Integration among Theoretical, Experimental, Computational and Data-Centric Sciences’ (JPMJPR15N4) and the ‘Materials Research by Information Integration’ Initiative (MI2I) project of the Support Program for Starting Up Innovation Hub from JST. We gratefully acknowledge Dr K. Suzuya for providing us with the neutron diffraction data for ZnO–P2O5.
Author information
Authors and Affiliations
Contributions
H.M. and S.K. formulated the research project; H.M. and S.O. performed the materials preparation and EXAFS analysis; H.M. and A.K. measured the elastic modulus using Brillouin scattering; H.M. performed the thermal expansion, refractive index and inductively coupled plasma-atomic emission spectrometry measurements; T.O. measured 31P MAS NMR spectra and analysed the resulting data; S.K. performed X-ray diffraction measurements and analysed the resulting data; Y.O. and S.K. performed RMC modelling; Y.O., H.M. and S.K. wrote the paper.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
Supplementary Figures and Supplementary Tables (PDF 472 kb)
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Onodera, Y., Kohara, S., Masai, H. et al. Formation of metallic cation-oxygen network for anomalous thermal expansion coefficients in binary phosphate glass. Nat Commun 8, 15449 (2017). https://doi.org/10.1038/ncomms15449
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/ncomms15449
This article is cited by
-
Formation of a zirconium oxide crystal nucleus in the initial nucleation stage in aluminosilicate glass investigated by X-ray multiscale analysis
NPG Asia Materials (2024)
-
Combinatorial characterization of metastable luminous silver cations
Scientific Reports (2024)
-
Sustainable concrete for circular economy: a review on use of waste glass
Glass Structures & Engineering (2022)
-
Examination of structure and optical properties of Ce3+-doped strontium borate glass by regression analysis
Scientific Reports (2021)
-
Photocatalytic hydrogen generation of monolithic porous titanium oxide-based glass–ceramics
Scientific Reports (2020)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.