Abstract
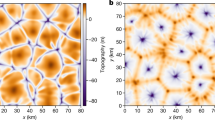
The vast, deep, volatile-ice-filled basin informally named Sputnik Planum is central to Pluto’s vigorous geological activity1,2. Composed of molecular nitrogen, methane, and carbon monoxide ices3, but dominated by nitrogen ice, this layer is organized into cells or polygons, typically about 10 to 40 kilometres across, that resemble the surface manifestation of solid-state convection1,2. Here we report, on the basis of available rheological measurements4, that solid layers of nitrogen ice with a thickness in excess of about one kilometre should undergo convection for estimated present-day heat-flow conditions on Pluto. More importantly, we show numerically that convective overturn in a several-kilometre-thick layer of solid nitrogen can explain the great lateral width of the cells. The temperature dependence of nitrogen-ice viscosity implies that the ice layer convects in the so-called sluggish lid regime5, a unique convective mode not previously definitively observed in the Solar System. Average surface horizontal velocities of a few centimetres a year imply surface transport or renewal times of about 500,000 years, well under the ten-million-year upper-limit crater retention age for Sputnik Planum2. Similar convective surface renewal may also occur on other dwarf planets in the Kuiper belt, which may help to explain the high albedos shown by some of these bodies.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Stern, S. A. et al. The Pluto system: initial results from its exploration by New Horizons. Science 350, aad1815 (2015)
Moore, J. M. et al. The geology of Pluto and Charon through the eyes of New Horizons. Science 351, 1284–1293 (2016)
Grundy, W. et al. Surface compositions across Pluto and Charon. Science 351, aad9189 (2016)
Yamashita, Y., Kato, M. & Arakawa, M. Experimental study on the rheological properties of polycrystalline solid nitrogen and methane: implications for tectonic processes on Triton. Icarus 207, 972–977 (2010)
Hammond, N. P. & Barr, A. C. Formation of Ganymede’s grooved terrain by convection-driven resurfacing. Icarus 227, 206–209 (2014)
Schenk, P. M. et al. A large impact origin for Sputnik Planum and surrounding terrains, Pluto? AAS/Div. Planet. Sci. Meeting 47, abstr. 200.06 (2015)
Greenstreet, S., Gladman, B. & McKinnon, W. B. Impact and cratering rates onto Pluto. Icarus 258, 267–288 (2015)
Moore, J. M. et al. Geology before Pluto: pre-encounter considerations. Icarus 246, 65–81 (2015)
Stern, S. A., Porter, S. B. & Zangari, A. M. On the roles of escape erosion and the viscous relaxation of craters on Pluto. Icarus 250, 287–293 (2015)
Eluszkiewicz, J. & Stevenson, D. J. Rheology of solid methane and nitrogen: application to Triton. Geophys. Res. Lett. 17, 1753–1756 (1990)
Protopapa, S. et al. Methane to nitrogen mixing ratio across the surface of Pluto. Proc. Lunar Planet. Sci. Conf. 47, abstr. 2815 (2016)
Eluszkiewicz, J. On the microphysical state of the surface of Triton. J. Geophys. Res. 96, 19217–19229 (1991)
Solomatov, V. S. Scaling of temperature- and stress-dependent viscosity convection. Phys. Fluids 7, 266–274 (1995)
Stansberry, J. A. & Yelle, R. V. Emissivity and the fate of Pluto’s atmosphere. Icarus 141, 299–306 (1999)
Scott, T. A. Solid and liquid nitrogen. Phys. Rep. (Phys. Lett. C) 27, 89–157 (1976)
McKinnon, W. B., Simonelli, D. & Schubert, G. in Pluto and Charon (eds Stern, S. A. & Tholen, D. J. ) 259–343 (Univ. Arizona Press, 1997)
Robuchon, G. & Nimmo, F. Thermal evolution of Pluto and implications for surface tectonics and a subsurface ocean. Icarus 216, 426–439 (2011)
Lodders, K. Solar System abundances and condensation temperatures of the elements. Astrophys. J. 591, 1220–1247 (2003)
McKinnon, W. B. et al. The Pluto-Charon system revealed: geophysics, activity, and origins. Lunar Planet. Sci. Conf. 47, abstr. 1995 (2016)
Robuchon, G., Nimmo, F., Roberts, J. & Kirchoff, M. Impact basin relaxation at Iapetus. Icarus 214, 82–90 (2011)
Estève, D. & Sullivan, N. S. NMR study of self-diffusion in solid N2 . Solid State Commun. 39, 969–971 (1981)
Moresi, L.-N. & Solomatov, V. S. Numerical investigation of 2D convection with extremely large viscosity variations. Phys. Fluids 7, 2154–2162 (1995)
Bland, M. T. & McKinnon, W. B. Forming Ganymede’s grooves at smaller strain: toward a self-consistent local and global strain history for Ganymede. Icarus 245, 247–262 (2015)
Singer, K. N. & Stern, S. A. On the provenance of Pluto’s nitrogen (N2). Astrophys. J. 808, L50 (2015)
Gladstone, G. R. et al. The atmosphere of Pluto as observed by New Horizons. Science 351, aad8866 (2016)
Brown, M. E. in The Solar System Beyond Neptune (eds Barucci, M. A., Boehnhardt, H., Cruikshank, D. & Morbidelli, A. ) 335–344 (Univ. Arizona Press, 2008)
Schenk, P. M., Wilson, R. R. & Davies, A. G. Shield volcano topography and the rheology of lava flows on Io. Icarus 169, 98–110 (2004)
Schenk, P. M. Thickness constraints on the icy shells of the Galilean satellites from a comparison of crater shapes. Nature 417, 419–421 (2002)
Frost, H. J. & Ashby, M. F. Deformation-Mechanism Maps: The Plasticity and Creep of Metals and Ceramics (Pergamon, 1982)
Solomatov, V. S. & Moresi, L.-N. Three regimes of mantle convection with non-Newtonian viscosity and stagnant lid convection on the terrestrial planets. Geophys. Res. Lett. 24, 1907–1910 (1997)
Barr, A. C. & McKinnon, W. B. Can Enceladus’ ice shell convect? Geophys. Res. Lett. 34, L09202 (2007)
Stengel, K. C., Oliver, D. S. & Booker, J. R. Onset of convection in a variable viscosity fluid. J. Fluid Mech. 120, 411–431 (1982)
Solomatov, V. S. & Barr, A. C. Onset of convection in fluids with strongly temperature-dependent, power-law viscosity: 2. Dependence on the initial perturbation. Phys. Earth Planet. Inter. 165, 1–13 (2007)
Schubert, G., Turcotte, D. L. & Olson, P. Mantle Convection in the Earth and Planets (Cambridge Univ. Press, 2001)
Goldsby, D. L. & Kohlstedt, D. L. Superplastic deformation of ice: experimental observations. J. Geophys. Res. 106, 11017–11030 (2001)
Barr, A. C. & McKinnon, W. B. Convection in ice I shells and mantles with self-consistent grain size. J. Geophys. Res. 112, E02012 (2007)
Cruikshank, D. P. et al. in Pluto and Charon (eds Stern, S. A. & Tholen, D. J. ) 221–267 (Univ. Arizona Press, 1997)
Durham, W. B., Prieto-Ballesteros, O., Goldsby, D. L. & Kargel, J. S. Rheological and thermal properties of icy materials. Space Sci. Rev. 153, 273–298 (2010)
Karato, S. & Wu, P. Rheology of the upper mantle: a synthesis. Science 260, 771–778 (1993)
Elbeshausen, D., Wünnemann, K. & Collins, G. S. The transition from circular to elliptical impact craters. J. Geophys. Res. 118, 2295–2309 (2013)
Acknowledgements
New Horizons was built and operated by the Johns Hopkins Applied Physics Laboratory (APL) in Laurel, Maryland, USA, for NASA. We thank the many engineers who have contributed to the success of the New Horizons mission, and NASA’s Deep Space Network (DSN) for a decade of support of New Horizons. This work was supported by NASA’s New Horizons project.
Author information
Authors and Affiliations
Consortia
Contributions
W.B.M. led the study and wrote the paper, with substantial input from F.N.; T.W. and J.H.R. performed the CitCom finite element convection calculations; P.M.S. developed the software to create stereographic and photoclinometric digital elevation models (DEMs) using New Horizons LORRI and MVIC images, and created the preliminary DEM for SP; O.L.W. mapped the SP region using New Horizons images in ArcGIS; J.M.M., J.R.S., A.D.H, O.M.U. and S.A.S. contributed to the understanding of the multiple roles N2 ice plays in the geology of SP and its environs. S.A.S., H.A.W., C.B.O., L.A.Y. and K.E.S. are the lead scientists of the New Horizons project. The entire Geology, Geophysics, and Imaging Theme Team (listed) contributed to the success of the Pluto encounter.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Additional information
All spacecraft data and higher-order products presented in this Letter will be delivered to NASA’s Planetary Data System (https://pds.nasa.gov) in a series of stages in 2016 and 2017 because of the time required to fully downlink and calibrate the data set.
Rights and permissions
About this article
Cite this article
McKinnon, W., Nimmo, F., Wong, T. et al. Convection in a volatile nitrogen-ice-rich layer drives Pluto’s geological vigour. Nature 534, 82–85 (2016). https://doi.org/10.1038/nature18289
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nature18289
This article is cited by
-
Large-scale cryovolcanic resurfacing on Pluto
Nature Communications (2022)
-
Sublimation-driven convection in Sputnik Planitia on Pluto
Nature (2021)
-
Recent Advancements and Motivations of Simulated Pluto Experiments
Space Science Reviews (2018)
-
The Pluto system after the New Horizons flyby
Nature Astronomy (2017)
-
Penitentes as the origin of the bladed terrain of Tartarus Dorsa on Pluto
Nature (2017)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.