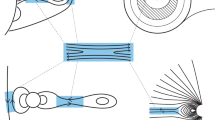
Abstract
Computer simulations have been playing an important role in the development of our understanding of the geodynamo1,2,3, but direct numerical simulation of the geodynamo with a realistic parameter regime is still beyond the power of today’s supercomputers. Difficulties in simulating the geodynamo arise from the extreme conditions of the core, which are characterized by very large or very small values of the non-dimensional parameters of the system. Among them, the Ekman number, E, has been adopted as a barometer of the distance of simulations from real core conditions, in which E is of the order of 10-15. Following the initial computer simulations of the geodynamo4,5, the Ekman number achieved has been steadily decreasing, with recent geodynamo simulations6,7,8 performed with E of the order of 10-6. Here we present a geodynamo simulation with an Ekman number of the order of 10-7—the highest-resolution simulation yet achieved, making use of 4,096 processors of the Earth Simulator. We have found that both the convection flow and magnetic field structures are qualitatively different from those found in larger-Ekman-number dynamos. The convection takes the form of sheet plumes or radial sheet jets9, rather than the columnar cell structures10 that are usually found. We have found that this sheet plume convection is an effective dynamo and the generated current is organized as a set of coils in the shape of helical springs or at times as a torus.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Roberts, P. H. & Glatzmaier, G. A. Geodynamo theory and simulations. Rev. Mod. Phys. 72, 1081–1123 (2000)
Jones, C. A. Convection-driven geodynamo models. Phil. Trans. R. Soc. Lond. A 358, 873–897 (2000)
Kono, M. & Roberts, P. H. Recent geodynamo simulations and observations of the geomagnetic field. Rev. Geophys. 40 1013 10.1029/2000RG000102 (2002)
Kageyama, A. et al. Computer simulation of a magnetohydrodynamic dynamo. II. Phys. Plasmas 2, 1421–1431 (1995)
Glatzmaier, G. A. & Roberts, P. H. A three-dimensional self-consistent computer simulation of a geomagnetic field reversal. Nature 377, 203–209 (1995)
Takahashi, F., Matsushima, M. & Honkura, Y. Simulations of a quasi-Taylor state geomagnetic field including polarity reversals on the Earth simulator. Science 309, 459–461 (2005)
Christensen, U. & Aubert, J. Scaling properties of convection-driven dynamos in rotating spherical shells and applications to planetary magnetic fields. Geophys. J. Int. 166, 97–114 (2006)
Sakuraba, A. & Hamano, Y. Turbulent structure in Earth’s fluid core inferred from time series of geomagnetic dipole moment. Geophys. Res. Lett. 34 L15308 10.1029/2007GL029898 (2007)
Sumita, I. & Olson, P. Laboratory experiments on high Rayleigh number thermal convection in a rapidly rotating hemispherical shell. Phys. Earth Planet. Inter. 117, 153–170 (2000)
Busse, F. A model of the geodynamo. Geophys. J. R. Astron. Soc. 42, 437–459 (1975)
Gilman, P. & Glatzmaier, G. Compressible convection in a rotating spherical shell. I. Anelastic equations. Astrophys. J. 45 (Suppl.). 335–349 (1981)
Zhang, K. & Schubert, G. Comparison of terrestrial and solar dynamos. Rep. Prog. Phys. 69, 1581–1605 (2006)
Kageyama, A. & Sato, T. “Yin-Yang grid”: An overset grid in spherical geometry. Geochem. Geophys. Geosyst. 5 10.1029/2004GC000734 (2004)
Kageyama, A. et al. A 15.2 TFlops simulation of geodynamo on the earth simulator. Proc. ACM/IEEE SC2004 Conf.–35–43 (2004)
Chesshire, G. & Henshaw, W. Composite overlapping meshes for the solution of partial differential equations. J. Comput. Phys. 90, 1–64 (1990)
Busse, F. Convective flows in rapidly rotating spheres and their dynamo action. Phys. Fluids 14, 1301–1314 (2002)
Plaut, E. & Busse, F. Multicellular convection in rotating annuli. J. Fluid Mech. 528, 119–133 (2005)
Aubert, J., Brito, D., Nataf, H.-C., Cardin, P. & Masson, J.-P. A systematic experimental study of rapidly rotating spherical convection in water and liquid gallium. Phys. Earth Planet. Inter. 128, 51–74 (2001)
Stellmach, S. & Hansen, U. Cartesian convection driven dynamos at low Ekman number. Phys. Rev. E 70, 056312 (2004)
Kageyama, A. & Sato, T. Generation mechanism of a dipole field by a magnetohydrodynamic dynamo. Phys. Rev. E 55, 4617–4626 (1997)
Davidson, P. An Introduction to Magnetohydrodynamics (Cambridge Univ. Press, 2001)
Aubert, J., Aurnou, J. & Wicht, J. The magnetic structure of convection-driven numerical dynamos. Geophys. J. Int. 172, 945–956 (2008)
Kageyama, A., Tamura, Y. & Sato, T. Visualization of vector field by virtual reality. Prog. Theor. Phys. 138 (Suppl.). 665–673 (2000)
Ohno, N. & Kageyama, A. Scientific visualization of geophysical simulation data by the CAVE VR system with volume rendering. Phys. Earth Planet. Inter. 163, 305–311 (2007)
Olson, P. & Christensen, U. R. Dipole moment scaling for convection-driven planetary dynamos. Earth Planet. Sci. Lett. 250, 561–571 (2006)
Ochi, M. M., Kageyama, A. & Sato, T. Dipole and octupole field reversals in a rotating spherical shell: Magnetohydrodynamic dynamo simulation. Phys. Plasmas 6, 777–787 (1999)
Kageyama, A., Ochi, M. M. & Sato, T. Flip-flop transitions of the magnetic intensity and polarity reversals in the magnetohydrodynamic dynamo. Phys. Rev. Lett. 82, 5409–5412 (1999)
Li, J., Sato, T. & Kageyama, A. Repeated and sudden reversals of the dipole field generated by a spherical dynamo action. Science 295, 1887–1890 (2002)
Cruz-Neira, C., Sandin, D. & DeFanti, T. Surrounded-screen projection-based virtual reality. Proc. SIGGRAPH 93, 135–142 (1993)
Acknowledgements
We thank P. Olson and J. Aurnou for pointing out the close connection between the sheet plume structure observed in this simulation and the experiments of ref. 9. We thank I. Sumita for a detailed explanation of his laboratory experiments and comments, and for providing Fig. 2a. We thank N. Ohno for helping with the visualization. This work was supported by KAKENHI (17540404) and The Mitsubishi Foundation.
Author Contributions A.K. was involved in project planning, simulation code development, simulation runs, data analysis and manuscript preparation. T.M. was involved in a part of the simulation runs, data analysis and manuscript preparation. T.S. was involved in project planning and manuscript preparation.
Author information
Authors and Affiliations
Corresponding author
Supplementary information
Supplementary Figures
The file contains Supplementary Figures 1-2 and Legends. Supplementary Figure 1 shows an equatorial cross section of the convection velocity at Ekman number E=2.3e-7. Supplementary Figure 2 shows the current field at E=2.6e-6. Characteristic feature of the current field lines of helical coils is less distinctive in this case. (PDF 1230 kb)
Rights and permissions
About this article
Cite this article
Kageyama, A., Miyagoshi, T. & Sato, T. Formation of current coils in geodynamo simulations. Nature 454, 1106–1109 (2008). https://doi.org/10.1038/nature07227
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1038/nature07227
This article is cited by
-
In-situ visualization library for Yin-Yang grid simulations
Earth, Planets and Space (2021)
-
Recent progress in identification of the geomagnetic signature of 3D outer core flows
Acta Geodaetica et Geophysica (2020)
-
The competition between Lorentz and Coriolis forces in planetary dynamos
Progress in Earth and Planetary Science (2015)
-
Effect of electromagnetic boundary condition on dynamo actions
Science China Physics, Mechanics & Astronomy (2015)
-
Zonal flow formation in the Earth’s core
Nature (2010)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.