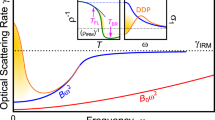
Abstract
Landau–Fermi liquid theory, with its pivotal assertion that electrons in metals can be simply understood as independent particles with effective masses replacing the free electron mass, has been astonishingly successful. This is true despite the Coulomb interactions an electron experiences from the host crystal lattice, lattice defects and the other ∼1022 cm-3 electrons. An important extension to the theory accounts for the behaviour of doped semiconductors1,2. Because little in the vast literature on materials contradicts Fermi liquid theory and its extensions, exceptions have attracted great attention, and they include the high-temperature superconductors3, silicon-based field-effect transistors that host two-dimensional metals4, and certain rare-earth compounds at the threshold of magnetism5,6,7,8. The origin of the non-Fermi liquid behaviour in all of these systems remains controversial. Here we report that an entirely different and exceedingly simple class of materials—doped small-bandgap semiconductors near a metal–insulator transition—can also display a non-Fermi liquid state. Remarkably, a modest magnetic field functions as a switch which restores the ordinary disordered Fermi liquid. Our data suggest that we have found a physical realization of the only mathematically rigorous route to a non-Fermi liquid, namely the ‘undercompensated Kondo effect’, where there are too few mobile electrons to compensate for the spins of unpaired electrons localized on impurity atoms9,10,11,12.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Al’tshuler, B. L., Aronov, A. G., Gershenson, M. E. & Sharvin, Yu. V. Quantum effects in disordered metal films. Sov. Sci. Rev. Phys. 9, 223–354 (1987)
Lee, P. A. & Ramakrishnan, T. V. Disordered electron systems. Rev. Mod. Phys. 57, 287–337 (1985)
Cava, R. J. et al. Bulk superconductivity at 91 K in a single-phase oxygen-deficient perovskite Ba2YCu3O9–δ . Phys. Rev. Lett. 58, 1676–1679 (1987)
Kravchenko, S. V. et al. Electric field scaling at a B = 0 metal–insulator transition in two dimensions. Phys. Rev. Lett. 77, 4938–4941 (1996)
Custers, J. et al. The break-up of heavy electrons at a quantum critical point. Nature 424, 524–527 (2003)
Mathur, N. D. et al. Magnetically mediated superconductivity in heavy fermion compounds. Nature 394, 39–43 (1998)
Si, Q. M., Rabello, S., Ingersent, K. & Smith, J. L. Locally critical quantum phase transitions in strongly correlated metals. Nature 413, 804–808 (2001)
Schroder, A. et al. Onset of antiferromagnetism in heavy-fermion metals. Nature 407, 351–355 (2000)
Coleman, P. & Pepin, C. Singular Fermi liquid behavior in the underscreened Kondo model. Phys. Rev. B 68, 220405 (2003)
Mehta, P. et al. Regular and singular Fermi-liquid fixed points in quantum impurity models. Phys. Rev. B 72, 104430 (2005)
Posazhennikova, A. & Coleman, P. Anomalous conductance of a spin-1 quantum dot. Phys. Rev. Lett. 94, 036802 (2005)
Sacramento, P. D. & Schlottmann, P. Thermodynamics of the n-channel Kondo model for general n and impurity spin S in a magnetic field. J. Phys. Condens. Matter 3, 9687–9696 (1991)
Wernick, J. H., Wertheim, G. K. & Sherwood, R. C. Magnetic behavior of monosilicides of 3D-transition elements. Mater. Res. Bull 7, 1431–1441 (1972)
Schlesinger, Z. et al. Unconventional charge gap formation in FeSi. Phys. Rev. Lett. 71, 1748–1751 (1993)
Mattheiss, L. F. & Hamann, D. R. Band-structure and semiconducting properties of FeSi. Phys. Rev. B 47, 13114–13119 (1993)
Beille, J., Voiron, J. & Roth, M. Long period helimagnetism in the cubic-B20 Fe1–x Co x Si and Co1–x Mn x Si alloys. Solid State Commun. 47, 399–402 (1983)
DiTusa, J. F. et al. Metal–insulator transitions in the Kondo insulator FeSi and classic semiconductors are similar. Phys. Rev. Lett. 78, 2831–2834 (1997)
Chernikov, M. A. et al. Low-temperature transport, optical, magnetic, and thermodynamic properties of Fe1–x Co x Si. Phys. Rev. B 56, 1366–1375 (1997)
DiTusa, J. F. et al. Heavy fermion metal–Kondo insulator transition in FeSi1–x Al x . Phys. Rev. B 58, 10288–10301 (1998)
Manyala, N. et al. Magnetoresistance from quantum interference effects in ferromagnets. Nature 404, 581–584 (2000)
Manyala, N. et al. Large anomalous Hall effect in a silicon-based magnetic semiconductor. Nature Mater. 3, 255–262 (2004)
Ashcroft, N. W. & Mermin, N. D. Solid State Physics (Saunders College, Philadelphia, 1976)
Pfleiderer, C., Julian, S. R. & Lonzarich, G. G. Non-Fermi-liquid nature of the normal state of itinerant-electron ferromagnets. Nature 414, 427–430 (2001)
Rosenbaum, T. F. et al. Metal–insulator transition in a doped semiconductor. Phys. Rev. B 27, 7509–7523 (1983)
Husmann, A. et al. Dynamical signature of the Mott–Hubbard transition in Ni(S,Se)2 . Science 274, 1874–1876 (1996)
von Molnar, S., Briggs, A., Flouquet, J. & Remenyi, G. Electron localization in a magnetic semiconductor: Gd3–x v x S4 . Phys. Rev. Lett. 51, 706–709 (1983)
Paalanen, M. A., Graebner, J. E., Bhatt, R. N. & Sachdev, S. Thermodynamic behavior near a metal–insulator transition. Phys. Rev. Lett. 61, 597–600 (1988)
Lakner, M., von Lohneysen, H., Langenfeld, A. & Wolf, P. Localized magnetic-moments in Si:P near the metal–insulator transition. Phys. Rev. B 50, 17064–17073 (1994)
Bhatt, R. N. & Lee, P. A. Scaling studies of highly disordered spin-1/2 antiferromagnetic systems. Phys. Rev. Lett. 48, 344–347 (1982)
Sarachik, M. P. et al. Scaling behavior in the magnetization of insulating Si:P. Phys. Rev. B 34, 387–390 (1986)
Ghosh, S., Rosenbaum, T. F., Aeppli, G. & Coppersmith, S. N. Entangled quantum state of magnetic dipoles. Nature 425, 48–51 (2003)
Aeppli, G. & Fisk, Z. Kondo insulators. Comments Cond. Mater. Phys. 16, 155–165 (1992)
Acknowledgements
We thank Z. Fisk for discussions. J.F.D. acknowledges support from the National Science Foundation and G.A. acknowledges support from a Wolfson-Royal Society Research Merit Award and the Basic Technologies Programme of the UK Research Councils.
Author information
Authors and Affiliations
Corresponding author
Supplementary information
Supplementary Notes
The file contains Supplementary Notes on the magnetoconductance and the magnetization of the materials presented in the main paper. It includes references and figure captions for the two Supplementary Figures S1-S2. (PDF 291 kb)
Rights and permissions
About this article
Cite this article
Manyala, N., DiTusa, J., Aeppli, G. et al. Doping a semiconductor to create an unconventional metal. Nature 454, 976–980 (2008). https://doi.org/10.1038/nature07137
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1038/nature07137
This article is cited by
-
Observation of Kondo condensation in a degenerately doped silicon metal
Nature Physics (2023)
-
Many-body Hamiltonian with screening parameter and ionization energy
Pramana (2010)
-
Long, short, entangled, cut off...
Nature Physics (2008)
-
A metal left spinning
Nature (2008)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.