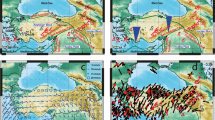
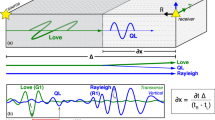
Abstract
Differences in the thickness of the high-velocity lid underlying continents as imaged by seismic tomography, have fuelled a long debate on the origin of the ‘roots’ of continents1,2,3,4,5. Some of these differences may be reconciled by observations of radial anisotropy between 250 and 300 km depth, with horizontally polarized shear waves travelling faster than vertically polarized ones2. This azimuthally averaged anisotropy could arise from present-day deformation at the base of the plate, as has been found for shallower depths beneath ocean basins6. Such deformation would also produce significant azimuthal variation, owing to the preferred alignment of highly anisotropic minerals7. Here we report global observations of surface-wave azimuthal anisotropy, which indicate that only the continental portion of the Australian plate displays significant azimuthal anisotropy and strong correlation with present-day plate motion in the depth range 175–300 km. Beneath other continents, azimuthal anisotropy is only weakly correlated with plate motion and its depth location is similar to that found beneath oceans. We infer that the fast-moving Australian plate contains the only continental region with a sufficiently large deformation at its base to be transformed into azimuthal anisotropy. Simple shear leading to anisotropy with a plunging axis of symmetry may explain the smaller azimuthal anisotropy beneath other continents.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
References
Jordan, T. H. The continental tectosphere. Rev. Geophys. 13, 1–12 (1975)
Gung, Y., Panning, M. & Romanowicz, B. Global anisotropy and the thickness of continents. Nature 422, 707–711 (2003)
Ekström, G. & Dziewonski, A. M. The unique anisotropy of the Pacific upper mantle. Nature 394, 168–172 (1998)
Ritsema, J., van Heijst, H. J. & Woodhouse, J. H. Global transition zone tomography. J. Geophys. Res. 109, B02302, doi:10.1029/2003JB002610 (2004)
Montagner, J. P. & Tanimoto, T. Global upper mantle tomography of seismic velocities and anisotropies. J. Geophys. Res. 96, 20337–20351 (1991)
Forsyth, D. W. The early structural evolution and anisotropy of the oceanic upper mantle. Geophys. J. R. Astron. Soc. 43, 103–162 (1975)
Nicolas, A. & Christensen, N. I. in Composition, Structure and Dynamics of the Lithosphere-Asthenosphere System (eds Fuchs, F. & Froidevaux, C.) 111–123 (Geodyn. Ser. Vol. 16, AGU, Washington DC, 1987)
Trampert, J. & Woodhouse, J. H. Global anisotropic phase velocity maps for the fundamental mode surface waves between 40 and 150 s. Geophys. J. Int. 154, 154–165 (2003)
Becker, T. W., Kellog, J. B., Ekstrom, G. & O'Connell, R. J. Comparison of azimuthal seismic anisotropy from surface waves and finite strain from global mantle-circulation models. Geophys. J. Int. 155, 696–714 (2003)
Debayle, E. SV-wave azimuthal anisotropy in the Australian upper-mantle: Preliminary results from automated Rayleigh waveform inversion. Geophys. J. Int. 137, 747–754 (1999)
Debayle, E. & Kennett, B. L. N. The Australian continental upper mantle: structure and deformation inferred from surface waves. J. Geophys. Res. 105, 25423–25450 (2000)
Debayle, E. & Kennett, B. L. N. Anisotropy in the Australasian upper mantle from Love and Rayleigh waveform inversion. Earth Planet. Sci. Lett. 184, 339–351 (2000)
Simons, F. J. & van der Hilst, R. D. Seismic and mechanical anisotropy and the past and present deformation of the Australian lithosphere. Earth Planet. Sci. Lett. 211, 271–286 (2003)
Park, J. & Levin, V. Seismic anisotropy: Tracing plate dynamics in the mantle. Science 296, 485–489 (2002)
Savage, M. K. Seismic anisotropy and mantle deformation: What have we learned from shear wave splitting? Rev. Geophys. 37, 65–106 (1999)
Ozalabey, S. & Chen, W. P. Frequency dependent analysis of SKS/SKKS waveforms observed in Australia: evidence for null birefringence. Phys. Earth Planet. Inter. 114, 197–210 (1999)
Clitheroe, G. & van der Hilst, R. D. in Structure and Evolution of the Australian Continent (eds Braun, J., Dooley, J., Goleby, B., van der Hilst, R. & Klootwijk, C.) 73–78 (Geodyn. Monogr. 26, AGU, Washington DC, 1998)
Zhang, S. & Karato, S. I. Lattice preferred orientation of olivine aggregate deformed in simple shear. Nature 375, 774–777 (1995)
Jung, H. & Karato, S. I. Water-induced fabric transitions in olivine. Science 293, 1460–1463 (2001)
Kaminski, E. The influence of water on the development of lattice preferred orientation in olivine aggregates. Geophys. Res. Lett 29, 12, doi:10.1029/2002GL014710 (2002)
Babuska, V. & Cara, M. Seismic Anisotropy in the Earth (Kluwer Academic, Dordrecht, 1991)
Beghein, C. & Trampert, J. Probability density functions for radial anisotropy: implications for the upper 1200 km of the mantle. Earth Planet. Sci. Lett. 217, 151–162 (2003)
Gaherty, J. B. & Jordan, T. Lehmann discontinuity as the base of an anisotropic layer beneath continents. Science 268,1468–1471 (1995)
Maupin, V. & Cara, M. Love-Rayleigh wave incompatibility and possible deep upper mantle anisotropy in the Iberian penninsula. Pure Appl. Geophys. 138, 429–444 (1992)
Debayle, E. & Sambridge, M. Inversion of massive surface wave data sets: Model construction and resolution assessment. J. Geophys. Res 109, B02316 doi:10.1029/2003JB002652, (2004)
Cara, M. & Lévêque, J. J. Waveform inversion using secondary observables. Geophys. Res. Lett. 14, 1046–1049 (1987)
Smith, M. & Dahlen, F. The azimuthal dependence of Love and Rayleigh wave propagation in a slightly anisotropic medium. J. Geophys. Res. 78, 3321–3333 (1973)
Montagner, J. P. & Nataf, H. C. A simple method for inverting the azimuthal anisotropy of surface waves. J. Geophys. Res. 91, 511–520 (1986)
Lévêque, J. J., Debayle, E. & Maupin, V. Anisotropy in the Indian Ocean upper mantle from Rayleigh- and Love-waveform inversion. Geophys. J. Int. 133, 529–540 (1998)
Montagner, J. P., Griot-Pommera, D. A. & Lavé, J. How to relate body wave and surface wave anisotropy? J. Geophys. Res. 105(B8), 19015–19027 (2000)
DeMets, C., Gordon, R. G., Argus, D. F. & Stein, S. Current plate motion. Geophys. J. Int. 101, 425–478 (1990)
Acknowledgements
This work was supported by programme DyETI conducted by the French Institut National des Sciences de l'Univers (INSU). The data used in this work were obtained from the GEOSCOPE, GDSN, IDA, MEDNET and GTSN permanent seismograph networks, and completed with data collected after the PASSCAL broadband experiments, the SKIPPY and subsequent broadband deployments in Australia, and the INSU deployments in the Horn of Africa and the Pacific (PLUME experiment). Supercomputer facilities were provided by the IDRIS and CINES national centres in France. Special thanks to J. M. Brendle at EOST for technical support, S. Fishwick for providing broadband data from the Western Australian craton field deployment, the staff of the Research School of Earth Science who collected the SKIPPY data in the field, and A. Maggi for suggestions that improved the manuscript.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Competing interests
The authors declare that they have no competing financial interests.
Supplementary information
Supplementary Figure 1
Ray path density per 400 × 400 km cell across the globe for the Rayleigh wave data set used to build our tomographic model. (PDF 346 kb)
Supplementary Figure 2
Distribution of epicentre-station lengths for the 100779 Rayleigh waveforms used in this study. (PDF 11 kb)
Supplementary Figure 3
Optimized Voronoi diagram based on the work of Debayle and Sambridge26. (PDF 374 kb)
Supplementary Figure 4
Influence of the non-inverted parameters: a, 2θ azimuthal anisotropy of the phase velocity predicted from integration of the G parameters. b, 2θ azimuthal anisotropy obtained from direct regionalization of the phase velocity curves. c, Same as b but the 4θ azimuthal terms are included in the inversion. (PDF 950 kb)
Supplementary Figure 5
Synthetic experiment to test vertical smearing. (PDF 27 kb)
Supplementary Figure 6
Predicted SKS splitting following Montagner et al.31 in the input model of Supplementary Fig. 5a (a);the inversion output of Supplementary Fig. 5b (b); and the model from the actual inversion (Fig. 1) (c). (PDF 64 kb)
Supplementary Figure Legends
Figure legends for Supplementary Figs 1-6. (PDF 7 kb)
Rights and permissions
About this article
Cite this article
Debayle, E., Kennett, B. & Priestley, K. Global azimuthal seismic anisotropy and the unique plate-motion deformation of Australia. Nature 433, 509–512 (2005). https://doi.org/10.1038/nature03247
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1038/nature03247
This article is cited by
-
Influence of the asthenosphere on earth dynamics and evolution
Scientific Reports (2023)
-
Sulfide metallogenic model for the ultraslow-spreading Southwest Indian Ridge
Science China Earth Sciences (2023)
-
Crust-mantle decoupling beneath Afar revealed by Rayleigh-wave tomography
Scientific Reports (2022)
-
Quasi-Love wave scattering reveals tectonic history of Australia and its margins reflected by mantle anisotropy
Communications Earth & Environment (2021)
-
Seismic evidence for subduction-induced mantle flows underneath Middle America
Nature Communications (2020)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.