Abstract
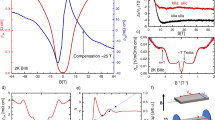
The point at absolute zero where matter becomes unstable to new forms of order is called a quantum critical point (QCP). The quantum fluctuations between order and disorder1,2,3,4,5 that develop at this point induce profound transformations in the finite temperature electronic properties of the material. Magnetic fields are ideal for tuning a material as close as possible to a QCP, where the most intense effects of criticality can be studied. A previous study6 on the heavy-electron material YbRh2Si2 found that near a field-induced QCP electrons move ever more slowly and scatter off one another with ever increasing probability, as indicated by a divergence to infinity of the electron effective mass and scattering cross-section. But these studies could not shed light on whether these properties were an artefact of the applied field7,8, or a more general feature of field-free QCPs. Here we report that, when germanium-doped YbRh2Si2 is tuned away from a chemically induced QCP by magnetic fields, there is a universal behaviour in the temperature dependence of the specific heat and resistivity: the characteristic kinetic energy of electrons is directly proportional to the strength of the applied field. We infer that all ballistic motion of electrons vanishes at a QCP, forming a new class of conductor in which individual electrons decay into collective current-carrying motions of the electron fluid.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
References
Hertz, J. A. Quantum critical phenomena. Phys. Rev. B 14, 1165–1184 (1976)
Millis, A. J. Effect of a nonzero temperature on quantum critical points in itinerant fermion systems. Phys. Rev. B 48, 7183–7196 (1993)
Continentino, M. A. Quantum scaling in many-body systems. Phys. Rep. 239, 179–213 (1994)
Si, Q., Rabello, S., Ingersent, K. & Smith, J. L. Locally critical quantum phase transitions in strongly correlated metals. Nature 413, 804–808 (2001)
Coleman, P. & Pépin, C. What is the fate of the heavy electron at a quantum critical point? Physica B 312, 383–389 (2002)
Gegenwart, P. et al. Magnetic-field induced quantum critical point in YbRh2Si2 . Phys. Rev. Lett. 89, 056402 (2002)
Heuser, K. et al. Inducement of non-Fermi-liquid behavior with a magnetic field. Phys. Rev. B 57, R4198–R4201 (1998)
Stockert, O. et al. Pressure versus magnetic-field tuning of a magnetic quantum phase transition. Physica B 312-313, 458–460 (2002)
Trovarelli, O. et al. YbRh2Si2: Pronounced non-Fermi-liquid effects above a low-lying magnetic phase transition. Phys. Rev. Lett. 85, 626–629 (2000)
Kadowaki, K. & Woods, S. B. Universal relationship of the resistivity and specific heat in heavy-fermion compounds. Solid State Commun. 58, 507–509 (1986)
Gegenwart, P. et al. Divergence of the heavy quasiparticle mass at the antiferromagnetic quantum critical point in YbRh2Si2 . Acta. Phys. Pol. B 34, 323–334 (2003)
Landau, L. D. The theory of a Fermi liquid. Sov. Phys. JETP 3, 920–925 (1957)
Paul, I. & Kotliar, G. Thermoelectric behavior near the magnetic quantum critical point. Phys. Rev. B 64, 184414 (2001)
Giamarchi, T., Varma, C. M., Ruckenstein, A. E. & Nozières, P. Singular low energy properties of an impurity model with finite range interactions. Phys. Rev. Lett. 70, 3967–3970 (1993)
Schröder, A. et al. Onset of antiferromagnetism in heavy-fermion metals. Nature 407, 351–355 (2000)
Mederle, S. et al. Unconventional metallic state in YbRh2(Si1-xGex)2—a high pressure study. J. Phys. Condens. Matter 14, 10731–10736 (2002)
Plessel, J. et al. Unusual behavior of the low-moment mangetic ground-state of YbRh2Si2 under high pressure. Phys. Rev. B 67, 180303 (2003)
Francois, M., Venturini, G., Marchêché, J. F., Malaman, B. & Roques, B. De Nouvelles séries de germaniures, isotopes de U4Re7Si6, ThCr2Si2 et CaBe2Ge2, dans les systèmes ternaires R-T-Ge où R est un élément des terres rares et T ≡ Ru, Os, Rh, Ir: supraconductivité de LaIr2Ge2 . J. Less Common Metals 113, 231–237 (1985)
Carter, G. C., et al. in Metallic shifts in NMR. Progress in Materials Science Vol. 20, Part I, Ch. 9 (eds Chalmers, B., Christian, J. W. & Massalski, T. B.) 123–124 (Oxford, Pergamon, 1977)
Acknowledgements
We acknowledge discussions with J. Ferstl, C. Langhammer, S. Mederle, N. Oeschler, I. Zerec, G. Sparn, O. Stockert, M. Abd-Elmeguid, J. Hopkinson, A. I. Larkin and I. Paul. Work at Dresden is partially supported by the Fonds der Chemischen Industrie and by the FERLIN project of the European Science Foundation. P. C. is supported by the National Science Foundation. Y. T. is a Young Scientist Research Fellow supported by the Japan Society for the Promotion of Science.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Competing interests
The authors declare that they have no competing financial interests.
Rights and permissions
About this article
Cite this article
Custers, J., Gegenwart, P., Wilhelm, H. et al. The break-up of heavy electrons at a quantum critical point. Nature 424, 524–527 (2003). https://doi.org/10.1038/nature01774
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1038/nature01774
This article is cited by
-
The scaled-invariant Planckian metal and quantum criticality in Ce1−xNdxCoIn5
Nature Communications (2023)
-
Critical slowing down near a magnetic quantum phase transition with fermionic breakdown
Nature Physics (2023)
-
Transport evidence for decoupled nematic and magnetic criticality in iron chalcogenides
Communications Physics (2022)
-
Control of electronic topology in a strongly correlated electron system
Nature Communications (2022)
-
Anisotropy-driven quantum criticality in an intermediate valence system
Nature Communications (2022)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.