Proximity to a critical point driven by electronic entropy in URu₂Si₂

Abstract

The strongly correlated actinide metal URu₂Si₂ exhibits a mean field-like second order phase transition at T_o ≈ 17 K, yet lacks definitive signatures of a broken symmetry. Meanwhile, various experiments have also shown the electronic energy gap to closely resemble that resulting from hybridization between conduction electron and 5f-electron states. We argue here, using thermodynamic measurements, that the above seemingly incompatible observations can be jointly understood by way of proximity to an entropy-driven critical point, in which the latent heat of a valence-type electronic instability is quenched by thermal excitations across a gap, driving the transition second order. Salient features of such a transition include a robust gap spanning highly degenerate features in the electronic density of states, that is weakly (if at all) suppressed by temperature on approaching T_o, and an elliptical phase boundary in magnetic field and temperature that is Pauli paramagnetically limited at its critical magnetic field.

Introduction

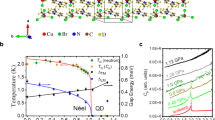
URu₂Si₂ remains of immense interest owing to the possibility of it exhibiting a form of order distinct from that observed in other known materials¹. The term “hidden order” (HO) has been coined^2,3 to describe the finding of an apparent Bardeen–Cooper–Schrieffer (BCS)-like phase transition at T_o ≈ 17 K^4,5, yet the absence of any definitive signatures of symmetry-breaking reconcilable with the entropy at the transition temperature¹. Whereas the majority of the proposed forms of HO^{3,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21,22} involve translational symmetry breaking^{3,8,9,10,11,12,13,14,15,16,17,18,19,20,21,22}, the experimental evidence in favor of this is indirect (see Methods)^{23,24,25,26,27,28}. By contrast, neutron scattering, x-ray scattering, and scanning tunneling microscopy studies of URu₂Si₂ have shown that the usual momentum-space signatures of translational symmetry-breaking are missing within the HO phase^29,30,31. The form of the electronic energy gap within the HO phase is found instead to be consistent with the opening of a hybridization gap between conduction and f-electron states centered on the chemical potential μ, as shown schematically in Fig. 1^{29,30,31,32,33}. This gap has further been reported to evolve into a pseudogap at temperatures above T_o^32,34,35, suggesting departures from the Landau formalism.

Fig. 1: Formation of a hybridization gap.

a Conduction electron band (red) and weakly dispersive f-electron band (green) in the absence of a hybridization gap. b Hybridization bands forming at low temperatures, where V is the hybridization potential. c Indirect gap 2Δ separating peaks in the electronic density of states.

The motivation for the present study is the realization that a HO phase corresponding to the formation (or opening) of a hybridization gap centered on μ would be expected to exhibit thermodynamic signatures distinct from those of a BCS-like phase transition^36,37,38,39. For example, since the process of hybridization causes f electrons to become more delocalized (see Fig. 1)^40,41, such a phase transition would be expected to be accompanied by changes in the 5f-electron core occupancy and valence⁴², thereby displaying some parallels to valence transition systems. Furthermore, since all of the available states in a narrow f-electron band (i.e., two electrons per formula unit—one filled and one empty) typically participate in the formation of hybridization gap^40,41, in contrast to the small fraction of the carriers in a conduction band that participate in the formation of a BCS-like gap^36,37,38,39, significant differences in the entropy of the quasiparticle excitations would be expected in thermodynamic experiments. Finally, since there is no absolute requirement for the magnitude of a hybridization gap to vanish at a phase transition, it need not be thermally suppressed in the same manner as a BCS-type gap.

Experimental observations that could already be considered conducive to 5f-electron hybridization playing an important role in URu₂Si₂ include the intermediate valence character of the 5f electrons identified in x-ray scattering studies^43,44 and the finding of measureable volume and lattice changes across the HO transition in dilatometry experiments^45,46, which become increasingly sharp under a strong magnetic field^47,48.

We show here by way of thermodynamic^49,50 measurements that the HO phase in URu₂Si₂ exhibits signatures consistent with a critical point. In this unprecedented case, a discontinuous electronic phase transition into a state with a robust hybridization gap between the conduction and f electrons centered on μ becomes concealed as a mean field-like second-order phase transition owing to the effect of substantial quasiparticle excitations across the gap. We find the gap excitations, which are responsible for the exponential tail in the low temperature (T < T_o) heat capacity^4,5, to contribute significantly more to the internal energy than they do to the free energy, leading to an unusual situation in which the transition manifests itself second order in nature in all the observables, while maintaining some characteristics of a discontinuous phase transition. These include a robust gap that is weakly (if at all) suppressed by temperature, spanning highly degenerate features in the electronic density of states that are quantitatively consistent with a half-filled narrow f-electron band that has become gapped^29,30 (see Fig. 1), and an elliptical phase boundary that is usually the hallmark of valence transition systems^51,52,53. The effective g-factor characterizing the ratio of the Pauli-limited critical magnetic field B_o to the critical temperature T_o is in quantitative agreement with prior measurements^54,55,56. The near-degeneracy in the free energies of the HO and paramagnetic phase over a range of temperatures T ≥ T_o further causes the hybridization gap in URu₂Si₂ to become highly susceptible to the formation of a pseudogap^34,35, should fluctuations or inhomogeneities be present.

Results

Thermally robust hybridization gap

The salient feature of the HO phase in URu₂Si₂ is a sharp anomaly in the specific heat suggestive of a continuous phase transition (see Fig. 2 and Methods)^{1,2,3,4,5,49,57}. One of the more striking properties of this transition is an exponential tail that extends over a broad range of temperatures and is often compared to the exponential tail observed in BCS-type phases at low temperatures^36,37,38,39. In the BCS theory³⁶, the excitation gap Δ causing the exponential tail has the form $C\propto {{\rm{e}}}^{-\frac{\Delta }{T}}$, where Δ = aT_c, a ≈ 1.5, and T_c is the transition temperature. Were one to set T_c = 17 K, the BCS theory would predict an excitation gap of Δ ≈ 25 K for which the population of excited quasiparticles ($\sim {{\rm{e}}}^{-\frac{\Delta }{T}}$) would reach at least ≈22% of its saturated value as the transition temperature is approached from below. In such a scenario, the excitation of quasiparticles across the gap would cause a suppression of the amplitude of the order parameter with increasing temperature, and its ultimate closure at the phase transition temperature. This closure would, in turn, cause the number of quasiparticles excited across the gap to reach 100%. By contrast, previous reports of a much larger excitation gap of Δ ~ 100 K in URu₂Si₂^4,5 imply that only a small population of excited quasiparticles of order ~0.3% occurs on reaching the transition temperature. This is too small a fraction for Δ to be significantly suppressed by thermal excitations in the manner of a BCS-type phase transition.

Fig. 2: Heat capacity and entropy of URu₂Si₂.

a C/T at B = 0 from ref. ⁵⁷ (blue line), with the phonon (i.e., ThRu₂Si₂ in gray) component and the total electronic contribution (magenta curve) indicated. γ_HO is the residual electronic contribution within the hidden-order phase (see Methods). b Total entropy obtained from ${S}_{{\rm{tot}}}=\mathop{\int}\nolimits_{0}^{\infty }({C}_{{\rm{e}}}/T){\rm{d}}T$, where C_e is the total electronic contribution to the heat capacity.

A further difficulty with the application of the BCS phenomenology to URu₂Si₂ is that its validity requires only a small fraction (n_Δ ≪ 1 electrons per formula unit) of the carriers in the conduction band to be displaced in energy on either side of the chemical potential when the gap opens^36,37,38,39. However, when we estimate the number of electrons displaced by the opening of a 2Δ-wide BCS gap in URu₂Si₂ (using Δ = 100 K), we obtain n_Δ = 2ΔD(ε) = 6Δ × Δγ/π²R ~ 0.5 electrons per formula unit (where R is the gas constant). We base this estimate on the entropy ${S}_{\exp }={{\Delta }}\gamma {T}_{{\rm{o}}}$ associated with the formation of the HO phase having been found experimentally by the integration of ${C}_{\exp }/T$ to be ${S}_{\exp }=$ 0.2 $R\mathrm{ln}\,2$ (see Fig. 3)^4,5, for which the change in Sommerfeld coefficient at T_o is Δγ ≈ 68 mJmol⁻¹K⁻². Since our estimate of n_Δ ~ 0.5 electrons per formula unit corresponds to a significant fraction of a band, it implies that a major reconfiguration of the electronic bands accompanies the formation of the HO phase in URu₂Si₂.

Fig. 3: Electronic entropy and free energy of quasiparticle excitations in URu₂Si₂.

a The entropy divided by $R\mathrm{ln}\,2$ (where R = k_BN_A) at different values of the magnetic field calculated using ${S}_{\exp }=\mathop{\int}\nolimits_{0}^{\infty }\frac{{C}_{\exp }}{T}{\rm{d}}T$ where ${C}_{\exp }$ refers to the measured heat capacity after subtracting the phonon contribution and γ_HO (see Methods). The entropy is similar to that previously obtained^4,5. b Φ_Δ calculated from the experimental heat capacity data of URu₂Si₂ using Eq. (2) as described in the Methods at different magnetic fields as indicated. The horizontal axis is the temperature T divided by the observed transition temperature T_o(B) at each magnetic field.

In the case of a hybridization gap, by contrast, the majority of the gapped states originate from an f-electron band (or level) that is already likely to have been highly degenerate prior to the onset of a hybridization gap, as shown schematically in Fig. 1a. When hybridization occurs at a characteristic energy V between the conduction electron and a coherent lattice of f electrons (see Fig. 1b), the majority of the f-electron states become piled up at energies ±Δ on either side of the gap (see Fig. 1c), where Δ ≪ V. In such a situation, 2Δ usually corresponds to the indirect gap shown schematically in Fig. 1b, c. On assuming the chemical potential to be located at μ = 0, a more appropriate approximation for the gap is an electronic density of states of the form

$${g}_{\Delta }(\varepsilon )=\frac{{n}_{f}}{2}\sum _{i=\pm 1}\delta (\varepsilon -i\Delta )$$

(1)

consisting of delta functions, where each is comprised of n_f/2 ≈ 1 electron per formula unit and is separated from the other by a gap 2Δ. The level below the chemical potential is filled, whereas that above the chemical potential is empty. We assume a Kondo semimetallic ground state in which a hybridization gap coexists with small ungapped sections of Fermi surface; the latter likely originate from a small finite dispersion of the unhybridized f-electrons and are responsible for the residual specific heat (γ_HO in the Methods) and quantum oscillations. Assuming a density of states of the form given by Eq. (1), the quasiparticle excitation term to the free energy of the HO phase is given by

$$\begin{array}{l}{{{\Phi }}}_{\Delta }=-{n}_{f}RT\displaystyle\mathop{\int}\nolimits_{-\infty }^{\infty }{g}_{\Delta }(\varepsilon )\mathrm{ln}\,(1+{{\rm{e}}}^{-\frac{\varepsilon }{T}}){\rm{d}}\varepsilon -{{{\Phi }}}_{0}\\ \quad \,\,\,\,=-{n}_{f}RT\mathrm{ln}\,(1+{{\rm{e}}}^{-\frac{\Delta }{T}}),\end{array}$$

(2)

where Φ₀ is a constant whose value enforces the constraint Φ_Δ → 0 in the limit T → 0. While we assume the gap to be symmetric with regard to μ, the same form for the free energy is obtained for the case of n_f electrons contained in a single delta function located at ε = +Δ or ε = −Δ. On calculating the specific heat, we find the Schottky form

$${C}_{\Delta }=-T\frac{{\partial }^{2}{{{\Phi }}}_{\Delta }}{\partial {T}^{2}}=\frac{{n}_{f}R{\Delta }^{2}}{{T}^{2}}\frac{{{\rm{e}}}^{-\frac{\Delta }{T}}}{{(1+{{\rm{e}}}^{-\frac{\Delta }{T}})}^{2}},$$

(3)

where ${{\rm{e}}}^{-\frac{\Delta }{T}}\ll 1$. We obtain Δ = 84 ± 2 K to reasonable accuracy by performing a straight line fit to an Arrhenius plot of the experimentally measured specific heat ${C}_{\exp }$ multiplied by T² (shown in Fig. 4). The finding of a straight line up to ~14 K suggests that Δ remains largely unchanged up to this temperature, which corresponds to ≈80% of T_o.

Fig. 4: Arrhenius plot.

$\mathrm{ln}\,[{C}_{\exp }{T}^{2}]$ (blue circles) versus 1/T at B = 0, which we fit to $\mathrm{ln}\,[{C}_{\Delta }{T}^{2}]$ (blue line) where C_Δ is given by Eq. (3). We compare this against $\mathrm{ln}\,[{\chi }_{\exp }T]$ (red circles, shifted to bring it into alignment with the specific heat) fit to χ_Δ (red line) given by Eq. (4), where ${\chi }_{\exp }$ is the measured susceptibility (shown in the inset). Solid lines fits are used to provide gap estimates Δ_C and Δ_χ, obtained from the specific heat and susceptibility, respectively.

An identically large hybridization gap governing the quasiparticle excitations is evidenced by the presence of a similar activated behavior in the susceptibility (also shown in Fig. 4). We model the spin susceptibility using

$$\begin{array}{l}{\chi }_{\Delta }={\mu }_{0}{[{g}_{{\rm{eff}}}^{* }\sigma {\mu }_{{\rm{B}}}]}^{2}R{k}_{{\rm{B}}}^{-1}\mathop{\int}\nolimits_{-\infty }^{\infty }{{\rm{f}}}_{{\rm{FD}}}^{\prime}(\varepsilon )g(\varepsilon ){\rm{d}}\varepsilon \\ \quad \,\,\,\,=\frac{{n}_{f}{\mu }_{0}{[{g}_{{\rm{eff}}}^{* }\sigma {\mu }_{{\rm{B}}}]}^{2}R}{{k}_{{\rm{B}}}T}\frac{{{\rm{e}}}^{-\frac{\Delta }{T}}}{{(1+{{\rm{e}}}^{-\frac{\Delta }{T}})}^{2}},\,\end{array}$$

(4)

where ${{\rm{f}}}_{{\rm{FD}}}^{\prime}(\varepsilon )$ is the energy derivative of the Fermi–Dirac distribution function. Figure 4 shows that the experimental ${C}_{\exp }$ and ${\chi }_{\exp }$ data in URu₂Si₂ are characterized by similar values of the excitation gap Δ within experimental uncertainty, indicating that the spin and charge excitations are representative of the same electronic states. The observation of an activated behavior somewhat closer to T_o in the susceptibility reinforces the finding from heat capacity measurements of a large mostly temperature-independent gap. It also represents a marked departure from the case of a BCS-like order parameter, such as a charge-density wave⁵⁸, where the gap magnitude shrinks and rapidly fills in with thermally excited quasiparticles close to the transition.

The values of Δ in Fig. 4 are of comparable magnitude to those typically found in optical conductivity and tunneling experiments^{29,30,32,33,59,60}. We find stronger quantitative consistency with the range of gap values, 63 ≤ Δ ≤ 82 K, attributed to hybridization in point contact spectroscopy experiments (see Fig. 3c, d of refs ^32,33), thereby reinforcing our interpretation that the exponential tail of the heat capacity anomaly in URu₂Si₂^1,2,3,4,5 is caused by quasiparticle excitations across a hybridization gap rather than a BCS-type gap.

Participation of two electrons per formula unit

The Arrhenius fit of Eq. (3) to the specific heat data also provides us with a means to extract an experimental estimate for the absolute number n_f of f-electron states participating in the hybridization gap. From the intercept $\mathrm{ln}\,[{n}_{f}R{\Delta }^{2}]\approx$ 11.9 of the Arrhenius plot in Fig. 4, we obtain n_f ≈ 2.47 ± 0.25 per formula unit, which corresponds to roughly two 5f electrons per formula unit—again, suggesting one filled and one empty. This value is significantly larger than the estimate of n_Δ ≈ 0.4 electrons displaced by the gap that we obtain on substituting Δ = 84 K into n_Δ = 2ΔD(ε) = 6Δ × Δγ/π²R, suggesting that the n_f ≈ 2 electrons participating in the formation of the hybridization gap must involve states that are much farther from the Fermi surface than those typically participating in a BCS-type gap. This can include states that are farther removed from the chemical potential than ±Δ or f electrons that are confined to the atomic core above T_o.

Entropy considerations add further weight to a scenario in which the number of electrons participating in the gap are too numerous to have originated from states close to the Fermi surface. Since the entropy of the gap excitations is given by

$$\begin{array}{lll}{S}_{\Delta }&=&-\frac{\partial {{{\Phi }}}_{\Delta }(B,T)}{\partial T}\\ &=&{n}_{f}R\left[\mathrm{ln}\,(1+{{\rm{e}}}^{-\frac{\Delta }{T}})+\frac{\Delta }{T}\frac{{{\rm{e}}}^{-\frac{\Delta }{T}}}{(1+{{\rm{e}}}^{-\frac{\Delta }{T}})}\right],\end{array}$$

(5)

on substituting n_f ≈ 2 electrons per formula unit participating in the gap we arrive at a total “projected” entropy (on taking the limit Δ → 0) of ${S}_{\Delta }\approx R\mathrm{ln}\,4$. This is ten times larger than the experimentally observed entropy associated with the transition of ${S}_{\exp }\approx 0.2R\mathrm{ln}\,2$ (see Fig. 3 and Methods)^4,5 at T_o, and twice as large as the total electronic entropy ${S}_{{\rm{tot}}}\approx R\mathrm{ln}\,2$ obtained on integrating the full electronic contribution to C/T (magenta curve in Fig. 2a) up to 50 K in Fig. 2b. One way to reconcile the existence of a gapped f-electron band supporting a projected electronic entropy of $R\mathrm{ln}\,4$ (were this to persist beyond T_o) with an actual integrated total electronic entropy of $R\mathrm{ln}\,2$ at T = 50 K ~ T_K is if T_o involves a transition from a screened Kondo lattice state consisting of local spins with a twofold degeneracy at T > T_o into a partially gapped state with fully itinerant f electrons including spin and charge degrees of freedom at T < T_o. A transition of this nature has indeed been suggested on the basis of scanning tunneling microscopy studies^29,30, which find a hybridization gap at T < T_o and single impurity-type behavior at T > T_o³⁰.

Elliptical phase boundary

Further support for a robust hybridization gap and a significant change in the electronic structure accompanying the transition in URu₂Si₂ is to be found in the elliptical form of the phase boundary (see Fig. 5). This can be understood phenomenologically by considering a minimal model for the free energies of the paramagnetic (P) and the HO phases of the form

$$\begin{array}{lll}{F}_{{\rm{P}}}^{\prime}&=&-\frac{1}{2}{{\Delta }}\gamma {T}^{2}-\frac{1}{2{\mu }_{0}}{{\Delta }}{\chi }_{zz}{B}_{z}^{2}\\ {F}_{{\rm{HO}}}^{\prime}&=&-{U}_{{\rm{o}}}+{{{\Phi }}}_{\Delta }(B,T).\end{array}$$

(6)

An important factor in determining our choice of ${F}_{{\rm{P}}}^{\prime}$ in URu₂Si₂ is that the Kondo temperature T_K ~ 70 K^61,62 greatly exceeds T_o, suggesting the development of Kondo screening⁴⁰ well in advance of the transition at T_o. The Sommerfeld coefficient and spin susceptibility are both found to be strongly enhanced at temperatures both above and below T_o^4,5,63,64, while quantum oscillation experiments^54,65,66 find moderately enhanced quasiparticle effective masses deep inside the HO phase.

Fig. 5: Elliptical phase diagram.

a T_o(B_z) phase boundary of URu₂Si₂, taken from the peak in the specific heat capacity at the transition^49,50 and the step in the resistivity at high fields⁷⁴, where B_z refers to the magnetic field applied along the crystalline c-axis. The solid line represents a fit to Eq. (7). We neglect the additional phase transitions at B ≳ 36 T⁷⁴. b Same data plotted in ${T}_{{\rm{o}}}^{2}$-versus-B² coordinates.

While Kondo screening implies that there is a finite V within the paramagnetic phase at T > T_o, there is a lack of thermodynamic evidence for a gap (see Fig. 2 and Methods for alternative possibilities)—there is, however, spectroscopic evidence for a broadened gap possibly attributed to a pseudogap for a narrow range of temperatures above T_o^32,34,35 (see Methods). Given the comparatively slowly varying electronic contribution to C/T with temperature at T > T_o, as a simplifying measure, we consider constant changes Δγ and Δχ_zz in the respective Sommerfeld coefficient and the c-axis spin susceptibility between the paramagnetic and HO phases in Eq. (6), and neglect terms that are common to both phases (such as that due to phonons). Also, since the spin susceptibility exhibits a strong Ising-like anisotropy^{23,54,55,67,68} on both sides of the transition, ${B}_{z}=B\cos \theta$ refers here to the c-axis component of the magnetic field, while U_o represents the internal energy gained by the formation of the hybridization gap; we assume U₀ to be approximately constant in view of the robust Δ (see Methods). One advantage of adopting such a simple form for the free energy is that it can be compared directly against specific heat measurements without needing to assume a priori whether the Ehrenfest classification⁶⁹ of the transition is of first or second order, or the question of whether a symmetry is broken.

Since a phase transition is defined as the point at which ${F}_{{\rm{P}}}^{\prime}={F}_{{\rm{HO}}}^{\prime}$, a value for U_o that remains finite and positive at the transition allows us to obtain an equation for the phase boundary that has the elliptical form

$${\left(\frac{{T}_{{\rm{o}}}(B)}{{T}_{{\rm{o}}}(B = 0)}\right)}^{2}+{\left(\frac{{B}_{z}}{{B}_{o}}\right)}^{2}=1,$$

(7)

where

$$2[{U}_{{\rm{o}}}-{{{\Phi }}}_{\Delta }(B,T)]\,=\,{{\Delta }}\gamma {T}_{{\rm{o}}}^{2}\,\equiv \,{\mu }_{0}^{-1}{{\Delta }}{\chi }_{zz}{B}_{{\rm{o}}}^{2}.$$

(8)

This form is verified by the experimental finding of an elliptical form for the phase boundary for URu₂Si₂ in Fig. 5. A near constant U_o corresponds to the phase boundary having a similar form to that of valence transition systems^51,52,53. Another important factor in the elliptical shape of the phase boundary is the finding that the excitation term Φ_Δ(B, T) in Fig. 3b is a small contribution to the free energy for T ≤ T_o, (see Methods) and rises to nearly the same value at the phase boundary for all values of the applied magnetic field.

Pauli paramagnetic limiting

Our findings that the spin and charge excitations in Fig. 4 are governed by the same gap (further supported by the reduction in carrier number found in Hall effect measurements)⁶¹ and that the phase boundary is primarily determined by a simple balance between quadratic energy terms imply that the excitations behave as though they were conventional quasiparticles. We confirm this by showing that the HO phase boundary is Pauli paramagnetically limited. Turning to the phase boundary given by Eq. (7), we expect changes in the values of the Sommerfeld coefficient ${{\Delta }}\gamma =\frac{{\pi }^{2}}{3}{k}_{{\rm{B}}}^{2}{{\Delta }}D(\varepsilon )$ and the spin susceptibility ${{\Delta }}{\chi }_{zz}={\mu }_{0}{[{g}_{{\rm{eff}}}^{* }\sigma {\mu }_{{\rm{B}}}]}^{2}{{\Delta }}D(\varepsilon )$ to be defined in terms of the same change in the electronic density of states ΔD(ε)⁷⁰. The ratio T_o/B_o,θ of the transition temperature to the strength of the critical magnetic field therefore depends solely on the effective quasiparticle g-factor

$${g}_{{\rm{eff}},\theta }^{* }=\frac{2\pi {k}_{{\rm{B}}}}{\sqrt{3}{\mu }_{{\rm{B}}}}\,\frac{{T}_{{\rm{o}}}}{{B}_{{\rm{o}},\theta }}$$

(9)

(assuming $\sigma =\frac{1}{2}$ pseudospins). Here, ${g}_{{\rm{eff}},\theta }^{* }\approx {g}_{{\rm{eff}}}^{* }\cos \theta$ refers to the angle-dependent effective g-factor (including its renormalization by Landau Fermi liquid parameters) that accounts for Zeeman splitting of the Landau-level states observed in quantum oscillation experiments⁵⁴ and the spin susceptibility of the Fermi liquid state that competes with superconductivity within the HO phase at low temperatures⁵⁵. On inserting the previously measured⁷¹ angle-dependent critical magnetic field B_o,θ of the HO phase into Eq. (9), we obtain excellent quantitative agreement between the angle-dependent g_eff,θ and prior estimates of g_eff,θ from quantum oscillation and Pauli-limited superconducting upper critical field measurements in Fig. 6^54,55. These findings are, again, consistent with U_o and Δ both being largely robust against an increase in temperature within the HO phase.

Fig. 6: Polar g-factor plot.

Angle θ-dependent ${g}_{{\rm{eff}},\theta }^{* }$ (magenta diamonds) obtained from θ-dependent critical magnetic field B_o,θ of the hidden-order phase⁷¹ using Eq. (9). This is compared against prior g-factor estimates from spin zeroes in the quantum oscillation amplitude⁵⁴ (blue filled circles) and the upper critical field B_c2 of the Pauli-limited superconducting state⁵⁵ (open brown circles) within the hidden-order phase. The solid black line represents ${g}_{{\rm{eff}},\theta }^{* }={g}_{{\rm{eff}}}^{* }\cos \theta$, while the dotted line represents an isotropic g = 2.

Our finding that the critical field is determined by Eq. (9), and not the conventional expression^72,73

$${g}_{{\rm{eff}},\theta }^{* }=\frac{\sqrt{2}{k}_{{\rm{B}}}{{\Psi }}}{{\mu }_{{\rm{B}}}{B}_{{\rm{P}},\theta }}$$

(10)

for the Pauli paramagnetic limit defined in terms of an order parameter Ψ, is another strong indication that the HO gap in URu₂Si₂ is caused by hybridization rather than a conventional BCS-like order parameter. While Eq. (10) has been shown to be valid for the superconducting phase of URu₂Si₂⁵⁵, setting Ψ = Δ in Eq. (10) would yield a 90% overestimate for the critical field of the HO phase.

Quenched latent heat at the transition

The experimental observations presented thus far are suggestive of a discontinuous phase transition in URu₂Si₂. The gap is found to retain a large amplitude close to the phase transition (in Fig. 4), with the pre-factor of the specific heat indicating that n_f ≈ 2 electrons participate in its formation. The phase boundary is further found to have the elliptical form (in Fig. 5) in common with first-order valence transitions systems, and a critical field that is Pauli paramagnetically limited (in Fig. 6). Experimentally, however, the transition is found to be of first order only near the upper critical field B₀ of the HO phase^{47,48,49,64,74}.

A key feature of URu₂Si₂ that distinguishes it from established valence transition systems is the presence of quasiparticle excitations across a gap. Whereas these excitations give rise to a large contribution to the specific heat for T < T_o (see Methods)^4,5, their contribution to the free energy is comparatively small—too small, in fact, to cause a significant departure of the phase boundary from an ideal elliptical form in Fig. 5. The entropic energy term S_ΔT is, however, found to be large enough to almost completely quench the latent heat at the transition. This can be demonstrated by comparing the relative free and internal energies of the paramagnetic and HO phases in Fig. 7. Figure 7a, b shows the relative free energies of the paramagnetic and HO phases obtained using Eq. (6), both in the absence (by artificially setting Φ_Δ = 0 in Fig. 7a) and presence (by setting Δ = 84 K in Φ_Δ in Fig. 7b) of quasiparticle excitations across the gap. The parameter U_o has been arbitrarily adjusted so that ${{\Delta }}F={F}_{{\rm{HO}}}^{\prime}-{F}_{{\rm{P}}}^{\prime}=0$ at the known transition temperature.

Fig. 7: Free and internal energies.

a Free energies according to Eq. (6) in the absence of thermal excitations (i.e., Φ_Δ = 0) at B = 0 for the paramagnetic (P) and hidden-order (HO) phases, computed using Δγ = 68 mJmol⁻¹K⁻² (parameter similar to that found in URu₂Si₂ at B = 0)⁵ and U_o ≈ 10 Jmol⁻¹. At the transition T_o, ${F}_{{\rm{P}}}^{\prime}={F}_{{\rm{HO}}}^{\prime}$. b Free energies according to Eq. (6) inclusive of thermal excitations, computed using Δγ = 68 mJmol⁻¹K⁻², Δ = 84 K, and U_o ≈ 8 Jmol⁻¹. c Internal energies according to Eq. (11) in the absence of thermal excitations (i.e., Φ_Δ = 0) at B = 0. d Internal energies according to Eq. (11) inclusive of thermal excitations. The latent heat at the transition is given by ΔU.

We proceed to extract the relative internal energies of the two phases in the absence (again, by artificially setting Φ_Δ = 0 in Fig. 7c) and presence (again, by setting Δ = 84 K in Φ_Δ in Fig. 7d) of gap excitations. The relative internal energies are therefore given by

$$\begin{array}{lll}{U}_{{\rm{P}}}^{\prime}&=&\frac{1}{2}{{\Delta }}\gamma {T}^{2}-\frac{1}{2{\mu }_{0}}{{\Delta }}\chi {B}^{2}\\ {U}_{{\rm{HO}}}^{\prime}&=&-{U}_{{\rm{o}}}+{{{\Phi }}}_{\Delta }(B,T)+T{S}_{\Delta },\end{array}$$

(11)

where S_Δ is the entropy associated with gap excitations given by Eq. (5). Figure 7 shows that whereas the absence of gap excitations ordinarily leads to a large latent heat ΔU at the transition (see Fig. 7c), analogous to that encountered in valence transition systems^51,52,53, the presence of quasiparticle excitations in Fig. 7d causes ΔU to be quenched in the vicinity of the transition; we find that ΔU vanishes within ~3 K of the transition temperature in URu₂Si₂.

Change in the Ehrenfest classification of the transition

Depending on the degree of suppression of the latent heat, the Ehrenfest classification⁶⁹ of the transition therefore has the potential either to be of first or second order in URu₂Si₂. The order of the transition is ultimately determined by the form of the departure of the measured specific heat ${C}_{\exp }$ (blue circles in Fig. 4) near the transition temperature from the ideal Arrhenius form C_Δ (blue line in Fig. 4) given by Eq. (3). This places quantitative constraints on the manner in which the hybridization gap and phase transition evolve as a function of temperature. In Fig. 8, we extend the fits of C_Δ (black curves) to the measured specific heat ${C}_{\exp }$ (red, green, and blue curves) to different magnetic field values, showing the departure of C_Δ from ${C}_{\exp }$ to become larger with increasing magnetic field.

Fig. 8: Fits to the electronic specific heat.

a The measured electronic specific heat ${C}_{\exp }(T)$ of URu₂Si₂ (data points from ref. ⁴⁹, after having subtracted γ_HO; see Methods) at different externally applied values of the magnetic field, as indicated. Alternating blue, red, and olive lines (color scheme maintained in subsequent panels) represent interpolations of ${C}_{\exp }(T)$ data points (black symbols), while black lines indicate fits of C_Δ given by Eq. (3) to ${C}_{\exp }(T)$ made up to a temperature ${T}_{\max }$, beyond which an exponential form no longer fits the data. To show the degree of departure, the fitted lines are extrapolated beyond ${T}_{\max }$. b A comparison of Δ values (pink squares) obtained from fits of C_Δ to ${C}_{\exp }(T)$ at different magnetic fields with Δ values (blue circles) obtained by setting ΔU = 0 in Eq. (13). The black dashed line represents $\Delta (B)=\Delta (B=0)-[{g}_{{\rm{eff}}}^{* }\sigma ]{\mu }_{{\rm{B}}}B$, which is the expected behavior should Δ be reduced in a magnetic field by Zeeman splitting with ${g}_{{\rm{eff}}}^{* }=$ 2.6 and Δ(B = 0) = 84 K. The inset shows n_f values (open pink squares) obtained from fits of C_Δ to ${C}_{\exp }(T)$ in (a). All error bars refer to the standard error of the mean.

One possibility is that Δ = 84 K (at B = 0) remains constant up to the transition temperature, giving rise to a weakly first-order phase transition (see Fig. 7b) in which the latent heat is similarly small to that depicted in Fig. 7d. In this case, the latent heat must approximately equate to the difference in heat capacity via

$${{\Delta }}U(T)\approx \int ({C}_{\exp }-{C}_{\Delta }){\rm{d}}T$$

(12)

in Fig. 4. On considering this as a possibility, we estimate ΔU ≈ 4 Jmol⁻¹. This value is somewhat smaller than the value ΔU ≈ 8 Jmol⁻¹ (shown as a pink square in Fig. 9) obtained from

$${{\Delta }}U(T={T}_{{\rm{o}}})={T}_{{\rm{o}}}\left({S}_{\exp }-{S}_{\Delta }\right),$$

(13)

in which we equate the experimental entropy ${S}_{\exp }\approx 0.2R\mathrm{ln}\,2$ associated with excitations at the transition^4,5 (see Methods) with that S_Δ obtained on inserting the fitted values of n_f and Δ = 84 K into Eq. (5). The difference between these estimates suggests that the ~3 K range in temperature over which ${C}_{\exp }$ deviates from C_Δ is too large to be solely attributable to the latent heat of a first-order phase transition. Figure 10a (details provided in the Methods) shows that the difference between ΔU(T) given by Eq. (12) and ΔU(T = T_o) and given by Eq. (13) occurs for all values of the applied magnetic field.

Fig. 9: Vanishing latent heat.

a Change in latent heat ΔU of the phase transition between the paramagnetic and hidden-order phases as a function of Δ according to Eq. (13), determined by setting F_HO = F_P. For large Δ, a finite latent heat causes the transition to be of first order (brown solid line). This is the case for the value of Δ = 84 K (solid pink square) obtained from an Arrhenius plot made for the regime T ≪ T_o in Fig. 4. However, at a smaller value of Δ ≈ 73 K, ΔU vanishes causing the transition to become of second order (filled blue circle). At smaller values of Δ, the free energies can no longer be made to intersect at T = T_o (dotted brown line). b A comparison of the temperature-dependent Δ(T) (brown line) estimated for B = 0 using Eq. (14) and the value of Δ (blue circle) consistent with a second-order end point, identified by setting ΔU = 0 in Eq. (13). Yellow shading indicates where the hidden-order phase exists. Cyan shading illustrates schematically the possible region where phase coexistence due to inhomogeneities of fluctuations is observed.

An alternative possibility is that Δ(T) undergoes a small reduction on the approach to the transition, leading to an increase in the entropy (and number) of the quasiparticles excited across the gap, and to a further reduction in the value of ΔU given by Eq. (13). On considering such a possibility, Figure 9a shows that there is a narrow range of Δ values for which ΔU is finite, giving rise to a weakly first-order phase transition. Remarkably, however, we find that there exists a value Δ ≈ 73 K for which ΔU vanishes (blue circle in Fig. 9a), causing the Ehrenfest classification of the transition to change from first to second order⁶⁹. This value of Δ therefore corresponds to a second-order critical point at the end of a line of first-order phase transitions.

We can verify whether the deviation of the experimental ${C}_{\exp }(T)$ from C_Δ is consistent with a small reduction in Δ(T) by comparing the result (brown line in Fig. 9b) of the integral

$$\Delta (T)\approx {\Delta }_{{\rm{C}}}-{\int}_{0}\frac{({C}_{\exp }-{C}_{{\Delta }_{{\rm{C}}}})}{{n}_{f}R}\left(\frac{T}{{\Delta }_{{\rm{C}}}}\right){{\rm{e}}}^{\frac{{\Delta }_{{\rm{C}}}}{T}}{\rm{d}}T$$

(14)

(derived in the Methods, under the assumption that the deviation of ${C}_{\exp }$ from the fitted C_Δ is caused solely by a small reduction in Δ) with the value Δ = 73 K at T_o(B = 0) (blue circle in Fig. 9b) estimated by setting ΔU = 0 in Eq. (13). Consistency between these two estimates at T_o strongly suggests that the HO transition in URu₂Si₂ lies close to a critical point. We further find that Δ(T) estimated using Eq. (14) exhibits a similar temperature-dependent change to that obtained in point contact spectroscopy measurements up to T_o^32,33.

On repeating the above analysis at different values of the applied magnetic field (see Fig. 10b and Methods) in Fig. 8a, we always find the same ≈10 K small difference between the values (pink squares in Fig. 8b) of Δ obtained from fits of C_Δ to the heat capacity tail (see Fig. 8a) and the values (blue circles in Fig. 8b) compatible with a second-order critical point in Eq. (13). Since the blue circles collapse to zero before the pink squares on approaching the critical field B₀ in Fig. 8b, we can now understand why the transition becomes of first order at B₀.

Fig. 10: Comparison of ΔU and Δ estimates.

a A comparison of the estimated change in internal energy ΔU(T) (lines) integrated using Eq. (12), should the transition be of weakly first order, with ΔU(T = T_o) (open squares) at the transition estimated using Eq. (13). b A comparison Δ(T) (lines) estimated using Eq. (14), should the upturn in ${C}_{\exp }$ be caused by a temperature-dependent gap, with Δ at the transition (open circles) obtained by setting ΔU = 0 in Eq. (13). All error bars refer to the standard error of the mean.

We have therefore identified an intriguing situation where a critical point can be realized in which a discontinuity occurs in Δ that coincides with a vanishing (or nearly vanishing) change in the latent heat, giving rise to an unconventional second-order phase transition. The condition for observing a second-order transition may be further relaxed if the separation in temperature between the points at which ΔU and ΔF vanish is less than the width of the phase transition, or in the presence of inhomogeneities or fluctuations(see Methods)³⁵. Our finding of a critical point has the potential to shed light on prior reports of a pseudogap in URu₂Si₂³⁴, which has further been argued³⁵ to account for the continued observation of a gap in point contact spectroscopy measurements over a range of temperatures above T_o^32,33. The small difference we find in internal energy between the HO and paramagnetic phase at T > T₀ further implies that the amplitude of fluctuations of Δ needed for producing a pseudogap is significantly smaller than that required for a BCS-like gap³⁵ (see Methods).

Discussion

We return to the crucial question in URu₂Si₂ of whether a symmetry within the HO phase is broken and whether this is responsible for the observed second-order phase transition. Several observations indicate the second-order phase transition into the HO phase of URu₂Si₂^4,5 to have an unconventional origin. The ratio of the gap magnitude is several times larger than that in the BCS theory^36,37,38,39, while the magnitude of the gap is weakly (if at all) suppressed with increasing temperature. Moreover, the pre-factor of the quasiparticle excitations in the specific heat indicates highly degenerate features in the electronic density of states comprising two electrons per formula unit, which are too large to have arisen from Fermi surface nesting (see Methods)^{23,25,26,27,28}. The large number of states involved suggests instead that the HO phase primarily concerns an electronically driven phase transition in which a hybridization gap between the conduction and f-electron states develops, as has also been inferred from tunneling and neutron scattering experiments^{29,30,31,32,33}. Such a scenario differs from that where the amplitude of a broken symmetry order parameter varies continuously at the transition temperature^36,37,38,39.

Evidence for an electronic phase transition that modifies the core occupancy of the 5f-electron states is provided by the phase boundary having an elliptical form, closely resembling that found in valence transition systems^51,52,53, and by significant anomalies in the lattice^45,46,47,48. Pauli paramagnetic limiting of this phase further implies that the electronic excitations retain their spin and charge quantum numbers throughout the HO phase and across the transition, both in weak and strong magnetic fields. Similarities in the magnitude and anisotropy of the quasiparticle g-factor to that previously observed suggest that these are the same quasiparticles that are responsible for the observed quantum oscillations^54,56 and that undergo pairing to produce a superconducting state at low temperatures^55,56,68.

While the near temperature-independence of the electronic structure prior to a discontinuous phase transition with increasing T and the elliptical form of the phase boundary are characteristic features of a first-order transition of the type seen in valence transition systems^51,52,53, a first-order phase transition is found to exist only at the high magnetic field extremity of the phase boundary⁷⁴, where the transition temperature goes to zero. The single most significant factor that distinguishes URu₂Si₂ from conventional valence transition systems is a semimetallic low temperature phase hosting a large gap in the electronic density of states. At finite temperatures, the robustness of the hybridization gap with increasing temperature is shown to lead to a situation in which the entropy of the quasiparticle excitations increases exponentially with increasing temperature—the experimental signature of which is an exponential tail in the specific heat that spans a wide range in temperature and continues up to the phase transition temperature^4,5. We show that, by suppressing the latent heat at the transition, the entropy of these excitations has the effect of driving the otherwise discontinuous transition toward a critical point. The combined results and arguments here point to an almost perfect concealment of a first-order transition—turning it continuous in all experimental observables, a condition that could change the way we understand a broad variety of condensed matter systems where order parameters have yet to be identified or where reported broken symmetries cannot easily be reconciled with the magnitude of the excitation gap¹.

Methods

Possible indirect evidence for broken translational symmetry

While there may also be a separate gap related to a broken translational symmetry order parameter, this gap does not appear to contribute significantly to the point contact spectroscopy, nor to the excitations in the heat capacity or the spin susceptibility, and therefore appears unlikely to be the main driver of the phase transition at T_o. URu₂Si₂ is known to become antiferromagnetic with a doubled unit cell under pressure^1,23, giving rise to a possible situation analogous to those in UPd₂Al₃⁷⁵ and U(Pt_1−xPd_x)₃⁷⁶ in which hybridized bands (and possibly a hybridization gap) coexist with large moment antiferromagnetism. In URu₂Si₂, photoemission and quantum oscillation studies have reported evidence for Fermi surface nesting also to exist within the ambient pressure HO phase^{23,25,26,27,28}. The proposed nesting within the HO phase, however, pertains to small sections of Fermi surface for which the number of gapped states²⁴ is one or two orders of magnitude smaller than the gapped states we identify to be responsible for the quasiparticle excitations in specific heat measurements. An alternative possibility is that the small Fermi surface pockets are the pre-existing product of a Kondo semimetallic ground state, and that the antiferromagnetism observed under pressure is secondary. The Fermi surface pockets in URu₂Si₂ are sufficiently small and different in size and small in number, that there is a reasonably large probability for them not to intersect each other upon halving the Brillouin zone, in which case they would be expected to remain largely unchanged on entering the antiferromagnetic phase. Interpretations of quantum oscillations are also constrained by the fact that whereas ambient pressure quantum oscillation experiments can be used to infer the shapes of the Fermi surface pockets, this has not been done under pressure²³.

Specific heat background subtraction

Following the procedure outlined in previously published specific heat measurements^49,57, the phonon contribution is obtained from measurements of ThRu₂Si₂ (see Fig. 2). After subtracting the phonon component, the remaining heat capacity is assumed to be electronic in origin. At B = 0, the exponential contribution to the heat capacity is observed to extend down to ≈6 K, below which the specific heat is observed to have a residual contribution C(T) ≈ γ_HOT.

In order to extract the form of ${C}_{\exp }$ attributable to excitations within the HO phase, γ_HOT needs to be subtracted. This was initially done by subtracting a constant γ_HO from the measured C/T⁵. More recent heat capacity studies have shown that γ_HO increases slowly with decreasing temperature. Moriya and Takimoto⁷⁷ and van Dijk et al.⁵⁷ attributed this behavior to spin fluctuations, originating from the close proximity of URu₂Si₂ to an antiferromagnetic ground state. Throughout the manuscript, we have assumed γ_HO(T) to have the form determined by van Dijk et al. (see Fig. 2).

Alternatives to a hybridization gap at T > T _o

Given the finding of a Fano line shape at T > T_o in scanning tunneling microscopy measurements on URu₂Si₂^29,30, which is generally considered to be consistent with a lattice of locally screened Kondo impurities, we might expect the electronic contribution to C/T to have the form of a Schotte–Schotte anomaly⁷⁸. A Zeeman-split Schotte–Schotte anomaly has been shown to provide a good approximation to the evolution of C/T over a wide range of magnetic fields in the sister heavy fermion compound CeRu₂Si₂ and at B > 40 T in URu₂Si₂⁷⁹. The various phase transitions in URu₂Si₂ prevent us from verifying whether a Schotte–Schotte anomaly describes the paramagnetic phase for B < 40 T and T > T_o, however. We therefore make the simplifying assumption that Δγ and Δχ_zz are constants in Eq. (6)—an approximation that appears to be valid up to ≈25 K in Fig. 2a.

Free energy contribution Φ_Δ from gap excitations

While the Φ_Δ term gives a prominent contribution to the specific heat ${C}_{\bigtriangleup }=-T\frac{{\partial }^{2}{{{\Phi }}}_{\bigtriangleup }}{\partial {T}^{2}}$ within the HO phase, its overall contribution to the free energy is smaller by a factor (Δ/T)², making its contribution only a small fraction of U_o. Nevertheless, the question remains as to whether Φ_Δ causes a discernible departure of the phase boundary from the elliptical form. To test this, in Fig. 3b we plot Φ_Δ (using the fitted parameters n_f and Δ in Fig. 8) as a function of the reduced temperature T/T_o(B), finding it to reach a similar value Φ ≈ 1.5 ± 0.1 Jmol⁻¹ at the phase boundary at nearly all values of the magnetic field. The largest deviation occurs for the highest magnetic field datapoint at 32 T, but the fact that no corresponding deviation is observed in Fig. 5 suggests that the deviation in Fig. 3b points to the source of the error originating from the fitting of C_Δ over a short range of T at the highest magnetic field. The differences in the values of Φ_Δ at the transition appear to be too small to cause a significant departure of the phase diagram from the elliptical form.

Departures of C _Δ from C _exp approaching T _o

While the accuracy of fitting C_Δ in Fig. 8 is likely to be reduced in strong magnetic fields due to a reduction in the temperature range, the degree of departure of the experimental specific heat ${C}_{\exp }$ from a simple exponential form becomes significantly more pronounced. We consider two possible sources of the departure of ${C}_{\exp }$ from C_Δ. Latent heat change at a weakly first order transition: in the case of a first-order phase transition, a fit of C_Δ given by Eq. (3) to the specific heat data in Figs. 2 and 8a is expected to apply everywhere, except very close to the transition where the latent heat ΔU causes an upturn in the heat capacity. There, we expect an integration of the heat capacity minus the fit to yield a change in internal energy (ΔU(T) in Eq. (12)) that is the same as the difference in internal energy between the phases given by Eq. (13). We verify this not to be the case in Fig. 10a.

Change in Δ near a second-order phase transition: the alternative possibility is that ΔU is tuned to zero near the transition, causing the Ehrenfest classification of the transition to become of second order. One way this can happen is if Δ(T) falls with increasing temperature as is, for example, reported to occur in point contact spectroscopy measurements³². Since the strength of hybridization is generally weakened as the lattice spacing a between f-electron sites increases, the increase in the lattice parameter a accompanying the transition into the high temperature paramagnetic phase could be one possible factor in causing the transition to become of second order. In this case, we would expect the reduction in Δ(T) with increasing T to be responsible for the upturn in the specific heat close to the transition temperature.

To estimate the effect of a temperature-dependent gap Δ(T) on C, we reduce Eq. (2) to a more approximate form

$${{{\Phi }}}_{0}\approx -{n}_{f}RT{{\rm{e}}}^{-\frac{\Delta (T)}{T}}$$

(given that ${{\rm{e}}}^{-\frac{\Delta (T)}{T}}\ll 1$), from which we obtain

$$\begin{array}{lll}C&\approx &{n}_{f}R{{\rm{e}}}^{-\frac{\Delta }{T}}\left(1+\left(\frac{{\Delta }^{2}}{{T}^{2}}+\frac{\partial \Delta }{\partial T}\left(1-\frac{\Delta }{T}\right)\right.\right.\\ &&\left.-T\frac{{\partial }^{2}\Delta }{\partial {T}^{2}}+{\left(\frac{\partial \Delta }{\partial T}\right)}^{2}\right)\end{array}$$

(15)

after double differentiation. Assuming the limits Δ/T ≫ 1 and Δ/T ≳ ∂Δ/∂T, we obtain the approximate result

$$C\approx {C}_{\Delta }-{n}_{f}RT{{\rm{e}}}^{-\frac{\Delta }{T}}\left(\frac{\Delta }{T}\frac{\partial \Delta }{\partial T}\right),$$

(16)

which we rearrange to yield Eq. (14), from which Δ(T) can be estimated numerically (lines in Fig. 10b).

Meanwhile, the smallest permissible equilibrium value of Δ is that corresponding to an end point in Fig. 9a. We determine this value by setting ΔU = 0 in Eq. (13)—yielding an equation

$${S}_{\Delta }(\Delta ,{T}_{{\rm{o}}})={S}_{\exp }$$

(17)

that can be solved for Δ(T_o(B)), the results of which are plotted in Fig. 10b (circles). Here, T_o(B) refers to the value of the transition temperature in a magnetic field. Consistency between the value of Δ(T) at T_o(B) (i.e., the highest temperature limit of the lines in Fig. 10b) estimated using Eqs. (16) and (14) and that obtained independently for a second-order end point by solving Eq. (17) suggests that Δ(T) retains a near constant value until close to the transition, but then ultimately falls by ≈10 K to produce a second-order end point. For smaller values of Δ, the free energy curves (e.g., that in Fig. 7b) given by Eq. (6) cannot be made to intersect at T = T_o(B). Only fluctuations of Δ are therefore possible once T > T_o(B), and their existence is strongly suggested by the near-degeneracy in the free energy curves in this regime. This is also the same regime in which a pseudogap or crossover is observed in spectroscopic experiments^32,33,34,59.

Changes in internal energy

In the case of a BCS order parameter, we would expect ${U}_{{\rm{o}}}=\frac{1}{2}D(\varepsilon ){({k}_{{\rm{B}}}\Delta )}^{2}\approx \frac{3}{2{\pi }^{2}}{{\Delta }}\gamma {\Delta }^{2}$ (noting that Δ is expressed in degrees kelvin), from which we estimate U_o ≈ 73 Jmol⁻¹. The fact that this is much larger than the actual value of U_o ≈ 8 Jmol⁻¹ in Fig. 7b again reinforces our prior finding that the observed gap does not originate solely from states at the Fermi surface. An alternative scenario in the case of a hybridization transition is that U_o is the consequence of a difference in energy between competing electronic configurations (possibly with different numbers of 5f electrons confined to the atomic core), and Δ = 84 K is determined independently by V. If Δ does at some point change, then one would expect the dependence of U_o on Δ to be parabolic to lowest order, so that ${U}_{{\rm{o}}}={U}_{{\rm{o}},0}-{U}^{\prime}{({{\Delta }}-{{{\Delta }}}_{0})}^{2}$, where ${U}^{\prime}\ll \frac{3}{2{\pi }^{2}}{{\Delta }}\gamma$ and U_o,0 is the low temperature value valid for Δ = Δ₀; here, Δ₀ refers specifically to the value of Δ determined from lower temperature fits in Fig. 4.

Fluctuations and pseudogap formation

Owing to the likely discontinuous nature of the change in Δ, an interesting situation arises in which fluctuations can cause phase coexistence to occur at a second-order phase transition. One possible source of fluctuations or inhomogeneities is the proximity to the antiferromagnetically ordered phase under pressure³⁵, which has already been shown to give rise to a tiny volume fraction of antiferromagnetic order within the HO phase¹. Indeed, one of the more unusual discoveries in recent spectroscopy experiments^32,33,34,59 in URu₂Si₂ is the emergence of what appears to be a coexistence region, pseudogap, or crossover at temperatures T > T_o, which also has the unusual property (depending on the experimental technique) of the gap maintaining a finite amplitude on passing through T = T_o, despite the phase transition. The discontinuous change in Δ that we have identified implies that fluctuations can give rise to regions with a full gap amplitude (73 ≤ Δ ≤ 84 K) coexisting with regions with an absent hybridization gap in the paramagnetic state (see Methods). Such a scenario is made likely by the very small difference in free energy between the fully gapped HO state and the paramagnetic state (see Fig. 7b) over a range of temperatures T > T_o. The normal state maximum at ~22 K in Fig. 2a is indeed consistent with a heavily broadened gap of ~73 K.

By considering a BCS-like order parameter, Haraldsen et al.³⁵ were able to simulate a pseudogap, but with the caveat that the amplitude σ_Δ of the order parameter fluctuations was required to be ≈60% of Δ, bringing into question the viability of a sharp phase transition in URu₂Si₂. In the present work, the extreme sensitivity of the transition to small changes in Δ means that a pseudogap over a substantial region of T can be realized with fluctuations that are only a small fraction of Δ. Gap fluctuations of amplitude σ_Δ produce a statistical broadening of the HO free energy of size

$${\sigma }_{{{\Delta }}F}\approx {\sigma }_{\Delta }\times \left|\frac{\partial {{{\Phi }}}_{\Delta }}{\partial \Delta }\right|\approx {n}_{f}R{\sigma }_{\Delta }\frac{{{\rm{e}}}^{-\frac{\Delta }{T}}}{1+{{\rm{e}}}^{-\frac{\Delta }{T}}}.$$

(18)

We find that σ_Δ/Δ = 5% is all that is required for σ_ΔF to become comparable to the ≈0.5 Jmol⁻¹ difference in free energy between the curves at T ~ 20 K in Fig. 7b.

Given the lattice changes at the transition, fluctuations would further be expected to affect the lattice dynamics of URu₂Si₂. Thermal expansion measurements have previously shown that the HO and paramagnetic phases have different values of the c/a ratio (the ratio of the c-axis to the a-axis lattice parameters)^45,46,48, implying that this ratio is coupled to the strength of the hybridization and Δ. Fluctuations of Δ within the pseudogap region are therefore likely to lead to a considerable softening of the crystalline lattice with respect to the A_1g mode. A lattice softening of this nature has recently been reported in resonant ultrasound spectroscopy experiments⁸⁰, although the existing interpretation has assumed strictly localized 5f electrons—in effect, neglecting the extreme sensitivity of the f-electron core occupancy and hybridization strength to volume changes and lattice distortions. Because it preserves the fourfold symmetry of the lattice, the A_1g mode is the only one (other than a change in volume) that couples to the degree of delocalization of the 5f electrons (and thereby the strength of Δ) uniformly across all sites.