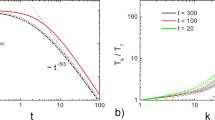
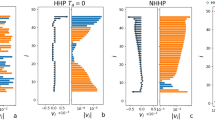
Abstract
Edwards has proposed1,2,3 a thermodynamic description of dense, slowly flowing granular matter, in which the grains (the ‘atoms’ of the system) interact with inelastic forces and enduring contacts. In Edwards' ensemble—one of the very few generalizations of standard statistical mechanics—thermodynamic quantities are computed as flat averages over configurations in which the grains are static or jammed, leading to a natural definition of configurational temperature. But the approach is not justified from first principles and hence, in the absence of explicit tests of its validity, has not been widely accepted. Here we report a numerical experiment involving a realistic model of slowly sheared granular matter; our results strongly support the thermodynamic description. Considering particles of different sizes in a slowly sheared dense granular system, we extract an effective temperature from a relation connecting their diffusivity and mobility. We then perform an explicit computation to show that the effective temperature measured from this relation coincides with the Edwards configurational temperature. Our approach, which is specifically conceived to be reproducible in the laboratory, may thus render the Edwards temperature accessible to experiments.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
References
Edwards, S. F. in Granular Matter: An Interdisciplinary Approach (ed. Mehta, A.) 121–140 (Springer, New York, 1994).
Edwards, S. F. in Disorder in Condensed Matter Physics (eds Blackman, J. & Taguena, J.) 147–154 (Oxford Univ. Press, Oxford, 1991).
Mehta, A. & Edwards, S. F. Statistical mechanics of powder mixtures. Physica A 157, 1091–1097 (1989).
Cugliandolo, L. F., Kurchan, J. & Peliti, L. Energy flow, partial equilibration, and effective temperatures in systems with slow dynamics. Phys. Rev. E 55, 3898–3914 (1997).
Kurchan, J. in Jamming and Rheology: Constrained Dynamics on Microscopic and Macroscopic Scales (eds Liu, A. & Nagel, S. R.) 72–79 (Taylor and Francis, London, 2001).
Nicodemi, M. Dynamical response functions in models of vibrated granular media. Phys. Rev. Lett. 82, 3734–3737 (1999).
Barrat, A., Kurchan, J., Loreto, V. & Sellitto, M. Edwards measures for powders and glasses. Phys. Rev. Lett. 85, 5034–5037 (2000).
Javier Brey, J., Prados, A. & Sanchez-Rey, B. Thermodynamic description in a simple model for granular compaction. Physica A 275, 310–324 (2000).
Lefevre, A. & Dean, D. S. Tapping spin-glasses and ferromagnets on random graphs. Phys. Rev. Lett. 86, 5639–5642 (2001).
Sciortino, F. & Tartaglia, P. Extension of the fluctuation-dissipation theorem to the physical aging of a model glass-forming liquid. Phys. Rev. Lett. 86, 107–110 (2001).
Nowak, E. R., Knight, J. B., Ben-Naim, E., Jaeger, H. M. & Nagel, S. R. Density fluctuations in vibrated granular materials. Phys. Rev. E 57, 1971–1974 (1998).
Johnson, K. L. Contact Mechanics (Cambridge Univ. Press, Cambridge, 1985).
Cundall, P. A. & Strack, O. D. L. A Discrete numerical model for granular assemblies. Géotechnique 29, 47–65 (1979).
Schäfer, J., Dippel, S. & Wolf, D. E. Force schemes in simulations of granular materials. J. Phys. I (France) 6, 5–20 (1996).
Makse, H. A., Johnson, D. L. & Schwartz, L. M. Packing of compressible granular materials. Phys. Rev. Lett. 84, 4160–4163 (2000).
Allen, M. P. & Tildesley, D. J. Computer Simulations of Liquids (Clarendon, Oxford, 1987).
Savage, S. B. The mechanics of rapid granular flows. Adv. Appl. Mech. 24, 289–365 (1994).
Durian, D. J. Foam mechanics at the bubble scale. Phys. Rev. Lett. 75, 4780–4783 (1995).
Lacasse, M.-D., Grest, G. S., Levine, D., Mason, T. G. & Weitz, D. A. Model for the elasticity of compressed emulsions. Phys. Rev. Lett. 76, 3448–3451 (1996).
Langer, S. A. & Liu, A. J. Sheared foam as a supercooled liquid? Europhys. Lett. 49, 68–73 (2000).
Herrmann, H. J., Hovi, J. P. & Luding, S. (eds) Physics of Dry Granular Matter (Kluwer, Dordrecht, 1998).
Ottino, J. M. & Khakhar, D. V. Mixing and segregation of granular materials. Annu. Rev. Fluid Mech. 32, 55–91 (2000).
Hoover, W. G. et al. Lennard-Jones triple-point bulk and shear viscosities. Green-Kubo theory, Hamiltonian mechanics, and non-equilibrium molecular dynamics. Phys. Rev. A 22, 1690–1697 (1980).
Drescher, A. & de Josselin de Jong, G. Photoelastic verification of a numerical model for the flow of a granular material. J. Mech. Phys. Solids 20, 337–351 (1972).
Liu, C.-H. et al. Force fluctuations in bead packs. Science 269, 513–515 (1995).
Jaeger, H. M., Nagel, S. R. & Behringer, R. P. Granular solids, liquids, and gases. Rev. Mod. Phys. 68, 1259–1273 (1996).
Acknowledgements
This work was partially supported by The Petroleum Research Fund.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Makse, H., Kurchan, J. Testing the thermodynamic approach to granular matter with a numerical model of a decisive experiment. Nature 415, 614–617 (2002). https://doi.org/10.1038/415614a
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1038/415614a
This article is cited by
-
Origin of the critical state in sheared granular materials
Nature Physics (2024)
-
Transport phenomena in active turbulence
Pramana (2022)
-
Fluctuating hydrodynamics of chiral active fluids
Nature Physics (2021)
-
Fluctuations and like-torque clusters at the onset of the discontinuous shear thickening transition in granular materials
Communications Physics (2021)
-
Rotational statistics in granular flows of smooth cylindrical particles
Granular Matter (2020)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.