Abstract
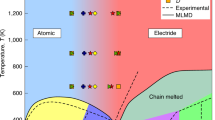
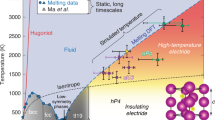
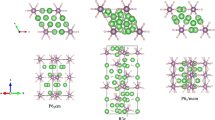
The light alkali metals have played an important role in the developing understanding of the electronic structure of simple metals. These systems are commonly viewed in terms of an underlying interacting electron gas permeated by periodic arrays of ions normally occupying only a small fraction of the crystal volume. The electron–ion interaction, or equivalently the pseudopotential, has been argued to be weak in such systems, reflecting the partial cancellation of nuclear attraction by the largely repulsive effects of Pauli exclusion by the core electrons. Current experiments can now achieve densities at which the core electrons substantially overlap, significantly reducing the fractional volume available to fixed numbers of valence electrons. Here we report the results of first-principles calculations1,2 indicating that lithium, the band structure of which is largely free-electron-like at ordinary densities, does not follow the intuitive expectations of quantum mechanics by becoming even more free-electron-like at higher densities. Instead, at high pressure its electronic structure departs radically from nearly free-electron behaviour, and its common symmetric structure (body-centred cubic, b.c.c.) becomes unstable to a pairing of the ions. Once paired, lithium possesses an even number of electrons per primitive cell which, although not sufficient, is at least necessary for insulating (or semiconducting) behaviour within one-electron band theory.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout





Similar content being viewed by others
References
Kresse, G. & Hafner, J. Ab initio molecular dynamics for liquid metals. Phys. Rev. B 47, RC558–RC561 (1993).
Kresse, G. & Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 54, 11169–11186 (1996).
Hohenberg, P. & Kohn, W. Inhomogeneous electron gas. Phys. Rev. 136, B864–B871 (1964).
Kohn, W. & Sham, L. J. Self-consistent equations including exchange and correlation effects. Phys. Rev. 140, A1133–A1138 (1965).
Blöchl, P. E. Projector augmented-wave method. Phys. Rev. B 50, 17953–17979 (1994).
Boettger, J. C. & Trickey, S. B. Equation of state and properties of lithium. Phys. Rev. B 32, 3391–3398 (1985).
Perdew, J. P. et al. Atoms, molecules, solids, and surfaces: applications of the generalized gradient approximation for exchange and correlation. Phys. Rev. B 46, 6671–6687 (1992).
Jaffe, J. E. et al. Gaussian-basis LDA and GGA calculations for alkali-metal equations of state. Phys. Rev. B 57, 11834–11837 (1998).
Overhauser, A. W. Crystal structure of lithium at 4.2 K. Phys. Rev. Lett. 53, 64–65 (1984).
Liu, A. Y. & Cohen, M. L. Electron-phonon coupling in bcc and 9R lithium. Phys. Rev. B 44, 9678–9684 (1991).
Needs, RJ., Martin, R. M. & Nielsen, O. H. Total-energy calculations of the structural properties of the group-V element arsenic. Phys. Rev. B 33, 3778–3784 (1986).
Johnson, K. A. & Ashcroft, N. W. Nature (submitted).
Straus, D. M. & Ashcroft, N. W. Self-consistent structure of metallic hydrogen. Phys. Rev. Lett. 38, 415–418 (1977).
Natoli, V., Martin, R. M. & Ceperly, D. Crystal structure of molecular hydrogen at high pressure. Phys. Rev. Lett. 74, 1601–1604 (1984).
Overhauser, A. W. Exchange and correlation instabilities of simple metals. Phys. Rev. 167, 691–698 (1968).
Richardson, C. F. & Ashcroft, N. W. High-temperature superconductivity in metallic hydrogen: electron-electron enhancements. Phys. Rev. Lett. 78, 118–121 (1997).
Siringo, F., Pucci, R. & Angilella, G. G. N. Are the light alkali metals still metals under high pressure? High Pressure Res. 15, 255–262 (1997).
Moulopoulos, K. & Ashcroft, N. W. Scaling relations for two-component charged systems: Application to metallic hydrogen. Phys. Rev. B 41, 6500–6519 (1990).
Moulopoulos, K. & Ashcroft, N. W. Generalized Coulomb pairing in the condensed state. Phys. Rev. Lett. 66, 2915–2918 (1991).
Moulopoulos, K. & Ashcroft, N. W. Coulomb interactions and generalized pairing in condensed matter. Phys. Rev. B (in the press).
Vanderbilt, D. Soft self-consistent pseudopotentials in a generalized eigenvalue formalism. Phys. Rev. B 41, 7892–7895 (1990).
Kresse, G. & Hafner, J. Norm-conserving and ultrasoft pseudopotentials for first-row and transition elements. J. Phys. Condens. Matter 6, 8245–8257 (1994).
Lin, T. H. & Dunn, K. J. High-pressure and low-temperature study of electrical resistance of lithium. Phys. Rev. B 33, 807–811 (1986).
Cracknell, A. P. Landolt-Börnstein: Numerical Data and Functional Relationships in Science and Technology (eds Hellwege, K.-H. & Olsen, J. L.) Group III, Vol. 13c, 451–462 (Springer, Berlin, (1984).
Sherrington, D. & Kohn, W. Frequency-dependent dielectric function of a zero-gap semiconductor. Phys. Rev. Lett. 21, 153–156 (1968).
Zittel, W. G. et al. Band-reordering effects in the ultra-high-pressure equation of state of lithium. J. Phys. F 15, L247–L251 (1985).
Boettger, J. C. & Albers, R. C. Structural phase stability in lithium to ultrahigh pressures. Phys. Rev. B 39, 3010–3014 (1989).
Cohen, M. H. Optical constants, heat capacity, and the Fermi surface. Phil. Mag. 3, 762–775 (1958).
Acknowledgements
We thank K. A. Johnson and M. P. Teter for discussions, and we thank G. Kresse, J.Furthmüller and J. Hafner for providing the VASP software. This work was supported by the NSF.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Neaton, J., Ashcroft, N. Pairing in dense lithium. Nature 400, 141–144 (1999). https://doi.org/10.1038/22067
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1038/22067
This article is cited by
-
Data-driven prediction of complex crystal structures of dense lithium
Nature Communications (2023)
-
Integrating surface and interface engineering to improve optoelectronic performance and environmental stability of MXene-based heterojunction towards broadband photodetection
Nano Research (2023)
-
The high-pressure lithium–palladium and lithium–palladium–hydrogen systems
Scientific Reports (2022)
-
Materials under high pressure: a chemical perspective
Applied Physics A (2022)
-
Chemistry under high pressure
Nature Reviews Chemistry (2020)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.