Main

General relativity and quantum physics are expected to merge at the Planck scale, defined by distances of the order of ∼Lp and/or extremely high energies of the order of ∼Ep = cℏ/Lp = 1.2×1019 GeV. Therefore, present approaches to test quantum gravitational effects are mainly focused on high-energy astronomical events13,14,15, which allowed stringent limits to the predicted breaking of Lorentz invariance at the Planck scale to be put in place16. On the other hand, the emergence of a minimal length scale can result in relevant consequences also for low-energy quantum mechanics experiments. The Heisenberg relation states that the uncertainties in the measurements of a position Δx and its conjugate momentum Δp are related by ΔxΔp≥ℏ/2; that is, the position and the momentum of a particle cannot be determined simultaneously with arbitrarily high accuracy. However, an arbitrarily precise measurement of only one of the two observables, say position, is still possible at the cost of our knowledge about the other (momentum), a fact that is obviously incompatible with the existence of a minimal observable distance. This consideration motivates the introduction of generalized Heisenberg uncertainty principles1,2,3,4,5,6,7. As a consequence, an alternative way to check quantum gravitational effects would be to perform high-sensitivity measurements of the uncertainty relation, to reveal any possible deviation from predictions of standard quantum mechanics9,10,11.

In this framework, an optical experiment has recently been proposed to test quantum gravitational modifications of the canonical commutator for the variables associated with the centre of mass of a macroscopic object12. The generalization from the position/momentum of a single particle to that defining a collective motion is well described in standard quantum mechanics. The canonical commutator [x,p] = iℏ remains valid for any Lagrangian coordinate x and its conjugate momentum p, including the coordinates describing the centre of mass or a normal vibrational mode of a macroscopic object. Recent experiments have indeed cooled down mechanical modes of micro-oscillators to their quantum ground state17,18,19, and in this condition the oscillators indeed exhibited peculiar quantum properties17,20. This clearly proves that a mechanical normal mode can be described by quantum-mechanical observables. On the other hand, if a modified commutator is considered, a self-consistent description in terms of macroscopic coordinates is not straightforward. Some recent works have tackled the problem, proposing solutions that imply a strong suppression of the expected effect of Planck-scale physics when probed by multi-particle objects21,22. However, none of such approaches is fully satisfactory. An experimental analysis is therefore highly desirable, in particular if performed on macroscopic variables that exhibit quantum properties.

A direct consequence of a modified commutator, when applied to the position and momentum of an harmonic oscillator, is a change in the oscillator ground-state energy Emin with respect to the usual ℏω0/2. The commutators commonly proposed in quantum gravity theories23,24, giving origin to a position indetermination larger than in standard quantum mechanics (gravitational induced uncertainty), translate into a larger minimal energy. Therefore, an experiment measuring a low energy level Eexp for a normal mode of a macroscopic system puts a straightforward upper limit to the corresponding Emin:

Experimental systems particularly suitable for exploiting this relation are the cryogenic Weber bars, originally conceived and still working as detectors for gravitational waves25. They consist of large metallic bars, weighing several tons and having a main longitudinal mechanical mode oscillating around ω0/2π≃1 kHz. For our purpose, their favourable characteristics are their very large mass (M∼1013 times the Planck mass Mp = Ep/c2 = 2.2×10−8 kg); the large size of the ground-state momentum wave packet associated with their main modes (where, with respect to, for example, micro-oscillators, the relatively low frequency is compensated by the large effective mass Meff); and the low level of thermal energy that is reached experimentally.

Some Weber bars have been operated at ultracryogenic temperature26. However, for our purpose we focus on the AURIGA detector, whose first longitudinal mode, starting from a background bar temperature of 4.2 K, has been further cooled using a cold damping technique down to the millikelvin regime27, a level that could be reached owing to its high mechanical quality factor. We remark that modal cooling techniques have been exploited to bring mechanical modes of micro-oscillators to their quantum ground state and that these experiments have proved that quantum behaviour of macroscopic coordinates can be obtained in this way17,18,19,20. Therefore cold damping is a valid technique for our purpose of reaching and measuring the lowest oscillator modal energy, even if such modal cooling cannot be exploited to increase the sensitivity of the oscillating system as a detector of external excitation.

In AURIGA, the modal motion is measured by coupling the bar to two further oscillators: the first is a mechanical one, having the same frequency but a lower mass, and working as a resonant mechanical amplifier read by a capacitive transducer; the second one is a nearly resonant electrical LC circuit coupled to a d.c. superconducting quantum interference device amplifier28,29. All of the three oscillators have been cooled down by cold damping, to roughly the same temperature. A detailed analysis of the system shows that a minimal energy of 1.3×10−26 J can be attributed to the first longitudinal mode of the bar, whose resonance frequency is ω0/2π = 900 Hz (ref. 27). The motion of this mode is symmetrical with respect to the plane, perpendicular to the bar axis, that bisects the bar, and implies an oscillation of the centre-of-mass of each half-bar. The reduced mass of this couple of centre-of-masses is Mred = M/2 = 1.1×103 kg (where M is the bar physical mass), and the energy associated with the oscillation of the centre-of-masses is ∼ 80% of the total modal energy. The measured modal energy Eexp is therefore an upper limit also for the minimal energy Emin of the oscillation of the centre-of-masses and it can be used in equation (1) and in the relations that follow from it (a detailed description of the AURIGA detector, of the minimal measured energy and a discussion on the appropriate value of the mass to be used in this analysis are reported in the Supplementary Information).

We start our analysis from the uncertainty relation1,2,7

where β0 is a dimensionless parameter that should be around unity if the modification is efficient at the Planck scale. If β0 is larger than unity, it defines a new length scale where some new physics should come into play9. Normalizing the coordinate and momentum to their ground-state wave-packet size according to and , the Hamiltonian operator for a harmonic oscillator is written as

and the uncertainty relation given in equation (2) becomes

where β = β0 (ℏm ω0/Mp2c2). The minimal oscillator energy is found for 〈X〉 = 〈P〉 = 0 using the equality in equation (4). Extracting ΔX from equation (4) and inserting it in equation (3) we obtain

and minimizing with respect to ΔP we find the minimal energy

where the last approximation (which simplifies the form but is not indeed necessary) is valid for β≫1. Comparing this expression of Emin with the measured Eexp, according to equation (1), we obtain β<(2Eexp/ℏω0), which for β0 can be written in the meaningful form

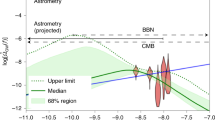
We now come back to the first longitudinal mode of AURIGA with cold damping, and consider the oscillation of the couple of centre-of-masses of the half-bars. Using the mode resonance frequency, the reduced mass Mred and the measured modal energy Eexp = 1.3×10−26 J, we obtain β<4.4×104 and β0<3×1033. Our upper limit for β0 is still far from forbidding new physics at the Planck scale. It can be compared to similar limits of β0<1034, imposed by the lack of observed deviations from standard theory at the electroweak scale; β0<1036, calculated from the accurate measurement of the Lamb shift in hydrogen9; and β0<4×1034 from the 1S–2S level energy difference, again in hydrogen22. These limits are summarized in Fig. 1, together with those obtainable with the same method from other experiments with cold macroscopic oscillators (listed in the Supplementary Information).

Figure 1: Upper limits to the parameter β0 that quantifies the deformation of the standard uncertainty relation in equation (2).
figure 1

The star corresponds to the AURIGA experiment discussed in the main text, and filled circles refer to several experiments on mechanical oscillators that are summarized in the Supplementary Information. The experimental energy Eexp is reported on the vertical axis in terms of oscillator occupation number nt, according to . The dashed lines are levels of , which can be interpreted as the upper limit for the length scale (normalized to the Planck length) where some new physics could come into play. Solid lines report the upper limit to β0 that are given by: the lack of observed deviations from standard theory at the electroweak scale (blue line); the measurement of the Lamb shift in hydrogen (green line); the measurement of the 1S–2S level energy difference, again in hydrogen (red line).

Full size image

In analysing the consequence of generalized Heisenberg uncertainty principles on the foreseen behaviour of a macroscopic object, the preliminary assumption that the modified rules can be applied to macroscopic coordinates must be carefully taken into account. With this consideration in mind, our experimental analysis has a double interpretation. On one side, it sets interesting limits to the Planck-scale physics, and helps to compare the consequences of various approaches to quantum gravity. On the other side, it strongly calls for an effort in developing theories that offer a reasonable path from basic properties of spacetime geometry and of the measurement process (that is, main issues of the theories that should merge into quantum gravity) to multi-particle and macroscopic reality. This research field, which suffers from poor experimental feedback, is likely to benefit from precise metrological systems, including for example interferometric gravitational wave detectors25,30,31, whose data are already the subject of analyses focused on quantum gravity effects32,33, and ultracryogenic devices that could in the near future include, owing to dedicated experiments, oscillators with mass of the order of, or larger than, Mp operating in their fundamental quantum state27.