Main

Unlike the well-known quantum phenomena of superfluidity and superconductivity, the fractional quantum Hall effect1 does not entail any Bose–Einstein condensation but occurs as a result of the formation of topological electron–vortex bound states called composite fermions3. Transport4, light scattering5,6 and phonon scattering7,8 have been extensively used during the past quarter of a century to probe its numerous excitations. Of particular significance is the neutral collective mode, which was first studied theoretically at Landau-level filling ν=1/3 in a single-mode approximation9, wherein, following Feynman’s theory of the phonon–roton mode of helium superfluid, the excitation is modelled as a density wave. The neutral collective mode was detected by Raman scattering5, with the observations generally consistent with the predictions of the single-mode approximation in the long-wavelength limit. More recently, however, Hirjibehedin et al. 2 have discovered that this mode is not a single mode, as believed earlier, but splits into two as the wave vector is increased. By definition, the single-mode approximation cannot accommodate a doublet. A hydrodynamic approach has been proposed10 to account for the experimental observation, but does not take into account the microscopic physics of the fractional quantum Hall effect (FQHE), does not naturally explain the merging of the two modes in the long-wavelength limit and also greatly underestimates the splitting.

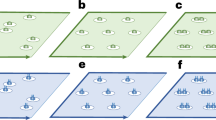
We show here that this new mode finds a natural explanation within the composite-fermion theory3. Composite fermions are bound states of electrons and an even number (2p) of quantized vortices. Because of the Berry phases produced by the bound vortices, composite fermions effectively experience a much reduced magnetic field B*=B−2p ρ φ0 (B is the external magnetic field, ρ is the two-dimensional density and φ0=h c/e is called the flux quantum). Composite fermions form their own Landau-like kinetic energy levels in this reduced magnetic field, called Λ levels, and their filling factor ν* is related to the electron filling factor ν through the relation ν=ν*/(2p ν*+1). In particular, at ν=n/(2p n+1), the ground state consists of n filled Λ levels. In the composite-fermion theory, the lowest-energy neutral excitation is a particle–hole pair, or an exciton, of composite fermions, wherein a single composite fermion from the topmost occupied Λ level is excited into the lowest unoccupied Λ level (Fig. 1b shows the fundamental composite-fermion exciton at ν=2/5). The validity of this description has been confirmed for fractions of the form ν=n/(2p n+1) by comparison to exact diagonalization results as well as to experiment11,12. This physical explanation for the neutral collective excitations is distinct from the single-mode approximation, and, in particular, suggests the possibility of extra collective modes, in which a composite fermion is excited across two or more Λ levels, as shown schematically in Fig. 1c, in complete analogy to the collective modes of an integral quantum Hall state13. However, it is far from obvious that the composite-fermion collective modes across different Λ levels should merge in the long-wavelength limit. In fact, a model that takes composite fermions as non-interacting produces collective modes spaced by the effective cyclotron energy in the long-wavelength limit, as also found for the dispersions obtained in the composite-fermion Chern–Simons approach14,15; if correct, this would make such physics irrelevant to the new collective mode discovered in ref. 2. For a more definitive test, however, the composite-fermion exciton-mode spectrum must be evaluated in a microscopic approach that includes effects of inter-composite-fermion interactions.

Figure 1: Schematic diagram of composite-fermion excitons.
figure 1

Each composite fermion is shown as an electron carrying vortices represented by arrows. a, Representation of the ground state at ν=2/5 as two filled Λ levels. b,c, 1→2 (b) and 0→2 (c) composite-fermion excitons.

Full size image

Exact diagonalization studies of the FQHE state do not by themselves provide an understanding of the underlying physics, and are not useful in the present context, because, as seen below, systems as large as 200 particles are required for investigating the experimentally relevant wave vectors; the Fock space increases exponentially with the number of particles, and at present exact diagonalization is possible only for 10–12 particles for the filling factors of interest here. Our quantitative investigations below exploit accurate trial wavefunctions for composite fermions3. The standard spherical geometry is used in our calculations, which considers electrons moving on the surface of a sphere, subjected to a radial magnetic field. The magnetic field can be thought to emanate from a ‘magnetic monopole’ of strength Q at the centre, which produces a total magnetic flux of 2Q φ0 through the surface of the sphere. This maps into a system of composite fermions at an effective flux Q*=Q−N+1, with Q chosen so that the state at Q* is an integral quantum Hall state at filling ν*=n. The wavefunction ΨCF−g for the FQHE ground state at ν=n/(2n+1) is obtained by composite-fermionizing the ν*=n integral quantum Hall state Φg. To model neutral collective excitations, we first construct wavefunctions of the excitons of the integral quantum Hall state, denoted by {Φλ,Lex}, where L is the total orbital angular momentum of the exciton and λ labels different excitons of the type shown in Fig. 1. We composite-fermionize this basis to obtain {Ψλ,LCF−ex}, which gives a set of basis functions for composite-fermion excitons. We orthonormalize this basis and diagonalize the Coulomb Hamiltonian to obtain the energies of the physical excitations, and also their wavefunctions {χλ,LCF−ex}. The scalar products of various basis functions and the Hamiltonian matrix elements are evaluated by the Metropolis Monte Carlo method. Blocks of different L are not coupled by the interaction, so can be diagonalized separately. More details can be found in refs 16, 17 and Supplementary Information.

Even though our immediate interest is in understanding the splitting of the collective mode at ν=1/3, we consider, for completeness, the three lowest collective modes at two filling factors: 0→1, 0→2 and 0→3 modes at ν=1/3, and 1→2, 1→3 and 0→2 modes at ν=2/5. We have studied systems with 50, 100 and 200 particles at both ν=1/3 and ν=2/5. The results shown in Fig. 2a, b refer to the 200-particle system, which we believe accurately represents the thermodynamic limit. The exciton dispersions are quoted as a function of the wave vector q, defined as q=L/R, where is the radius of the sphere in the unit of magnetic length . The dispersion curves are obtained by averaging over 1.2×107 Monte Carlo iterations.

Figure 2: Dispersions of several composite-fermion excitons and their spectral weights.
figure 2

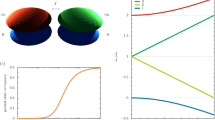
a, The three lowest composite-fermion exciton modes at ν=1/3, obtained from 0→1, 0→2 and 0→3 excitons. The error bar at the end of each curve represents the typical statistical error in the energy determined by the Monte Carlo method. The energies are quoted in units of e2/εℓ, where ε is the dielectric constant of the background semiconductor and is the magnetic length. b, The three lowest composite-fermion exciton modes at ν=2/5, obtained from 1→2, 0→2 and 1→3 excitons. c, Spectral weights for the three modes at ν=1/3 for N=100. The curves from top to bottom correspond to the three modes shown in a respectively from bottom to top. d, The spectral weight for the lowest-energy composite-fermion exciton at ν=1/3 at small qℓ for N=50 and N=100. All results in this figure are for the Coulomb eigenstates χλCF−ex.

Full size image

The qualitative and quantitative features of experiment at ν=1/3 are nicely reproduced by our theory. Most strikingly, in Fig. 2a all modes are seen to merge in the long-wavelength limit. We note that this does not require any fine tuning of parameters (the wavefunctions ΨCF−g and ΨCF−ex do not contain any adjustable parameters) but is a robust effect in the composite-fermion theory. To gain an insight into this merging, we compute the overlap between Ψ1,LCF−ex, Ψ2,LCF−ex and Ψ3,LCF−ex at ν=1/3, shown in Fig. 3 for N=200; surprisingly, the overlaps approach unity at small L, becoming precisely equal to 1 for L=2 and L=3. (A comparison is not possible for L=1 because, in the spherical geometry, the smallest total orbital angular momentum available for the n→n+λ exciton is λ.) We have tested this result for many system sizes and believe that it holds in the thermodynamic limit. This demonstrates that all three modes become identical at small wave vectors, explaining why they merge into a single mode at small wave vectors; the single mode at qℓ=0 is to be distinguished from two degenerate modes. When the wavefunctions are linearly independent, multiple modes are produced after orthogonalization.

Figure 3: Overlaps between the ‘unorthogonalized’ exciton basis states.
figure 3

Overlaps 〈Ψ1,LCF−ex|Ψ2,LCF−ex〉 (red circles), 〈Ψ1,LCF−ex|Ψ3,LCF−ex〉 (blue uptriangles) and 〈Ψ2,LCF−ex|Ψ3,LCF−ex〉 (green downtriangles), where Ψλ,LCF−ex are composite-fermion exciton wavefunctions at ν=1/3 before orthogonalization. (The dispersions in Fig. 2a are obtained after orthogonalization of these modes and diagonalizing the Coulomb Hamiltonian in the orthogonal basis.) The overlaps are evaluated for N=200.

Full size image

Even though the composite-fermion exciton states obtained from orthogonal collective modes of the integral quantum Hall effect become exactly equal at L=2 and 3 in our numerical study, we have not succeeded in deriving this result analytically. The wavefunctions are very complicated and the lowest-Landau-level projection does not lend itself to simple analytical treatments. We note that a Fock space reduction for excited states in going from the integral quantum Hall effect to the fractional quantum Hall effect has been found previously in another related context: for total orbital angular momentum L=1, the wavefunction for the 0→1 composite-fermion excitation is identically annihilated by the lowest-Landau-level projection operator11.

For a quantitative confirmation, we compare the theoretical splitting to the observed one. The light scattering experiments probe only very small wave-vector exchanges, but with our large systems we are able to make meaningful quantitative comparisons. The experimental splitting at qℓ=0.15, which is the largest wave vector accessible in experiments, is approximately 0.009(3) e2/(εℓ). With 200 particles, the smallest wave vector directly accessible is qℓ=0.17, but from a smooth extrapolation of the theoretical dispersion (assuming convergence at qℓ→0), we obtain at qℓ=0.15 a splitting of 0.013(5) e2/(εℓ), which is in very good agreement with experiment, especially given the smallness of the energy difference and the neglect, in our calculations, of disorder, Landau-level mixing and finite-width effects, which are all expected to slightly reduce the splitting.

The Raman intensity is proportional to the spectral weight, given by , where the density operator at q=L/R is defined as in terms of spherical harmonics. Figure 2c shows our calculated spectral weights for various orthogonal modes, suggesting that the collective modes involving excitations across several Λ levels are expected to be weaker than the fundamental mode. It is well known9 that in the long-wavelength limit the leading term in the spectral weight, proportional to (qℓ)2, is exhausted by the inter-Landau-level Kohn mode at the cyclotron energy. We have explicitly verified that the spectral weight of the lowest mode at ν=1/3 goes as (qℓ)4 at small wave vectors (Fig. 2d), which serves as an independent test of the accuracy of the method. We believe that the same is true for the other modes at ν=1/3 as well, but have not confirmed it explicitly because their much smaller spectral weights would require substantially more computational time. In spite of the small spectral weight, the intra-Landau-level collective modes can be observable in resonant Raman scattering owing to a strong resonant enhancement of the matrix elements18, although a detailed theory is not yet available.

The relation of the results presented above to the so-called two-roton mode is worth discussing. Motivated by a discrepancy between the experimental and theoretical energies in the long-wavelength limit5,12, previous theoretical studies modelled the long-wavelength mode as a two-roton bound state9,19,20, which was shown in explicit calculations19,20 to have a slightly lower energy than the single exciton, bringing the theoretical energy closer to the experimental one. The two-roton mode can also be interpreted as the single exciton mode hybridized with excitations consisting of two composite-fermion excitons; this is a natural interpretation given that the energy of the two-roton bound state is close to the single exciton energy (in the long-wavelength limit) and the wavefunctions of the two are not orthogonal. The physics discussed in the present work is distinct, in the sense that whereas the two-roton physics deals with corrections to the energy of the fundamental mode in the long-wavelength limit, the present work is concerned with the splitting of that mode at finite wave vectors. In fact, a mixing with excitations consisting of two composite-fermion excitons will lower the energies of all of the collective modes considered here, but we have not studied that effect because it is unlikely to alter the amount of the splitting significantly.

The results presented above have a number of experimental implications. The collective mode at ν=1/3 is in fact seen to split into several modes (rather than just two), although the higher modes are very close in energy at small wave vectors and may not be readily resolvable. Multiple modes are predicted also at ν=2/5. However, in contrast to ν=1/3, the two lowest modes at ν=2/5 do not merge in the long-wavelength limit; the composite-fermion theory thus predicts an absence of splitting of the long-wavelength collective mode at ν=2/5. (This is to be contrasted with the hydrodynamic approach10, which obtains similar behaviour for all fractions.) Our theory also obtains the full dispersion of the new collective modes, which is outside the range of Raman experiments, but possibly observable in photoluminescence experiments in the presence of a ‘grating’ produced by piezoelectric coupling to certain frozen-in phonons, which picks out certain wave vectors in absorption spectra21.