Abstract
We investigate the electromechanical coupling between a nanomechanical resonator and two parametrically coupled superconducting coplanar waveguide cavities that are driven by a two-mode squeezed microwave source. We show that, with the selective coupling of the resonator to the cavity Bogoliubov modes, the radiation-pressure type coupling can be greatly enhanced by several orders of magnitude, enabling the single photon strong coupling to be reached. This allows the investigation of a number of interesting phenomena such as photon blockade effects and the generation of nonclassical quantum states with electromechanical systems.
Similar content being viewed by others
Introduction
Cavity optomechanics1,2,3 and electromechanics4,5,6 are pretty promising for fundamental studies of large-scale quantum phenomena as well as appealing applications in quantum science and technology. Recent experimental progresses have demonstrated ground state cooling of the mechanical resonators7,8, coherent coupling between cavity and mechanical modes9,10,11, optomechanically induced transparency12,13 and the generation of squeezed light14,15. In despite of these remarkable advances, however, there is a serious hindrance in this exciting field, i.e., the radiation-pressure coupling is too weak to ensure dynamics of the system in the single photon strong coupling regime. Current experiments have mainly relied on strong optical driving, which enhances the coupling at the expense of making the effective interaction linear. As a result, this linear optomechanical interaction does not possess the ability of generating nonclassical states or give rise to true photon-photon interactions for implementing single-photon quantum processes.
To give a better understanding and fully exploit the regime of strong radiation pressure coupling, it is highly desirable to find an efficient method for realizing the strong nonlinear interaction between the vibrations and the electromagnetic field in a realistic setup. Such a regime is particularly important to test the fundamental theory of quantum physics and to explore potential applications of optomechanical or electromechanical devices to future quantum technology16,17,18,19,20,21,22,23,24,25,26,27,28,29,30. Some recent theoretical proposals for entering the strong coupling regime include the use of collective effects in arrays of mechanical oscillators31 and Kerr nonlinearity via the Josephson effect32,33, as well as the usage of an inductive coupling to a flux-dependent quantum circuit34.
Recently, a proposal using the squeezed optical cavity field to enhance the nonlinear coupling in an optomechanical system has been introduced35. In that proposal, the single-mode cavity field is squeezed by an optical χ(2) nonlinear medium, while the broadband-squeezed vacuum is introduced to suppress the noise of the squeezed cavity mode. Though this protocol seems promising, it can not be straightforwardly applied to electromechanical systems. First, that proposal needs an optical nonlinear crystal possessing a large χ(2) nonlinearity, which is quite demanding and technically challenging in the field of electromechanics with microwave frequencies. Secondly, in order to have implications in quantum information science, it is desirable to consider multi-cavities rather than a single cavity for the purpose of distributed quantum computation and quantum network36. However, the generalization of this model to the case of coupled optical cavities is still difficult. Fortunately, the above issues can be overcome by considering two coupled superconducting coplanar waveguide (CPW) cavities with the parametrical coupling form37,38.
In this work, we investigate an electromechanical system consisting of a nanomechanical resonator capacitively interacting with two parametrically coupled CPW cavities. We show that, when the cavities are driven by a spectrally broadband two-mode squeezed vacuum, the radiation-pressure type coupling of the resonator to the cavity Bogoliubov modes can be greatly enhanced by several orders of magnitude. By suitably tuning the system parameters such as the squeezing parameter of the driving source, the flux driving frequency and the parametric coupling strength, the single photon electromechanical coupling strength can be tailored such that it can exceed the cavity decay rate. This single photon strong coupling of electromechanical interactions allows the studies of single-photon quantum processes such as photon blockade and the production of nonclassical photon states harnessing the optomechanical nonlinearity. With currently available technology in cavity electromechanics, this proposal can be realistically implemented in experiments and provides an appealing platform for implementing quantum technologies.
Results
The setup
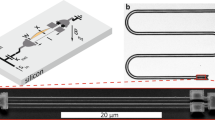
As shown in Fig. 1, we consider an electromechanical system consisting of a nanomechanical resonator capacitively coupled to a superconducting CPW cavity, which is also coupled to another auxiliary waveguide cavity by means of a superconducting quantum interference device (SQUID)37,38. The SQUID driven by external fluxes allows a fast modulation of the electrical boundary condition of the cavities and their interaction37,38. In a recent experiment, it has demonstrated a 3 D microwave superconducting cavity parametrically coupled to a transmission line cavity by a Josephson ring modulator39. The mechanical resonator couples to the cavity field via radiation-pressure coupling, while the two cavities interact with each other with the form of parametric coupling. In the frame rotating at half the flux driving frequency ωd, the system Hamiltonian reads
Schematic of the proposed electromechanical setup.
A nanomechanical resonator capacitively couples to a superconducting CPW cavity, which is also coupled to another cavity with the form of parametric coupling. These cavities are driven by a spectrally broadband two-mode squeezed vacuum from the output of a non-degenerate Josephson parametric amplifier.

where  is the annihilation operator for the jth cavity mode with frequency ωj, Δj = ωj − ωd/2, ξ the parametric coupling strength between the cavities, ωm the mechanical vibration frequency with annihilation operator
is the annihilation operator for the jth cavity mode with frequency ωj, Δj = ωj − ωd/2, ξ the parametric coupling strength between the cavities, ωm the mechanical vibration frequency with annihilation operator  and g the radiation-pressure coupling strength between the resonator and the first cavity. The cavities are driven by an external source of two-mode squeezed microwave field, which can be produced with a non-degenerate Josephson parametric amplifier40,41,42,43. Assuming that the bandwidth of the squeezed microwave field is larger than the cavity damping rate κ, then the interaction between the cavity modes and the external squeezed field is described by44
and g the radiation-pressure coupling strength between the resonator and the first cavity. The cavities are driven by an external source of two-mode squeezed microwave field, which can be produced with a non-degenerate Josephson parametric amplifier40,41,42,43. Assuming that the bandwidth of the squeezed microwave field is larger than the cavity damping rate κ, then the interaction between the cavity modes and the external squeezed field is described by44

Here M and N are related to the statistics of the driving broadband two-mode squeezed field: M accounts for the intermode correlations and N is the average photon number for both modes. For perfect two-mode squeezed vacuum, we have M = sinh r0 cosh r0 and N = sinh2 r0, with r0 the squeezing parameter. Therefore, the master equation describing the system dynamics reads

where

with γm the mechanical decay rate and nth the thermal phonon number of the mechanical mode.
Enhanced electromechanical coupling via squeezed source driving
To get more insight into the system’s dynamics, it is convenient to introduce two delocalized cavity Bogoliubov modes45,46,47

Using these cavity Bogoliubov modes, the cavity driven term can be rewritten as

This means that for the cavity Bogoliubov modes, the dissipation caused by the system-bath coupling is just like that induced by a vacuum bath. Furthermore, if we choose  , with
, with  , then through straightforward derivations, the system Hamiltonian can be written as
, then through straightforward derivations, the system Hamiltonian can be written as

Here

In the interaction picture with respect to

and under the condition Ω1 + Ω2 ≫ g sinh r0 cosh r0, ωm, this allows us to selectively activate interaction terms in the system dynamics as

with  and
and  . In the case of
. In the case of  and
and  , one has
, one has  . This Hamiltonian describes the electromechanical coupling between the resonator and the cavity Bogoliubov modes
. This Hamiltonian describes the electromechanical coupling between the resonator and the cavity Bogoliubov modes  with the effective coupling strengths
with the effective coupling strengths  . Together with the cavity dissipation described by (6) and mechanical decay, the system dynamics is just like that a mechanical resonator simultaneously couples to two photonic modes, with photon dissipation caused by a vacuum bath. In this case, the radiation-pressure coupling strength
. Together with the cavity dissipation described by (6) and mechanical decay, the system dynamics is just like that a mechanical resonator simultaneously couples to two photonic modes, with photon dissipation caused by a vacuum bath. In this case, the radiation-pressure coupling strength  can be greatly enhanced. By suitably tuning the system parameters Δ1,Δ2, ξ and the driving source parameter r0, this coupling strength can be increased by several orders of magnitude to reach the strong-coupling regime, i.e.,
can be greatly enhanced. By suitably tuning the system parameters Δ1,Δ2, ξ and the driving source parameter r0, this coupling strength can be increased by several orders of magnitude to reach the strong-coupling regime, i.e.,  . This is the main result of this work: by coupling the mechanical resonator to cavity Bogoliubov modes and driving the cavities by a broadband two-mode squeezed vacuum, the radiation-pressure coupling can be greatly enhanced, even allowed to reach the strong coupling regime.
. This is the main result of this work: by coupling the mechanical resonator to cavity Bogoliubov modes and driving the cavities by a broadband two-mode squeezed vacuum, the radiation-pressure coupling can be greatly enhanced, even allowed to reach the strong coupling regime.
Figure 2 presents the calculated coupling strength  and the Bogoliubov mode frequency Ω1 as functions of the system parameter ξ. The results for
and the Bogoliubov mode frequency Ω1 as functions of the system parameter ξ. The results for  and Ω2 are similar to those for
and Ω2 are similar to those for  and Ω1 as presented in Fig. 2. As the parameter ξ approaches the critical value ξ0 = 0.5(Δ1 + Δ2), the coupling strength
and Ω1 as presented in Fig. 2. As the parameter ξ approaches the critical value ξ0 = 0.5(Δ1 + Δ2), the coupling strength  can get a very large value, while the Bogoliubov mode frequency Ω1(Ω2) tends to be very small. However, there is an optimal point at which both the coupling strength
can get a very large value, while the Bogoliubov mode frequency Ω1(Ω2) tends to be very small. However, there is an optimal point at which both the coupling strength  and the frequency Ω1(Ω2) can get a relatively large value, i.e.,
and the frequency Ω1(Ω2) can get a relatively large value, i.e.,  and Ω1 ~ 8ωm. The Bogoliubov mode frequencies Ω1 and Ω2 are controllable frequencies, which are determined by the driving frequency detunings Δ1, Δ2 and the parametric pumping strength ξ. They should have a relatively large value as compared to the parameters g sinh r0 cosh r0 and ωm. In this case we can ignore the parametric amplification terms for the Bogoliubov modes in Eq. (7) and obtain the standard electromechanical radiation-pressure interactions displayed in Eq. (10). This confirms that the single photon strong coupling can be reached with the chosen parameters.
and Ω1 ~ 8ωm. The Bogoliubov mode frequencies Ω1 and Ω2 are controllable frequencies, which are determined by the driving frequency detunings Δ1, Δ2 and the parametric pumping strength ξ. They should have a relatively large value as compared to the parameters g sinh r0 cosh r0 and ωm. In this case we can ignore the parametric amplification terms for the Bogoliubov modes in Eq. (7) and obtain the standard electromechanical radiation-pressure interactions displayed in Eq. (10). This confirms that the single photon strong coupling can be reached with the chosen parameters.
We now consider whether the parameter regime is experimentally accessible with current experimental setups. From Fig. 2 we find that the scheme works well when ξ ~ 1000ωm. For nanomechanical resonators with frequencies from several kHz to several MHz, the parametrical coupling strength ξ between the cavities is on the order of hundreds of MHz. This coupling strength is well accessible with current circuit QED setups39. The value of g ~ 0.001ωm is an order of magnitude lager than that achieved via mechanical resonators with frequencies of several MHz in current technology5. However, by the usage of mechanical resonators with low frequencies of hundreds of kHz, the value of g ~ 0.001ωm is accessible in present experiments48. From the inset of this figure, we also note that this proposal requires a two-mode squeezing source with a very large squeezing parameter r0. Though this requirement is somewhat challenging, it is within reach of the state-of-the-art experiments. Recent experiments in two-mode squeezing of microwave radiation rely on the amplification of quantum noise by the Josephson Parametric Converter40,41,42,43. It has been demonstrated that the generated two-mode squeezing source can possess the highest gain of  dB, which corresponds to a squeezing parameter r0 ~ 6.5. Therefore, this protocol can be realized with the state-of-the-art techniques of superconducting integrated circuits and electromechanical systems.
dB, which corresponds to a squeezing parameter r0 ~ 6.5. Therefore, this protocol can be realized with the state-of-the-art techniques of superconducting integrated circuits and electromechanical systems.
Applications
The effective strong coupling offers great potential for single-photon manipulation and quantum states generation. For example, the photon blockade phenomenon existing in the strongly coupled optomechanical system can occur in this system. This is quantitatively characterized by the zero-delay second-order correlation function

This quantity provides a direct experimental measure for nonclassical antibunching effects if  and for
and for  indicates a full photon blockade for the ith Bogoliubov mode.
indicates a full photon blockade for the ith Bogoliubov mode.
We now discuss the feasibility of the measurement of the photon statistics in experiments. A challenge here is that the Bogoliubov modes are a linear combination of the two cavity modes and are more difficult to be addressed separately. We assume the first cavity is weakly driven by a probe field with frequency ωp and amplitude  . The Hamiltonian is given by
. The Hamiltonian is given by

In the frame rotating at half the flux driving frequency ωd and in the Bogoliubov mode representation, we have

with  . Under the condition
. Under the condition  ,
,  , we can safely ignore the rapidly oscillating terms under the rotating-wave approximation
, we can safely ignore the rapidly oscillating terms under the rotating-wave approximation

Therefore, with suitably choosing the probe parameters, one can selectively address the Bogoliubov modes on demand. The measurement of the photon statistics needs monitoring the signal coming out from the system and it is not known how the detailed measurement goes.
In Fig. 3(a), we plot the excitation spectrum for the first Bogoliubov mode

obtained by numerically solving Eq (3), as a function of the detuning  for different values of the coupling strength
for different values of the coupling strength  , with
, with  . We observe in the resolved sideband regime κ ≪ ωm, a redshift of the zero phonon line (ZPL) towards
. We observe in the resolved sideband regime κ ≪ ωm, a redshift of the zero phonon line (ZPL) towards  and the appearance of additional resonances at multiples of the mechanical frequency. These peaks result from phonon-assisted excitation processes, providing a clear indication for single-photon strong coupling optomechanics. In Fig. 3(b) we present numerical results for the second-order correlation function
and the appearance of additional resonances at multiples of the mechanical frequency. These peaks result from phonon-assisted excitation processes, providing a clear indication for single-photon strong coupling optomechanics. In Fig. 3(b) we present numerical results for the second-order correlation function  versus time through solving the master equation (3) with the transformed Hamiltonian (7). It is clear that the steady state value for
versus time through solving the master equation (3) with the transformed Hamiltonian (7). It is clear that the steady state value for  approaches zero for the first Bogoliubov mode, which is a strong signature of photon blockade.
approaches zero for the first Bogoliubov mode, which is a strong signature of photon blockade.
Discussion and Conclusion
In this work, we focus on implementing the idea using two parametrically coupled superconducting cavities in an electromechanical system. The usage of two cavities rather than one is particularly appealing when one considers applying this proposal to quantum information processing such as distributed quantum computation and quantum network. This proposal can also apply to a single microwave cavity capacitively coupled to a mechanical resonator. In this case, it will need a single-mode squeezed field to drive the cavity and a superconducting qubit in the cavity to produce the desired nonlinearity for the cavity mode49.
To conclude, we have proposed an efficient method for enhancing the radiation-pressure type coupling in an electromechanical system. We have shown that, by driving the cavities with a two-mode squeezed microwave source and selectively coupling the resonator to the cavity Bogoliubov modes, the effective interaction strengths between the mechanical resonator and the cavities can be enhanced into the single-photon strong-coupling regime. This allows the investigation of a number of interesting phenomena such as photon blockade effects and the generation of quantum states with currently available technology in the field of cavity electromechanics.
Additional Information
How to cite this article: Li, P.-B. et al. Enhanced electromechanical coupling of a nanomechanical resonator to coupled superconducting cavities. Sci. Rep. 6, 19065; doi: 10.1038/srep19065 (2016).
References
Kippenberg, T. J. & Vahala, K. J. Cavity optomechanics: back-action at the mesoscale. Science 321, 1172–1176 (2008).
Marquardt, F. & Girvin, S. M. Trend: Optomechanics. Physics 2, 40 (2009).
Aspelmeyer, M., Kippenberg, T. J. & Florian Marquardt, F. Cavity optomechanics. Rev. Mod. Phys. 86, 1391–1452 (2014).
Regal, C. A. & Lehnert, K. W. From cavity electromechanics to cavity optomechanics. J. Phys. Conf. Ser. 264, 012025 (2011).
Teufel, J. D. et al. Circuit cavity electromechanics in the strong-coupling regime. Nature 471, 204–208 (2011).
Didier, N. & Fazio, R. Putting mechanics into circuit quantum electrodynamics. C. R. Phys. 13, 470–479 (2012).
Teufel, J. D. et al. Sideband cooling of micromechanical motion to the quantum ground state. Nature 475, 359–363 (2011).
Chan, J. et al. Laser cooling of a nanomechanical oscillator into its quantum ground state. Nature 478, 89–92 (2011).
Verhagen, E., Deléglise, S., Weis, S., Schliesser, A. & Kippenberg, T. J. Quantum-coherent coupling of a mechanical oscillator to an optical cavity mode. Nature 482, 63–67 (2012).
Palomaki, T. A., Harlow, J. W., Teufel, J. D., Simmonds, R. W. & Lehnert, K. W. Coherent state transfer between itinerant microwave fields and a mechanical oscillator. Nature 495, 210–214 (2013).
Zhou, X. et al. Slowing, advancing and switching of microwave signals using circuit nanoelectromechanics. Nature Physics 9, 179–184 (2013).
Weis, S. et al. Optomechanically Induced Transparency. Science 330, 1520–1523 (2010).
Safavi-Naeini, A. H. et al. Electromagnetically induced transparency and slow light with optomechanics. Nature 472, 69–73 (2011).
Brooks, D. W. C. et al. Non-classical light generated by quantum-noise-driven cavity optomechanics. Nature 488, 476–480 (2012).
Safavi-Naeini, A. H. et al. Squeezed light from a silicon micromechanical resonator. Nature 500, 185–189 (2013).
Law, C. K. Interaction between a moving mirror and radiation pressure: A Hamiltonian formulation. Phys. Rev. A 51, 2537–2541 (1995).
Bose, S., Jacobs, K. & Knight, P. L. Preparation of nonclassical states in cavities with a moving mirror. Phys. Rev. A 56, 4175–4186 (1997).
Rabl, P. Photon Blockade Effect in Optomechanical Systems. Phys. Rev. Lett. 107, 063601 (2011).
Nunnenkamp, A., Børkje, K. & Girvin, S. M. Single-Photon Optomechanics. Phys. Rev. Lett. 107, 063602 (2011).
Stannigel, K. et al. Optomechanical Quantum Information Processing with Photons and Phonons. Phys. Rev. Lett. 109, 013603 (2012).
Ludwig, M., Safavi-Naeini, A. H., Painter, O. & Marquardt, F. Enhanced Quantum Nonlinearities in a Two-Mode Optomechanical System. Phys. Rev. Lett. 109, 063601 (2012).
Lü, X. Y., Zhang, W. M., Ashhab, S., Wu, Y. & Nori, F. Quantum-criticality induced strong Kerr nonlinearities in optomechanical systems. Sci. Rep. 3, 2943 (2013).
Liao, J. Q. & Nori, F. Single-photon quadratic optomechanics. Sci. Rep. 4, 6302 (2014).
Vanner, M. R. Selective Linear or Quadratic Optomechanical Coupling via Measurement. Phys. Rev. X. 1, 021011 (2011).
Liu, Y. C., Xiao, Y. F., Chen, Y. L., Yu, X. C. & Gong, Q. H. Parametric Down-Conversion and Polariton Pair Generation in Optomechanical Systems. Phys. Rev. Lett. 111, 083601 (2013).
Lemonde, M.-A., Didier, N. & Clerk, A. A. Nonlinear Interaction Effects in a Strongly Driven Optomechanical Cavity. Phys. Rev. Lett. 111, 053602 (2013).
Børkje, K., Nunnenkamp, A., Teufel, J. D. & Girvin, S. M. Signatures of Nonlinear Cavity Optomechanics in the Weak Coupling Regime. Phys. Rev. Lett. 111, 053603 (2013).
Zhang, K. Y., Bariani, F. & Meystre, P. Quantum Optomechanical Heat Engine. Phys. Rev. Lett. 112, 150602 (2014).
Li, H. K. et al. Proposal for a near-field optomechanical system with enhanced linear and quadratic coupling. Phys. Rev. A 85, 053832 (2012).
Xu, G. F. & Law, C. K. Dark states of a moving mirror in the single-photon strong-coupling regime. Phys. Rev. A 87, 053849 (2013).
Xuereb, A., Claudiu Genes, C. & Dantan, A. Strong Coupling and Long-Range Collective Interactions in Optomechani cal Arrays. Phys. Rev. Lett. 109, 223601 (2012).
Heikkilä, T. T., Massel, F., Tuorila, J., Khan, R. & Sillanpää, M. A. Enhancing Optomechanical Coupling via the Josephson Effect. Phys. Rev. Lett. 112, 203603 (2014).
Liao, J.-Q., Jacobs, K., Nori, F. & Simmonds, R. W. Modulated electromechanics: large enhancements of nonlinearities. New J. Phys. 16, 072001 (2014).
Via, G., Kirchmair, G. & Romero-Isart, O. Strong Single-Photon Coupling in Superconducting Quantum Magnetomechanics. Phys. Rev. Lett. 114, 143602 (2015).
Lü, X.-Y. et al. Squeezed Optomechanics with Phase-Matched Amplification and Dissipation. Phys. Rev. Lett. 114, 093602 (2015).
Li, P.-B., Gu, Y., Gong, Q.-H. & Guo, G.-C. Quantum-information transfer in a coupled resonator waveguide. Phys. Rev. A 79, 042339 (2009).
Abdo, B., Kamal, A. & Devoret, M. Nondegenerate three-wave mixing with the Josephson ring modulator. Phys. Rev. B 87, 014508 (2013).
Felicetti, S. et al. Dynamical Casimir Effect Entangles Artificial Atoms. Phys. Rev. Lett. 113, 093602 (2014).
Flurin, E., Roch, N., Pillet, J. D., Mallet, F. & Huard, B. Superconducting Quantum Node for Entanglement and Storage of Microwave Radiation. Phys. Rev. Lett. 114, 090503 (2015).
Murch, K. W., Weber, S. J., Beck, K. M., Ginossar, E. & Siddiqi, I. Reduction of the radiative decay of atomic coherence in squeezed vacuum. Nature 499, 62–65 (2013).
Bergeal, N. et al. Phase-preserving amplification near the quantum limit with a Josephson ring modulator. Nature 465, 64–68 (2010).
Eichler, C. et al. Observation of Two-Mode Squeezing in the Microwave Frequency Domain. Phys. Rev. Lett. 107, 113601 (2011).
Bergeal, N., Schackert, F., Frunzio, L. & Devoret, M. H. Two-Mode Correlation of Microwave Quantum Noise Generated by Parametric Down-Conversion. Phys. Rev. Lett. 108, 123902 (2012).
Kraus, B. & Cirac, J. I. Discrete Entanglement Distribution with Squeezed Light. Phys. Rev. Lett. 92, 013602 (2004).
Li, P.-B., Gao, S.-Y. & Li, F.-L. Robust continuous-variable entanglement of microwave photons with cavity electromechanics. Phys. Rev. A 88, 043802 (2013).
Tian, L. Robust Photon Entanglement via Quantum Interference in Optomechanical Interfaces. Phys. Rev. Lett. 110, 233602 (2013).
Wang, Y. D. & Clerk, A. A. Reservoir-Engineered Entanglement in Optomechanical Systems. Phys. Rev. Lett. 110, 253601 (2013).
Massel, F. et al. Multimode circuit optomechanics near the quantum limit. Nat. Commun. 3, 987 (2012).
Bourassa, J., Beaudoin, F., Gambetta, J. M. & Blais, A. Josephson-junction-embedded transmission-line resonators: From Kerr medium to in-line transmon. Phys. Rev. A 86, 013814 (2012).
Acknowledgements
This work was supported by the National Nature Science Foundation of China (Grants No. 11474227, No. 11174233 and No. 11374239) and the Fundamental Research Funds for the Central Universities. Part of the simulations are coded in PYTHON using the QUTIP library.
Author information
Authors and Affiliations
Contributions
P.B.L. conceived the idea and carried out the calculations. H.R.L. and F.L.L. participated in the discussions. All authors contributed to the interpretation of the work.
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Li, PB., Li, HR. & Li, FL. Enhanced electromechanical coupling of a nanomechanical resonator to coupled superconducting cavities. Sci Rep 6, 19065 (2016). https://doi.org/10.1038/srep19065
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/srep19065
This article is cited by
-
Photon-phonon squeezing and entanglement in a cavity optomechanical system with a flying atom
Frontiers of Physics (2019)
-
Preparing entangled states between two NV centers via the damping of nanomechanical resonators
Scientific Reports (2017)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.


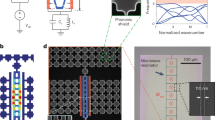


 and the Bogoliubov mode frequency Ω1 versus the system parameter ξ.
and the Bogoliubov mode frequency Ω1 versus the system parameter ξ. versus the squeezing parameter r0.
versus the squeezing parameter r0.
 in the resolved sideband regime κ ~ 0.1ωm. (b) The second-order correlation function
in the resolved sideband regime κ ~ 0.1ωm. (b) The second-order correlation function  versus time. In both plots, T = 0 and Q = 103.
versus time. In both plots, T = 0 and Q = 103.