Abstract
URu2Si2 is a uranium compound that exhibits a so-called ‘hidden-order’ transition at ~17.5 K. However, the order parameter of this second-order transition as well as many of its microscopic properties remain unclarified despite considerable research. One of the key questions in this regard concerns the type of spontaneous symmetry breaking occurring at the transition; although rotational symmetry breaking has been detected, it is not clear whether another type of symmetry breaking also occurs. Another key question concerns the property of Fermi-surface gapping in the momentum space. Here we address these key questions by a momentum-dependent observation of electronic states at the transition employing ultrahigh-resolution three-dimensional angle-resolved photoemission spectroscopy. Our results provide compelling evidence of the spontaneous breaking of the lattice's translational symmetry and particle-hole asymmetric gapping of a heavy quasiparticle pocket at the transition.
Similar content being viewed by others
Introduction
In the field of strongly correlated electron systems, the term ‘hidden order’ is often used to refer to a second-order phase transition whose order parameter has not yet been identified. One of the most well-known examples of this phenomenon is the ‘hidden-order’ transition in URu2Si2. This transition shows clear anomalies in terms of specific heat and transport data at THO = ~17.5 K1,2,3. However, previous studies have found no experimental indication of structural transition or conventional intrinsic magnetic order. Although a neutron scattering experiment detected a small ordered moment (~0.03 μB per uranium atom, where μB represents the Bohr magneton) below THO4, the magnitude was too small to explain the large entropy release upon the transition. This small moment is now ascribed to an extrinsic effect5. Many theoretical models have been proposed to explain this mysterious order6,7,8,9,10,11,12,13,14,15,16; nonetheless, various important questions remain to be addressed experimentally.
The key questions with regard to this mysterious second-order transition are the type of spontaneous symmetry breaking and the property of Fermi-surface gapping in the momentum space. A recent magnetic torque experiment reported the breaking of four-fold rotational symmetry upon the transition17; however, the existence of other types of symmetry breaking remains an open question. In particular, while scanning tunneling spectroscopy (STS) studies ruled out electronic periodicity modulation within the a–b plane18,19, possible translational symmetry breaking along the c axis (or kz direction in the momentum space) has been indirectly suggested by neutron scattering20 and further supported by quantum oscillation21. (Figure S1(a) in the ‘Supplementary Information’ illustrates the crystal structure of URu2Si2.) Despite no experimental indication of structural change, a spectroscopic study may still be beneficial because the change in electronic states can be more drastic. As for the Fermi-surface gapping, two complementary spectroscopic probes have not revealed any consistent information: STS showed a gap opening at the Fermi level in the local density of states18,19, but angle-resolved photoemission spectroscopy (ARPES), a momentum-space counterpart of STS, did not22,23,24,25. Therefore, although momentum-dependent electronic states have been reported, more detailed investigations both in the paramagnetic state and in the hidden-order state are necessary to address the above-mentioned key questions.
To study the momentum-dependent modifications of electronic states as a function of temperature, we employed ultrahigh-resolution three-dimensional ARPES. The tuning of excitation-photon energy allows us to probe various kz values in the Brillouin zone of URu2Si2. (The Brillouin zone is illustrated in Fig. S1(b) in the ‘Supplementary Information’.) Moreover, previous studies that reported the observation of a narrow band22,23 have indicated that meV-scale changes near the Fermi level can be important. Therefore, for our research purposes, we performed our experiments on high-quality single crystals at an ultrahigh-resolution ARPES endstation located at a third-generation synchrotron source.
Results
Paramagnetic state
Photon-energy-dependent measurements in the paramagnetic state reveal several important insights into the observed band structures. Figure 1 shows ultrahigh-resolution ARPES data for the Γ-X direction recorded at 20 K. We used hν ~ 19 eV and ~34 eV to probe the Γ point and the Z point, respectively26. The upper panel shows data measured using 19-eV photons; the intensity map in Fig. 1(a) shows a hole pocket (kF ~ 0.16 Å−1) as well as a hole-like dispersive band whose top is well below the Fermi level. In addition, the corresponding energy-distribution curves (EDCs) in Fig. 1(b) and the momentum-distribution curves (MDCs) in Fig. 1(c) consistently provide evidence for these bands. The lower panel shows ARPES data measured using 34-eV photons; the intensity map in Fig. 1(d) clearly shows a hole-like band whose top exists well below the Fermi level and the MDCs in Fig. 1(f) show the existence of a hole pocket. While the hole-like band observed at two different photon energies was experimentally shown as a surface-originated state22,27, the hole pocket is also suggested as a surface-derived state owing to the absence of kz dependence and its inconsistency with bulk-sensitive measurements (see Supplementary Fig. S2 and Supplementary Discussion 1 for details). Therefore, unlike previous studies that regarded the hole pocket as a bulk state, we suggest that the hole pocket is not related to the hidden-order transition.
High-resolution ARPES data measured along the Γ-X direction for the paramagnetic state of URu2Si2 (20 K).
(a) ARPES intensity map measured using 19-eV photons. The momentum-distribution curve (MDC) at the Fermi level fitted with Lorentzian functions is also shown. (b) The energy distribution curves (EDCs) corresponding to the data in (a). (c) The MDCs corresponding to (a). (d) ARPES intensity map measured using 34-eV photons. The MDC at the Fermi level fitted with Lorentzian curves is also shown. (e) EDCs corresponding to (d). (f) MDCs corresponding to (d). Note that the positions of kF are indicated by dots in (c) and (f) and the broken lines in (c), (e) and (f) are guides for the eye.
One striking observation in our data is the existence of an additional structure in the 34-eV data. Although this additional structure was reported as a broad feature near the Fermi level24,25, the EDCs in Fig. 1(e) clearly show for the first time that the peak disperses toward the Fermi level as |k110| increases; this observation directly evidences the existence of an electron-like band. Furthermore, the MDCs in Fig. 1(f) suggests that this electron-like band crosses the Fermi level at the wave vector kF ~ 0.30 Å−1. To further confirm this picture, ARPES data normalized by resolution-convoluted Fermi functions are shown in Supplementary Fig. S3; these data indicate that (1) no flat feature is discernible both above and below the Fermi level in the 19-eV data and (2) the electron band observed in the 34-eV data crosses the Fermi level at a wave vector consistent with the MDC-derived kF value (see Supplementary Discussion 2 for additional notes). In addition, because the electron band is kz dependent, it should be assigned as a bulk state. We note that the observation of a kz-dependent, well-defined dispersion of electron pocket is a new experimental insight which was not known previously.
Hidden-order state
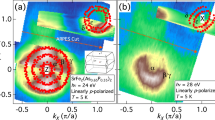
Entering the hidden-order state results in an abrupt change in the electronic structures near the Fermi level. Figure 2(a) and 2(d) show the ARPES intensity maps measured at 2 K by 19- and 34-eV photons, respectively; both maps show a narrow ‘M-shaped’ band near the Fermi level, a hole-like band and a hole pocket, of which the last two are irrelevant to the hidden-order transition. In the corresponding second-derivative maps shown in Fig. 2(b) and 2(e), the ‘M-shape’ of the band is more clearly resolved. Moreover, the EDCs in Fig. 2(c) and 2(f) do not support the Fermi-level crossing of the ‘M-shaped’ band because well-defined peaks appear below the Fermi level. This narrow ‘M-shaped’ band is similar to the one observed in the previous high-resolution studies22,23,24,25; however, it should be pointed out that while no flat dispersive feature was observed in the 19-eV data at 20 K, the ‘M-shaped’ band suddenly becomes observable by the use of 19-eV photons in the hidden-order state. In addition, we note that the ‘M-shaped’ band has its top at ktop ~ 0.16 Å−1 (indicated by the orange arrow in Fig. 2(e)) and disperses away from the Fermi level around kF ~ 0.30 Å−1 (Fermi vector of the electron pocket indicated by the red arrow in Fig. 2(e)); this observation strongly suggests gap opening in the electron-like Fermi surface. Furthermore, because EDC-derived peak positions in the 19-eV data (black circles in Fig. 2(e)) match the dispersion in the 34-eV data, it is suggested that these ‘M-shaped’ bands have the same origin.
High-resolution ARPES data measured along the Γ-X direction for the hidden-order state of URu2Si2 (2 K).
(a) ARPES intensity map measured using 19-eV photons. (b) The second-derivative map corresponding to (a). (c) The energy-distribution curves corresponding to (a). (d) ARPES intensity map measured using 34-eV photons. (e) The second-derivative map corresponding to (d). Black circles show the EDC-derived peak positions of the ‘M-shaped’ band in the 19-eV data. The red arrow indicates the position of kF and the orange arrow shows the top of the ‘M-shaped’ band. (f) The energy-distribution curves corresponding to (d). The broken lines in (c) and (f) are guides for the eye. Note that the spectra were measured with circular polarization.
Temperature-dependent electronic states
The temperature dependence of the photoemission spectra is shown in detail in Fig. 3, with an emphasis on how the ‘M-shaped’ band is formed through the transition. Figure 3(a) shows the temperature dependence of the second-derivative maps as measured using 34-eV photons (the second-derivative maps are compared with the corresponding raw data in Supplementary Figure S4). Above the critical temperature, the renormalized electron pocket is observed below the Fermi level. The electron pocket starts to change its shape when cooled below the critical temperature and further cooling results in the transformation of the electron pocket into an ‘M-shaped’ band. This temperature dependence confirms that the electron pocket is the origin of the ‘M-shaped’ band; since the hidden-order transition involves U 5f electrons2, it is suggested that the electron pocket originates from a U 5f state. In contrast, the temperature dependence of the intensity map as measured using 19-eV photons in Fig. 3(b) shows that the intensity of the ‘M-shaped’ band appears and is enhanced only below the critical temperature.
Temperature dependence of ‘M-shaped’ band probed at two high-symmetry points.
(a) Temperature dependence of the second-derivative maps measured using 34-eV photons (b) Temperature dependence of ARPES intensity maps measured using 19-eV photons. (c) The spectral cuts at k110 = 0 Å−1 recorded using 34-eV photons. (c) The spectral cuts at kF = 0.30 Å−1 for 34 eV. (d) The energy-distribution curves at k110 = 0 Å−1 for 19 eV. (f) The spectral cuts at k110 = 0.16 Å−1 for 19 eV. The EDCs at 2 K and 12 K are also presented in the inset after being normalized by the resolution-convoluted Fermi functions.
The temperature dependence of EDCs, as shown in Fig. 3(c)–(f), further clarify the momentum-dependent changes of electronic states. The spectral cut at k110 = 0 Å−1 as measured using 34-eV photons, shown in Fig. 3(c), indicates a peak develops upon cooling at EB ~ 7 meV. Moreover, at the Fermi wave vector of kF ~ 0.30 Å−1 (Fig. 3(d)), the leading edge shifts toward the higher EB and a sharp peak develops at E − EF ~ 7 meV; these observations further support the gapping of the electron pocket upon the hidden-order transition. In contrast to the 34-eV data, the EDCs of 19-eV data in Fig. 3(e) and Fig. 3(f) show that a peak appears both at k110 = 0 Å−1 and at k110 = 0.16 Å−1 below the critical temperature. Moreover, the both peaks shift toward higher binding energies; although the shift is not clear for the cut at k110 = 0.16 Å−1 owing to the thermal effect, the EDCs normalized by the resolution-convoluted Fermi fuctions clearly demonstrate the shift of the peak with temperature (see the inset of Fig. 3(f)). We note that this shift of the ‘M-shaped’ band is significantly different from the change of EDCs in the 34-eV data. Therefore, the data strongly suggest that the evolution of electronic states at the hidden-order transition is kz dependent.
Discussion
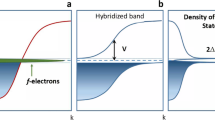
The major findings in the present study are summarized in Figure 4(a) and 4(b), from which we can learn about the nature of the hidden-order transition. In the paramagnetic state illustrated in Fig. 4(a), a renormalized electron-like Fermi surface exists around the Z point in the body-centered tetragonal Brillouin zone. We note that because no electron pocket is predicted at the Z point by local-density approximation (LDA), LDA may be insufficient to describe some of the electronic states near the Fermi level. The electron-like Fermi surface is gapped out upon the transition and it evolves into a narrow ‘M-shaped’ band, as illustrated in Fig. 4(b). This observation can be attributed to the observed decrease in the number of carriers28 and the development of a gap29. The observed gap is very peculiar because the top of the band after gapping (ktop ~ 0.16 Å−1) is not aligned with the original Fermi vector (k110 ~ 0.30 Å−1); this misalignment behaviour suggests that the gap is particlehole asymmetric. We note that similar misalignment is observed by ARPES for the case of a pseudogap in high-Tc cuprates and it evidences the opening of a particle-hole asymmetric gap30. The energy scale of the gap in URu2Si2 is of the order of ~2–7 meV (here, the lower limit is obtained from the leading-edge shift and the upper limit from the peak position), which is in accordance with optical spectroscopy and specific heat3,29,31. Moreover, the in-plane wave-vector position of the observed gap (kF ~ 0.30 Å−1) is in agreement with ultrafast time- and angle-resolved photoemission spectroscopy32.
Schematic description of electronic states observed (a) in the paramagnetic phase and (b) in the hidden-order phase.
Red lines represent bulk-originated states and blue lines indicate kz-independent states that are irrelevant to the hidden-order transition. The electron pocket at the Z point becomes gapped upon the transition and evolves into an ‘M-shaped’ band. A similar narrow band appears at the Γ point only in the hidden-order state, indicating the emergence of spontaneous translational symmetry breaking.
The particle-asymmetric gap in the heavy electron pocket may be explained by the development of hybridization with a conduction band at THO; however, we have the following remarks: (1) Our experimental data do not support the relevance of the hole pocket at k110 ~ 0.16 Å−1 to the bulk hybridization process at the transition. First of all, the hole pocket is suggested to be a surface-derived state, thus it should be irrelevant to the transition. In addition, if the light hole pocket at k110 = 0.16 Å−1 hybridizes with the heavy electron pocket and forms a gap below the Fermi level, two split peaks would show up in the EDCs of 19-eV data at k110 = 0.16 Å−1 (Fig. 3(f)); however, we did not find any evidence for such split features below the Fermi level. Moreover, the wave vector of the hole pocket is smaller than the value deduced in previous spectroscopic studies18,19,33, further supporting the irrelevance. (2) Our data support that the energy gap is formed at the Fermi level upon the transition and the ‘M-shaped’ band does not cross the Fermi level below THO. This picture is consistent with the previous STS studies18,19, but is in contrast to the opening of a gap in momentum suggested by a recent ARPES study25. (3) Recall that a well-defined heavy fermion state which already exists above THO evolves into the ‘M-shaped’ band below THO around the Z point while no coherent state is observed around the Γ point above THO; moreover, our data indicate that the temperature evolution of ARPES spectra is significantly kz dependent. (Note that the kz-dependent temperature evolution cannot be explained by the mere intensity modulation due to the photoelectron matrix-element effect.) These observations cannot be explained only by the onset of hybridization between an incoherent (not well-defined) heavy fermion state and a conduction band at the transition temperature24. Therefore, although we do not exclude the role of ‘spontaneous hybridization’, we emphasize that the hybridization process between an incoherent state and a conduction band should not be the only effect at the transition. (4) There is no conclusive evidence for any bulk hole-like band being involved in the hybridization. One possible reconciliation is that another hole pocket remains undetected in ultrahigh-resolution ARPES owing to the matrix-element effect; another interesting possibility is that the ‘M-shaped’ band is formed by a mechanism unique to a two-channel Kondo lattice33; this model does not necessarily require the existence of a hole pocket in the disordered state.
The 19-eV data show that the ‘M-shaped’ band appears only in the ordered state around the Γ point. Although we observed the shift of ‘M-shaped’ band in the 19-eV data, its shape matches the dispersion observed in the 34-eV data when the order is well developed (say, at ~2 K); this observation strongly suggests that two high-symmetry points (Γ point and Z point) become equivalent in the ordered state and the lattice's translational symmetry should be spontaneously broken along the c-axis with the ordering vector of (0, 0, 1). This picture is further supported by the existence of a well-defined heavy fermion state only around the Z point in the paramagnetic state; the electronic states at the Γ and the Z points are qualitatively different above THO. The breaking of the translational symmetry along the c-axis is consistent with recent neutron data20, the reentrant behaviour of the hidden order34 and the similarity of the Fermi surfaces between the hidden-order state and the antiferromagnetic state21. Furthermore, as the present data show only electrons near the Fermi level change upon the transition, they may explain why x-ray scattering could not detect translational symmetry breaking.
The present experimental results would impose strong constraints on the models of the hidden order. Our results may be attributable to an antiferro-type multipole ordering along the c-axis6,7,8,9,10,16; they are also in accordance with exotic Fermi-surface nesting instabilities, in which the nesting vector of (0, 0, 1) plays a role11,12,15. However, we also note that an explanation beyond simple textbook-type band folding is necessary, as also pointed out recently22,25; future theoretical studies should address why the energy shift of the ‘M-shaped’ band around the Γ point was observed. We expect that this study will promote a better understanding of complicated electronic states in URu2Si2 and also provide a framework for future studies on exotic phase-transition phenomena in correlated materials.
Methods
Preparation of URu2Si2 samples
The stoichiometric proportions of the starting ingredients (depleted uranium: 99.9%, ruthenium: 99.99% and silicon: 99.999%) were melted in a tetra-arc furnace and single crystals of URu2Si2 were synthesized by the Czochralski method. The single-crystal ingot was cut using a spark cutter. The samples were annealed at 1075°C for 5 days under ultrahigh vacuum. The details are described in Ref. 35. The high quality of the samples can be evidenced by quantum oscillation measurements21.
Ultrahigh-resolution three-dimensional ARPES
Ultrahigh-resolution three-dimensional ARPES was performed at a ‘One-Cubed’ ARPES endstation located at BESSY II (Berliner Elektronenspeicherring Gesellschaft fur Syn-chrotronstrahlung). The spectrometer consists of a Gammadata VG-Scienta R4000 electron analyser and a continuous flow He-3 cryomanipulator. Photons from the UE112_PGM-2b beamline were used and the total energy resolution was 4–7 meV for 19–51 eV. The polarization of the incident photon was set to linear vertical unless otherwise noted. The analyser's slit was aligned along the [110] direction, which corresponds to the Γ-X direction in the body-centered tetragonal Brillouin zone of URu2Si2. Samples were cleaved in-situ at room temperature in ultrahigh vacuum. Temperature-dependent measurements were performed in the range of 2–22 K in a cyclic manner.
References
Palstra, T. T. M. et al. Superconducting and Magnetic Transitions in the Heavy-Fermion System URu2Si2 . Phys. Rev. Lett. 55, 2727–2730 (1985).
Schlabitz, W. et al. Superconductivity and magnetic order in a strongly interacting fermi-system: URu2Si2 . Z. Phys. B Con. Mat. 62, 171–177 (1986).
Maple, M. B. et al. Partially gapped Fermi surface in the heavy-electron superconductor URu2Si2 . Phys. Rev. Lett. 56, 185–188 (1986).
Broholm, C. et al. Magnetic excitations and ordering in the heavy-electron superconductor URu2Si2 . Phys. Rev. Lett. 58, 1467–1470 (1987).
Amitsuka, H. et al. Pressure–temperature phase diagram of the heavy-electron superconductor URu2Si2 . J Magn. Magn. Mater. 310, 214–220 (2007).
Haule, K. & Kotliar, G. Arrested Kondo effect and hidden order in URu2Si2 . Nat. Phys. 5, 796–799 (2009).
Harima, H., Miyake, K. & Flouquet, J. Why the Hidden Order in URu2Si2 Is Still Hidden–One Simple Answer. J. Phys. Soc. Jpn. 79, 033705 (2010).
Cricchio, F., Bultmark, F., Grånäs, O. & Nordström, L. Itinerant Magnetic Multipole Moments of Rank Five as the Hidden Order in URu2Si2 . Phys. Rev. Lett. 103, 107202 (2009).
Thalmeier, P. & Takimoto, T. Signatures of hidden-order symmetry in torque oscillations, elastic constant anomalies and field-induced moments in URu2Si2 . Phys. Rev. B 83, 165110 (2011).
Kusunose, H. & Harima, H. On the Hidden Order in URu2Si2 – Antiferro Hexadecapole Order and Its Consequences. J. Phys. Soc. Jpn. 80, 084702 (2011).
Elgazzar, S., Rusz, J., Amft, M., Oppeneer, P. M. & Mydosh, J. A. Hidden order in URu2Si2 originates from Fermi surface gapping induced by dynamic symmetry breaking. Nat. Mater. 8, 337–341 (2009).
Oppeneer, P. et al. Electronic structure theory of the hidden-order material URu2Si2 . Phys. Rev. B 82, 205103 (2010).
Dubi, Y. & Balatsky, A. Hybridization Wave as the “Hidden Order” in URu2Si2 . Phys. Rev. Lett. 106, 086401 (2011).
Pépin, C., Norman, M., Burdin, S. & Ferraz, A. Modulated Spin Liquid: A New Paradigm for URu2Si2 . Phys. Rev. Lett. 106, 106601 (2011).
Fujimoto, S. Spin Nematic State as a Candidate of the Hidden Order Phase of URu2Si2 . Phys. Rev. Lett. 106, 196407 (2011).
Ikeda, H. et al. Emergent rank-5 nematic order in URu2Si2 . Nat. Phys. 8, 528–533 (2012).
Okazaki, R. et al. Rotational Symmetry Breaking in the Hidden-Order Phase of URu2Si2 . Science 331, 439–442 (2011).
Schmidt, A. R. et al. Imaging the Fano lattice to ‘hidden order’ transition in URu2Si2 . Nature 465, 570–576 (2010).
Aynajian, P. et al. Visualizing the formation of the Kondo lattice and the hidden order in URu2Si2 . P. Natl. Acad. Sci. 107, 10383–10388 (2010).
Villaume, A. et al. Signature of hidden order in heavy fermion superconductor URu2Si2: Resonance at the wave vector Q0 = (1,0,0). Phys. Rev. B 78, 012504 (2008).
Hassinger, E. et al. Similarity of the Fermi Surface in the Hidden Order State and in the Antiferromagnetic State of URu2Si2 . Phys. Rev. Lett. 105, 216409 (2010).
Santander-Syro, A. F. et al. Fermi-surface instability at the ‘hidden-order’ transition of URu2Si2 . Nat. Phys. 5, 637–641 (2009).
Yoshida, R. et al. Signature of hidden order and evidence for periodicity modification in URu2Si2 . Phys. Rev. B 82, 205108 (2010).
Chatterjee, S. et al. Formation of the Coherent Heavy Fermion Liquid at the Hidden Order Transition in URu2Si2 . Phys. Rev. Lett. 110, 186401 (2013).
Boariu, F. L. et al. Momentum-Resolved Evolution of the Kondo Lattice into “Hidden Order” in URu2Si2 . Phys. Rev. Lett. 110, 156404 (2013).
Denlinger, J. D. et al. Comparative study of the electronic structure of XRu2Si2: probing the Anderson lattice. J. Electron Spectrosc. 117, 347–369 (2001).
Boariu, F. L. et al. The surface state of URu2Si2 . J. Electron Spectrosc. 181, 82–87 (2010).
Schoenes, J., Schönenberger, C., Franse, J. & Menovsky, A. A. Hall-effect and resistivity study of the heavy-fermion system URu2Si2 . Phys. Rev. B 35, 5375–5378 (1987).
Bonn, D. A., Garrett, J. D. & Timusk, T. Far-Infrared Properties of URu2Si2 . Phys. Rev. Lett. 61, 1305–1308 (1988).
Hashimoto, M. et al. Particle–hole symmetry breaking in the pseudogap state of Bi2201. Nat. Phys. 6, 414–418 (2010).
Van Dijk, N. H. et al. Specific heat of heavy-fermion URu2Si2 in high magnetic fields. Phys. Rev. B 56, 14493 (1997).
Dakovski, G. L. et al. Anomalous femtosecond quasiparticle dynamics of hidden order state in URu2Si2 . Phys. Rev. B 84, 161103 (2011).
Hoshino, S., Otsuki, J. & Kuramoto, Y. Resolution of Entropy ln2 by Ordering in Two-Channel Kondo Lattice. J. Phys. Soc. Jpn. 82, 044707 (2013).
Aoki, D. et al. Field Reentrance of the Hidden Order State of URu2Si2 under Pressure. J. Phys. Soc. Jpn. 78, 053701 (2009).
Aoki, D. et al. Field re-entrant hidden-order phase under pressure in URu2Si2 . J. Phys.: Condens. Matter 22, 164205 (2010).
Acknowledgements
We thank T. Kim, K. Ott, A. Varykhalov and E. Rienks for assistance with the measurements. We also thank Y. Haga, Y. Onuki, K. Machida and S. Hoshino for the valuable discussions. The ARPES measurements were performed at the ‘One-Cubed’ ARPES station at BESSY II under proposal numbers 2011_2_110195 and 2012_2_120346. This work was supported by a Grant-in-Aid for Scientific Research on Innovative Areas ‘Heavy Electrons’ (20102003) from the Ministry of Education, Culture, Sports, Science and Technology, a Grant-in-Aid for Scientific Research from the Japan Society for the Promotion of Science (JSPS) and ERC starting grant (NewHeavyFermion). R.Y. also acknowledges the financial support from the Research Fellowships of JSPS for Young Scientists.
Author information
Authors and Affiliations
Contributions
The ARPES data were acquired and analyzed by R.Y. and T.Y. with support from K.T., T.I., M.S., J.S. and T.W. The high-quality single crystals were grown by D.A. and J.F. R.Y. and T.Y. designed the experiment and wrote the manuscript with the help of Y.M.
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Electronic supplementary material
Supplementary Information
Supplementary Information for Translational Symmetry Breaking and Gapping of Heavy-Quasiparticle Pocket in URu2Si2
Rights and permissions
This work is licensed under a Creative Commons Attribution-NonCommercial-ShareALike 3.0 Unported License. To view a copy of this license, visit http://creativecommons.org/licenses/by-nc-sa/3.0/
About this article
Cite this article
Yoshida, R., Tsubota, K., Ishiga, T. et al. Translational Symmetry Breaking and Gapping of Heavy-Quasiparticle Pocket in URu2Si2. Sci Rep 3, 2750 (2013). https://doi.org/10.1038/srep02750
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/srep02750
This article is cited by
-
Proximity to a critical point driven by electronic entropy in URu2Si2
npj Quantum Materials (2021)
-
Momentum-resolved hidden-order gap reveals symmetry breaking and origin of entropy loss in URu2Si2
Nature Communications (2014)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.