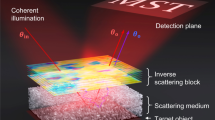
Abstract
Optical imaging has had a central role in elucidating the underlying biological and physiological mechanisms in living specimens owing to its high spatial resolution, molecular specificity and minimal invasiveness. However, its working depth for in vivo imaging is extremely shallow, and thus reactions occurring deep inside living specimens remain out of reach. This problem originates primarily from multiple light scattering caused by the inhomogeneity of tissue obscuring the desired image information. Adaptive optical microscopy, which minimizes the effect of sample-induced aberrations, has to date been the most effective approach to addressing this problem, but its performance has plateaued because it can suppress only lower-order perturbations. To achieve an imaging depth beyond this conventional limit, there is increasing interest in exploiting the physics governing multiple light scattering. New approaches have emerged based on the deterministic measurement and/or control of multiple-scattered waves, rather than their stochastic and statistical treatment. In this Review, we provide an overview of recent developments in this area, with a focus on approaches that achieve a microscopic spatial resolution while remaining useful for in vivo imaging, and discuss their present limitations and future prospects.
Key points
-
Optical microscopy is an indispensable tool in biology and medicine owing to its high spatial resolution, molecular specificity and minimal invasiveness, but it is limited to the interrogation of superficial layers for in vivo imaging.
-
The intensity of single-scattered waves used in conventional imaging decreases exponentially with depth; thus, the imaging depth limits are set by the detector dynamic range and the efficiency of the gating operations.
-
Approaches that make deterministic use of the abundant multiple-scattered (MS) waves have been proposed to enable deep optical imaging while maintaining the microscopic spatial resolving power.
-
Recording and controlling the wavefront of MS waves enables a complex scattering layer to be converted into a focusing lens, leading to the development of an ultrathin endoscope.
-
Acousto-optic interactions and wavefront sensing and/or control are integrated to exploit the large penetration depth of ultrasound and high spatial resolution of optical imaging.
-
Reflection-matrix approaches that record and process all MS waves enable the correction of sample-induced aberrations, exploitation of multiple scattering signals and suppression of multiple scattering noise, allowing for imaging at depths greater than those accessible with conventional confocal and adaptive optics microscopy.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 digital issues and online access to articles
$99.00 per year
only $8.25 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout







Similar content being viewed by others
References
Luker, G. D. & Luker, K. E. Optical imaging: current applications and future directions. J. Nucl. Med. 49, 1–4 (2008).
Wilt, B. A. et al. Advances in light microscopy for neuroscience. Annu. Rev. Neurosci. 32, 435–506 (2009).
Hadjipanayis, C. G., Jiang, H., Roberts, D. W. & Yang, L. Current and future clinical applications for optical imaging of cancer: from intraoperative surgical guidance to cancer screening. Semin. Oncol. 38, 109–118 (2011).
Betzig, E. et al. Imaging intracellular fluorescent proteins at nanometer resolution. Science 313, 1642–1645 (2006).
Rust, M. J., Bates, M. & Zhuang, X. Sub-diffraction-limit imaging by stochastic optical reconstruction microscopy (STORM). Nat. Methods 3, 793–796 (2006).
Klar, T. A., Jakobs, S., Dyba, M., Egner, A. & Hell, S. W. Fluorescence microscopy with diffraction resolution barrier broken by stimulated emission. Proc. Natl Acad. Sci. USA 97, 8206–8210 (2000).
Hell, S. W. Far-Field Optical nanoscopy. Science 316, 1153–1158 (2007).
Tyson, R. K. Principles of Adaptive Optics (CRC Press, 2015).
Liu, F., Yoo, K. M. & Alfano, R. R. Transmitted photon intensity through biological tissues within various time windows. Opt. Lett. 19, 740–742 (1994).
Kang, S. et al. Imaging deep within a scattering medium using collective accumulation of single-scattered waves. Nat. Photonics 9, 253–258 (2015). The first report of the measurement of a wide-field time-gated reflection matrix.
Badon, A., Boccara, A. C., Lerosey, G., Fink, M. & Aubry, A. Multiple scattering limit in optical microscopy. Opt. Express 25, 28914–28934 (2017).
Dunsby, C. & French, P. M. W. Techniques for depth-resolved imaging through turbid media including coherence-gated imaging. J. Phys. D. Appl. Phys. 36, R207–R227 (2003). An early study on the development of single-scattering gating methods for deep optical imaging.
Minsky, M. Microscopy apparatus. US Patent 3013467 (1961).
Huang, D. et al. Optical coherence tomography. Science. 254, 1178–1181 (1991).
Bouma, B. E. & Tearney, G. J. (eds) Handbook of Optical Coherence Tomography (Marcel Dekker, 2002).
Pawley, J. (ed.) Handbook of Biological Confocal Microscopy (Springer, 2010).
Yao, G. & Wang, L. V. Theoretical and experimental studies of ultrasound-modulated optical tomography in biological tissue. Appl. Opt. 39, 659–664 (2000).
Wang, L. V. Ultrasound-mediated biophotonic imaging: a review of acousto-optical tomography and photo-acoustic tomography. Dis. Markers 19, 123–138 (2004).
Izatt, J. A., Hee, M. R., Owen, G. M., Swanson, E. A. & Fujimoto, J. G. Optical coherence microscopy in scattering media. Opt. Lett. 19, 590–592 (1994).
Beaurepaire, E., Boccara, A. C., Lebec, M., Blanchot, L. & Saint-Jalmes, H. Full-field optical coherence microscopy. Opt. Lett. 23, 244–246 (1998).
Oh, W. Y. et al. Ultrahigh-resolution full-field optical coherence microscopy using InGaAs camera. Opt. Express 14, 726–735 (2006).
Denk, W., Strickler, J. H. & Webb, W. W. Two-photon laser scanning fluorescence microscopy. Science 248, 73–76 (1990).
Hell, S. W. et al. Three-photon excitation in fluorescence microscopy. J. Biomed. Opt. 1, 71–74 (1996).
Zipfel, W. R. et al. Live tissue intrinsic emission microscopy using multiphoton-excited native fluorescence and second harmonic generation. Proc. Natl Acad. Sci. USA 100, 7075–7080 (2003).
Barad, Y., Eisenberg, H., Horowitz, M. & Silberberg, Y. Nonlinear scanning laser microscopy by third harmonic generation. Appl. Phys. Lett. 70, 922–924 (1997).
Papagiakoumou, E. et al. Functional patterned multiphoton excitation deep inside scattering tissue. Nat. Photonics 7, 274–278 (2013).
Rowlands, C. J. et al. Wide-field three-photon excitation in biological samples. Light. Sci. Appl. 6, e16255 (2016).
Adrià, E.-M. et al. Wide-field multiphoton imaging through scattering media without correction. Sci. Adv. 4, eaau1338 (2018).
Booth, M. J. Adaptive optical microscopy: the ongoing quest for a perfect image. Light. Sci. Appl. 3, e165 (2014).
Jang, M., Ko, H., Lee, W. K., Lee, J.-S. & Choi, W. Coherent space-gated microscopy: a step towards deep-tissue phase imaging of biological cells. Preprint at arXiv https://arxiv.org/abs/1811.02755 (2018).
Durduran, T. et al. Diffuse optical measurement of blood flow, blood oxygenation, and metabolism in a human brain during sensorimotor cortex activation. Opt. Lett. 29, 1766–1768 (2004).
Ntziachristos, V., Yodh, A. G., Schnall, M. & Chance, B. Concurrent MRI and diffuse optical tomography of breast after indocyanine green enhancement. Proc. Natl Acad. Sci. USA 97, 2767–2772 (2000).
Wang, L. V. & Hu, S. Photoacoustic tomography: in vivo imaging from organelles to organs. Science 335, 1458–1462 (2012).
Vellekoop, I. M. & Mosk, A. P. Focusing coherent light through opaque strongly scattering media. Opt. Lett. 32, 2309–2311 (2007). The first demonstration of optical-wave focusing through a scattering layer.
Yaqoob, Z., Psaltis, D., Feld, M. S. & Yang, C. Optical phase conjugation for turbidity suppression in biological samples. Nat. Photonics 2, 110–115 (2008).
Dorokhov, O. N. On the coexistence of localized and extended electronic states in the metallic phase. Solid. State Commun. 51, 381–384 (1984).
Feng, S., Kane, C., Lee, P. A. & Stone, A. D. Correlations and fluctuations of coherent wave transmission through disordered media. Phys. Rev. Lett. 61, 834–837 (1988).
Freund, I., Rosenbluh, M. & Feng, S. Memory effects in propagation of optical waves through disordered media. Phys. Rev. Lett. 61, 2328–2331 (1988).
Beenakker, C. W. J. Random-matrix theory of quantum transport. Rev. Mod. Phys. 69, 731–808 (1997).
Mosk, A. P., Lagendijk, A., Lerosey, G. & Fink, M. Controlling waves in space and time for imaging and focusing in complex media. Nat. Photonics 6, 283–292 (2012).
Rotter, S. & Gigan, S. Light fields in complex media: mesoscopic scattering meets wave control. Rev. Mod. Phys. 89, 015005 (2017).
Fink, M. Time reversal of ultrasonic fields. I. Basic principles. IEEE Trans. Ultrason. Ferroelectr. Freq. Control. 39, 555–566 (1992).
Derode, A., Roux, P. & Fink, M. Robust acoustic time reversal with high-order multiple scattering. Phys. Rev. Lett. 75, 4206–4209 (1995).
Fink, M. Time reversed acoustics. Phys. Today 50, 34–40 (1997).
Lerosey, G. et al. Time reversal of electromagnetic waves. Phys. Rev. Lett. 92, 193904 (2004).
Lerosey, G., de Rosny, J., Tourin, A., Derode, A. & Fink, M. Time reversal of wideband microwaves. Appl. Phys. Lett. 88, 154101 (2006).
Vellekoop, I. M. & Mosk, A. P. Universal optimal transmission of light through disordered materials. Phys. Rev. Lett. 101, 120601 (2008).
Kim, M., Choi, W., Yoon, C., Kim, G. H. & Choi, W. Relation between transmission eigenchannels and single-channel optimizing modes in a disordered medium. Opt. Lett. 38, 2994–2996 (2013).
Katz, O., Small, E. & Silberberg, Y. Looking around corners and through thin turbid layers in real time with scattered incoherent light. Nat. Photonics 6, 549–553 (2012).
Judkewitz, B., Horstmeyer, R., Vellekoop, I. M., Papadopoulos, I. N. & Yang, C. Translation correlations in anisotropically scattering media. Nat. Phys. 11, 684–689 (2015).
Vellekoop, I. M., Lagendijk, A. & Mosk, A. P. Exploiting disorder for perfect focusing. Nat. Photonics 4, 320–322 (2010).
van Putten, E. G. et al. Scattering lens resolves sub-100 nm structures with visible light. Phys. Rev. Lett. 106, 193905 (2011).
Park, J.-H. et al. Subwavelength light focusing using random nanoparticles. Nat. Photonics 7, 454–458 (2013).
Choi, W. et al. Control of randomly scattered surface plasmon polaritons for multiple-input and multiple-output plasmonic switching devices. Nat. Commun. 8, 14636 (2017).
Gjonaj, B. et al. Focusing and scanning microscopy with propagating surface plasmons. Phys. Rev. Lett. 110, 266804 (2013).
Seo, E. et al. Far-field control of focusing plasmonic waves through disordered nanoholes. Opt. Lett. 39, 5838–5841 (2014).
Vellekoop, I. M. Feedback-based wavefront shaping. Opt. Express 23, 12189–12206 (2015).
Wang, D. et al. Focusing through dynamic tissue with millisecond digital optical phase conjugation. Optica 2, 728–735 (2015).
Conkey, D. B., Caravaca-Aguirre, M. A. & Piestun, R. High-speed scattering medium characterization with application to focusing light through turbid media. Opt. Express 20, 1733–1740 (2012).
Blochet, B., Bourdieu, L. & Gigan, S. Focusing light through dynamical samples using fast continuous wavefront optimization. Opt. Lett. 42, 4994–4997 (2017).
Liu, Y., Ma, C., Shen, Y., Shi, J. & Wang, L. V. Focusing light inside dynamic scattering media with millisecond digital optical phase conjugation. Optica 4, 280–288 (2017).
Feldkhun, D., Tzang, O., Wagner, K. H. & Piestun, R. Focusing and scanning through scattering media in microseconds. Optica 6, 72–75 (2019).
Ji, N., Milkie, D. E. & Betzig, E. Adaptive optics via pupil segmentation for high-resolution imaging in biological tissues. Nat. Methods 7, 141–147 (2009).
Park, J.-H., Sun, W. & Cui, M. High-resolution in vivo imaging of mouse brain through the intact skull. Proc. Natl Acad. Sci. USA 112, 9236–9241 (2015).
Frostig, H. et al. Focusing light by wavefront shaping through disorder and nonlinearity. Optica 4, 1073–1079 (2017).
Ruan, H. et al. Focusing light inside scattering media with magnetic-particle-guided wavefront shaping. Optica 4, 1337–1343 (2017).
Florentin, R. et al. Shaping the light amplified in a multimode fiber. Light Sci. Appl. 6, e16208 (2017).
Shen, Y., Liu, Y., Ma, C. & Wang, L. V. Focusing light through biological tissue and tissue-mimicking phantoms up to 9.6 cm in thickness with digital optical phase conjugation. J. Biomed. Opt. 21, 085001 (2016).
Popoff, S., Lerosey, G., Fink, M., Boccara, A. C. & Gigan, S. Image transmission through an opaque material. Nat. Commun. 1, 81 (2010).
Popoff, S. M. et al. Measuring the transmission matrix in optics: an approach to the study and control of light propagation in disordered media. Phys. Rev. Lett. 104, 100601 (2010). The first report of the measurement of a transmission matrix in optics.
Choi, Y. et al. Overcoming the diffraction limit using multiple light scattering in a highly disordered medium. Phys. Rev. Lett. 107, 023902 (2011).
Choi, Y. et al. Synthetic aperture microscopy for high resolution imaging through a turbid medium. Opt. Lett. 36, 4263–4265 (2011).
van Putten, E. & Mosk, A. The information age in optics: measuring the transmission matrix. Physics 3, 22 (2010).
Choi, Y. et al. Scanner-free and wide-field endoscopic imaging by using a single multimode optical fiber. Phys. Rev. Lett. 109, 203901 (2012).
Choi, Y., Yoon, C., Kim, M., Choi, W. & Choi, W. Optical imaging with the use of a scattering lens. IEEE J. Sel. Top. Quantum Electron. 20, 6800213 (2014).
Yoon, C. et al. Removal of back-reflection noise at ultrathin imaging probes by the single-core illumination and wide-field detection. Sci. Rep. 7, 6524 (2017).
Bianchi, S. & Di Leonardo, R. A multi-mode fiber probe for holographic micromanipulation and microscopy. Lab. Chip 12, 635–639 (2012). The first report of imaging through a multimode fibre.
Bianchi, S. et al. Focusing and imaging with increased numerical apertures through multimode fibers with micro-fabricated optics. Opt. Lett. 38, 4935–4938 (2013).
Čižmár, T. & Dholakia, K. Exploiting multimode waveguides for pure fibre-based imaging. Nat. Commun. 3, 1027 (2012).
Čižmár, T. & Dholakia, K. Shaping the light transmission through a multimode optical fibre: complex transformation analysis and applications in biophotonics. Opt. Express 19, 18871–18884 (2011).
Kim, D. et al. Toward a miniature endomicroscope: pixelation-free and diffraction-limited imaging through a fiber bundle. Opt. Lett. 39, 1921–1924 (2014).
Ohayon, S., Caravaca-Aguirre, A., Piestun, R. & DiCarlo, J. J. Minimally invasive multimode optical fiber microendoscope for deep brain fluorescence imaging. Biomed. Opt. Express 9, 1492–1509 (2018).
Caravaca-Aguirre, A. M. & Piestun, R. Single multimode fiber endoscope. Opt. Express 25, 1656–1665 (2017).
Turtaev, S. et al. High-fidelity multimode fibre-based endoscopy for deep brain in vivo imaging. Light. Sci. Appl. 7, 92 (2018).
Loterie, D. et al. Digital confocal microscopy through a multimode fiber. Opt. Express 23, 23845–23858 (2015).
Morales-Delgado, E. E., Psaltis, D. & Moser, C. Two-photon imaging through a multimode fiber. Opt. Express 23, 32158–32170 (2015).
Drémeau, A. et al. Reference-less measurement of the transmission matrix of a highly scattering material using a DMD and phase retrieval techniques. Opt. Express 23, 11898–11911 (2015).
del Hougne, P., Rajaei, B., Daudet, L. & Lerosey, G. Intensity-only measurement of partially uncontrollable transmission matrix: demonstration with wave-field shaping in a microwave cavity. Opt. Express 24, 18631–18641 (2016).
Porat, A. et al. Widefield lensless imaging through a fiber bundle via speckle correlations. Opt. Express 24, 16835–16855 (2016).
Farahi, S., Ziegler, D., Papadopoulos, I. N., Psaltis, D. & Moser, C. Dynamic bending compensation while focusing through a multimode fiber. Opt. Express 21, 22504–22514 (2013).
Loterie, D., Psaltis, D. & Moser, C. Bend translation in multimode fiber imaging. Opt. Express 25, 6263–6273 (2017).
Plöschner, M., Tyc, T. & Čižmár, T. Seeing through chaos in multimode fibres. Nat. Photonics 9, 529–535 (2015).
Boonzajer Flaes, D. E. et al. Robustness of light-transport processes to bending deformations in graded-index multimode waveguides. Phys. Rev. Lett. 120, 233901 (2018).
Freund, I. Looking through walls and around corners. Phys. A 168, 49–65 (1990).
Akkermans, E. & Montambaux, G. Mesoscopic Physics of Electrons and Photons (Cambridge Univ. Press, 2007).
Osnabrugge, G., Horstmeyer, R., Papadopoulos, I. N., Judkewitz, B. & Vellekoop, I. M. The generalized optical memory effect. Optica 4, 886–892 (2017).
Bertolotti, J. et al. Non-invasive imaging through opaque scattering layers. Nature 491, 232–234 (2012). The first study to use the optical memory effect for epi-fluorescence imaging.
Katz, O., Heidmann, P., Fink, M. & Gigan, S. Non-invasive single-shot imaging through scattering layers and around corners via speckle correlations. Nat. Photonics 8, 784–790 (2014).
Yang, X., Pu, Y. & Psaltis, D. Imaging blood cells through scattering biological tissue using speckle scanning microscopy. Opt. Express 22, 3405–3413 (2014).
Schott, S., Bertolotti, J., Léger, J.-F., Bourdieu, L. & Gigan, S. Characterization of the angular memory effect of scattered light in biological tissues. Opt. Express 23, 13505–13516 (2015).
Amitonova, L. V., Mosk, A. P. & Pinkse, P. W. H. Rotational memory effect of a multimode fiber. Opt. Express 23, 20569–20575 (2015).
Stasio, N., Moser, C. & Psaltis, D. Calibration-free imaging through a multicore fiber using speckle scanning microscopy. Opt. Lett. 41, 3078–3081 (2016).
Fienup, J. R. Phase retrieval algorithms: a comparison. Appl. Opt. 21, 2758–2769 (1982).
Fienup, J. R. Reconstruction of an object from the modulus of its Fourier transform. Opt. Lett. 3, 27–29 (2008).
Papadopoulos, I. N., Jouhanneau, J. S., Poulet, J. F. A. & Judkewitz, B. Scattering compensation by focus scanning holographic aberration probing (F-SHARP). Nat. Photonics 11, 116–123 (2017).
Feng, G. et al. Imaging neuronal subsets in transgenic mice expressing multiple spectral variants of GFP. Neuron 28, 41–51 (2000).
Szabo, T. L. Diagnostic Ultrasound Imaging: Inside Out (Elsevier, 2004).
Elson, D. S., Li, R., Dunsby, C., Eckersley, R. & Tang, M. X. Ultrasound-mediated optical tomography: a review of current methods. Interface Focus. 1, 632–648 (2011).
Yao, G. & Wang, L. V. in Biomedical Optical Spectroscopy and Diagnostics (Optical Society of America, 2000).
Wang, L. V. Prospects of photoacoustic tomography. Med. Phys. 35, 5758–5767 (2008).
Wang, L. V. (ed.) Photoacoustic Imaging and Spectroscopy (CRC press, 2017).
Culjat, M. O., Goldenberg, D., Tewari, P. & Singh, R. S. A review of tissue substitutes for ultrasound imaging. Ultrasound Med. Biol. 36, 861–873 (2010).
Horstmeyer, R., Ruan, H. & Yang, C. Guidestar-assisted wavefront-shaping methods for focusing light into biological tissue. Nat. Photonics 9, 563–571 (2015).
Jang, M., Sentenac, A. & Yang, C. Optical phase conjugation (OPC)-assisted isotropic focusing. Opt. Express 21, 8781–8792 (2013).
Xu, X., Liu, H. & Wang, L. V. Time-reversed ultrasonically encoded optical focusing into scattering media. Nat. Photonics 5, 154–157 (2011). The first study to combine acousto-optic modulation and optical phase conjugation for optical imaging in a scattering medium.
Wang, Y. M., Judkewitz, B., Dimarzio, C. A. & Yang, C. Deep-tissue focal fluorescence imaging with digitally time-reversed ultrasound-encoded light. Nat. Commun. 3, 928 (2012).
Si, K., Fiolka, R. & Cui, M. Fluorescence imaging beyond the ballistic regime by ultrasound-pulse-guided digital phase conjugation. Nat. Photonics 6, 657–661 (2012).
Si, K., Fiolka, R. & Cui, M. Breaking the spatial resolution barrier via iterative sound-light interaction in deep tissue microscopy. Sci. Rep. 2, 748 (2012).
Ruan, H., Jang, M., Judkewitz, B. & Yang, C. Iterative time-reversed ultrasonically encoded light focusing in backscattering mode. Sci. Rep. 4, 7156 (2014).
Judkewitz, B., Wang, Y. M., Horstmeyer, R., Mathy, A. & Yang, C. Speckle-scale focusing in the diffusive regime with time reversal of variance-encoded light (TROVE). Nat. Photonics 7, 300–305 (2013).
Katz, O., Ramaz, F., Gigan, S. & Fink, M. Controlling light in complex media beyond the acoustic diffraction-limit using the acousto-optic transmission matrix. Nat. Commun. 10, 717 (2019).
Izatt, J. A., Swanson, E. A., Fujimoto, J. G., Hee, M. R. & Owen, G. M. Optical coherence microscopy in scattering media. Opt. Lett. 19, 590–592 (2008).
Chaigne, T. et al. Controlling light in scattering media non-invasively using the photoacoustic transmission matrix. Nat. Photonics 8, 58–64 (2014).
Lai, P., Wang, L., Tay, J. W. & Wang, L. V. Photoacoustically guided wavefront shaping for enhanced optical focusing in scattering media. Nat. Photonics 9, 126–132 (2015).
Conkey, D. B. et al. Super-resolution photoacoustic imaging through a scattering wall. Nat. Commun. 6, 7902 (2015).
Kang, S. et al. High-resolution adaptive optical imaging within thick scattering media using closed-loop accumulation of single scattering. Nat. Commun. 8, 2157 (2017). A report of a reflection matrix analysis algorithm to correct aberrations without using guide stars.
Kim, M. et al. Label-free neuroimaging in vivo using synchronous angular scanning microscopy with single-scattering accumulation algorithm. Nat. Commun. 10, 3152 (2019).
Colomb, T. et al. Numerical parametric lens for shifting, magnification, and complete aberration compensation in digital holographic microscopy. J. Opt. Soc. Am. A 23, 3177–3190 (2006).
Boppart, S. A. et al. Computational adaptive optics for broadband optical interferometric tomography of biological tissue. Proc. Natl Acad. Sci. USA 109, 933505 (2012).
Shemonski, N. D. et al. Computational high-resolution optical imaging of the living human retina. Nat. Photonics 9, 440–443 (2015).
Zheng, G., Ou, X., Horstmeyer, R. & Yang, C. Characterization of spatially varying aberrations for wide field-of-view microscopy. Opt. Express 21, 15131–15143 (2013).
Pozzi, P. et al. Anisoplanatic adaptive optics in parallelized laser scanning microscopy. Preprint at arXiv https://arxiv.org/abs/1809.07529 (2018).
Kim, M. et al. Maximal energy transport through disordered media with the implementation of transmission eigenchannels. Nat. Photonics 6, 581–585 (2012).
Prada, C. & Fink, M. Eigenmodes of the time reversal operator: a solution to selective focusing in multiple-target media. Wave Motion 20, 151–163 (1994).
Aubry, A. & Derode, A. Random matrix theory applied to acoustic backscattering and imaging in complex media. Phys. Rev. Lett. 102, 084301 (2009).
Popoff, S. M. et al. Exploiting the time-reversal operator for adaptive optics, selective focusing, and scattering pattern analysis. Phys. Rev. Lett. 107, 263901 (2011).
Choi, Y. et al. Measurement of the time-resolved reflection matrix for enhancing light energy delivery into a scattering medium. Phys. Rev. Lett. 111, 243901 (2013).
Jeong, S. et al. Focusing of light energy inside a scattering medium by controlling the time-gated multiple light scattering. Nat. Photonics 12, 277–283 (2018).
Badon, A. et al. Smart optical coherence tomography for ultra-deep imaging through highly scattering media. Sci. Adv. 2, e1600370 (2016). A study analysing the time-gated reflection eigenchannels for deep optical imaging.
Kadobianskyi, M., Papadopoulos, I. N., Chaigne, T., Horstmeyer, R. & Judkewitz, B. Scattering correlations of time-gated light. Optica 5, 389–394 (2018).
Osnabrugge, G., Amitonova, L. V. & Vellekoop, I. M. Blind focusing through strongly scattering media using wavefront shaping with nonlinear feedback. Opt. Express 27, 11673–11688 (2019).
McCann, M. T., Jin, K. H. & Unser, M. Convolutional neural networks for inverse problems in imaging: a review. IEEE Signal. Process. Mag. 34, 85–95 (2017).
Belthangady, C. & Royer, L. A. Applications, promises, and pitfalls of deep learning for fluorescence image reconstruction. Nat. Methods 16, 1215–1225 (2019).
Barbastathis, G., Ozcan, A. & Situ, G. On the use of deep learning for computational imaging. Optica 6, 921–943 (2019).
Turpin, A., Vishniakou, I. & Seelig, J.d. Light scattering control in transmission and reflection with neural networks. Opt. Express 26, 30911–30929 (2018).
Rahmani, B., Loterie, D., Konstantinou, G., Psaltis, D. & Moser, C. Multimode optical fiber transmission with a deep learning network. Light. Sci. Appl. 7, 69 (2018).
Kamilov, U. S. et al. Learning approach to optical tomography. Optica 2, 517–522 (2015).
Sun, Y., Xia, Z. & Kamilov, U. S. Efficient and accurate inversion of multiple scattering with deep learning. Opt. Express 26, 14678–14688 (2018).
Ntziachristos, V. Going deeper than microscopy: the optical imaging. Nat. Methods 7, 603–614 (2010).
Acknowledgements
This research was supported by the Institute for Basic Science (grant no. IBS-R023-D1), the National Research Foundation of Korea (grant nos. NRF-2019R1C1C1008175 and NRF-2016R1A6A3A11936389), and the Catholic Medical Center Research Foundation in the programme year of 2018.
Author information
Authors and Affiliations
Contributions
The authors contributed equally to all aspects of the article.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Glossary
- Isoplanatic patch
-
The area over which the wavefront error remains almost the same.
- Guide stars
-
Bright, point-like light sources or scatterers that provide a wavefront reference for measuring and correcting wavefront distortions in adaptive optics systems.
- Point optimization
-
A method or algorithm that optimizes the point spread function by minimizing wavefront errors in adaptive optics systems.
- Spatial light modulator
-
A device that modulates the amplitude, phase or polarization of light waves in space.
- Optical memory effect
-
The phenomenon that speckle patterns of scattered light through thin and diffusive media are invariant to small tilts or shifts in an incident wavefront of light.
- Digital micromirror devices
-
Micromirrors used for high-speed, efficient and reliable spatial-light modulation; originally invented to create video displays in digital projectors.
- Epi-detection geometry
-
An imaging configuration in which an objective is used for both illumination and detection.
Rights and permissions
About this article
Cite this article
Yoon, S., Kim, M., Jang, M. et al. Deep optical imaging within complex scattering media. Nat Rev Phys 2, 141–158 (2020). https://doi.org/10.1038/s42254-019-0143-2
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s42254-019-0143-2
This article is cited by
-
Current and future applications of light-sheet imaging for identifying molecular and developmental processes in autism spectrum disorders
Molecular Psychiatry (2024)
-
Determining the optimal communication channels of arbitrary optical systems using integrated photonic processors
Nature Photonics (2024)
-
Active mode single-pixel imaging through strong scattering media via least squares conditional generative adversarial networks under low sampling rates
Journal of Optics (2024)
-
Highly crystallization-induced emissive luminophores with mechanoluminescent features for two-photon harvesting fluorescence imaging and latent fingerprint identification
Nano Research (2024)
-
Enhanced light focusing inside scattering media with shaped ultrasound
Scientific Reports (2023)