Introduction

Entanglement between spatially separated nodes is a unique resource for an array of quantum information applications including secure quantum communication1,2,3, distributed quantum computation4, and enhanced metrology5,6,7. Such capabilities fundamentally depend on the quality of the entanglement between distant nodes. Therefore, it is a key task to generate and manipulate the remote entanglement with high fidelity in the presence of various errors and imperfections. To address this problem, various entanglement purification (or distillation) protocols have been developed8,9,10,11. Entanglement purification has been successfully demonstrated based on entangled photon pairs in a series of experiments12,13,14. However, the purified entangled photon pairs are unavoidably lost upon the success of the purification procedures due to the lack of quantum memories, preventing them from being further exploited. Non-destructive entanglement purification is carried out using single N-V centers15 and single atoms16, in which the purified entangled states are stored for further use, such as conducting the entanglement purification procedure of the next round or the entanglement swapping procedure between neighboring nodes.

The atomic ensembles are promising candidates for quantum repeaters and quantum networks17, thanks to the collective enhancement between many atoms which results into high collection efficiency for signal photons18. Remarkable progresses have been made on the realization of quantum repeaters and quantum communication on the basis of the atomic ensembles17,18,19,20,21,22. However, there has been no demonstration of entanglement purification of discrete variables on atomic ensembles so far, since the purification protocols usually require the complex combination of high-fidelity quantum gates, long-term quantum storage, high-efficiency detection, or non-demolition qubit readout15,23,24, which are still challenging to be implemented on atomic ensembles.

Here, we propose the application of the filtering protocol25,26 using a single copy of two-qubits entangled state in atomic ensembles to gain increased fidelity. The present proposal is based on local operations (LOs) and detection on the spin states of the atomic ensembles in each node. We further illustrate that the entanglement connection (EC) process can be improved by combining these LOs and detection. As a proof-of-principle demonstration, we implement these elementary LOs on the hybrid electron-nuclear spins in a 143Nd3+:Y2SiO5 crystal, which is a promising material for quantum repeater nodes27,28,29,30. The combination of these high-quality LOs generates an entangled state between the electron spins and nuclear spins with a concurrence of 0.75 and a fidelity of 92.8% compared with the Bell state. These results provide useful tools toward the construction of large-scale quantum networks based on the solid-state atomic ensembles.

Results

Various protocols have been proposed for the construction of quantum repeaters based on atomic ensembles17. Here, we design our ED proposal based on the Duan–Lukin–Cirac–Zoller (DLCZ) scheme18, which is a well-known long-distance quantum communication scheme, proposed by L.-M. Duan, M. D. Lukin, J. I. Cirac & P. Zoller in 2001. It is a typical single-photon hierarchical scheme17, which is robust against the low efficiency in transmission18. The DLCZ scheme has been successfully demonstrated in diverse physical systems including cold atoms20,31,32,33,34,35, hot atoms36,37, micro-mechanical oscillators38,39, and diamonds40. Recently, non-classical correlations were observed in the rare-earth-ion-doped crystal41,42,43, which is a promising solid-state platform for the quantum repeater node due to its unique capability of long-lived optical storage44,45.

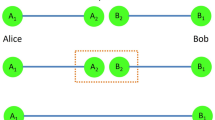
Here we provide a concise review on the original DLCZ protocol for introducing the following discussions on the entanglement distillation and connection proposal. The DLCZ scheme18 begins with the entanglement generation (EG) process. Suppose an atomic ensemble possesses two ground states (\(|g\rangle\) and \(\left|s\right\rangle\)) and an excited state (\(\left|e\right\rangle\)), and all atoms are initialized to the ground state \(|g\rangle\) at the beginning. The ensemble can be excited by a weak and off-resonant laser pulse, inducing Raman transitions to the state \(\left|s\right\rangle\), revealing scattered Stokes photons (Fig. 1a). Considering the relatively small excitation rate pc, the state of the atomic ensemble and the Stokes photon can be written as17,18,46:

$$\left|\phi \right\rangle ={\left|0\right\rangle }_{p}{\left|0\right\rangle }_{a}+\sqrt{{p}_{c}}{\left|1\right\rangle }_{p}{\left|1\right\rangle }_{a}.$$
(1)

Here \({\left|0\right\rangle }_{a}=\frac{1}{\sqrt{{N}_{A}}}\mathop{\sum }\nolimits_{i=1}^{{N}_{A}}{|{g}_{1}{g}_{2}\cdots {g}_{i}\cdots {g}_{{N}_{A}}\rangle }_{a}\), denotes the ensemble is in the ground state. \({\left|1\right\rangle }_{a}=\frac{1}{\sqrt{{N}_{A}}}\mathop{\sum }\nolimits_{i=1}^{{N}_{A}}{|{g}_{1}{g}_{2}\cdots {s}_{i}\cdots {g}_{{N}_{A}}\rangle }_{a}\), denotes the ensemble is charged with a collective excitation. \({\left|0\right\rangle }_{p}\) and \({\left|1\right\rangle }_{p}\) correspond to the photonic vacuum and single-photon state, respectively. Considering two atomic ensembles (named node A and node B) separated by a distance of L0, the stokes photons emitted by the two ensembles are transmitted and interfere on a beam splitter (BS) at the central station between these two nodes. Successful detection of a single Stokes photon indicates that node A and B are entangled (Fig. 1c), whose state is represented as:

$${\left|\psi \right\rangle }_{AB}^{}=\frac{1}{\sqrt{2}}({\left|0\right\rangle }_{A}{\left|1\right\rangle }_{B}+{e}^{i\theta }{\left|1\right\rangle }_{A}{\left|0\right\rangle }_{B}),$$
(2)

where θ denotes the relative phase.

Fig. 1: Processes of the Duan–Lukin–Cirac–Zoller scheme.
figure 1

a Write process. An off-resonant laser pulse with detuning of δ to \(\left|g\right\rangle \to \left|e\right\rangle\) transition induces a Stokes (S) photon with a small probability. b Read process. A resonant laser pulse drives single excitation from \(\left|s\right\rangle\) to \(\left|e\right\rangle\), inducing an anti-Stokes (AS) photon correlated with the Stoke photon. c Entanglement generation process. The stokes photons revealed by ensemble A and B interfere on a beam splitter (BS). d The first level entanglement connection. e Extending the communication length by utilizing entanglement connection. The single-photon detector D1 and D2 are used to detect the Stokes photon in the entanglement generation process, while D3 and D4 are utilized to detect the anti-Stokes photon in the entanglement connection process. L0 denotes the distance between two neighboring nodes.

Full size image

The next step is the EC process. Two pairs of entangled states are established between node A, B and node C, D using the protocol described above. Node A(C) and node B(D) are separated by L0, while node B and C are close to each other. The probabilistically stored atomic excitation in node B and C are converted back into anti-Stokes photons by a strong and resonant pulse (Fig. 1b). The induced anti-Stokes photons are combined on a BS between node B and C. Detecting a single anti-Stokes photon suggests the success of the EC process, i.e., node A and D are entangled with each other (Fig. 1d). Their state is written as:

$${\left|\psi \right\rangle }_{AD}^{}=\frac{1}{\sqrt{2}}({\left|0\right\rangle }_{A}{\left|1\right\rangle }_{D}+{e}^{i\theta ^{\prime} }{\left|1\right\rangle }_{A}{\left|0\right\rangle }_{D}).$$
(3)

Therefore, the communication length doubles. Once the EC process has been conducted successfully with n levels, the total communication length will be extended to 2nL0 (as shown in Fig. 1e).

Entanglement distillation proposal

Taking into account the photon loss in the channel as well as the noise of the single-photon detectors in practice, the state in Eq. (2) is modified into a density matrix:

$${\rho }_{AB}= \frac{1}{1+{p}_{2}+{p}_{0}}({\left|\psi \right\rangle }^{+}\left\langle \psi \right|+{p}_{2}{\left|11\right\rangle }_{AB}\left\langle 11\right|+{p}_{0}{\left|00\right\rangle }_{AB}\left\langle 00\right|),$$
(4)

where \({\left|\psi \right\rangle }^{+}=\frac{1}{\sqrt{2}}({\left|01\right\rangle }_{AB}+{\left|10\right\rangle }_{AB})\), is the target entangled state, hereafter we omit the phase shift for simplicity. Other coherence terms (for instance, \(\left|11\right\rangle \left\langle 10\right|\) and \(\left|10\right\rangle \left\langle 11\right|\)) are neglected47, since we are only interested in the coherence property of the target entangled state. \({\left|11\right\rangle }_{AB}\) represents the multi-photon component which affects the fidelity of the state with the weight of p2. It appears due to the event in which both ensembles are excited but only one photon is detected during the EG process. \({\left|00\right\rangle }_{AB}\) is the vacuum component which reduces the entanglement distribution rate with the weight of p0. It comes from the event heralded by the dark count of the single-photon detector. The fidelity can be expressed as:

$${F}_{0}=\frac{1}{1+{p}_{2}},$$
(5)

where F0 ≈ 1 − p218, with p2 ≪ 1.

According to Eq. (5), the multi-photon component is the primary source of errors that decrease the fidelity. In the following EC process, this error component dramatically amplifies, thus decreases the fidelity of the distributed entangled state between distant nodes. In the original and the improved DLCZ-like schemes47,48, minimizing the excitation probability pc to suppress the multi-photon component is a widely-used passive countermeasure to maintain the fidelity of the distributed entangled state18,47,48. However, a small pc directly translates into a low data rate for entanglement distribution and a long storage time required for the memory. Meanwhile, the dark counts of the single-photon detectors will cause an enormous vacuum component during the EG process when pc is smaller than 10−2, hampering the EC process (see explicit calculation in the next section). These inefficiency and demanding requirements have so far limited the implementation of the DLCZ protocol within a short scale. In the following paragraph, we will present an ED proposal to eliminate the multi-photon component in a more practical way.

In atomic ensembles that have coupled electron and nuclear spins (Fig. 2a), state \(|g\rangle\) and \(\left|s\right\rangle\) can be represented by \(|{0}_{e}{0}_{n}\rangle\) and \(\left|{1}_{e}{0}_{n}\right\rangle\), where the subscript e and n denotes the electron and nuclear degrees of freedom, respectively. \({|\psi \rangle }^{+}\) can be rewritten as:

$$\frac{1}{\sqrt{2}}({\left|{0}_{e}{0}_{n}\right\rangle }_{A}{\left|{1}_{e}{0}_{n}\right\rangle }_{B}+{\left|{1}_{e}{0}_{n}\right\rangle }_{A}{\left|{0}_{e}{0}_{n}\right\rangle }_{B}).$$
(6)

\({\left|{0}_{e}{0}_{n}\right\rangle }_{A}{\left|{1}_{e}{0}_{n}\right\rangle }_{B}={\left|01\right\rangle }_{AB}\), \({\left|{1}_{e}{0}_{n}\right\rangle }_{A}{\left|{0}_{e}{0}_{n}\right\rangle }_{B}={\left|10\right\rangle }_{AB}\). \({\left|{1}_{e}{0}_{n}\right\rangle }_{A}{\left|{1}_{e}{0}_{n}\right\rangle }_{B}={\left|11\right\rangle }_{AB}\), \({\left|{0}_{e}{0}_{n}\right\rangle }_{A}{\left|{0}_{e}{0}_{n}\right\rangle }_{B}={\left|00\right\rangle }_{AB}\). As shown in Fig. 2a, a radio frequency (RF) \(\frac{\pi }{2}\) pulse is applied between the spin levels \(\left|{1}_{e}{0}_{n}\right\rangle\) and \(\left|{1}_{e}{1}_{n}\right\rangle\) on ensemble A and B separately. After these operations, \({\left|{0}_{e}{0}_{n}\right\rangle }_{A(B)}\) unchanges and \({\left|{1}_{e}{0}_{n}\right\rangle }_{A(B)}\) turns to \(\frac{1}{\sqrt{2}}\big({\left|{1}_{e}{0}_{n}\right\rangle }_{A(B)}+{\left|{1}_{e}{1}_{n}\right\rangle }_{A(B)}\big)\). As a result,

$${\left|{0}_{e}{0}_{n}\right\rangle }_{A}{\left|{1}_{e}{0}_{n}\right\rangle }_{B} \to \frac{1}{\sqrt{2}}\big({\left|{0}_{e}{0}_{n}\right\rangle }_{A}{\left|{1}_{e}{0}_{n}\right\rangle }_{B}+{\left|{0}_{e}{0}_{n}\right\rangle }_{A}{\left|{1}_{e}{1}_{n}\right\rangle }_{B}\big),\\ {\left|{1}_{e}{0}_{n}\right\rangle }_{A}{\left|{0}_{e}{0}_{n}\right\rangle }_{B} \to \frac{1}{\sqrt{2}}\big({\left|{1}_{e}{0}_{n}\right\rangle }_{A}{\left|{0}_{e}{0}_{n}\right\rangle }_{B}+{\left|{1}_{e}{1}_{n}\right\rangle }_{A}{\left|{0}_{e}{0}_{n}\right\rangle }_{B}\big),\\ {\left|{1}_{e}{0}_{n}\right\rangle }_{A}{\left|{1}_{e}{0}_{n}\right\rangle }_{B} \to \frac{1}{2}\big({\left|{1}_{e}{0}_{n}\right\rangle }_{A}{\left|{1}_{e}{0}_{n}\right\rangle }_{B}+{\left|{1}_{e}{0}_{n}\right\rangle }_{A}{\left|{1}_{e}{1}_{n}\right\rangle }_{B}\\ \quad\;+{\left|{1}_{e}{1}_{n}\right\rangle }_{A}{\left|{1}_{e}{0}_{n}\right\rangle }_{B}+{\left|{1}_{e}{1}_{n}\right\rangle }_{A}{\left|{1}_{e}{1}_{n}\right\rangle }_{B}\big).$$
Fig. 2: The entanglement distillation proposal and its enhancement on entanglement fidelity and rate.
figure 2

a The energy-level diagram and the entanglement distillation proposal. A and B represent two distant communication nodes. The subscript e and n denotes the electron and nuclear degrees of freedom, respectively. Anti-Stokes, AS; beam splitter, BS; Detector, D. b The fidelity after entanglement distillation as a function of the iteration times with the initial fidelity of 0.2 (triangle symbol and yellow line), 0.6 (dot symbol and green line) and 0.8 (square symbol and purple line), respectively. c The speedup coefficient Rs as a function of the nesting levels by increasing the excitation rate pc and utilizing entanglement distillation after the last level’s entanglement connection process. pc = 10−3 (triangle symbol and yellow line), 10−4 (dot symbol and green line), 10−5 (square symbol and purple line). The dot, dash, solid line represents the total efficiency η = 90%, 95%, 100%, respectively.

Full size image

Then the excitations on \(\left|{1}_{e}{0}_{n}\right\rangle\) are retrieved and the induced anti-Stokes photons are detected. Only when D3, D4, D5, and D6 (as shown in Fig. 2a, these detectors are already assembled in the EC processes) both record no click, the distillation process is successful. Otherwise, the ensembles are initialized and the previous steps are repeated. The successful probability is \(\frac{1}{2}\) for the \({\left|\psi \right\rangle }^{+}\) component, \(\frac{1}{4}\) for the \({\left|11\right\rangle }_{AB}\) and 1 for the \({\left|00\right\rangle }_{AB}\) component, assuming perfect retrieval and detection efficiency. Upon success, a π pulse is applied between \(\left|{1}_{e}{0}_{n}\right\rangle\) and \(\left|{1}_{e}{1}_{n}\right\rangle\) on ensemble A and B separately, to bring back the useful \({\left|\psi \right\rangle }^{+}\) state. The remained state after distillation is:

$$\frac{1}{1+{p}_{2}/2+2{p}_{0}}\left({|\psi \rangle }^{+}\langle \psi |+\frac{{p}_{2}}{2}{\left|11\right\rangle }_{AB}\left\langle 11\right|+2{p}_{0}{\left|00\right\rangle }_{AB}\langle 00|\right).$$
(7)

The fidelity increases to \({F}_{1}=\frac{1}{1+{p}_{2}/2}\). The distillation process can be conducted for many times. After n levels’ distillation, the fidelity is improved to \({F}_{n}=\frac{1}{1+{p}_{2}/{2}^{n}}\). The fidelity of the entangled state after the ED process monotonously increases as the iteration time grows, even if taking the imperfections of the memories and detectors into account (as shown in Fig. 2b), the detailed analysis and calculations are provided in Supplementary Note 1 and Supplementary Fig. 1. To effectively implement our protocol, a high detection and memory efficiency (>50%) are required, which can be achieved by utilizing impedance-match optical cavity to enhance the memory efficiency49 and efficient detectors to maximize the detection efficiency50.

The proposal has no restriction on the fidelity of the raw state, thus the distillation process can be applied at any level of the EG and ES steps, which is not allowed for the previous entanglement purification schemes8,9,23.

Accelerating entanglement distribution with entanglement distillation

Now we will show that the ED introduced above can help accelerate the entanglement distribution rate, considering the dark counts of single-photon detectors in realistic situations. Suppose an entangled state is established between node A and B during the EG process. The excitation rate is pc, then the state is written as:

$$\rho =\frac{1}{1+{p}_{c}+{p}_{0}}\big(|\psi \rangle \langle \psi |+{p}_{c}\left|11\right\rangle \left\langle 11\right|+{p}_{0}\left|00\right\rangle \left\langle 00\right|\big).$$
(8)

p0 is the vacuum coefficient, and is expressed as18

$${p}_{0}=\frac{{p}_{dc}}{{p}_{c}{\eta }_{p}},$$
(9)
$${\eta }_{p}={\eta }_{t}{\eta }_{o}{\eta }_{d}.$$
(10)

Here, pdc is the probability of recording a click caused by dark count of the detector in a single detection window, \({\eta }_{t}=1{0}^{-\frac{{L}_{0}}{2{L}_{att}}}\) is the channel attenuation, L0 is the distance between two neighboring repeater stations, Latt = 40 km is the attenuation length of the channel. ηo results from other noise (for instance, the spontaneous emissions in the atomic ensembles and the coupling inefficiency of the photons into and out of the channel), and is independent of the communication distance. ηd is the detection efficiency. ηp is the overall efficiency. For a typical elementary link distance L0 = 160 km, ηt = 10−2. Suppose the realistic single-photon detectors have a dark count rate of 10 Hz and a detection window of 100 ns, pdc = 10−6. The fidelity is written as

$$F=\frac{1}{1+{p}_{c}}.$$
(11)

The entanglement generation rate is defined as:

$${R}_{g}={p}_{c}{\eta }_{p}.$$
(12)

Considering the memory efficiency of ηm, let

$$\eta ={\eta }_{m}{\eta }_{d},$$
(13)

the entangled state after the i-th level EC process can be expressed as

$${\rho }_{(i)}= \frac{1}{1+{p}_{2(i)}+{p}_{0(i)}}({|\psi \rangle }^{+}\langle \psi |+{p}_{2(i)}\left|11\right\rangle \left\langle 11\right|+{p}_{0(i)}\left|00\right\rangle \left\langle 00\right|),$$
(14)

where, p0(0) = p0, p2(0) = pc. p2(i) = 2p2(i−1), for i ≥ 1. For the conventional single-photon detectors that do not have photon number resolution, \({p}_{0(i)}=2{p}_{0(i-1)}+\frac{1}{2}(2-\eta )\). The successful establishment of the entangled state between two distant nodes requires the success of the EC processes at all levels, whose probability is expressed as:

$${P}_{tot}={\left(\frac{2}{3}\right)}^{n}{P}_{s(1)}{P}_{s(2)}\ldots {P}_{s(i)}\ldots {P}_{s(n)},$$
(15)

where

$${P}_{s(i)} =\, \frac{\frac{1}{2}+\frac{(2-\eta )}{4}+{p}_{2(i-1)}+{p}_{0(i-1)}}{{(1+{p}_{0(i-1)}+{p}_{2(i-1)})}^{2}}\eta \\ \approx\, \frac{1-\frac{\eta }{4}+{p}_{0(i-1)}}{{(1+{p}_{0(i-1)})}^{2}}\eta$$
(16)

is the successful probability of the i-th level’s EC process. The coefficient \(\frac{2}{3}\) is due to the fact that for every i-th level EC process one has to establish two nearby links at the (i − 1)-th level in advance, considering the low transmissivity regime17. The total entanglement distribution rate is

$${R}_{tot}={R}_{g}{P}_{tot}.$$
(17)

A large vacuum component will be generated and the entanglement distribution rate will be slowed down when pc is quite small. In order to suppress the effect of massive vacuum component, we can increase the excitation rate pc to \({p}_{c}^{\prime}\) in the EG process, and utilizing the ED process after the last level’s EC process to achieve the identical fidelity. The state with excitation rate of \({p}_{c}^{\prime}\) is

$$\rho ^{\prime} =\, \frac{1}{1+{p}_{c}^{\prime}+{p}_{0}^{\prime}}\big({|\psi\rangle }^{+}\langle \psi |+{p}_{c}^{\prime}\left|11\right\rangle \left\langle 11\right|+{p}_{0}^{\prime}\left|00\right\rangle \left\langle 00\right|\big),\\ {p}_{0}^{\prime} =\, \frac{{p}_{dc}}{{p}_{c}^{\prime}{\eta }_{p}}.\\ F^{\prime} =\, \frac{1}{1+{p}_{c}^{\prime}}.$$
$${R}_{g}^{\prime}={p}_{c}^{\prime}{\eta }_{p}.\kern13.7pc$$
(18)

The total entanglement distribution rate with excitation rate of \({p}_{c}^{\prime}\) and nesting level of n is

$${R}_{tot}^{\prime}={R}_{g}^{\prime}{P}_{tot}^{\prime}{P}_{d}^{\prime}.$$
(19)

Where, \({P}_{tot}^{\prime}\) is the probability that all the EC processes are successful with the excitation rate \({p}_{c}^{\prime}\), \({P}_{d}^{\prime}\) is the successful probability of the ED process.

Here we define a parameter

$${R}_{s}=\frac{{R}_{tot}^{\prime}}{{R}_{tot}}$$
(20)

to quantitatively characterize the speedup effect of our proposal. Figure 2c shows the speedup effect for different nesting levels (see explicit calculation in Supplementary Note 2).

Improvement of the entanglement connection process

The LOs and detection also can be utilized to improve the EC process. Suppose two pairs of entangled state have been generated between node A, B and node C, D. The state \({\left|{{\Psi }}\right\rangle }_{ABCD}\) is:

$$\frac{1}{2}\big(\left|{0}_{A}{1}_{B}{0}_{C}{1}_{D}\right\rangle +\left|{1}_{A}{0}_{B}{1}_{C}{0}_{D}\right\rangle +\left|{0}_{A}{1}_{B}{1}_{C}{0}_{D}\right\rangle +\left|{1}_{A}{0}_{B}{0}_{C}{1}_{D}\right\rangle \big).$$

EC process is based on detection of the interference of the anti-Stokes photons emitted by B and C, and is successful when only one click is recorded by the detectors. However, the \(\left|{0}_{A}{1}_{B}{1}_{C}{0}_{D}\right\rangle\) component also causes only one click if the detectors have no full photon number resolution, inducing a vacuum component \(\left|{0}_{A}{0}_{D}\right\rangle\)17,47. The state after the EC process can be written as

$${\rho }_{AD}=\frac{1}{1+{p}_{0(1)}}\big({|\psi \rangle }_{AD}\langle \psi |+{p}_{0(1)}{\left|00\right\rangle }_{AD}\left\langle 00\right|\big),$$
(21)

where \({|\psi \rangle }_{AD}\) is similar as \({|\psi \rangle }_{AB}\), p0(1) is the coefficient of the vacuum component generated during the EC process. This component amplifies during the later EC processes, decreases the successful probabilities of the EC processes and reduces the entanglement distribution rate. Figure 3b illustrates the successful probabilities of the EC processes as a function of the swapping levels, utilizing the single-photon detector with different photon number resolution. \({p}_{0(1)}=1-\frac{1}{2}\eta\) for the conventional single-photon detectors that do not possess photon number resolution.

Fig. 3: Improving the entanglement connection process.
figure 3

a Scheme for optimization of the entanglement connection process. B and C are two adjacent nodes. Anti-Stokes, AS; beam splitter, BS; Detector, D. b The successful probabilities of the entanglement connection process as a function of the swapping levels (n) utilizing single-photon detectors with photon number resolution of 0 (triangle), 50% (dot), and 100% (square). Here we have assumed the total efficiency η = 0.9 and excitation rate pc = 0.001.

Full size image

We propose that the LOs and detection can mimic the function of the photon-number resolving detector, which is currently a technology in development51,52. The EC processes can be optimized as follows (Fig. 3a).

As displayed in Fig. 2a, \(\left|{0}_{A}{1}_{B}{1}_{C}{0}_{D}\right\rangle\) can be rewritten as \({\left|{0}_{e}{0}_{n}\right\rangle }_{A}{\left|{1}_{e}{0}_{n}\right\rangle }_{B}{\left|{1}_{e}{0}_{n}\right\rangle }_{C}{\left|{0}_{e}{0}_{n}\right\rangle }_{D}\), in the same way, \(\left|{0}_{A}{1}_{B}{0}_{C}{1}_{D}\right\rangle ={\left|{0}_{e}{0}_{n}\right\rangle }_{A}{\left|{1}_{e}{0}_{n}\right\rangle }_{B}{\left|{0}_{e}{0}_{n}\right\rangle }_{C}{\left|{1}_{e}{0}_{n}\right\rangle }_{D}\), \(\left|{1}_{A}{0}_{B}{1}_{C}{0}_{D}\right\rangle ={\left|{1}_{e}{0}_{n}\right\rangle }_{A}{\left|{0}_{e}{0}_{n}\right\rangle }_{B}{\left|{1}_{e}{0}_{n}\right\rangle }_{C}{\left|{0}_{e}{0}_{n}\right\rangle }_{D}\),\(\left|{1}_{A}{0}_{B}{0}_{C}{1}_{D}\right\rangle ={\left|{1}_{e}{0}_{n}\right\rangle }_{A}{\left|{0}_{e}{0}_{n}\right\rangle }_{B}{\left|{0}_{e}{0}_{n}\right\rangle }_{C}{\left|{1}_{e}{0}_{n}\right\rangle }_{D}\). First, a \(\frac{\pi }{2}\) pulse is applied between \(\left|{1}_{e}{0}_{n}\right\rangle\) and \(\left|{1}_{e}{1}_{n}\right\rangle\) on ensemble B and C separately. In this case,

$$\begin{array}{l}{\left|{1}_{e}{0}_{n}\right\rangle }_{B}{\left|{0}_{e}{0}_{n}\right\rangle }_{C}\to \frac{1}{\sqrt{2}}\big({\left|{1}_{e}{0}_{n}\right\rangle }_{B}{\left|{0}_{e}{0}_{n}\right\rangle }_{C}+{\left|{1}_{e}{1}_{n}\right\rangle }_{B}{\left|{0}_{e}{0}_{n}\right\rangle }_{C}\big)\\ {\left|{0}_{e}{0}_{n}\right\rangle }_{B}{\left|{1}_{e}{0}_{n}\right\rangle }_{C}\to \frac{1}{\sqrt{2}}\big({\left|{0}_{e}{0}_{n}\right\rangle }_{B}{\left|{1}_{e}{0}_{n}\right\rangle }_{C}+{\left|{0}_{e}{0}_{n}\right\rangle }_{B}{\left|{1}_{e}{1}_{n}\right\rangle }_{C}\big)\\ {\left|{1}_{e}{0}_{n}\right\rangle }_{B}{\left|{1}_{e}{0}_{n}\right\rangle }_{C}\to \frac{1}{2}\left({\left|{1}_{e}{0}_{n}\right\rangle }_{B}{\left|{1}_{e}{0}_{n}\right\rangle }_{C}+{\left|{1}_{e}{0}_{n}\right\rangle }_{B}{\left|{1}_{e}{1}_{n}\right\rangle }_{C}\right.\\ \qquad\qquad\qquad\quad\;\;\left.+{\left|{1}_{e}{1}_{n}\right\rangle }_{B}{\left|{1}_{e}{0}_{n}\right\rangle }_{C}+{\left|{1}_{e}{1}_{n}\right\rangle }_{B}{\left|{1}_{e}{1}_{n}\right\rangle }_{C}\right).\end{array}$$

Then, the excitation on \(\left|{1}_{e}{0}_{n}\right\rangle\) of ensemble B and C is retrieved simultaneously.

Second, a π pulse between \(\left|{1}_{e}{0}_{n}\right\rangle\) and \(\left|{1}_{e}{1}_{n}\right\rangle\) is applied, following another retrieving process on \(\left|{1}_{e}{0}_{n}\right\rangle\). The success of the EC process is defined as the case when only one click is recorded during the two retrieving processes. As a result, the coefficient of the vacuum component p0(1) is modified to \(1-\frac{3}{4}\eta\) (see explicit calculation in Supplementary Note 3).

Moreover, the single retrieving process can be divided to 2l(l ≥ 1) times, once we have 2l sub-levels. Then the coefficient of the vacuum component can be further written to

$${p}_{0(1)}=1-\eta +\frac{1}{{4}^{l}}\eta ,$$
(22)

a specific example and detailed considerations has been provided in Supplementary Note 3.

The \(\left|{0}_{A}{1}_{B}{1}_{C}{0}_{D}\right\rangle\) component can be almost fully distinguished with a high l, which is equal to the function of the fully-photon-number-resolving detector. Such high dimensional Hilbert space can be obtained in rare-earth material with nuclear spin of high quantum numbers53,54. Hence, the \(\left|{0}_{A}{0}_{D}\right\rangle\) component is minimized after the EC process.

Initial experiments

These LOs on the hybrid spin levels are the primary requirements for our ED proposal for the atomic-ensemble-based quantum node. The functions of the \(\frac{\pi }{2}\) and π pulses employed here are similar to the transformations result from the controlled Hadamard and CNOT gates between electron and nuclear spins55,56, whose fidelity determines the final entanglement fidelity as well as the entanglement distribution rate. Here we demonstrate these operations with high fidelity in an actual physical system, i.e., a 143Nd3+:Y2SiO5 crystal. This material is chosen because of its large Hilbert space, with electron spin \(S=\frac{1}{2}\) and nuclear spin \(I=\frac{7}{2}\)[ 30,54. By combining series of these LOs and an efficient initial state preparation, we can prepare a high-fidelity entangled state between the electron and nuclear spins.

The spin Hamiltonian of our system is represented as30,54:

$$H={\mu }_{B}{{{{{{{\boldsymbol{{B}}}}}}}_{0}\cdot \; {{{{{\boldsymbol{g}}}}}}\; \cdot \; {{{{{\boldsymbol{S}}}}}}\; +\; {{{{{\boldsymbol{S}}}}}}\; \cdot \; {{{{{\boldsymbol{A}}}}}}\; \cdot \; {{{{{\boldsymbol{I}}}}}}}}.$$
(23)

Here μB is the Bohr magneton, A and g are the hyperfine and g tensors, respectively, and I (S) denotes the nuclear (electron) spin operator. The corresponding energy-level diagram is shown in Fig. 4. Here we have chosen \({\left|\!\pm\! \frac{1}{2}\right\rangle }_{e}{\left|\!+\!\frac{1}{2}\right\rangle }_{n}\), \({\left|\pm \frac{1}{2}\right\rangle }_{e}{\left|+\frac{3}{2}\right\rangle }_{n}\), and \({\left|\!+\!\frac{1}{2}\right\rangle }_{e}{\left|\!-\!\frac{1}{2}\right\rangle }_{n}\) to conduct the experiments. We encode \({\left|\!-\!\frac{1}{2}\right\rangle }_{e}\) (\({\left|\!+\!\frac{1}{2}\right\rangle }_{e}\)) as \({\left|1\right\rangle }_{e}\) (\({\left|0\right\rangle }_{e}\)) and \({\left|+\frac{1}{2}\right\rangle }_{n}\) (\({\left|+\frac{3}{2}\right\rangle }_{n}\)) as \({\left|1\right\rangle }_{n}\) (\({\left|0\right\rangle }_{n}\)) for simplicity. The four orthogonal states can be rewritten as \(\left|{0}_{e}{0}_{n}\right\rangle\), \(\left|{0}_{e}{1}_{n}\right\rangle\), \(\left|{1}_{e}{0}_{n}\right\rangle\), \(\left|{1}_{e}{1}_{n}\right\rangle\), forming a two-qubit subspace. \({\left|+\frac{1}{2}\right\rangle }_{e}{\left|-\frac{1}{2}\right\rangle }_{n}\) is labeled as \(\left|aux\right\rangle\), and is employed to transfer the unwanted population. The associated electron-spin-resonance (ESR) transition (ΔmS = ±1, ΔmI = 0) and nuclear-magnetic-resonance (NMR) transitions (ΔmS = 0, ΔmI = ±1) are labeled as MW (9.56 GHz), RF1 (212.2 MHz), RF2 (219.2 MHz), and RF3 (262.9 MHz), respectively. The NMR transition frequencies are measured and identified at 100 mK utilizing the method introduced by Pei-Yun et al.54. In the two-qubit subspace, the Bell state is written as:

$${\left|{{\Psi }}\right\rangle }^{\pm }=\frac{1}{\sqrt{2}}\left(\left|{0}_{e}{1}_{n}\right\rangle \pm \left|{1}_{e}{0}_{n}\right\rangle \right),$$
(24)
$${\left|{{\Phi }}\right\rangle }^{\pm }=\frac{1}{\sqrt{2}}\left(\left|{0}_{e}{0}_{n}\right\rangle \pm \left|{1}_{e}{1}_{n}\right\rangle \right).$$
(25)

Here we prepare the \({\left|{{\Psi }}\right\rangle }^{+}\) state by combining series of these spin operations and an efficient initial state preparation. Our experiment proceeds in two steps: (1) initialization and entanglement generation, (2) tomography of the density matrices.

Fig. 4: The energy-level structure of the 4I9/2(0) state of 143Nd3+:Y2SiO5 under an external magnetic field of B0 = 439.8 mT.
figure 4

The red arrow indicates the electron-spin-resonance transition between the \({\left|+\frac{1}{2}\right\rangle }_{e}{\left|+\frac{1}{2}\right\rangle }_{n}\) and \({\left|-\frac{1}{2}\right\rangle }_{e}{\left|+\frac{1}{2}\right\rangle }_{n}\) state. The green arrows represent the relevant nuclear-magnetic-resonance transitions, whose frequencies are also labeled. \(\left|aux\right\rangle\) represent the auxiliary energy level. mS and mI denote the electron and nuclear spin quantum number, respectively. Microwave, MW; radio-frequency, RF.

Full size image

For a thermal equilibrium state, the populations are predominantly determined by the electron spin polarization, which is expressed as \(\alpha ={e}^{(-\frac{g{\mu }_{B}{B}_{0}}{{k}_{B}T})}\)57, where B0 is the magnetic field, g is the electron g-factor, and T is the sample temperature. In a previous work, a high field (3.4 T) and a low temperature (2.9 K) are combined to polarize the electron spins of phosphorus doped in silicon to 66%57. Here, the electron spins approach fully polarized (α = 1.02%) with a modest magnetic field of 439.8 mT with the assistance of an ultra-low sample temperature of 100 mK54. As shown in Fig. 5a, starting from the thermal equilibrium state, a MW pulse P11,01(π) and a RF pulse P01,aux(π) are applied successively to create an almost pure initial state \(\left|{1}_{e}{0}_{n}\right\rangle\) in the 4-dimensional subspace. Then, \({\left|{{\Psi }}\right\rangle }^{+}\) can be generated by applying \({P}_{10,11}(\frac{\pi }{2})\) and P11,01(π) on the initial state one after another, as shown in Fig. 5b. The other entangled states can be achieved by using different pulse sequences. For instance, applying P10,11(π), \({P}_{11,01}(\frac{\pi }{2})\), P01,00(π) on the initial state successively will produce \({\left|{{\Phi }}\right\rangle }^{+}\).

Fig. 5: Sequences for initialization and entanglement generation process.
figure 5

a Preparation of the initial state. A microwave (MW) π pulse and radio-frequency (RF) π pulse are applied successively to achieve the population inversion between \(\left|{1}_{e}{1}_{n}\right\rangle\) and the auxiliary energy level \(\left|aux\right\rangle\). Hence, an almost pure initial state is presented in the two-qubit subspace. b Generation of the entangled state between the electron and nuclear spins. A RF π/2 generates the superposition between \(\left|{1}_{e}{0}_{n}\right\rangle\) and \(\left|{1}_{e}{1}_{n}\right\rangle\), then a MW π pulse is applied between \(\left|{1}_{e}{1}_{n}\right\rangle\) and \(\left|{0}_{e}{1}_{n}\right\rangle\), inducing the entangled state: \(\frac{1}{\sqrt{2}}\left(\left|{0}_{e}{1}_{n}\right\rangle +\left|{1}_{e}{0}_{n}\right\rangle \right)\). P denotes pulse.

Full size image

In order to characterize the fidelity of the spin operations and the degree of the entanglement, we employ density matrix tomography to reveal the initial state and the final entangled state55,57,58,59,60,61. For the initial state, we only need to determine the diagonal elements since no coherence is excited during the initialization process. By mapping different pairs of population difference into the electron spin echo between the \(\left|{1}_{e}{1}_{n}\right\rangle\) and \(\left|{0}_{e}{1}_{n}\right\rangle\) state, and comparing the echo amplitude with that of the thermal equilibrium state, the corresponding diagonal elements can be obtained. The experimental result is:

$${\rho }_{i}^{exp}=\left(\begin{array}{lllll}0.011&0&0&0&\\ 0&0.051&0&0&\\ 0&0&0.933&0&\\ 0&0&0&0&0.005\end{array}\right).$$
(26)

Here we use the formula \({F}_{{{{{{\rm{state}}}}}}}=tr\sqrt{\sqrt{{\rho }_{}^{th}}{\rho }_{}^{exp}\sqrt{{\rho }_{}^{th}}}\)57,62,63 to calculate the state fidelity, \({\rho }_{}^{th}\) and \({\rho }_{}^{exp}\) denote the theoretical and experimental density matrix, respectively. The fidelity compared with the target initial state \({\rho }_{i}^{th}={{{{{{{\boldsymbol{diag}}}}}}}}\{\frac{\alpha }{1+3\alpha },\frac{\alpha }{1+3\alpha },\frac{1}{1+3\alpha }\,\frac{\alpha }{1+3\alpha }\}\) is 99.1(4)%, indicating the reliability of the initial state preparation process. Starting from this initial state, two selective pulses \({P}_{10,11}(\frac{\pi }{2})\) and P11,01(π) will produce the target entangled state in theory (with the absence of pulse imperfections and decoherence):

$${\rho }_{f}^{th}=\left(\begin{array}{lllll}0.011&0&0&0&\\ 0&0.469&0.464&0&\\ 0&0.464&0.469&0&\\ 0&0&0&0&0.051\end{array}\right).$$
(27)

Now we turn to the reconstruction of the final entangled state. The determination of the diagonal elements of the final entangled state is the same as that of the initial state. The detection of the off-diagonal elements is more complicated. Here we follow the method introduced by Stephanie et al.57 to add different geometric phases for each pair of the states before detecting their coherence. A pair of pulses \({P}_{i,j}^{\varphi }(\!\pm\! \pi )\) (or \({P}_{i,j}^{\sigma }(\!\pm\! \pi )\)) are applied along different axes on the Bloch sphere spanned by a pair of states \(\left|i\right\rangle\) and \(|j\rangle\), where the superscript denotes the nominal rotation axes with angle φ (or σ). Each state accumulates a geometric phase which is equal to half of the solid angle of its trajectory on the Bloch sphere57,64,65. The effect of the two pulses can be described as an operation \({e}^{-i\varphi }\left|i\right\rangle \left\langle i\right|+{e}^{i\varphi }|j\rangle \langle j|\) (or \({e}^{-i\sigma }\left|i\right\rangle \left\langle i\right|+{e}^{i\sigma }|j\rangle \langle j|\)) with a geometric phase φ (or σ).

As the experiments repeated, φ and σ increase with different steps. Thus, the difference of the geometric phases between different pair of states is modulated with a particular frequency. Hence, the echo amplitudes are also modulated when mapping the coherence between different pair of states into population difference between \(\left|{1}_{e}{1}_{n}\right\rangle\) and \(\left|{0}_{e}{1}_{n}\right\rangle\). The modulation frequency varies for different pairs of states. Thus the off-diagonal element of the density matrix (i.e., the coherence between a pair of states \(\left|i\right\rangle\) and \(|j\rangle\)) can be identified at a particular frequency corresponding to the labeled geometric phase of the Fourier component. As a specific example, Fig. 6a illustrates the complete pulse sequence for initialization, entanglement generation, phase labeling, and detection of the \(\left|{1}_{e}{0}_{n}\right\rangle \left\langle {0}_{e}{1}_{n}\right|\) element. Figure 6b shows the corresponding oscillation of the echo amplitude utilizing quadrature detection. Other off-diagonal elements relevant to \(\left|{0}_{e}{0}_{n}\right\rangle\) are zero due to the fact that the excitation bandwidths of the MW and RF pulses are relatively small and no coherence could have been generated there55. At last, we obtain the full density matrix of the final entangled state:

$${\rho }_{f}^{exp}= \left(\begin{array}{llll}0.017&0&0&0\\ 0&0.481&0.380&0.021+0.110i\\ 0&0.380&0.480&0.095+0.161i\\ 0&0.021-0.110i&0.095-0.161i&0.022\end{array}\right).$$
(28)

Here we have applied a global phase correction to cancel the imaginary part of the \(\left|{1}_{e}{0}_{n}\right\rangle \left\langle {0}_{e}{1}_{n}\right|\) element61. Figure 6c and d displays the real and imaginary part of the density matrix.

Fig. 6: Reconstruction of the density matrix.
figure 6

a The complete pulse sequences for initialization, entanglement generation, geometric phase labeling, and detection for extracting the \(\left|{1}_{e}{0}_{n}\right\rangle \left\langle {0}_{e}{1}_{n}\right|\) element. Microwave, MW; radio-frequency, RF. P denotes pulse. b The oscillation of the echo amplitude acquired by quadrature detection and the corresponding Fourier transform spectrum. The solid lines and dash lines represent the real (in-phase) and imaginary (quadrature) component, respectively. Imag denotes the imaginary part. c, d The reconstructed density matrix shown in the basis of hybrid-spin system. The zero elements are displayed with green shading.

Full size image

The fidelity of the generated entangled state with respect to the target entangled state is calculated as 94.1(8)%. The fidelity compared with the Bell state is determined as 92.7(8)%, and the corresponding concurrence is calculated as C = 0.75, which is greatly enhanced compared with the previous work57, indicating the high quality of the generated entangled state. The reduction of the state fidelity from 99.1(4)% to 94.1(8)% is mainly due to the loss of the coherence during the phase labeling and detection process and the pulse imperfections. The operation fidelity is determined as Fg = 97.2% (see Supplementary Note 4 for details). The infidelity is due to the inhomogeneous broadening of electron spins, and can be eliminated by applying composite pulses66,67,68. We point out that, the fidelity and concurrence of the final entangled state will be upgraded when taking the decoherence effect into account. As a result, the operation fidelity of Fg = 97.2% is actually a lower bound.

Conclusion

We propose a specific positive-operator valued measure of the filtering protocol which can be effectively implemented on the ensemble-based quantum nodes. This proposal only requires the LOs applied on the hybrid electron-nuclear spins along with the detection of single photons, and has no restriction on the fidelity of the raw state. By demonstrating these LOs with fidelity as high as 97.2%, a high-quality entangled state is established between the electron and nuclear spins in a rare-earth-ion-doped crystal. Combined with the DLCZ or the DLCZ-like quantum repeater schemes17,47,48, our proposal can upgrade the fidelity of the distributed entangled state as well as increase the entanglement distribution rate. Our proposal can also be adapted to other quantum repeater schemes, for instance, achieving partial readout of the anti-Stokes photon by applying high-fidelity spin operations17.

Improvements in the near future can be expected by several approaches: First, in our ED proposal, only the errors from multi-photon excitation and vacuum state is considered. However, the current distillation proposal is fully compatible with the specially designed protocol to further correct the phase errors as shown by Jiang et al.47 and Nicolas et al.69. Second, the LO itself is a powerful method to counteract unavoidable decoherence and imperfections during the whole process. Using optimal pulse parameters design and advanced composite pulse sequences66,67,68 can increase the fidelity to reach the fault-tolerance threshold. Finally, the method developed provides the toolkit to explore similar platforms such as other solid-state spin systems.

Methods

The sample is a 143Nd3+:Y2SiO5 crystal. It has a dopant concentration of 20 ppm and the 143Nd has an isotope enrichment of 91%. The crystal is cut along its optical axes, i.e., D1, D2, and b70, with dimensions of 1.2 × 1.0 × 1.4 mm. With the help of a home-built sub-Kelvin pulsed electron-nuclear-double-resonance (ENDOR) spectrometer, the sample can be faithfully cooled to 100 mK (see detail in the work of Pei-Yun54).

The spectrometer can generate Microwave (MW) and radio-frequency (RF) pulses with peak power of 20 and 100 W, which can be utilized to coherently drive the electron and nuclear spins. An electromagnet provides a magnetic field of 439.8 mT along the D1 axis of the crystal, inducing a Zeeman splitting of the electron spin levels of 9.56 GHz, matching the resonant frequency of the resonator. Typically, the duration of the MW (RF) π pulse is 50 ns (1 µs) in our experiments.