Introduction

Hybrid nanofluids showed enhanced characteristics of thermal conductivity in comparison with the conventional nanofluids; this is because hybrid nanofluids are a composite mixture of metallic, polymeric or non-metallic nano-sized power with base fluid used to improve the heat transfer rate in different applications. By Adding hybrid nanoparticles, one can significantly enhance thermal conductivity of the base fluid. It is worth noted that hybrid nanofluid can have higher heat transfer rate as compared to pure fluid as it is proved experimentally and numerically by numerous researchers1,2. It is established fact that metal nanoparticles exhibit unique properties that are highly appreciated in many novel-material applications, for instance, process of coatings, e.g., process of antibacterial, manufacturing of sensors and several others1,2,3,4,5,6. Suresh et al.7 analyzed the experimental aspects of heat transfer phenomena and friction factor in laminar flow of hybrid nanofluid with 0.1% volume fraction of Al2O3-Cu water. They conclude that Nusselt number as well as friction characteristic increases for considered hybrid nanofluid when compared to water and simple nanofluid containing the Al2O3 nanoparticles. Nine et al.8 investigated the thermal behavior of Al2O3-multiwall CNTs hybrid nanofluid dispersed in deionized water. They examined the thermal conductivity and dispersion quality for ground Multiwall CNTs and non-ground Multiwall CNTs. Moreover, greater thermal conductivity was observed for hybrid nanofluid compared to monotype nanofluid with same concentration of Al2O3. A numerical technique based on finite volume discretization method has been applied by Takabi and Shokouhmand9 to analyze the turbulent flow of Al2O3-Cu/water hybrid nanofluid, Al2O3/water nanofluid and pure water through a circular tube within their comparison. Sundar et al.10 studied the flow for turbulence with CNTs-Fe3O4/water hybrid nanofluid for enhancement of heat transfer. They emphasized high thermal conductivity and greater magnetic effects on the nano-composition of carbon nanotubes. Sheikholeslami and Shamlooei11 presented an analysis for thermal and magnetic behavior of Fe3O4-water nanofluid. They presented the impact of pertinent parameters and volume concentration. Here, the conclusion was deeply discussed for thermal radiations when buoyancy force is raised. A model of micropolar hybrid nanofluid under thermal and momentum slip condition has been investigated by Nadeem and Abbas12. They presented and compared different parameters on thermal and velocity profiles for micropolar hybrid nanofluid versus nanofluid. Sajid and Ali13 presented an excellent review on experimental and theoretical consideration of thermal conductivity, synthesizes of nanomaterials and stability for enhancement of hybrid nanofluids. Different study models were discussed for thermal conductivity of hybrid nanofluid and the improvement in rate of heat transfer. Fallah et al.14 investigated the governing equation for laminar flow of hybrid nanofluid over a porous rotating disc for the sake of numerical treatment. They present the variation in temperature distribution and velocity profile for different parameters by means of graphical illustration. Dinarvand et al.15 traced out semi-analytical solution of governing equation for mixed convection flow of hybrid nanofluid over a linearly stretching horizontal sheet near a stagnation point. Ali et al.16 investigated a fully developed flow of hybrid nanofluid based on permeable channel of two plates. Khashie et al.17 studied the magnetized flow of water-based hybrid nanofluid over a permeable disc. Their results for temperature, velocity, Nusselt number and skin friction were depicted for the affected suction as well as Joule heating. Besides these studies of hybrid nanofluid, there are few studies for hybrid nanofluid to enhance rate of heat transfer by using various technical studies embedded here with18,19,20,21,22,23,24,25,26. On the other hand, some recent attempts are hybrid based nanofluid flow over hydromagnetic slippery27,28,29, hybrid nanofluid flow over a slender stretching sheet with zero nanoparticles flux18,30,31,32 and few multi-dimensional approaches for nanofluid with different geometries33,34,35. For the sake of novelty, a new fractionalized model based on hybrid nanofluid is proposed and investigated by employing singular verses and non-singular kernels. The mathematical modeling of hybrid nanofluid is handled via modern fractional definitions of differentiations. The combined Laplace and Fourier Sine transforms have been configurated on the governing equations of hybrid nanofluid. The analytical expression of the governing temperature and velocity equations of hybrid nanofluid have been solved via special functions. For the sake of thermal performance, dimensional analysis of governing equations and suitable boundary conditions based on Mittage-Leffler function have been invoked for the first time in literature. The comparative analysis of heat transfer from hybrid nanofluid has been observed through Caputo-Fabrizio and Atangana-Baleanu differential operators. Finally, our results suggest that inclined and declined profile of heat transfer depends upon the use of copper and alumina nanoparticles.

Fractionalized mathematical modeling of hybrid nanofluid

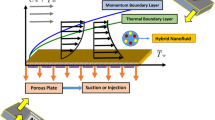
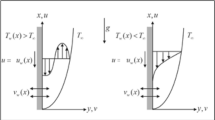
Unsteady, viscous and incompressible flow of hybrid nanofluid lying on a plate is considered, such hybrid nanofluid is suspended through an addition of copper and alumina nanoparticles in ethylene glycol. To deal with the flow of hybrid nanofluid, hybrid nanofluid is assumed over a plate which is at rest initially. When \(t \to 0^{ + }\), the velocity is \(u = u_{0} {\mathbf{E}}_{\alpha } \left( {at} \right)\). Here \(u_{0}\) defines a free stream velocity of boundary. \({\mathbf{E}}_{\alpha } \left( {at} \right)\) represents Mittage-Leffler function and \(t\) is time which cause the movement in terms of generalized exponential plate. The flow of hybrid nanofluid is induced and its velocity varies with time and distance from the plate. The governing equations for a hybrid nanofluid on the basis of assumptions are:

$$ \frac{\partial u}{{\partial x}} + \frac{\partial v}{{\partial y}} = 0,\frac{\partial u}{{\partial t}} = \frac{{\mu_{hnf} }}{{\rho_{hnf} }}\frac{{\partial^{2} u}}{{\partial y^{2} }} + \frac{{\left( {\rho \beta_{T} } \right)_{hnf} g\left( {T - T_{\infty } } \right)}}{{\rho_{hnf} }}, \frac{\partial T}{{\partial t}} = \frac{{{\mathcal{K}}_{hnf} }}{{\left( {\rho C_{p} } \right)_{hnf} }}\frac{{\partial^{2} T}}{{\partial y^{2} }} + \frac{{Q \left( {T - T_{0} } \right)}}{{\left( {\rho C_{p} } \right)_{hnf} }}, $$
(1)

Equation (1) is subjected to the imposed conditions as:

$$ \begin{array}{*{20}l} {y = 0: {\text{and }}t > 0, u = u_{0} {\mathbf{E}}_{\alpha } \left( {at} \right), T = T_{w} , } \hfill \\ {y \to \infty : {\text{and }}t > 0, u \to 0, T \to T_{\infty } .} \hfill \\ \end{array} $$
(2)

The surface and ambient temperature to the hybrid nanolufluid is \(T_{w}\) and \(T_{\infty }\) respectively. Here, \(\left( {x,y} \right)\) is used to measure the distances and normal to surface, while \(\left( {u,v} \right)\) defines the velocities in \(x\) and \(y\) directions. For the sake of simplicity, the thermo-physical parameters used in hybrid nanofluid are described in Appendix (A1–A8). The typical and rheological parameters defined in Appendix (A1–A8) are illustrated as, \(\mu_{hnf}\), \({\mathcal{K}}_{hnf}\) and \(\rho_{hnf}\) are the viscosity, thermal conductivity and density of the hybrid nanofluid respectively. \(\mu_{f} , {\mathcal{K}}_{f} \) and \(\rho_{f}\) are the base fluids. The heat capacity and thermal expansion coefficient are \(C\) and \(\beta\). While \(Al_{2} O_{3}\) and \(C_{u}\) are the nanoparticles and the subscripts \(f,1\) and 2 used for base fluid. The symbolic form of the dynamic viscosity for base fluid is \(\nu_{f}\). Dimensionless parameters of time, normal distance to the surface, temperature and velocity of the hybrid nanofluid are expressed in Eq. (3) as

$$\theta \left(y\right)=\frac{\widehat{T}-{T}_{\infty }}{{T}_{w}-{T}_{\infty }},t=a\widehat{t}, u=\frac{\widehat{u}}{{u}_{\infty }},y=\widehat{y}\sqrt{\frac{a}{{\nu }_{f}}},$$
(3)

Employing Eq. (3) into Eqs. (1–2), we arrive at

$$\frac{1}{{Q}_{0}}\left\{\frac{\partial u (y,t)}{\partial t}-{Q}_{1}Ri\theta (y,t)\right\}=\frac{{\partial }^{2}u \left(y,t\right)}{{\partial y}^{2}},$$
(4)
$$\frac{{P}_{r}}{{\mathcal{Q}}_{2}}\left\{\frac{\partial \theta \left(y,t\right)}{\partial t}-{\mathcal{Q}}_{3}\Delta \theta \left(y,t\right)\right\}=\frac{{\partial }^{2}\theta \left(y,t\right)}{{\partial y}^{2}}.$$
(5)

The imposed conditions are

$$\begin{array}{c}y=0: \mathrm{and }t>0, u={u}_{0}{\mathbf{E}}_{\alpha }\left(at\right), \theta =t, \\ y\to \infty : \mathrm{and }t>0, u\to 0, \theta \to 0. \end{array}$$
(6)

where, the rheological and functional letting parameters for Eqs. (4–5) are illustrated in Appendix (A9–A10). In Appendix (A9–A10), \({P}_{r}\) represents Prandtl number, \(Ri\) shows mixed convection, \(\Delta \) defines heat source, \({R}_{e}\) is Reynolds number and \({G}_{r}\) suggests Grashof number. In order to generalize the integer order Eqs. (4–5) for hybrid nanofluid into non-classical differentiations namely Caputo-Fabrizio differential operator36 and Atangana-Baleanu differential operator37, an energy and velocity Eqs. (4–5) can be transformed into fractionalized format with normalized functions as

$$\frac{{P}_{r}}{{\mathcal{Q}}_{2}}\left\{\frac{{\partial }^{\alpha }\theta \left(y,t\right)}{\partial {t}^{\alpha }}-{\mathcal{Q}}_{3}\Delta \theta \left(y,t\right)\right\}=\frac{{\partial }^{2}\theta \left(y,t\right)}{{\partial y}^{2}},$$
(7)
$$\frac{{P}_{r}}{{\mathcal{Q}}_{2}}\left\{\frac{{\partial }^{\beta }\theta \left(y,t\right)}{\partial {t}^{\beta }}-{\mathcal{Q}}_{3}\Delta \theta \left(y,t\right)\right\}=\frac{{\partial }^{2}\theta \left(y,t\right)}{{\partial y}^{2}},$$
(8)
$$\frac{1}{{Q}_{0}}\left(\frac{{\partial }^{\alpha }u\left(y,t\right)}{\partial {t}^{\alpha }}-{Q}_{1}Ri\theta \left(y,t\right)\right)=\frac{{\partial }^{2}u\left(y,t\right)}{{\partial y}^{2}},$$
(9)
$$\frac{1}{{Q}_{0}}\left(\frac{{\partial }^{\beta }u(y,t)}{\partial {t}^{\beta }}-{Q}_{1}Ri\theta (y,t)\right)=\frac{{\partial }^{2}u\left(y,t\right)}{{\partial y}^{2}}.$$
(10)

Here, \(\frac{{\partial }^{\alpha }}{\partial {t}^{\alpha }}\) and \(\frac{{\partial }^{\beta }}{\partial {t}^{\beta }}\) are non-classical differentiations namely Caputo-Fabrizio differential operator and Atangana-Baleanu differential operator have been defined in Appendix (A11–A12). The normalization functions for Appendix (A11–A12) are defined as \(M\left(0\right)=M\left(1\right)=M\left(\alpha \right)=1\) and \(M\left(0\right)=M\left(1\right)=M\left(\beta \right)=1\). The primary and foremost objective of non-classical differentiations is to assess a comprehensive analysis regarding the fractionalized heat transfer of flow of hybrid nanofluids with memory effects.

Analytic solutions of fractionalized energy equation of hybrid nanofluid

Equations (7–8) are the fractionalized energy equation of hybrid nanofluid which subjected to the imposed conditions, mentioned in Eq. (6). To determine the fractionalized temperature field from Eqs. (7–8) with functional differential operators of CF and AB via integral transform, we employ Fourier sine transform on Eqs. (7–8) as:

$${T}_{s}\left(\xi ,t\right)\left({D}_{t}^{\alpha }+\frac{{\mathcal{Q}}_{2}}{{P}_{r}}{\xi }^{2}-{\mathcal{Q}}_{3}\right)=\frac{{\mathcal{Q}}_{2}}{{P}_{r}}\sqrt{\frac{2}{\pi }}\xi t$$
(11)
$${T}_{s}\left(\xi ,t\right)\left({D}_{t}^{\beta }+\frac{{\mathcal{Q}}_{2}}{{P}_{r}}{\xi }^{2}-{\mathcal{Q}}_{3}\right)=\frac{{\mathcal{Q}}_{2}}{{P}_{r}}\sqrt{\frac{2}{\pi }}\xi t,$$
(12)

For the sake of elimination of time variable involved in Eq. (11–12), the fractionalized temperature field is tackled through Laplace transform, the resulting expressions are:

$$\overline{{T}_{s}}\left(\xi ,s\right)=\frac{\xi {A}_{0}}{{s}^{2}} \sqrt{\frac{2}{\pi }} \frac{\left(s+{\mathcal{Q}}_{5}\right)}{\left(s{\mathcal{Q}}_{6}-{\mathcal{Q}}_{7}\right)},$$
(13)
$$\overline{{T}_{s}}\left(\xi ,s\right)=\frac{\xi {A}_{0}}{{s}^{2}} \sqrt{\frac{2}{\pi }} \frac{\left({s}^{\beta }+{\mathcal{Q}}_{9}\right)}{\left({s}^{\beta }{\mathcal{Q}}_{10}-{\mathcal{Q}}_{11}\right)},$$
(14)

Here, the letting parameters for Eqs. (13–14) are expressed as \({\mathcal{Q}}_{4}=\frac{1}{1-\alpha }\), \({\mathcal{Q}}_{5}={\alpha \mathcal{Q}}_{4},\) \({\mathcal{Q}}_{6}={P}_{r}{\mathcal{Q}}_{4}+{A}_{0}{\xi }^{2}+{A}_{1}{P}_{r}\), \({\mathcal{Q}}_{7}={A}_{1}{P}_{r}{\mathcal{Q}}_{5}+{A}_{0}{\xi }^{2}{\mathcal{Q}}_{5},\) and \({\mathcal{Q}}_{8}=\frac{1}{1-\beta }\), \({\mathcal{Q}}_{9}=\beta {\mathcal{Q}}_{8},\) \({\mathcal{Q}}_{10}={P}_{r}\beta +{A}_{0}{\xi }^{2}-{A}_{1}{P}_{r},\) \({\mathcal{Q}}_{11}={{A}_{1}P}_{r}{\mathcal{Q}}_{9}+{A}_{0}{\xi }^{2}{\mathcal{Q}}_{9}\). Inverting Eqs. (13–14) through Fourier sine transform and writing them in the equivalent form, we get

$$\overline{T}\left(y,s\right)=\frac{1}{{s}^{2}}-\frac{2}{\pi }\underset{0}{\overset{\infty }{\int }}\left(\frac{{\mathcal{Q}}_{6}-{A}_{0}{\xi }^{2}}{{\mathcal{Q}}_{6}}\right)\frac{\mathit{sin}\left(y\xi \right)}{\xi }\left(\frac{s+{\mathcal{Q}}_{12}}{{s}^{2}\left(s+{\mathcal{Q}}_{13}\right)}\right)d\xi ,$$
(15)
$$\overline{T}\left(y,s\right)=\frac{1}{{s}^{2}}-\frac{2}{\pi }\underset{0}{\overset{\infty }{\int }}\left(\frac{{\mathcal{Q}}_{9}-{A}_{0}{\xi }^{2}}{{\mathcal{Q}}_{9}}\right)\frac{\mathit{sin}\left(y\xi \right)}{\xi }\left(\frac{{s}^{\beta }+{\mathcal{Q}}_{14}}{{s}^{2}\left({s}^{\beta }+{\mathcal{Q}}_{15}\right)}\right)d\xi ,$$
(16)

Equation (15–16) have been functionalized for parametric letting variables as: \({\mathcal{Q}}_{12}=\frac{{\xi }^{2}{A}_{0}{\mathcal{Q}}_{5}-{\mathcal{Q}}_{7}}{{\mathcal{Q}}_{6}-{A}_{0}},{\mathcal{Q}}_{13}=-\frac{{\mathcal{Q}}_{7}}{{\mathcal{Q}}_{6}},{\mathcal{Q}}_{14}=\frac{{\xi }^{2}{A}_{0}{\mathcal{Q}}_{9}-{\mathcal{Q}}_{11}}{{\mathcal{Q}}_{10}-{\xi }^{2}{A}_{0}} \mathrm{and }{\mathcal{Q}}_{15}=-\frac{{\mathcal{Q}}_{11}}{{\mathcal{Q}}_{10}}\). Inverting Eqs. (15–16) through Laplace transform and expressing them into special functions, we investigate the final solutions of fractionalized energy equation of hybrid nanofluid as:

$$T\left(y,t\right)=t-\frac{4}{{\pi }^{2}\sqrt{{\mathcal{Q}}_{13}t}}\underset{0}{\overset{\infty }{\int }}\left(\frac{{\mathcal{Q}}_{6}-{A}_{0}{\xi }^{2}}{{\mathcal{Q}}_{6}}\right)\frac{\mathit{sin}\left(y\xi \right)}{\xi }\underset{0}{\overset{\sqrt{{\mathcal{Q}}_{13}t}}{\int }}{e}^{{\tau }^{2}-{\mathcal{Q}}_{13}\left(t-\tau \right)}d\xi +{\mathcal{Q}}_{13}\underset{0}{\overset{t}{\int }}t{e}^{{\mathcal{Q}}_{13}\left(t-\tau \right)}d\tau .$$
(17)
$$ \begin{aligned} T\left( {y,t} \right) & = t - \frac{2}{\pi }\mathop \smallint \limits_{0}^{\infty } \left( {\frac{{{\mathcal{Q}}_{10} - A_{0} \xi^{2} }}{{{\mathcal{Q}}_{10} }}} \right)\frac{{\sin \left( {y\xi } \right)}}{\xi }\mathop \smallint \limits_{0}^{t} \delta_{{{\mathcal{Q}}_{15} }} \left( { - {\mathcal{Q}}_{15} t^{\alpha } } \right)d\tau d\xi - \frac{2}{\pi }\mathop \smallint \limits_{0}^{\infty } \left( {\frac{{{\mathcal{Q}}_{10} - A_{0} \xi^{2} }}{{{\mathcal{Q}}_{10} }}} \right)\frac{{\sin \left( {y\xi } \right)}}{\xi } \\ & \quad \times \,\mathop \smallint \limits_{0}^{t} t^{\alpha - 1} {\varvec{\delta}}_{\alpha ,\alpha } \left( { - {\mathcal{Q}}_{15} t^{\alpha } } \right)\left( {t - \tau } \right)d\tau d\xi . \\ \end{aligned} $$
(18)

Equations (17–18) are the generalized non-integer order analytical solutions based on hybrid nanofluid for energy. Such equations can satisfy the imposed initial and boundary conditions, they can be verified by employing \(t\to 0\) and \(y\to \infty \).

Analytic solutions of fractionalized velocity equation of hybrid nanofluid

Equations (9–10) are the fractionalized energy equation of hybrid nanofluid which subjected to the imposed conditions, mentioned in Eq. (6). To determine the fractionalized temperature field from Eqs. (9–10) with functional differential operators of CF and AB via integral transform, we employ Fourier sine transform on Eqs. (9–10) as:

$${u}_{s}\left(\xi ,t\right)\left({D}_{t}^{\alpha }+{\mathcal{Q}}_{0}{\xi }^{2}\right)-{\mathcal{Q}}_{1}{T}_{s}\left(\xi ,t\right)={\mathcal{Q}}_{0}\xi \sqrt{\frac{2}{\pi }} {u}_{0}{\mathbf{E}}_{\alpha }\left(at\right),$$
(19)
$${u}_{s}\left(\xi ,t\right)\left({D}_{t}^{\beta }+{\mathcal{Q}}_{0}{\xi }^{2}\right)-{\mathcal{Q}}_{1}{T}_{s}\left(\xi ,t\right)={\mathcal{Q}}_{0}\xi \sqrt{\frac{2}{\pi }} {u}_{0}{\mathbf{E}}_{\alpha }\left(at\right),$$
(20)

For the sake of elimination of time variable involved in Eq. (19–20), the fractionalized temperature field is tackled through Laplace transform, the resulting expressions are:

$$\overline{{u}_{s}}\left(\xi ,s\right)=\sqrt{\frac{2}{\pi }}\left(\frac{{u}_{0}{\mathcal{Q}}_{0}\xi \left(s+{\mathcal{Q}}_{1}\right){s}^{\alpha }}{s\left(s{\mathcal{Q}}_{6}+{\mathcal{Q}}_{0}{\xi }^{2}{\mathcal{Q}}_{1}\right)\left({s}^{\alpha }-a\right)}\right)+\left(\frac{{\mathcal{Q}}_{1}\left(s+{\mathcal{Q}}_{1}\right)}{s{\mathcal{Q}}_{6}+{\mathcal{Q}}_{0}{\xi }^{2}{\mathcal{Q}}_{1}}\right)\overline{{T}_{s}}\left(\xi ,s\right),$$
(21)
$$\overline{{u}_{s}}\left(\xi ,s\right)=\sqrt{\frac{2}{\pi }}\left(\frac{{u}_{0}{\mathcal{Q}}_{0}\xi \left({s}^{\alpha }+{\mathcal{Q}}_{10}\right){s}^{\alpha }}{s\left({s}^{\alpha }{\mathcal{Q}}_{15}+{\mathcal{Q}}_{0}{\xi }^{2}{\mathcal{Q}}_{10}\right)\left({s}^{\alpha }-a\right)}\right)+\left(\frac{{\mathcal{Q}}_{1}\left({s}^{\alpha }+{\mathcal{Q}}_{10}\right)}{{s}^{\alpha }{\mathcal{Q}}_{15}+{\mathcal{Q}}_{0}{\xi }^{2}{\mathcal{Q}}_{10}}\right)\overline{{T}_{s}}\left(\xi ,s\right),$$
(22)

Here, the letting parameters for Eqs. (13–14) are expressed as \({\mathcal{Q}}_{16}=\frac{2{\mathcal{Q}}_{0}{\mathcal{Q}}_{10}{\xi }^{2}}{{\mathcal{Q}}_{15}+{\mathcal{Q}}_{0}{\xi }^{2}} \mathrm{and} {\mathcal{Q}}_{17}=\frac{{\mathcal{Q}}_{0}{\xi }^{2}{\mathcal{Q}}_{10}}{{\mathcal{Q}}_{15}}\). Inverting Eqs. (21–22) through Fourier sine transform and writing them in the equivalent form, we get

$$ \begin{aligned} \overline{u}\left( {y,s} \right) & = \frac{{u_{0} s^{\alpha } }}{{s\left( {s^{\alpha } - a} \right)}} - \frac{{2u_{0} }}{\pi }\mathop \smallint \limits_{0}^{\infty } \left( {\frac{{{\mathcal{Q}}_{6} + {\mathcal{Q}}_{0} \xi^{2} }}{{{\mathcal{Q}}_{6} }}} \right)\frac{{\sin \left( {y\xi } \right)}}{\xi }\frac{{s^{\alpha } \left( {s + {\mathcal{Q}}_{7} } \right)}}{{s\left( {s^{\alpha } - a} \right)\left( {s + {\mathcal{Q}}_{8} } \right)}}d\xi \\ & \quad + \,\frac{2}{\pi }\mathop \smallint \limits_{0}^{\infty } \left( {\frac{{{\mathcal{Q}}_{2} {\mathcal{Q}}_{1} \xi }}{{{\mathcal{Q}}_{6} {\mathcal{Q}}_{2} }}} \right)\frac{{sin\left( {y\xi } \right)}}{\xi } \times \frac{{\left( {s + {\mathcal{Q}}_{1} } \right)^{2} }}{{s^{2} \left( {s + {\mathcal{Q}}_{9} } \right)\left( {s - {\mathcal{Q}}_{5} } \right)}}d\xi , \\ \end{aligned} $$
(23)
$$ \begin{aligned} \overline{u}\left( {y,s} \right) & = \frac{{u_{0} s^{\alpha } }}{{s\left( {s^{\alpha } - a} \right)}} - \frac{{2u_{0} }}{\pi }\mathop \smallint \limits_{0}^{\infty } \left( {\frac{{{\mathcal{Q}}_{15} + {\mathcal{Q}}_{0} \xi^{2} }}{{{\mathcal{Q}}_{15} }}} \right)\frac{{\sin \left( {y\xi } \right)}}{\xi }\frac{{\left( {s^{\alpha } + {\mathcal{Q}}_{16} } \right) d\xi }}{{s\left( {s^{\alpha } - a} \right)\left( {s^{\alpha } + {\mathcal{Q}}_{17} } \right)}} \\ & \quad + \,\frac{2}{\pi }\mathop \smallint \limits_{0}^{\infty } \left( {\frac{{{\mathcal{Q}}_{2} {\mathcal{Q}}_{1} \xi }}{{{\mathcal{Q}}_{11} {\mathcal{Q}}_{15} }}} \right)\frac{{\sin \left( {y\xi } \right)}}{\xi } \times \frac{{\left( {s^{\alpha } + {\mathcal{Q}}_{10} } \right)^{2} d\xi }}{{s^{2} \left( {s^{\alpha } + {\mathcal{Q}}_{14} } \right)\left( {s^{\alpha } + {\mathcal{Q}}_{17} } \right)}}, \\ \end{aligned} $$
(24)

Inverting Eqs. (23–24) through Laplace transform and expressing them into special functions, we investigate the final solutions of fractionalized energy equation of hybrid nanofluid as via invoked Appendix (A13–A17):

$$ \begin{aligned} u\left( {y,t} \right) & = u_{0} {\varvec{E}}_{\alpha } \left( {at} \right) + \frac{{2u_{0} }}{\pi }\mathop \smallint \limits_{0}^{\infty } \frac{{\sin \left( {y\xi } \right)}}{\xi }\left( {\frac{{{\mathcal{Q}}_{6} + {\mathcal{Q}}_{0} \xi^{2} }}{{{\mathcal{Q}}_{6} }}} \right)\mathop \smallint \limits_{0}^{t} \left\{ {t^{\alpha } {\varvec{\delta}}_{\alpha ,\alpha } \left( {at^{\alpha } } \right)\left( {t - \tau } \right)^{\alpha } {\varvec{\delta}}_{1,1 - \alpha } \left( { - {\mathcal{Q}}_{8} \left( {t - \tau } \right)} \right)} \right\}d\tau d\xi \\ & \quad + \frac{{2u_{0} }}{\pi }\mathop \smallint \limits_{0}^{\infty } \frac{{\sin \left( {y\xi } \right)}}{\xi }\left( {\frac{{{\mathcal{Q}}_{6} + {\mathcal{Q}}_{0} \xi^{2} }}{{{\mathcal{Q}}_{6} }}} \right)\mathop \smallint \limits_{0}^{t} \left\{ {e^{{ - {\mathcal{Q}}_{8} t}} {\varvec{\delta}}_{\alpha } \left( {a\left( {t - r} \right)^{\alpha } } \right)} \right\}drd\xi - \frac{2}{\pi }\mathop \smallint \limits_{0}^{\infty } \frac{{\sin \left( {y\xi } \right)}}{\xi }\left( {\frac{{{\mathcal{Q}}_{1} {\mathcal{Q}}_{0} {\mathcal{Q}}_{1} \xi }}{{{\mathcal{Q}}_{9} {\mathcal{Q}}_{6} {\mathcal{Q}}_{5} {\mathcal{Q}}_{2} }}} \right)d\xi \\ & \quad + \frac{2}{\pi }\mathop \smallint \limits_{0}^{\infty } \frac{{\sin \left( {y\xi } \right)}}{\xi }\left( {\frac{{{\mathcal{Q}}_{2} {\mathcal{Q}}_{1} \xi }}{{{\mathcal{Q}}_{6} {\mathcal{Q}}_{2} }}} \right)\left( {\frac{{2{\mathcal{Q}}_{1} {\mathcal{Q}}_{9} {\mathcal{Q}}_{5} - {\mathcal{Q}}_{1} \left( {{\mathcal{Q}}_{9} - {\mathcal{Q}}_{5} } \right)}}{{{\mathcal{Q}}_{9}^{2} {\mathcal{Q}}_{5}^{2} }}} \right)d\xi - \frac{2}{\pi }\mathop \smallint \limits_{0}^{\infty } \frac{{\sin \left( {y\xi } \right)}}{\xi }\left( {\frac{{{\mathcal{Q}}_{2} {\mathcal{Q}}_{1} \xi }}{{{\mathcal{Q}}_{6} {\mathcal{Q}}_{2} }}} \right)e^{{ - {\mathcal{Q}}_{9} t}} \\ & \quad \times \left( {\frac{{{\mathcal{Q}}_{9}^{2} - 2{\mathcal{Q}}_{1} {\mathcal{Q}}_{9} + {\mathcal{Q}}_{1} }}{{{\mathcal{Q}}_{9}^{2} \left( { - {\mathcal{Q}}_{5} - {\mathcal{Q}}_{9} } \right)}}} \right)d\xi - \frac{2}{\pi }\mathop \smallint \limits_{0}^{\infty } \frac{{\sin \left( {y\xi } \right)}}{\xi }\left( {\frac{{{\mathcal{Q}}_{2} {\mathcal{Q}}_{1} \xi }}{{{\mathcal{Q}}_{6} {\mathcal{Q}}_{2} }}} \right)\left( {\frac{{{\mathcal{Q}}_{5}^{2} + 2{\mathcal{Q}}_{1} {\mathcal{Q}}_{5} + {\mathcal{Q}}_{1} }}{{{\mathcal{Q}}_{5}^{2} \left( {{\mathcal{Q}}_{9} + {\mathcal{Q}}_{5} } \right) }}} \right)e^{{{\mathcal{Q}}_{5} t}} d\xi , \\ \end{aligned} $$
(25)
$$ \begin{aligned} u\left( {y,t} \right) & = u_{0} {\varvec{E}}_{\alpha } \left( {at} \right) - \frac{{2u_{0} }}{\pi }\mathop \smallint \limits_{0}^{\infty } \frac{{\sin \left( {y\xi } \right)}}{\xi }\left( {\frac{{{\mathcal{Q}}_{15} + {\mathcal{Q}}_{0} \xi^{2} }}{{{\mathcal{Q}}_{15} }}} \right)\mathop \smallint \limits_{0}^{t} \left\{ {t^{\alpha - 1} {\varvec{\delta}}_{\alpha ,\alpha } \left( { - {\mathcal{Q}}_{17} t^{\alpha } } \right){\varvec{\delta}}_{\alpha } \left( {a\left( {t - \tau } \right)} \right)} \right\}d\tau d\xi \\ & \quad + \frac{{2u_{0} }}{\pi }\mathop \smallint \limits_{0}^{\infty } \frac{{\sin \left( {y\xi } \right)}}{\xi }\left( {\frac{{{\mathcal{Q}}_{16} }}{{{\mathcal{Q}}_{17} }}} \right)\left( {\frac{{{\mathcal{Q}}_{15} + {\mathcal{Q}}_{0} \xi^{2} }}{{{\mathcal{Q}}_{15} }}} \right)\mathop \smallint \limits_{0}^{t} \left\{ {1 - {\varvec{\delta}}_{\alpha } \left( { - {\mathcal{Q}}_{17} t^{\alpha } } \right)\left( {t - \tau } \right)^{\alpha - 1} {\varvec{\delta}}_{\alpha ,\alpha } \left( {a\left( {t - \tau } \right)^{\alpha } } \right)} \right\} d\tau d\xi \\ & \quad + \frac{2}{\pi }\mathop \smallint \limits_{0}^{\infty } \frac{{\sin \left( {y\xi } \right)}}{\xi }\left( {\frac{{{\mathcal{Q}}_{2} {\mathcal{Q}}_{1} \xi }}{{{\mathcal{Q}}_{11} {\mathcal{Q}}_{15} }}} \right)\mathop \sum \limits_{n = 0}^{\infty } \mathop \sum \limits_{m = 0}^{\infty } \frac{{\left( { - {\mathcal{Q}}_{14} } \right)^{n} }}{{\left( { - {\mathcal{Q}}_{17} } \right)^{ - m} }}\frac{{t^{1 + \alpha n + \alpha m} }}{{\Gamma \left( {2 + \alpha n + \alpha m} \right)}}d\xi + \frac{4}{\pi }\mathop \smallint \limits_{0}^{\infty } \frac{{\sin \left( {y\xi } \right)}}{\xi }\left( {\frac{{{\mathcal{Q}}_{10} {\mathcal{Q}}_{2} {\mathcal{Q}}_{1} \xi }}{{{\mathcal{Q}}_{11} {\mathcal{Q}}_{15} }}} \right) \\ & \quad \times \mathop \sum \limits_{n = 0}^{\infty } \mathop \sum \limits_{m = 0}^{\infty } \frac{{\left( { - {\mathcal{Q}}_{14} } \right)^{n} }}{{\left( { - {\mathcal{Q}}_{17} } \right)^{ - m} }}\frac{{s^{1 + \alpha + \alpha n + \alpha m} }}{{\Gamma \left( {2 + \alpha + \alpha n + \alpha m} \right)}}d\xi + \frac{2}{\pi }\mathop \smallint \limits_{0}^{\infty } \frac{{\sin \left( {y\xi } \right)}}{\xi }\left( {\frac{{{\mathcal{Q}}_{10}^{2} {\mathcal{Q}}_{2} {\mathcal{Q}}_{1} \xi }}{{{\mathcal{Q}}_{11} {\mathcal{Q}}_{15} }}} \right)\mathop \sum \limits_{n = 0}^{\infty } \mathop \sum \limits_{m = 0}^{\infty } \frac{{\left( { - {\mathcal{Q}}_{14} } \right)^{n} }}{{\left( { - {\mathcal{Q}}_{17} } \right)^{ - m} }} \\ & \quad \times \frac{{t^{1 + 2\alpha + \alpha n + \alpha m} }}{{\Gamma \left( {2 + 2\alpha + \alpha n + \alpha m} \right)}}d\xi . \\ \end{aligned} $$
(26)

Equations (25–26) are the generalized non-integer order analytical solutions based on hybrid nanofluid for velocity field. Such equations can satisfy the imposed initial and boundary conditions, they can be verified by employing \(t\to 0\) and \(y\to \infty \).

Numerical results and discussion

This section is dedicatedly written for the significant role of nanoparticles (copper and alumina) and base fluid ethylene glycol. Ethylene glycol is often used in the industry for the processing of nanofluids because it is highly viscous and accessible. Here, hybrid nanofluid is modeled via modern fractional definitions of differentiations and the mathematical models of energy and momentum are simplified by Laplace and Fourier Sine transforms. The comparative analysis of heat transfer and velocity profile from hybrid nanofluid have been observed through Caputo-Fabrizio and Atangana-Baleanu differential operators. The results mentioned below for heat transfer and velocity profile have captured a comparative analysis between the dynamics of ethylene glycol conveying alumina-copper nanoparticles.

Physical significance of volume fraction

It is established fact that when materials of similar dynamical characteristics are combined then volume fraction differences differ from the individual volumes of the mixture. In this context, temperature distribution has been investigated via modern fractional differential operators in Fig. 1 in which it is observed that increasing rate of volume fraction, the temperature distribution has decelerated trends through both differential techniques of fractional calculus. A selective range of volume fractions is sketched in Figs. 2 and 3 and numerically investigated for exceptional heat transfer to improve the effective thermal conductivity and thermal diffusivity of nanoparticle suspensions in base fluids. Additionally, Figs. 2 and 3 are prepared for contour plot and three-dimensional graphs for volume fraction of hybrid nanofluid respectively. It is observed that reciprocal behavior of temperature distribution is perceived for hybrid nanofluid.

Figure 1
figure 1

Variation of volume fraction for two-dimensional temperature distribution through AB-differential operator verses CF-differential operator.

Full size image
Figure 2
figure 2

Variation of volume fraction for contour temperature distribution through AB-differential operator verses CF-differential operator.

Full size image
Figure 3
figure 3

Variation of volume fraction for three-dimensional temperature distribution through AB-differential operator verses CF-differential operator.

Full size image

Physical significance of heat source parameter

It is necessary to calculate the temperature distribution within the hybrid nanofluid when heat sources are present in the hybrid nanofluid. This is because, heat source is an important factor in the hybrid nanofluid for enhancing the reactivity of hybrid nanofluid. In order to signify the effects of heat source at \(\Delta = 4.5,5.5, 6.0,6.5\), temperature distribution of hybrid nanofluid is depicted in Fig. 4 via two types of fractional differentiations. Here, it is perceived that enhancing the heat source raises heat transfer through both fractional techniques within sufficient uniformity. In short, from physical aspects, fractional techniques have envisaged the temperature distribution subject to the employment of heat source in symmetry.

Figure 4
figure 4

Variation of heat source for two-dimensional velocity profile through AB-differential operator verses CF-differential operator.

Full size image

Physical significance of Grashof number

There is no denying fact that the Grashof number is a way to quantify the opposing forces. The profile of velocity is observed through both differential techniques of fractional calculus when Grashof number is varying at \(Gr = 5,10,15,20\) in Fig. 5 for hybrid nanofluid. In is noticed from Fig. 5 that increasing Grashof number decreases velocity profile in free convection system. Physically, the larger values of Grashof number have reflected in which buoyant forces overcome the viscous forces. Hence, the flow starts a transition to the turbulent regime for hybrid nanofluid.

Figure 5
figure 5

Variation of Grashof number for two-dimensional velocity profile through AB-differential operator verses CF-differential operator.

Full size image

Physical significance of Reynold number

For flow from hybrid nanofluid over a flat plate, if Reynolds number is larger then inertia forces are in command. While viscous forces dominate the boundary layer when the Reynolds number is smaller. Keeping this fact for hybrid nanofluid, velocity field is sketched in Fig. 6 at the smaller values of Reynolds number via local and non-local kernel approach of differential operators. It is explored that velocity field raises when Reynolds number is increased. From practical point of view, the transport properties of hybrid nanofluid reacted to a variation in fluids from gases to liquids.

Figure 6
figure 6

Variation of Reynold number for two-dimensional velocity profile through AB-differential operator verses CF-differential operator.

Full size image

Physical significance of suspension of nanoparticles in ethylene glycol

Due to extraordinary performance and high conductivity of copper and alumina nanoparticles in comparison with other nanoparticles, the copper and alumina nanoparticles are suspended in ethylene glycol. Copper nanoparticles may have similar principle of action as that of alumina nanoparticles; however, the precise mechanism regarding the suspension of both types of nanoparticles can cause viability and critical activity against the suspension of copper and alumina nanoparticles in ethylene glycol. Figure 7 is prepared for the profile of velocity for suspension of copper and alumina nanoparticles in ethylene glycol through Atangana-Baleanu and Caputo-Fabrizio fractional differential operators. Here, the comparative analysis of suspension of copper and alumina nanoparticles in ethylene glycol for velocity field is observed at three different times via both types of fractional differential operators. It is examined that ethylene glycol-alumina nanoparticles have faster velocity profile via both fractional differential operators at smaller time \(t = 5 {\text{seconds}}\) in comparison with ethylene glycol-copper nanoparticles. While it is perceived that ethylene glycol-copper nanoparticles have faster velocity profile via Atangana-Baleanu fractional differential operator at lager time \(t = 300 \,{\text{s}}\) in comparison with ethylene glycol-alumina nanoparticles. To conclude, identical trends of velocity profile is recognized for copper and alumina nanoparticles suspended in ethylene glycol at unit time \(t = 60\, {\text{s}}\). For the sake of simplicity, the similar observation can also be investigated for temperature distribution.

Figure 7
figure 7

Comparison of copper and alumina nanoparticles suspended in ethylene-glycol for two-dimensional velocity profile through AB-differential operator verses CF-differential operator at three different times.

Full size image

Conclusion

A new fractionalized model based on hybrid nanofluid is proposed and investigated by employing singular verses and non-singular kernels. The mathematical modeling of hybrid nanofluid is handled by invoking modern fractional definitions of differentiations. The combined Laplace and Fourier Sine transforms have been configurated on the governing equations of temperature and velocity of hybrid nanofluid. The analytical expression of the governing temperature and velocity equations of hybrid nanofluid have been solved via special functions. In brevity, following outcomes have been underlined as:

  • Exact analytical solutions by invoking generalized non-integer order fractional differential operator have been investigated from basic equations of hybrid nanofluid. The investigated exact analytical solutions of temperature distribution [Eqs. (17–18)] and velocity field [Eqs. (25–26)] are stable for the proposed parametric values of fractional differential operators within the range \(0 < \alpha ,\beta \le 1\).

  • Increasing rate of volume fraction resulted the decelerated and accelerated trends of temperature distribution via Caputo-Fabrizio and Atangana-Baleanu fractional differential operators respectively.

  • Temperature distribution of hybrid nanofluid is perceived by enhancing the heat source that raises heat transfer through both fractional techniques within sufficient uniformity.

  • The larger values of Grashof number have reflected that buoyant forces overcome the viscous forces.

  • Velocity field raises when Reynolds number is increased.

  • The ethylene glycol-alumina nanoparticles have faster velocity profile via both fractional differential operators at smaller time \(t = 5 \,{\text{s}}\) and ethylene glycol-copper nanoparticles have faster velocity profile via Atangana-Baleanu fractional differential operator at lager time \(t = 300 \,{\text{s}}\).