3D MHD nonlinear radiative flow of CuO-MgO/methanol hybrid nanofluid beyond an irregular dimension surface with slip effect

Abstract

The 3D MHD nonlinear radiative hybrid nanofluid flow across an irregular dimension sheet with slip effect is studied numerically. The hybrid nanofluid consists of copper oxide (CuO) and magnesium oxide (MgO) nanoparticles embedded in methanol or methyl alcohol (MA). The governing PDEs’ are altered as ODEs’ using similarities and numerical solutions are attained using shooting scheme. The role of corporal factors on the transport phenomenon is analyzed and reflected by plots and numerical interpretations. Simultaneous solutions presented for CuO-MA nanofluid and CuO-MgO/MA hybrid nanofluid. Results ascertain that the temperature and flow boundary layer thicknesses are not unique for the hybrid nanofluid and nanofluid. The heat transfer enactment of CuO-MA nanofluid is high when equated to CuO-MgO/MA hybrid nanofluid. This concludes that the CuO-MgO combination works as a good insulator.

Introduction

The novelty of discoveries in heat transport to stimulate the efficiency of hybrid nanofluid with several assortments of conductive nanoparticles has wide applications in engineering and technology. There is a tiny evolution for hybrid nanofluid studies in recent days. In 1995, the nanofluids were developed and got changes in the efficiency of heat transfer drastically¹. Generally, the nanofluid is a synthesis of mono-nanoparticle in base liquid to promote superior thermal liquids. The crises of cooling demand can be cracked by evolving nanofluid were reported by Kumar et al.². The advancement in nanofluid is a hybrid-nanofluid to enrich more heat transfer. The fusion of two or more than two nanoparticles in a base liquid will get a hybrid nanofluid³. Several scholars have pointed out that the assets of hybrid nanofluid are greater as associated with nanofluid^4,5,6. These fluids are mostly used in renewable energy, emollients, air conditioning, microelectronics, etc⁷.. Yarmand et al.⁸ deliberate the fusion of platinum hybrid nanofluids and clarified its physical properties and stability. The pressure drop of these solutions is smaller than that of nanofluid or any base liquid, so that it has auspicious applications that were suggested by Huang et al.⁹.

Later on, Labib et al.¹⁰ considered the CNT-Al2O3 hybrid nanofluid and originated the mix of Al2O3-CNT nanoparticle in base-liquid to improve the convection as equated to Al2O3-nanoliquid. Waini et al.¹¹ discussed the unsteady flow above a widening area and established the stability of the heat transfer of hybrid nanofluid, which is depending on the miscellany of the nanoparticle. Ma et al.¹² stated the bearings of MHD on hybrid nanofluid in a channel with powerful heater and cooler walls. They invented that heat transmission is more active in the heater wall as related to the cooler wall. Sheikholeslami et al.¹³ considered the heat transmission of magnetizable hybrid nanofluid in a circular cavity and theoretically showed that the strength of magnetic fields located in circular heaters and MWCNT-Fe3O4 with base liquid would maximize the heat transfer rate. The hybrid nanofluid past a spongy medium was described by Mehryan et al.¹⁴. They found that the sponginess ratio will augment the convective flow inside the cavity. Acharya et al.¹⁵ exhibited the effects of thermodiffusion on hybrid-nanofluid past a spinning disk and initiated that the radiation factor was escalations the heat transfer rate. Using the lattice Boltzmann method, Reza and Shahriari¹⁶ examine the assets of the magnetic field in existence of Rayleigh number and pointed out that Hartmann number digresses in the absences of Rayleigh number.

The heat transport in the flow over an elongated sheet has widely used in manufacturing process, polymer extrusion, paper fabrication, hot rolling process, aeronautical, civil and marine engineering, etc. Moreover, the consideration of variable thickness is to develop an essential and consistent design. Fang et al.¹⁷ explored the importance of variable thickness geometry. Khader and Megahed¹⁸ examined the boundary layer movement over a nonlinear elongating plate and found that the heat rate enriches due to movable thickness. Khan et al.¹⁹ studied Carreau nanofluid over an elongating sheet, solved numerically under some influences of thermal radiation and movable thickness property. The behavior of the movement of the heat of dusty nanofluids over an extending superficial was considered by Sandeep et al.²⁰. Hayat et al.²¹ studied the boundary layer flow with homogeneous-heterogeneous reaction and found that the property of movable thickness indicates acceleration in flow rate. The 3-D slip flow above an elongating sheet with cross-diffusion was adopted by²² and elucidated that the uneven thickness stretching sheet enhances the energy. The authors^23,24 considered the MHD flow across a slender surface and moving the needle and examined the transport phenomena.

Furthermore, MHD effects are used in metal casting, metallurgy, the invention of medicine, extraction of geothermal energy, polymer industry, melting reactors, fusion reactor, etc. Sulochana et al.²⁵ measured the flow over a revolving cone with magnetic and Soret effects. Usman et al.²⁶ examines heat flow assets of Al2O3-Cu-hybrid nanofluid with magnetic and radiation effects. Nayak et al.²⁷ exposed that the existence of MHD slowdowns the fluid motion and escalations of thermal profile. Khan et al.²⁸ explained the rotating flow of hybrid nanofluid under magnetic effects. Reddy et al.²⁹ analyzed the MHD nanofluid flow through the spongy passage by the perturbation technique. Samrat et al.³⁰ scrutinized an unsteady flow of Casson fluid under the magnetic and radiation effects over an elongating surface. Conduct of hybrid nanofluid in a wavering upright channel with hall current was scrutinized by Iqbal et al.³¹. Recently, the researchers^32,33,34, numerically investigated the convective heat transfer in magnetohydrodynamic flows past a stretched surface with non-uniform thickness in the presence of various physical effects. The impact of Lorentz force and slip effect on free convection flow past a annulus was numerically investigated by the researchers^35,36,37. Further, Jagadeesha et al.³⁸ studied the effect of magnetic field on the flow through a porous enclosure. Later on, the researchers^39,40 discussed the influence of buoyancy and magnetic field on electrically conducting fluid past a vertical annulus.

By keeping the above developments in view, we portrayed the convective heat transmission in hybrid nanofluid drift over the variable dimension elongating sheet. For this, we considered the magnetohydrodynamic nonlinear radiative CuO-MgO/MA hybrid nanofluid with velocity slip and temperature jump. Numerical solutions are attained using shooting scheme. The role of corporeal factors on the transport phenomenon is analyzed and reflected by plots and numerical interpretations. Simultaneous solutions are presented for CuO-MA nanofluid and CuO-MgO/MA hybrid nanofluid.

Mathematical Formulation

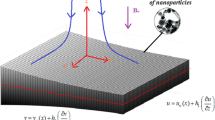
A steady, 3-D electrically conducting magnetohydrodynamic flow of CuO-MgO/MA hybrid nanofluid past an extended surface of non-uniform thickness is considered. The sheet of non-uniform thickness is considered as $z=A{\delta }^{(1-n)/2},\,\delta =x+y+c,n\ne 1$ we have chosen A is small. It is assumed that the surface is stretched along the xy-plane while fluid is placed along the $z$-axis. It is also presumed, the sheet temperature as ${T}_{w}={T}_{0}{\delta }^{\frac{1-n}{2}}+{T}_{\infty }$. The magnetic field of strength $B$ is applied in parallel with the $z-$ axis as revealed in Fig. 1.

Figure 1

Schematic Model.

With conventions made above, the governing equations can be expressed as:^11,17,18,19

$${u}_{x}+{v}_{y}+{w}_{z}=0,$$

(1)

$${\rho }_{hnf}(u{u}_{x}+v{u}_{y}+w{u}_{z})={\mu }_{hnf}{u}_{zz}-{\sigma }_{hnf}{B}^{2}u,$$

(2)

$${\rho }_{hnf}(u{v}_{x}+v{v}_{y}+w{v}_{z})={\mu }_{hnf}{v}_{zz}-{\sigma }_{hnf}{B}^{2}v,$$

(3)

$${\rho }_{hnf}(u{T}_{x}+v{T}_{y}+w{T}_{z})={\mu }_{hnf}{T}_{zz}-{({q}_{r})}_{z},$$

(4)

with the frontier situations

$$\begin{array}{c}u-{u}_{w}={h}_{1}^{\ast }\left(\frac{\partial u}{\partial z}\right),v-{v}_{w}={h}_{1}^{\ast }\left(\frac{\partial v}{\partial z}\right),\\ T-{T}_{w}-{h}_{2}^{\ast }\left(\frac{\partial T}{\partial z}\right)=0,z=A{\delta }^{(1-n)/2},n\ne 1\\ and\,u(\infty )\to 0,v(\infty )\to 0,T(\infty )\to {T}_{\infty }\end{array}\}$$

(5)

where u,v are velocity components in x and y directions, ${u}_{w},{v}_{w}$ are velocities near surface, ${h}_{1}^{\ast },{h}_{2}^{\ast }$ are dimensional velocity slip and temperature jump parameters, ${T}_{W}$ is the wall temperature^32,33,34

$$\begin{array}{c}{\zeta }_{1}=\frac{{k}_{B}T}{\sqrt{2}\pi {d}^{2}p},\delta =x+y+c,{h}_{1}^{\ast }=\left[\frac{2-{f}_{1}}{{f}_{1}}\right]{\zeta }_{1}{\delta }^{\frac{1-n}{2}},\\ {\zeta }_{2}=\left(\frac{2\gamma }{\gamma +1}\right)\frac{{\zeta }_{1}}{\Pr },{h}_{2}^{\ast }=\left[\frac{2-b}{b}\right]{\zeta }_{2}{\delta }^{\frac{1-n}{2}},B={B}_{0}{\delta }^{\frac{n-1}{2}},\end{array}\}$$

(6)

$${u}_{w}=a{\delta }^{\frac{n-1}{2}},{v}_{w}=a{\delta }^{n},{T}_{w}-{T}_{\infty }={T}_{0}{\delta }^{\frac{1-n}{2}},{\rm{for}}\,n\ne 1$$

(7)

By Rosseland approximation, the heat flux is given by

$${q}_{r}=-\frac{4{\sigma }^{\ast }}{3{k}^{\ast }}\left(\frac{\partial {T}^{4}}{\partial z}\right)$$

(8)

after expanding T⁴ through Taylor series about T_∞ and neglecting higher-order terms and considering ${\theta }_{w}=\frac{{T}_{w}}{{T}_{\infty }}$, the resulting similarity variable of energy becomes

$$T={T}_{\infty }[1+({\theta }_{w}-1)\theta ]$$

(9)

The above novel form is involved for the parameterization of the nonlinear radiation term apparent in the energy equation. Where ${\sigma }^{\ast },{k}^{\ast }$ denotes the Stefan-Boltzmann constant and mean absorption coefficient.

The hybrid nanofluid parameters can be used as:¹¹

$$\begin{array}{c}\frac{{k}_{hnf}}{{k}_{f}}=\frac{{k}_{2s}+2{k}_{f}-2{\phi }_{2}({k}_{f}-{k}_{2s})}{{k}_{2s}+2{k}_{f}+{\phi }_{2}({k}_{f}-{k}_{2s})}\times \frac{{k}_{1s}+2{k}_{f}-2{\phi }_{1}({k}_{f}-{k}_{1s})}{{k}_{1s}+2{k}_{f}+{\phi }_{1}({k}_{f}-{k}_{1s})},\\ \frac{{\rho }_{hnf}}{{\rho }_{f}}=(1-{\phi }_{2})\left[(1-{\phi }_{1})+\frac{{\phi }_{1}{\rho }_{1s}}{{\rho }_{f}}\right]+\frac{{\phi }_{2}{\rho }_{2s}}{{\rho }_{f}},\phi ={\phi }_{1}+{\phi }_{2},\\ \frac{{(\rho {c}_{p})}_{hnf}}{{(\rho {c}_{p})}_{f}}=(1-{\phi }_{2})\left[(1-{\phi }_{1})+\frac{{\phi }_{1}{(\rho {c}_{p})}_{1s}}{{(\rho {c}_{p})}_{f}}\right]+\frac{{\phi }_{2}{(\rho {c}_{p})}_{2s}}{{(\rho {c}_{p})}_{f}},\\ \frac{{\mu }_{hnf}}{{\mu }_{f}}=\frac{1}{{(1-{\phi }_{1})}^{2.5}{(1-{\phi }_{2})}^{2.5}},\frac{{\sigma }_{hnf}}{{\sigma }_{f}}=\left[1+\frac{3({\sigma }_{1s}{\phi }_{1s}-\phi {\sigma }_{f})+{\phi }_{2s}{\sigma }_{2s}}{{\sigma }_{1s}(1-{\phi }_{1s})+{\sigma }_{2s}(1-{\phi }_{2s})+(2+\phi ){\sigma }_{f}}\right],\end{array}\}$$

(10)

where ϕ₁, ϕ₂ denotes the CuO and MgO nanoparticle volume fractions. The following similarity transformations are used for non-dimensionalization³³

$$\begin{array}{c}\eta =z{\left(\frac{(n+1)a}{2\upsilon }\right)}^{1/2}{\delta }^{(n-1)/2},\theta =(T-{T}_{\infty })/({T}_{w}-{T}_{\infty }),\\ u=a{\delta }^{n}f{\prime} (\eta ),v=a{\delta }^{n}g{\prime} (\eta )\\ w=-{\left(\frac{2a\nu }{n+1}\right)}^{0.5}{\delta }^{(n-1)0.5}\left[\frac{n+1}{2}(f+g)+\eta \left(\frac{n-1}{2}\right)(f{\prime} +g{\prime} )\right]\end{array}\}$$

(11)

by making use of Eqs. (6–9), the Eqs. (1–5) can be transformed as

$$\begin{array}{c}\frac{n+1}{2{(1-{\phi }_{1})}^{2.5}{(1-{\phi }_{2})}^{2.5}}f\prime\prime\prime -\left(1+\frac{3({\sigma }_{1s}{\phi }_{1s}-\phi {\sigma }_{f})+{\phi }_{2s}{\sigma }_{2s}}{{\sigma }_{1s}(1-{\phi }_{1s})+{\sigma }_{2s}(1-{\phi }_{2s})+(2+\phi ){\sigma }_{f}}\right)Mf{\prime} \\ -\left((1-{\phi }_{2})\left((1-{\phi }_{1})+\frac{{\phi }_{1}{\rho }_{1s}}{{\rho }_{f}}\right)+\frac{{\phi }_{2}{\rho }_{2s}}{{\rho }_{f}}\right)\left(n{(f{\prime} )}^{2}+nf{\prime} g{\prime} -\frac{n+1}{2}(f+g)f{\prime\prime} \right)=0,\end{array}\}$$

(12)

$$\begin{array}{c}\frac{n+1}{2{(1-{\phi }_{1})}^{2.5}{(1-{\phi }_{2})}^{2.5}}g\prime\prime\prime -\left(1+\frac{3({\sigma }_{1s}{\phi }_{1s}-\phi {\sigma }_{f})+{\phi }_{2s}{\sigma }_{2s}}{{\sigma }_{1s}(1-{\phi }_{1s})+{\sigma }_{2s}(1-{\phi }_{2s})+(2+\phi ){\sigma }_{f}}\right)Mg{\prime} \\ -\left((1-{\phi }_{2})\left((1-{\phi }_{1})+\frac{{\phi }_{1}{\rho }_{1s}}{{\rho }_{f}}\right)+\frac{{\phi }_{2}{\rho }_{2s}}{{\rho }_{f}}\right)\left(n{(g{\prime} )}^{2}+nf{\prime} g{\prime} -\frac{n+1}{2}(f+g)g{\prime} \right)=0,\end{array}\}$$

(13)

$$\begin{array}{c}\left(\begin{array}{c}\left(\frac{{k}_{2s}+2{k}_{f}-2{\phi }_{2}({k}_{f}-{k}_{2s})}{{k}_{2s}+2{k}_{f}+{\phi }_{2}({k}_{f}-{k}_{2s})}\right)\left(\frac{{k}_{1s}+2{k}_{f}-2{\phi }_{1}({k}_{f}-{k}_{1s})}{{k}_{1s}+2{k}_{f}+{\phi }_{1}({k}_{f}-{k}_{1s})}\right)\theta {\prime\prime} +R{(1+({\theta }_{w}-1)\theta )}^{3}\theta {\prime\prime} +\\ \,3R{(1+({\theta }_{w}-1)\theta )}^{2}({\theta }_{w}-1){\theta {\prime} }^{2}\end{array}\right)\\ \,-\frac{2\Pr }{n+1}\left((1-{\phi }_{2})\left[(1-{\phi }_{1})+\frac{{\phi }_{1}{(\rho {c}_{p})}_{1s}}{{(\rho {c}_{p})}_{f}}\right]+\frac{{\phi }_{2}{(\rho {c}_{p})}_{2s}}{{(\rho {c}_{p})}_{f}}\right)\left(\frac{1-n}{2}(f{\prime} +g{\prime} )\theta -\frac{n+1}{2}(f+g)\theta {\prime} \right)=0,\end{array}\}$$

(14)

the transmuted boundary restrictions are

$$\begin{array}{c}f(0)=\lambda \left(\frac{1-n}{n+1}\right)[1+{h}_{1}f{\prime\prime} (0)],f{\prime} (0)=[1+{h}_{1}f{\prime\prime} (0)],\\ g(0)=\lambda \left(\frac{1-n}{n+1}\right)[1+{h}_{1}g{\prime\prime} {(\eta )}_{\eta =0}],\theta (0)=[1+{h}_{2}\theta {\prime} (0)],\\ g{\prime} (0)=[1+{h}_{1}g{\prime\prime} (0)],f{\prime} {(\eta )}_{\eta \to \infty }=0,g{\prime} {(\eta )}_{\eta \to \infty }=0,\theta {(\eta )}_{\eta \to \infty }=0,\end{array}\}$$

(15)

where

$$M=\frac{{\sigma }_{f}{B}_{0}^{2}}{{\rho }_{f}a},\Pr =\frac{{\mu }_{f}{({c}_{p})}_{f}}{{k}_{f}}$$

(16)

are the magnetic field parameter and Prandtl number and $\lambda$ is wall thickness parameter.

For engineering curiosity the ${C}_{f}$ and $N{u}_{x}$ are defined as²²

$${C}_{f}=2\frac{{\mu }_{hnf}}{{\mu }_{f}\sqrt{\mathrm{Re}}}{\left(\frac{n+1}{2}\right)}^{0.5}{f{\prime\prime} |}_{\eta =0},N{u}_{x}=-\sqrt{\mathrm{Re}}(1+R{\theta }_{w})\frac{{k}_{hnf}}{{k}_{f}}{\left(\frac{n+1}{2}\right)}^{0.5}{\theta {\prime} |}_{\eta =0}\}$$

(17)

where $\mathrm{Re}=\frac{{u}_{w}\delta }{{\upsilon }_{f}},$

Discussion of the results

The system of Eqs. (12–14) are solved by shooting scheme under the limitations of Eq. (15). We discussed the eccentricities of velocity and temperature with impacts of non-dimensional parameters through graphs. The velocity power index n, thermal radiation R, temperature ratio ${\theta }_{w}$, wall thickness $\lambda$, velocity slip ${h}_{1}$, temperature jump ${h}_{2}$, and nanoparticle volume fraction $\phi (={\phi }_{1}+{\phi }_{2}),{\phi }_{1}$ parameters are used in this study. Also, the friction factor and Nusselt number are scrutinized with the above said parameters through the table. The physical parametric values are set to $M=1,n=0.7,{h}_{1}=0.4,{\theta }_{w}=0.3,R=1,{h}_{2}=0.4,\lambda =0.1,\Pr =7.38$ in order to attain the required results. These values are invariant unless the variations shown in the respective figures and tables. Table 1 predicts the thermo physical properties. Table 2 displays the authentication of the results. Tables 3 and 4 discuss the impact of pertinent parameters on flow and heat rate. The heat flow rate uplift and contradictory is seen in friction factor due to the variation in $\lambda$. Nusselt number is drastically reducing for rising values of thermal radiation. The same trend has been observed for boosting values of magnetic field and slip parameters.

Table 1 Thermo physical properties.

Table 2 Validation of the results for $f\text{'}\text{'}(0)$ (2D case-water with ϕ = 0) for various values of $\lambda$ and ${h}_{1}$ in the absence of radiation.

Table 3 Effects of non-dimensional quantities on f″(0),–θ′(0) for Methanol+CuO+MgO.

Table 4 Effects of non-dimensional quantities on f″(0),–θ′(0) for Methanol+CuO.

The outcome of $\lambda$ on $f\text{'}(\eta ),g\text{'}(\eta )$ and $\theta (\eta )$ profiles are displayed in Figs. 2–4. The lessening of all the profiles has perceived by increase in $\lambda$. Generally, for escalations in wall thickness oppose the velocity. Temperature shrinks due to the transmission of heated particles is less in a denser area. The variation of $R$ on $\theta (\eta )$ is revealed in Fig. 5. It is clear that, thermal profile boosts by raising the thermal radiation. The growth in thermal profile is observed for escalations in $R$ which represents aid of an external source leads to rise in temperature.

Figure 2

Variation of $\lambda$ on $f\text{'}(\eta )$.

Figure 3

Variation of $\lambda$ on $g\text{'}(\eta )$.

Figure 4

Variation of $\lambda$ on $\theta (\eta )$.

Figure 5

Variation of $R$ on $\theta (\eta )$.

The impact of ${\theta }_{w}$ on $\theta (\eta )$ is showed in Fig. 6. It illustrates that the thermal profile increases by evolving ${\theta }_{w}$. The variation of $n$ over $f\text{'}(\eta ),g\text{'}(\eta )$ and $\theta (\eta )$ profiles are discussed in Figs. 7–9, which reveals that all the profiles are increasing with rise in $n$. The object behind this is the thin boundary layer is executed by enhancing values of $n$. Figures 10–12 show the influence of $\phi ,{\phi }_{1}$ on $\theta (\eta ),f\text{'}(\eta )$ and $g\text{'}(\eta )$ profiles. It showed that the rise in $\phi$ increases the temperature profile, but the reverse action has perceived by the impacts of ${\phi }_{1}$. Also the rise in $\phi$ digresses the both velocity profiles but converse action have seen in existence of ${\phi }_{1}$. As volume fraction grows, then fluid turns to hike its density and get more concentration leads to reduce the velocity and hike temperature.

Figure 6

Variation of ${\theta }_{w}$ on $\theta (\eta )$.

Figure 7

Variation of $n$ on $\theta (\eta )$.

Figure 8

Variation of $n$ on $g\text{'}(\eta )$.

Figure 9

Variation of $n$ on $f\text{'}(\eta )$.

Figure 10

Variation of $\phi ,{\phi }_{1}$ on $\theta (\eta )$.

Figure 11

Variation of $\phi ,{\phi }_{1}$ on $g\text{'}(\eta )$.

Figure 12

Variation of $\phi ,{\phi }_{1}$ on $f\text{'}(\eta )$.

Figures 13–15 represented the effect of $M$ on $\theta (\eta )$ and $f\text{'}(\eta ),g\text{'}(\eta )$ profiles. Obviously, increasing in temperature and a reduction in velocity has seen for developing $M$. The vitality of Lorentz force is gained for movements of electrically colloidal particles attracted by the effects of magnetic field. Therefore the velocity reduces and temperature enriches by Lorentz force. Figures (16–18) elucidates that an impact of ${h}_{1}$ on $\theta (\eta ),f\text{'}(\theta )$ and $g\text{'}(\theta )$ profiles. Raise in ${h}_{1}$ advances the thermal fields, but opposite action has seen for both the velocity. Cumulative the velocity slip parameter improves wall friction; this will cause the lessening in flow velocity. Generally, the slowdown in velocity turns to raise the temperature. Figure 19 displays the variant of ${h}_{2}$ over thermal field. It is noted that the temperature is falling due to hiking on ${h}_{2}$.

Figure 13

Variation of $M$ on $\theta (\eta )$.

Figure 14

Variation of $M$ on $g\text{'}(\eta )$.

Figure 15

Variation of $M$ on $f\text{'}(\eta )$.

Figure 16

Variation of ${h}_{1}$ on $\theta (\eta )$.

Figure 17

Variation of ${h}_{1}$ on $f\text{'}(\eta )$.

Figure 18

Variation of ${h}_{1}$ on $g\text{'}(\eta )$.

Figure 19

Variation of ${h}_{2}$ on $\theta (\eta )$.

Conclusions

Several scholars have pointed the assets of hybrid nanofluid are greater as associated with nanofluid. The persistence of this study is to examine the significance of heat transport with utilization of available nanoparticles to find greater hybrid nanofluid to enrich/decline the energy transfer. This study helps to identify the better combination of heat transfer material to enrich the thermal transport phenomenon. The conclusions are

• CuO+Methanol nanofluid shows better convection properties as related to CuO+MgO+Methanol hybrid nanofluid.

• The boundary thickness reduces by enhancing $n$.

• A Rise in ${h}_{1}$ advances the thermal field and decays the velocity.

• A Rise in ${h}_{2}$ intensifies the thermal field and monotonically deviate the Nusselt number.

• ${\theta }_{w}$ has propensity to lessen the heat rate.

• ${\phi }_{1}$ leads to magnify the flow and heat rate.

• CuO+MgO+Methanol combination may be used as an insulator.

• Application of the present study can be found in manufacturing industries.