Abstract
While the benefits of common and public goods are shared, they tend to be scarce when contributions are provided voluntarily. Failure to cooperate in the provision or preservation of these goods is fundamental to sustainability challenges, ranging from local fisheries to global climate change. In the real world, such cooperative dilemmas occur in multiple interactions with complex strategic interests and frequently without full information. We argue that voluntary cooperation enabled across overlapping coalitions (akin to polycentricity) not only facilitates a higher generation of non-excludable public goods, but it may also allow evolution toward a more cooperative, stable, and inclusive approach to governance. Contrary to any previous study, we show that these merits of multi-coalition governance are far more general than the singular examples occurring in the literature, and they are robust under diverse conditions of excludability, congestion of the non-excludable public good, and arbitrary shapes of the return-to-contribution function. We first confirm the intuition that a single coalition without enforcement and with players pursuing their self-interest without knowledge of returns to contribution is prone to cooperative failure. Next, we demonstrate that the same pessimistic model but with a multi-coalition structure of governance experiences relatively higher cooperation by enabling recognition of marginal gains of cooperation in the game at stake. In the absence of enforcement, public-goods regimes that evolve through a proliferation of voluntary cooperative forums can maintain and increase cooperation more successfully than singular, inclusive regimes.
Similar content being viewed by others
Introduction
In cooperation dilemmas, approaches to governance commonly face trade-offs alternatively between fragility and depth, and scope and participation (in other words, choosing between a weak agreement that includes everyone, or a strong agreement with holdouts or defections)1. The 2015 Paris Agreement on climate change is an example that sought to overcome these tradeoffs resulting from substantial heterogeneity in the national interests of participant countries, and its success or failure may clarify the potential for coalition-structure to sustain contributions to public goods2. The Paris Agreement relies on sovereign mitigation pledges and a periodic “global stocktake” intended to “ratchet” cooperation3,4,5, allowing for voluntary contributions to be deepened incrementally. The Agreement also embraced a role for overlapping coalitions of non-Party stakeholders, including cities, regions, businesses, and other non-state actors6 in facilitating the attainment of global objectives. This proliferation of cooperative arrangements with variable participation and scope – alternately conceived as building blocks7 or polycentricity8 – calls into question the view of these approaches as inferior to the “first-best approach”9 of a self-enforcing agreement with universal participation that disincentivizes free-riding10 and also captures emissions leakage11. Can these principles be applied to the overall sustainability of public- and common-goods provisioning?
Whereas single coalitions can be stable and cooperative, held together by spillovers such as learning from early experiences and technology sharing, or by side payments that balance the valuation of different members and change participation calculus for others12,13, multiple coalitions in a single realm of governance can also be beneficial14. The multiple, overlapping, cooperative forums characterizing polycentricity can be more productive for cooperative outcomes than politically attainable comprehensive regimes15,16,17,18,19. Voluntary, distributed participation is known to provide benefits to coalitions without additional mechanisms such as reciprocity and/or reward and punishment schemes20,21,22,23. These effects were explored in the context of the voluntary prisoner’s dilemma (VPD) and its extension to groups engaging in public goods N-person prisoner’s dilemma games, where individuals decide to play or not a prisoner’s dilemma with their neighbors. Whenever individuals decide to not play the game, they get a fixed payoff, independent of others’ action.
Extensions to these models include explicit spatial and other network representations24, varying density of players and the ability for players to move and participate in different groups25,26,27, revealing the effects of connectivity and group participation on the fate of cooperation. The work by Hannam et al.19 has shown, based on case studies, that polycentricity in voluntary agreements can deepen international cooperation. In their model, the authors study a particular game that collectively describes the examples they consider. The model includes two different levels of impure public-good provision: coalition co-benefits and direct co-benefits to contribution. Though it also represents a voluntary game, the model does not include explicit spatial structure and the outcome of the game for outsiders is linked to the creation of a public good that spills over to them. This means that the incentives to participation are even harder than in the traditional VPD. However, the authors consider a growing voluntary coalition with both differentiated structures and group sizes. Here, we take their control system and simultaneously show that polycentricity can be applied to a much broader class of problems – not only public goods but also common goods and any group interactions – and that its effects are stemming from improvements to lack of information, complementary to small group size effects, voluntary participation, or direct co-benefits to cooperation.
Polycentric governance is not a panacea but has promising features. If, on the one hand, polycentricity may increase the influence of cooperators28, on the other, the same might be said for defectors, except that, in practice, such cooperative arrangements are constructed as “coalitions of the willing.” Mechanisms such as reciprocity – either direct, where cooperation is expected to be retributed, or indirect, where cooperation is expected to boost reputation – create more favorable outcomes for cooperative endeavors29,30,31,32,33,34,35,36, including by allowing social norms37 and fairness38 to evolve. These approaches produce a plethora of setups and solutions for overcoming free-riding in collective-action problems.
Different coalition structures, ranging from polycentrism to a single coalition, alter the conditions under which individuals interact with others in groups. However, coalition structures and their dynamics remain under-examined in the science of cooperation and world politics on issues as diverse as trade, human rights, and security2,39, and even less explored in the governance of commons. Under which conditions coalition structures can be applied to a generality of cases is an important unresolved question that is urgent to answer, especially in the context of global climatic change. Experimentation is revealing that coalition-based and polycentric approaches can show substantial value for governing short-lived climate pollutants40,41, protecting fisheries42, and managing forest resources43,44. Improved theories for the origin of these advantages can inform future governance-of-commons challenges.
Using a combination of best-response analysis, typical of game theory, and myopic response, typical of evolutionary-game-theoretic (EGT) approaches, the model presented here focuses on cooperation in different coalition structures within a complex dynamical system to reveal fundamental insights about the behavior of coalitions in the generation of General Public Goods (GPGs). Best responders45 have full information about the game they are playing and can compute the outcome of a hypothetical change in strategy, the so-called marginal gains from switching strategy. On the contrary, myopic responders46 have no access to the game they are playing and base their response on the outcome of different behaviors they observe. We start with a game-theoretical analysis of marginal gains from switching between different strategies, an ideal type of strategic profile representing informed players (though still boundedly rational, without perfect foresight of future preferences)47. We then follow by describing another stylized-type: individuals that have no information about the game and can only access the outcomes of their and others’ current experience. The latter situation of “uninformed” players intuitively leads to less cooperative behavior, even in conditions that should be favorable to it. We show that constraining the size of each coalition relative to the total coalition engagement – creating multiple overlapping coalitions – enables “uninformed” players to recognize marginal gains of cooperation, such that they attain cooperative outcomes similar to those of the “informed” players. In other words, myopic players respond as best-responders, even if they do not directly access the game they are playing. The finding resonates with fundamental insights regarding why regimes are sought in international politics and suggests that further study of coalition-structured governance and polycentricity could be advantageous for sustaining cooperation in a range of issues, including climate change. We finish discussing the key assumptions that allow this analysis and drive the results, providing additional context in which we expect the general results to hold in the real world.
Model
We consider a population of size Z representing the relevant actors of the system and potential members of coalitions. By forming a coalition, players can produce a public good with a specified degree of excludability. We do not assume coalitions to be cooperative. Players chose alternatively to be members of the coalitions, M – who either cooperate, C, or defect, D – or outsiders, O. The lack of punishment mechanisms purposefully creates a difficulty for cooperation. We represent the coalitions as contribution games in which members interact in (sub-)groups of size N to obtain some benefit, B(C), that depends on the total contribution of each coalition, C. In games of loss, the benefit is often thought of as the loss that is not created, which, in reality, can be hard for the players to grasp. Our first strong assumption relies on considering that, at any given time, all existing coalitions have the same size and that the functional form B(C) is the same for all coalitions. Members contribute to the coalition, cc ≥ 0, which conceptually mimics potential running costs and signals shared goals. Cs additionally contribute a given amount, c, to the game considered to be the cost of cooperation, whereas Ds make no other contributions. A fraction, \(0\le e\le 1\), of the benefit produced is shared exclusively among Ms, creating a so-called club good48 and making Ds the free riders. The remaining fraction, \(1-e\), spills over to everyone, including outsiders, making it a public good. This parameter controls the extent to which the GPG can be privatized to the coalition. The model leaves out the institutional mechanisms of forum shopping and advocacy networks, and it is also comprised of homogeneous players, each with equal weight, so that there is no potential for a large player to nucleate or enforce cooperation (i.e., as a hegemon might do in international politics). Where the model could be conceived in terms of relations between cities, nations, and even aggregations such as the EU, we presume each entity makes decisions on its own accord, with no homophily49. There is no perception of a collective goal among players50, and action is based on return on contribution, not on reciprocation or retaliation of others’ actions51,52; only the unconditional pursuit of self-interest in each discrete time-step, with no foresight of the future or memory of past interactions. Even where there are gains to full cooperation, there is no capacity for ex-ante coordinated action, including in small groups. There is no potential for collective punishment or enforcement in the model, either among the players or externally imposed. Incorporating those mechanisms would each conduct to further and more stable cooperation in the structured games presented here16,17,53. The goal of our model is to show that allowing for coalitions with overlapping membership is on its own a positive mechanism for the sustainability of cooperation under minimal information.
Informed players
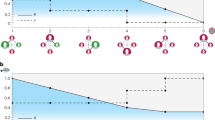
We start by assessing a game of “informed players,” who have complete information on the game and, hence, by definition, can compute payoffs assuming different behaviors and choose their approach strategically (even if without foresight). More precisely, these players can calculate their payoff and a hypothetical payoff with an alternative strategy. In Fig. 1 we specify six different states representing possible individual perceptions of the game at any given point, resulting from combinations of the shape of the benefit generated by total contribution, B(C), and three effective game values: coalition member share, \({\varepsilon }_{1}\equiv e/{N}^{\theta {\prime} }\); public-good spillover, \({\varepsilon }_{2}\); and relative cost of engagement, \(\kappa \) (see Methods for extended parameter description). Figure 1 includes representations of marginal gains from switching from strategy Y to X, \({\Delta \Pi }_{XY}\). In the decision-making, the relative cost of coalition engagement \(\kappa \) is judged against the relative benefit, \(b\equiv B/c\), whereas the cost of cooperation is judged against the marginal return on investment, \(R(C{\prime} )\equiv b(C{\prime} +c)-b(C{\prime} )\), for a fixed contribution of the remaining players, \(C\text{'}\). To tend toward a cooperative state, A, players need both (i) high marginal returns on investment, \(R > 1/({\varepsilon }_{1}+{\varepsilon }_{2})\), and (ii) sufficiently high gains from coalition engagement, \(b{\varepsilon }_{1} > 1-R({\varepsilon }_{1}+{\varepsilon }_{2})+\kappa \). This means that even if the coalition provides large benefits, with \(\max \,b > (1+\kappa )/{\varepsilon }_{1}\), in which case condition ii) is fulfilled, the ratio at which the benefit is produced per unit of investment is crucial for sustaining cooperative coalitions.
Possible states within the dynamic system, computed for informed players. Depending on the total contribution of the other players, C′, an informed player faces different scenarios depending on the game parameters. On the top right, we consider the marginal gains from switching between each pair of strategies given by \({\Delta \Pi }_{XY}\equiv {\Pi }_{X}-{\Pi }_{Y}\), where \({\Pi }_{X}\) are given in Eqs. (3). Here, we set \(b(C{\prime} )\equiv B(C{\prime} )/c\); \(R(C{\prime} )\equiv b(C{\prime} +c)-b(C{\prime} )\approx B{\prime} (C{\prime} )\), representing the marginal return per unit of investment; \({\varepsilon }_{1}\cong e/{N}^{\theta {\prime} }\); \({\varepsilon }_{2}\equiv (1-e)/{Z}^{\theta }\); and \(\kappa \equiv {c}_{c}/c\). Parameters θ and θ′ control congestion of the public and club goods. The sign of each of these three quantities controls the direction of its respective arrow in the states represented.
For a typical growing sigmoidal shape for b(C), we can reproduce general and well-known results. Starting with a large and highly cooperative coalition, if \(\max \,b\equiv b({C{\prime} }_{\max })\) is high enough, outsiders will join. However, for very high levels of cooperation, typically the variation of b is small, and new members join as defectors while cooperators are likely to stop contributing, state B. If contributions decrease, but b is still sufficiently high, marginal returns on cooperation increase, which leads to a stable large coalition, creating a dynamic balance between states A and B. Complementarily, as the coalition grows, member share \({\varepsilon }_{1}\) decreases, effectively reducing the balance \({\varepsilon }_{1}b\), which leads to lessened cooperation and, consequently, reduced total benefit produced and appeal of the coalition, leading to one of the remaining scenarios, C to E. We expect state A, even if stable, to reach a dynamic equilibrium with other states. Therefore, as a consequence of the assumption that benefits to contribution present decreasing returns for high contributions, even the most successful coalitions tend to have non-universal engagement and must tolerate free riders. These results are not new, but their application to our model of GPG is essential for comparison with a situation in which players cannot access the value of the return to their contributions.
As we already mentioned, a crucial assumption of our model is that players are entirely consistent in their strategy across different coalitions. Reputational gains and trust-building are examples of mechanisms that create a tendency for decisions being taken by an actor in one set of coalitions to have a bearing for similar actions in other coalitions54 – e.g., interests shaped in one coalition transfer to another. Overall, considering they use the same strategy in all coalitions, in models with informed players, cooperation increases with \(\langle R\rangle ({\varepsilon }_{1}+{\varepsilon }_{2})-1\) and coalition engagement with either \(\langle b\rangle {\varepsilon }_{1}-\kappa \) (for Ds) or \(\langle b\rangle {\varepsilon }_{1}-\kappa +\langle R\rangle ({\varepsilon }_{1}+{\varepsilon }_{2})-1\) (for Cs), where \(\langle \,\cdot \,\rangle \) represents average values over different coalitions (see Supplementary Material for detailed calculations). These conditions, leading to the possibility of stable cooperation, form the basis of our comparison of models with different coalition structures in the remainder of the paper.
Uninformed players and the mitigating impacts of coalition structure
In contrast to the assumption that players have complete information about their options, individuals in cooperation dilemmas often must make decisions without complete knowledge of the game. The same is true of nations with incomplete knowledge of the interests and strategies of others during complex negotiations55. As before, actors cannot act “strategically,” i.e., they do not anticipate the (re)action of others56. Contrary to the previous section, we now consider the extreme case in which information is absent, and individuals rely on theirs and others’ experiences to make decisions. This assumption is a substantial simplification of the recognition in the theory of world politics that information imperfections and high transaction costs motivate governments to create international regimes57. To show that polycentricity offers additional information, we compare two scenarios in which “uninformed individuals,” by definition, use the average payoff of players with a given behavior to evaluate the performance of that behavior. Then, we manipulate the structure of interactions without affecting the source of information. This model of behavioral change is inspired in works in evolutionary game theory applied to social contexts37,58,59, where individuals use social learning60 to adopt the currently best strategy in their neighborhood of influence. In our model, contrary to the standard literature in EGT, the structure of interactions is not limited to a fixed group size. Importantly, the interactions can occur between a fraction of the population that scales with the population size – for instance, when the whole population is engaged in a single interaction. As we will show, this means that the result that Nash equilibria are necessarily equilibria of this evolutionary dynamics no longer hold61,62. Accordingly, we develop a model where interaction structure can change over time with changes in behavior. Two factors determine the dynamics: (i) average cooperative behavior within coalitions changes depending on the difference in the average payoff of Cs and Ds; and (ii) the average payoff of those members relative to the average payoff of Outsiders governs the change in the number of coalition members. Considering this interpretation, our results can be compared with the analysis in the previous section.
We are interested in studying the effect of overlapping coalitions, rather than a single coalition, which requires the typical coalition size to be smaller than the number of members. For this purpose, we use an exogenously determined shape that both constrains the growth of any coalition and leads to coalition proliferation19. In Fig. 2A, we show how \(N(y)\) is an increasing function of the fraction of members in the population, y, which allows for the continuous growth of the typical coalition size as more individuals engage in coalitions (either as Cs or Ds). If \(\alpha =1\), then the coalition size is simply the number of players engaged in coalitions (i.e., there is only one coalition). However, for any larger value of \(\alpha \), the coalition size is bound to be smaller, until there is universal participation when y approaches 1. Notice that a value of \(\alpha \) bigger than 5 already makes the size of the coalition restricted to its small baseline value, gm, until more than half of the population is participating. This also means that its effects on the dynamics are smaller and smaller.
Operationalization and effect of structuring cooperation in overlapping coalitions. Panel (A) shows how coalitions grow and proliferate in the dynamic system; for any fraction of members in the population, y, increasing \(\alpha \) constrains the size of the typical coalition. Panel (B) shows the distribution (mean ± standard deviation) of the engagement in any coalition, y, and share of those coalition members who interact cooperatively, x. Increasing \(\alpha \) results in both higher levels of coalition engagement and in greater cooperation within coalitions. Panels (C–E) further demonstrate the dynamic benefit for cooperation with increasing values of \(\alpha \), for a specific (sigmoidal) choice of the benefit function. They represent the most likely direction of evolution of the system with warmer colors representing faster rates of evolution whereas the background shadow represents the regions where the system spends more time. These results (panels B–E) indicate that increasing parameter \(\alpha \) enables player with limited knowledge of the game to better recognize potential gains of cooperation. Notice that in C, even though the system spends most time near the O vertex, the vertex is unstable due to exogenous factors introduced (see below), creating a cyclic dynamic. In effect, coalition-structured governance reduces the cost of absence of information, K. Parameters: Z = 100, \({g}_{m}=5/Z\), \(e=0.5\), c = 1, \({c}_{c}=1\), \(\beta ={10}^{-1}\), \(\mu =1/Z\), \(B(C{\prime} )=100(F(C{\prime} )-F(0))/(F(Nc)-F(0))\), with \(F(C{\rm{{\prime} }})=(1+{e}^{100\left(\frac{C{\rm{{\prime} }}}{Nc}-\frac{3}{4}\right)})\), a sigmoidal function specified here with a sharp threshold at ¾ of the group. In order to guarantee the system has no absorbing states, we introduce the possibility for random changes of strategies – an added factor of noise or exogenous shocks – by resetting \({T}_{XY}=\frac{{i}_{X}}{Z}\frac{{i}_{Y}}{Z-1}p(X,Y)(1-\mu )+\frac{\mu }{2}\). (See18,63,64 for connection between the arrows indicating the most likely direction of evolution and the prevalence times and for details on their computation).
A single coalition (α = 1)
The case of a single coalition can be described by setting \(\alpha =1\). It is trivial to show that for all shapes of \(B\) and values of the parameters, the fraction of cooperators always decreases, leading to the collapse of any “uniformed” single coalition. In Fig. 2B, the lowest level of cooperation happens for \(\alpha =1\), with non-zero values being due to exogenously imposed noise perturbing the system. Accordingly, Fig. 2C shows the dynamics of this system pointing to configurations in which most individuals are Os. Consequently, the probability distribution, represented by the shadow background, is also close to that point.
This proves how a single coalition is very hard to bootstrap with players whose information is bounded to their current interactions, resulting in an uncooperative equilibrium even for very favorable game conditions with a fully cooperative Nash equilibrium – e.g., a marginal return on a unit of contribution much greater than one. This information limitation is a harsh scenario and, in reality, players negotiate on the basis of some information – even if scarce, uncertain or simply created by analogy with previously known/experienced games or dilemmas; for instance, the Kyoto Protocol climate negotiations applied the Montreal Protocol model of regime structure, even though the game was different10. However, the extreme case presented here sets a pessimistic baseline, which, as shown below, can be improved without adjusting the capabilities of the players in the game.
Multiple coalitions (α > 1)
Multiple coalitions allow for experimentation with different actors and under different circumstances, which is essential given the complex interests in commons governance challenges and uncertainties as to the strategic interests and intentions of other players. Lack of information – or a deluge of it – can hobble recognition of welfare-improving opportunities for cooperation. To examine whether coalition-structured governance can overcome the disadvantages of the single-coalition case, we constrain the size of coalitions as players become Members, thus creating multiple overlapping coalitions for \(\alpha > 1\). We show that this will change the dynamics of coalition engagement and cooperation, all else equal. The dynamics of the fraction of cooperators within the coalitions, x, are still governed by the fitness difference between Cs and Ds. That can be expressed in a way similar to what we showed for informed players (see Supplementary Material for proofs) as
The first term corresponds to the marginal gains of cooperating that governed the informed dynamics, \({\Delta \Pi }_{CD}\), with R standing as the average return on investment, which compares to a cooperation cost, normalized to 1. The last term, K, corresponds to the difference between informed and uninformed players and entails an effective cost for cooperation that (i) exactly cancels the marginal return on investment – when \(\alpha =1\) (the single coalition), \(K=R(C{\prime} )({\varepsilon }_{1}+{\varepsilon }_{2})\) and ii) vanishes when the coalition size is highly constrained, \(K\ll R({\varepsilon }_{1}+{\varepsilon }_{2})\). We can interpret K as the cost of the limitations on information available to Cs, which can be zero in some cases. Looking back at our definition of group size constraint, in its essence, the restriction on information is controlled by \(N/Zy \sim {y}^{\alpha -1}\). For high \(\alpha \), larger values of \(y\), engagement in coalitions, can be attained for which the K term remains small, and the lack of information plays a small role. This effect is not an effect of small group size, as setting \(\alpha \) to 1 removes any perception of return of contributions, independently of Z and its consequent N. Instead, the effect is the result of the experimentation with different configurations between updates, which is the only way individuals can access more information about the game and the returns to contributions. Polycentricity – in particular, but not exclusively – can achieve such an outcome.
As for the growth of the coalitions, it can be described as
with \(b\) standing as the average relative benefit produced. As we saw for the informed case, it is crucial that the benefit being produced is high, which can be directly assessed from a comparison between members and non-members. The creation of multiple coalitions allows players to access the marginal gains of the excludable and non-excludable part of the benefit produced by joining a coalition (see Supplementary Material for details on \({{\rm{{\rm K}}}}_{M}\) and proofs).
Going back to Fig. 2B, as \(\alpha \) increases, the cost of lack of information, \(K\), decreases, which induces an internal fixed point with high participation and cooperation. Nonetheless, the nature of that fixed point is a complex one. In the bottom panels of Fig. 2, we show how, for the sigmoidal shape of the benefit B with a sharp threshold, the internal fixed point with high participation goes from a (stable) spiral, in panel D, to a sink in panel E as \(\alpha \) grows. Different shapes of B allow for different classifications of the fixed point, including for its stability, but also change the basin of attraction. Notice, additionally, that the slow regions, represented with arrows with cooler colors, even if transient in the dynamics, have non-negligible probability, increasing the variance of the distribution around the fixed points.
Discussion
Problems of collective action are more easily overcome in small groups, but effective management of common resources frequently requires broad participation. We allow for cooperation to emerge through polycentric structures through a control variable that determines the rules of coalition size and growth (and, consequently, coalition proliferation). Isolating the effect of this variable, we show that, for a general class of public-goods, higher degrees of coalition-based governance not only facilitate a greater generation of non-excludable public goods, but it may also allow evolution toward a more cooperative, stable, and inclusive singular regime. Contrary to any previous study, our results are applicable to the whole range of excludability of the public good, congestion of the non-excludable public good, and for any shape of the return function, whether it implies a need for behavior coordination, dominance, or an optimal mix.
The model caricaturizes dynamic cooperation dilemmas in the provision of GPGs, with non-hierarchical actors making decisions that are both normative and selfish in an information-poor environment. The advantages of increasing multi-coalition regime structure for generation of GPGs, relative to fixed coalition size involving the total population, are a result of minimizing the apparent advantage of free-riders (ubiquitous in the provision of GPGs). By creating multiple references for cooperative action, the multi-coalition structure enables the recognition of marginal gains of cooperation in the game at stake. Indeed, the value of regime creation for overcoming the costs of information has long been known in world politics; governments create regimes to correct for “market failures” in international relations, enabling them to develop agreements in their mutual interest. We argue that coalition-structured governance strengthens the mechanism of information transmission relative to inclusive governance approaches, allowing actors to recognize opportunities for cooperative gains better. This finding builds on benefits already well understood of cooperation in smaller groups, including the development of trust, reciprocity, and ease of enforcement via punishment and ostracism65,66,67. These other mechanisms are important, since they may help to sustain cooperation once domestic spillovers are exploited, for instance. We notice that coalition structure governance is not a sufficient guarantee of success. Whenever the incentives are not present, marginal gains cannot be accessed by this mechanism. As an example, Fig. 2E evidences rare bursts, with different sizes, where cooperation collapses before coming back up, a phenomenon observed in other systems, including recently in spatially explicit dynamics68. Additionally, we note that the added information created by the coalition-structured governance is reliant on a couple of key assumptions we identified in the text. The first is the tendency for players to have consistent strategies in different coalitions. We argued that non-modeled reputational gains could induce this, but it requires recognition that all coalitions have a common goal even if the lines of action are quite distinct. A balance between redundancy and efficiency would need to be studied. To some extent, this effect can be rephrased on the ability of players to associate actions to an outcome, and that might not be the case if (i) the stance of the individual players is not public or if (ii) the players are involved in coalitions across which they have very heterogeneous responses. The second assumption regards the existence of an identical game being played by all coalitions. Naturally, the different coalitions could be structured to derive co-benefits conditional on overall climate mitigation performance – and that would be a policy recommendation for the coalition co-benefit distribution. However, we expect heterogeneity in terms of efficiency and that the game being played by each coalition to be different. Nonetheless, in an environment where information about the returns of investment is scarce, decisions might be made according to an overall perceived benefit, which can homogenize the effective games different coalitions play.
Defining the conditions under which coalition-based governance may be more effective will require further study. This work has suggested additional merits for multi-level governance and plurilateral coalitions – for regimes lacking outside external authority, with incomplete information, and strong free-riding dynamics. These merits need to be weighed against inefficiency costs arising from having multiple coalitions. The interplay between the scale of the public good and the scale of the decisions for participation in the governing institutions may also play a paramount role that needs further exploration and in-depth analysis, both in theoretical and practical settings. Our work is also not intended to suggest that fragmentation would benefit cooperation in otherwise functional inclusive regimes69. However, the multi-coalition structure – mainly the encouragement of initiatives with domestic co-benefits – may be the most productive way to “boot-strap” a coalition with broadening participation, including for instance the potential for sub-national, national, and regional carbon markets to eventually harmonize into a global market, gradually removing inefficiencies once behavioral coordination barriers are overcome70. Our results suggest that reducing the scale at which the coordination game occurs – as with overlapping coalitions – can facilitate larger-scale cooperative outcomes.
Community-based natural resource management is an example of decentralized management of public goods71. There, the identified need of power transfer to the local institutions and of accountable representation could be achieved through this overlapping structure, not only by contributing to the management of multiple parcels in diverse groups but also by involving the local institutions in a panoply of related issues, rather than isolating their presumably increased control. Our model suggests that decentralization without overlap may not provide enough incentives, especially when information about the resource dynamics – and the corresponding response to different extracting behaviors – is limited.
As we have reinforced throughout the text, climate will be a crucial test case. Following decades of diplomatic effort, the Paris Agreement has achieved (nearly) full participation by being “catalytic and facilitative” of overlapping cooperative arrangements, including carbon markets and sub-national actions in synergy with country contributions6. This structure much more closely resembles a multi-coalition regime than a “comprehensive regime” structure with uniform rules for all countries that had long been sought as a replacement to the Kyoto Protocol. Nonetheless, key questions remain. Does coalition structured governance continue to excel when excludable benefits for cooperators start to run out? Does polycentricity still perform better than an inclusive coalition when the largest player in the system free-rides across coalitions? We leave these important questions for further study.
Methods
We consider a population of finite size Z. Players interact in groups/coalitions of size N to obtain some benefit, B(C), that depends on the total contribution of each coalition, C, and this dependence is the same for all existing coalitions. Players can adopt one of three strategies: cooperate, C, defect, D, or remain outside, O. Cs and Ds contribute to the coalition, cc, but Cs contribute an additional amount, c. A fraction, e, of the benefit produced is shared exclusively among Ms, whereas the remaining fraction, \(1-e\), spills over to everyone, including outsiders. This parameter allows us to interpolate between games with and without co-benefits. With this, we can write the payoff of a player with a given strategy when the amount contributed by the remaining players in the coalition is \({C}^{\text{'}}\):
and
Notice that \(\theta {\prime} \) and \(\theta \), defined between 0 and 1, control the “congestibility” of the good72. For \(\theta =1\), the good is fully congestible, meaning the participation of one player reduces the spillover produced by the next, a characteristic of common-pool resources; for \(\theta =0\), there is no congestion, which is a common property of air quality and other public goods that spill to other players outside of the game. A variation of this model, which studies a particular functional form of \(B\) and type of public good, is discussed in Hannam et al. 2015. but without examination of the system dynamics or mechanisms. The group size is set as \(N(y)=Z\,\min \,\{y,{g}_{m}+(1-{g}_{m}){y}^{\alpha }\}\le Zy\), where gm is the minimum group size for a single coalition before multiple coalitions may form.
For the evolutionary game, we use a well-documented dynamic of imitating the better neighbor73,74. In each time step, we randomly select a player, X, to potentially change strategy. Player X randomly selects another player, Y, and compares her own average payoff, fX, obtained in all the coalitions that she is part of, to that of player Y, fY. With probability \(p(X,Y)={(1+{e}^{\beta ({f}_{X}-{f}_{Y})})}^{-1}\) player X changes her strategy to that of player Y, where \(\beta \) controls the intensity of selection or, equivalently, the level of errors/certainty in the imitation process. This is often called the logit rule, or Fermi update, also known as pairwise comparison rule59, which is the noise version of the “best response” dynamics we discuss, and which entails very interesting and convenient statistical properties75. Finally, if we let \({i}_{C}\), \({i}_{D}\), and \({i}_{O}\) be the number of Cs, Ds, and Os, respectively, we can set the number of members as \({i}_{M}={i}_{C}+{i}_{D}\) and use the hypergeometric sampling of different coalitions, \(P(k;z,n,i)={(\begin{array}{c}z\\ n\end{array})}^{-1}(\begin{array}{c}i\\ k\end{array})(\begin{array}{c}z-i\\ n-k\end{array})\), to write the average payoff of each strategy
With this, one can write the probability that a player with strategy X changes into strategy Y as \({T}_{XY}=\frac{{i}_{X}}{Z}\frac{{i}_{Y}}{Z-1}p(X,Y)\). Given that we are interested in a scenario in which information is scarce, certainty on the payoff difference is likely small, so \(\beta \) is small in comparison. In this case, one can show that the average fraction of each of the three strategies, \({x}_{X}={i}_{X}/Z\), \(X=\{C,D,O\}\), can be described by the so-called replicator equation64,76. This equation states that \({\dot{x}}_{X}={x}_{X}({f}_{X}-f)\), where \(f={x}_{C}\,{f}_{C}+{x}_{D}\,{f}_{D}+{x}_{O}\,{f}_{O}\) is the average payoff of all individuals. Therefore, if \(y\) is the fraction of members, C and D players, in the whole population, \(y\equiv {i}_{M}/Z\), and \(x\) is the fraction of Cs among those, \(x\equiv {x}_{C}/({x}_{C}+{x}_{D})\), we can write
where \(x\) describes how cooperative the coalitions are, and \(y\) is a proxy for the maximum size of the coalitions.
A single coalition (α = 1)
In this case, \(N(y)=Zy\), which simplifies Eqs. (4) and, in turn, Eqs. (5). Given that \(P(k;Zy-1,Zy-1,i)={\delta }_{ki}\), the Kronecker delta, \({f}_{C}-{f}_{D}=-c\) for all shapes of \(B\) and values of the parameters. Thus, \(\dot{x} < 0\) for any fraction of cooperators and, therefore, no cooperation, \(x=0\), is the only stable state. In turn, \(\dot{y} < 0\), and, necessarily, \((x,y)=(0,0)\) is the final stable state, in which there is no coalition or contributions.
Multiple coalitions (α > 1)
We obtain Eq. (1) by plugging Eq. (4.1) and Eq. (4.2) in Eq. (5.1). We can rearrange the latter as \(\dot{x}=x(1-x)c(R({\varepsilon }_{1}+{\varepsilon }_{2})-1-K)\), with \(R=\mathop{\sum }\limits_{k=0}^{N-1}(P(k;Zy-1,N-1,{i}_{C})+\)\(P(k;Zy-1,N-1,{i}_{C}-1))/2\,R(kc)\) and \(K\) represents the difference in computed payoff difference between informed and uninformed individuals (see supplementary material for details). Finally, Eq. (2) is obtained by plugging Eqs. (4) in Eq. (5.2). We rearrange to obtain \(\dot{y}=y(1-y)c(b{\varepsilon }_{1}-x-\kappa )\) with \(b=x\mathop{\sum }\limits_{k=0}^{N-1}P(k;\Upsilon -1,N-1,{i}_{C}-1)b(kc+c)+(1-x)\mathop{\sum }\limits_{k=0}^{N-1}P(k;\Upsilon -1,N-1,{i}_{C})b(kc)\). In models of infinite populations that do not consider outsider and consider groups of finite and fixed size which experience all possible configurations, the \({\rm K}\) term is zero, reflecting no cost of lack of information (see an illustrative example of such a case in77). In this paper, however, our control structure allows for coalitions that are of a size comparable with that of the population.
Data availability
Source data for Fig. 2 were generated at the authors’ computers and are available from the corresponding author on request. The authors declare that the data supporting the findings of this study are available and reproducible with the information provided within the paper.
References
Downs, G. W., Rocke, D. M. & Barsoom, P. N. Is the good news about compliance good news about cooperation? Int. Organ. 50, 379–406 (1996).
Keohane, R. O. & Victor, D. G. The Regime Complex for Climate. Perspect. Polit. 9, 7–23 (2011).
Falkner, R. The Paris Agreement and the new logic of international climate politics. Int. Aff. 92, 1107–1125 (2016).
UNFCCC. The Paris Agreement. Article 14 (2015).
Christens, S. & Dannenberg, A. “Naming and shaming” of individuals and groups in a public goods experiment. Coalition Theory Network (CTN), Available at, http://www.coalitiontheory.net/content/“naming-and-shaming”-individuals-and-groups-public-goods-experiment (2017).
Hale, T. All Hands on Deck: The Paris Agreement and Nonstate Climate Action. Glob. Environ. Polit. 16, 12–22 (2016).
Stewart, R. B., Oppenheimer, M. & Rudyk, B. A new strategy for global climate protection. Clim. Change 120, 1–12 (2013).
Ostrom, E. Polycentric systems for coping with collective action and global environmental change. Glob. Environ. Chang. 20, 550–557 (2010).
Wiener, J. B., Stewart, R. B., Hammitt, J. K. & Hourcade, J.-C. Madison and Climate Change Policy. Science 311, 335–336 (2006).
Barrett, S. Environment and statecraft: The strategy of environmental treaty-making: The strategy of environmental treaty-making. (OUP Oxford, 2003).
Peters, G. P., Davis, S. J. & Andrew, R. M. A synthesis of carbon in international trade. Biogeosciences Discuss. 9, 3949–4023 (2012).
Carraro, C. & Siniscalco, D. Strategies for the international protection of the environment. J. Public Econ. 52, 309–328 (1993).
Hovi, J., Sprinz, D. F., Sælen, H. & Underdal, A. The Club Approach: A Gateway to Effective Climate Co-operation? Br. J. Polit. Sci. 1–26 (2017).
Silva, E. C. D. & Zhu, X. Overlapping international environmental agreements. Strateg. Behav. Environ. 5, 255–299 (2015).
Ostrom, V., Tiebout, C. M. & Warren, R. The Organization of Government in Metropolitan Areas: A Theoretical Inquiry. Am. Polit. Sci. Rev. 55, 831–842 (1961).
Ostrom, E. Governing the Commons, (Cambridge university press), https://doi.org/10.1017/CBO9781316423936 (2015).
Vasconcelos, V. V., Santos, F. C. & Pacheco, J. M. A bottom-up institutional approach to cooperative governance of risky commons. Nat. Clim. Chang. 3, 797–801 (2013).
Vasconcelos, V. V., Santos, F. C. & Pacheco, J. M. Cooperation dynamics of polycentric climate governance. Math. Model. Methods Appl. Sci. 25, 2503–2517 (2015).
Hannam, P. M., Vasconcelos, V. V., Levin, S. A. & Pacheco, J. M. Incomplete cooperation and co-benefits: deepening climate cooperation with a proliferation of small agreements. Clim. Change 144, 65–79 (2017).
Hauert, C., De Monte, S., Hofbauer, J. & Sigmund, K. Volunteering as Red Queen mechanism for cooperation in public goods games. Science, https://doi.org/10.1126/science.1070582 (2002).
Szabó, G. & Hauert, C. P Transitions and Volunteering in Spatial Public Goods Games. Phys. Rev. Lett., https://doi.org/10.1103/PhysRevLett.89.118101 (2002).
Szabó, G. & Hauert, C. Evolutionary prisoner’s dilemma games with voluntary participation. Phys. Rev. E - Stat. Physics, Plasmas, Fluids, Relat. Interdiscip. Top., https://doi.org/10.1103/PhysRevE.66.062903 (2002).
Orbell, J. M. & Dawes, R. M. Social Welfare, Cooperators’ Advantage, and the Option of Not Playing the Game. Am. Sociol. Rev., https://doi.org/10.2307/2095951 (1993).
Szabó, G. & Fáth, G. Evolutionary games on graphs. Physics Reports, https://doi.org/10.1016/j.physrep.2007.04.004 (2007).
Hauert, C. & Szabó, G. Prisoner’s dilemma and public goods games in different geometries: Compulsory versus voluntary interactions. Complexity, https://doi.org/10.1002/cplx.10092 (2003).
Cardinot, M., O’Riordan, C., Griffith, J. & Szolnoki, A. Mobility restores the mechanism which supports cooperation in the voluntary prisoner’s dilemma game. New J. Phys. https://doi.org/10.1088/1367-2630/ab3064 (2019).
Szabó, G. & Vukov, J. Cooperation for volunteering and partially random partnerships. Phys. Rev. E - Stat. Nonlinear, Soft Matter Phys., https://doi.org/10.1103/PhysRevE.69.036107 (2004).
Davis, C. L. Overlapping Institutions in Trade Policy. Perspect. Polit. 7, 25–31 (2009).
Trivers, R. L. The evolution of reciprocal altruism. Q. Rev. Biol. 46, 35–57 (1971).
Berg, J., Dickhaut, J. & McCabe, K. Trust, reciprocity, and social history. Games Econ. Behav. 10, 122–142 (1995).
Roberts, G. Evolution of direct and indirect reciprocity. Proc. R. Soc. B Biol. Sci. 275, 173–179 (2007).
Delton, A. W., Krasnow, M. M., Cosmides, L. & Tooby, J. Evolution of direct reciprocity under uncertainty can explain human generosity in one-shot encounters. Proc. Natl. Acad. Sci. 108, 13335–13340 (2011).
Pinheiro, F. L., Vasconcelos, V. V., Santos, F. C. & Pacheco, J. M. Evolution of all-or-none strategies in repeated public goods dilemmas. PLoS Comput. Biol. 10, e1003945 (2014).
Van Veelen, M., García, J., Rand, D. G. & Nowak, M. A. Direct reciprocity in structured populations. Proc. Natl. Acad. Sci. 109, 9929–9934 (2012).
Nowak, M. A. & Sigmund, K. Evolution of indirect reciprocity by image scoring. Nature 393, 573 (1998).
Keohane, R. O. Reciprocity in international relations. Int. Organ. 40, 1–27 (1986).
Santos, F. P., Santos, F. C. & Pacheco, J. M. Social norm complexity and past reputations in the evolution of cooperation. Nature 555, 242 (2018).
Van Segbroeck, S., Pacheco, J. M., Lenaerts, T. & Santos, F. C. Emergence of fairness in repeated group interactions. Phys. Rev. Lett. 108, 158104 (2012).
Alter, K. J. & Meunier, S. The Politics of International Regime Complexity. Perspect. Polit. 7, 13–24 (2009).
Aakre, S., Kallbekken, S., Van Dingenen, R. & Victor, D. G. Incentives for small clubs of Arctic countries to limit black carbon and methane emissions. Nat. Clim. Chang. 8, 85 (2018).
Victor, D. G., Zaelke, D. & Ramanathan, V. Soot and short-lived pollutants provide political opportunity. Nat. Clim. Chang. 5, 796 (2015).
Deacon, R. T., Parker, D. P. & Costello, C. Reforming fisheries: lessons from a self-selected cooperative. J. Law Econ. 56, 83–125 (2013).
Agrawal, A., Chhatre, A. & Hardin, R. Changing governance of the world’s forests. Science 320, 1460–1462 (2008).
Nagendra, H. & Ostrom, E. Polycentric governance of multifunctional forested landscapes. Int. J. Commons 6 (2012).
Matsui, A. Best response dynamics and socially stable strategies. J. Econ. Theory 57, 343–362 (1992).
Ellison, G. Learning, local interaction, and coordination. Econom. J. Econom. Soc. 1047–1071 (1993).
March, J. G. Bounded Rationality, Ambiguity, and the Engineering of Choice. Bell J. Econ. 9, 587–608 (1978).
Buchanan, J. M. An Economic Theory of Clubs. Economica 32, 1–14 (1965).
Vasconcelos, V. V., Santos, F. C., Pacheco, J. M. & Levin, S. a. Climate policies under wealth inequality. Proc. Natl. Acad. Sci. USA 111, 2212–6 (2014).
Mulvey, P. & Klein, H. The Impact of Perceived Loafing and Collective Efficacy on Group Goal Processes and Group Performance. Organ. Behav. Hum. Decis. Process. 74, 62–87 (1998).
Press, W. H. & Dyson, F. J. Iterated Prisoner’s Dilemma contains strategies that dominate any evolutionary opponent. Proc. Natl. Acad. Sci. 109, 10409–10413 (2012).
Stewart, A. J. & Plotkin, J. B. From extortion to generosity, evolution in the iterated prisoner’s dilemma. Proc. Natl. Acad. Sci. 110, 15348–15353 (2013).
Riolo, R. L., Cohen, M. D. & Axelrod, R. Evolution of cooperation without reciprocity. Nature 414, 441–443 (2001).
Cole, D. H. Advantages of a polycentric approach to climate change policy. Nat. Clim. Chang. 5, 114–118 (2015).
Hedstrom, P., Green, D. P. & Shapiro, I. Pathologies of Rational Choice Theory: A Critique of Applications in Political Science. Contemporary Sociology 25, (Yale University Press, 1996).
Powell, R. & Lake, D. A. Strategic Choice and International Relations. (Princeton, NJ: Princeton University Press, 1999).
Keohane, R. O. The Demand for International Regimes. Int. Organ. 36, 325–355 (1982).
Santos, F. C., Santos, M. D. & Pacheco, J. M. Social diversity promotes the emergence of cooperation in public goods games. Nature 454, 213–216 (2008).
Traulsen, A., Nowak, M. A. & Pacheco, J. M. Stochastic dynamics of invasion and fixation. Phys. Rev. E 74, 11909 (2006).
Börgers, T. & Sarin, R. Learning Through Reinforcement and Replicator Dynamics. J. Econ. Theory 77, 1–14 (1997).
Sigmund, K. & Nowak, M. A. Evolutionary game theory. Curr. Biol. 9, R503–R505 (1999).
Hofbauer, J. & Sigmund, K. Evolutionary games and population dynamics. (Cambridge university press, 1998).
Vasconcelos, V. V., Santos, F. P., Santos, F. C. & Pacheco, J. M. Stochastic Dynamics through Hierarchically Embedded Markov Chains. Phys. Rev. Lett. 118, 058301 (2017).
Pacheco, J. M., Vasconcelos, V. V. & Santos, F. C. Climate change governance, cooperation and self-organization. Phys. Life Rev. 11, 573–586 (2014).
Tavoni, A., Schlüter, M. & Levin, S. The survival of the conformist: Social pressure and renewable resource management. J. Theor. Biol. 299, 152–161 (2012).
Tilman, A. R., Watson, J. R. & Levin, S. Maintaining cooperation in social-ecological systems. Theor. Ecol. 10, 155–165 (2017).
Somanathan, E. & Sethi, R. The Evolution of Social Norms in Common Property Resource Use. Am. Econ. Rev. 86, 766–788 (1996).
Hódsági, K. & Szabó, G. Bursts in three-strategy evolutionary ordinal potential games on a square lattice. Phys. A Stat. Mech. its Appl. https://doi.org/10.1016/j.physa.2019.04.144 (2019).
Sekar, N. et al. Ivory crisis: Growing no-trade consensus. Science 360, 276–277 (2018).
Barrett, S. & Dannenberg, A. Tipping versus cooperating to supply a public good. J. Eur. Econ. Assoc. 15, 910–941 (2017).
Ribot, J. C. Democratic decentralization of natural resources. World Resour. Institute, Washingt. DC (2002).
Dixit, A. & Levin, S. Social Creation of Pro-social Preferences for Collective Action. In The Theory of Externalities and Public Goods: Essays in Memory of Richard C. Cornes (eds. Buchholz, W. & Rübbelke, D.) 127–143 (Springer International Publishing, https://doi.org/10.1007/978-3-319-49442-5_7 (2017).
Schlag, K. H. Why Imitate, and If So, How?: A Boundedly Rational Approach to Multi-armed Bandits. J. Econ. Theory, https://doi.org/10.1006/jeth.1997.2347 (1998).
Traulsen, A., Semmann, D., Sommerfeld, R. D., Krambeck, H.-J. & Milinski, M. Human strategy updating in evolutionary games. Proc. Natl. Acad. Sci. 107, 2962–2966 (2010).
Szabó, G. & Borsos, I. Evolutionary potential games on lattices. Physics Reports, https://doi.org/10.1016/j.physrep.2016.02.006 (2016).
Traulsen, A., Claussen, J. C. & Hauert, C. Evolutionary dynamics: From finite to infinite populations. Phys. Rev. Lett. 95, 1–4 (2005).
Pacheco, J. M., Santos, F. C., Souza, M. O. & Skyrms, B. Evolutionary dynamics of collective action in N-person stag hunt dilemmas. Proc. R. Soc. B Biol. Sci. 276, 315–321 (2008).
Inc., Wolfram Research. Mathematica, Version 11.3.
Acknowledgements
Supported by US Defense Advanced Research Projects Agency (D17AC00005), National Science Foundation grant GEO-1211972, and Fundação para a Ciência e Tecnologia (FCT) through grants PTDC/MAT/STA/3358/2014, PTDC/EEI-SII/5081/2014, and UID/BIA/04050/2013. P.M.H. was supported by the Walbridge Fund at the Princeton Environmental Institute.
Author information
Authors and Affiliations
Contributions
V.V.V. and P.M.H. contributed equally and wrote the paper, with input and comments from all authors. V.V.V. P.M.H, J.M.P., and S.A.L. all contributed intellectual content in designing and analyzing the model.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Disclaimers: This work is not submitted elsewhere for publication. It is our original work, and all authors have contributed to qualify for authorship.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Vasconcelos, V.V., Hannam, P.M., Levin, S.A. et al. Coalition-structured governance improves cooperation to provide public goods. Sci Rep 10, 9194 (2020). https://doi.org/10.1038/s41598-020-65960-8
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41598-020-65960-8
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.