Introduction

Nature impresses us with images, changes and tendencies that repeat themselves innumerable times even though “similar observations” are not identical to each other. In science, we recognize each ubiquitous tendency as a distinct phenomenon. Over the centuries, our predecessors have summarized each distinct phenomenon with its own law of physics, which then serves as a ‘first principle’ in the edifice of science. A principle is a ‘first principle’ when it cannot be deduced from other first principles.

This aspect of organization in science is illustrated by the evolution of thermodynamics to its current state1,2. For example, 150 years ago the transformation of potential energy into kinetic energy and the conservation of “caloric” were fused into one statement—the first law of thermodynamics—which now serves as a first-principle in physics. It was the same with another distinct tendency in nature: everything flows (by itself) from high to low. This, the phenomenon of one-way flow, or irreversibility, was summarized in another statement at the same time—the second law of thermodynamics—which serves as another first-principle in physics.

Why do the most common occurrences need such a long time to be recognized as natural tendencies (phenomena), and even longer to be recorded in physics with a short statement, a first principle? Because the evolution of the human mind is an integral part of the evolution of the human, to adapt and survive while struck by unexpected dangers, environmental, animal, and human. The first thing that we question is the unusual (the “surprise”, which means being grabbed from above, as if in the claws of a predator). Questioned the least are the most common observations, the familiar, the not threatening. This is why new questions in science are rare.

Nowhere is the human approach to science more evident than in the face of the natural phenomenon of evolutionary organization1,3. Images, morphing images, impress us constantly, yet the most common images go unnoticed. For example, the oneness of natural tree-shaped architectures of the inanimate realm (e.g., river basins) and the animate realm (e.g., human lungs, city traffic) is evident and intriguing. Recent articles are drawing attention to phenomena of evolution that are general and belong in physics3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21,22,23. This literature shows that such phenomena are predictable. Examples are the architectures of lungs24 and corals25, the life span and life travel of animals, vehicles, rivers and the winds26, the round cross sections of all jets and plumes27, the dendritic architecture and S-shaped history of dendritic solidification such as snowflakes28, the arrow of time of evolutionary organization29, and the fact that humans prefer unwittingly certain shapes and proportions, from the shapes of the Egyptian pyramids30 and the shapes of fires (piles of fuel)31, to the golden-ratio shape of drawings, images and text.

Because of its physics basis, the phenomenon of evolution can be imagined the way it happened, in retrospect. Further ahead along this line, evolution can be not only predicted but also witnessed in our life time, for example, by observing technological evolution. The geometric similarity of modern commercial aircraft32, like the similarity of helicopters33 and automobiles34, shows that human movement on the world map is facilitated by the generation and persistence of certain shapes and structures.

In this paper we strengthen this message by questioning an evolutionary phenomenon of technology that is evident (Fig. 1) but goes unquestioned. Why do boats with sails look the same? They have sails that are roughly as tall as the length of the hull. They have hulls that are longer than they are wide. Furthermore, they are submerged to a depth that is greater when the hull is wider. The boat has been this way since antiquity. Even more intriguing is that the large boat looks just like the small boat. Why?

Figure 1
figure 1

The geometric similarity of boats with sails, clockwise: ancient Egyptian galley, the essential length scales of the moving body, and modern sailboats (photo: Adrian Bejan).

Full size image

Theory

The reason for all these observations is the human tendency to move more easily on earth1,3. The vehicle architecture that emerges is a reflection of the urge of all its builders and users to move more easily, to have greater access to the surroundings. In recorded times, this tendency gave birth to artifacts (vehicles) in which people encapsulate themselves to acquire greater access. From this idea, the convergent evolution of all boats with sails is deducible.

The boat moves horizontally with the speed Vw on the surface of the water. The wind with the speed Va engages the sail with the force,

$${{\rm{F}}}_{{\rm{a}}} \sim {{\rm{C}}}_{{\rm{D}}}({\rm{HL}}/2)\frac{1}{2}{\rho }_{{\rm{a}}}{{\rm{V}}}_{{\rm{a}}}^{2}$$
(1)

where CD ~ 1 is the drag coefficient, ρa is the air density, and (HL/2) is the sail area: H is the height of the mast, and L is the hull length. The driving force Fa is matched by the drag force experienced by the hull against the water,

$${{\rm{F}}}_{{\rm{w}}} \sim [{{\rm{C}}}_{{\rm{D}}}{{\rm{D}}}_{{\rm{x}}}{{\rm{D}}}_{{\rm{y}}}+{{\rm{C}}}_{{\rm{f}}}({{\rm{D}}}_{{\rm{x}}}+2{{\rm{D}}}_{{\rm{y}}}){\rm{L}}]\frac{1}{2}{\rho }_{{\rm{w}}}{{\rm{V}}}_{{\rm{w}}}^{2}$$
(2)

where Dx is the hull width, Dy is the depth of the submerged portion of the hull, ρw is the water density, and Cf is the skin friction coefficient for turbulent flow, the order of magnitude of which is Cf ~ 10−2 35. Note the two terms in the square brackets: the first accounts for the drag experienced by the hull frontally, as a blunt body, and the second is due to the fluid friction along the hull. As we show later in the discussion of Fig. 2, the forces that propel the boat can vary depending on the angle of attack.

Figure 2
figure 2

Two cases showing the relationship between wind speed (W), apparent wind speed (A), boat speed (S) and apparent wind angle (β). The sails are trimmed to account for apparent wind angle.

Full size image

From the balance between Fw and Fa emerges the ratio Vw/Va, which is larger when the quantity in square brackets in Eq. (2) is smaller. This quantity varies in accord with the two aspect ratios of the configuration, Dx/Dy and Dx/L, subject to the displaced volume of water (DxDyL), which is fixed because it is dictated by the total weight of the boat. It is easy to show analytically that the quantity in the square brackets in Eq. (2) is minimum when

$$\frac{{{\rm{D}}}_{{\rm{x}}}}{{{\rm{D}}}_{{\rm{y}}}} \sim 2$$
(3)

and

$$\frac{{\rm{L}}}{{{\rm{D}}}_{{\rm{x}}}} \sim \frac{{{\rm{C}}}_{{\rm{D}}}}{2{{\rm{C}}}_{{\rm{f}}}} > 1$$
(4)

This settles the question of the shape of the hull and that of most fish: they should be slender in profile, and relatively round when viewed in cross section.

What about the shape of the sail? When the aspect ratios of Eqs. (3, 4) apply, the drag force in the water [Eq. (2)] becomes

$${{\rm{F}}}_{{\rm{w}}} \sim 3{(\frac{2{{\rm{C}}}_{{\rm{f}}}}{{{\rm{C}}}_{{\rm{D}}}})}^{2}{\rm{L}}\,{\rho }_{{\rm{w}}}{{\rm{V}}}_{{\rm{w}}}^{2}$$
(5)

From the balance between Eqs. (1) and (5) we deduce that

$$\frac{{\rm{H}}}{{\rm{L}}} \sim 3{(\frac{2{{\rm{C}}}_{{\rm{f}}}}{{{\rm{C}}}_{{\rm{D}}}})}^{2}\frac{{\rho }_{{\rm{w}}}}{{\rho }_{{\rm{a}}}}{(\frac{{{\rm{V}}}_{{\rm{w}}}}{{{\rm{V}}}_{{\rm{a}}}})}^{2}$$
(6)

which in view of CD ~ 1, Cf ~ 10−2, and ρw/ρa ~ 103, becomes

$$\frac{{{\rm{V}}}_{{\rm{w}}}}{{{\rm{V}}}_{{\rm{a}}}} \sim {(\frac{{\rm{H}}}{{\rm{L}}})}^{1/2}$$
(7)

In the evolutionary pursuit of higher boat speeds Vw, the height of the sail approaches the length of the hull. Expressed in terms of scale analysis, the conclusion is that H and L must have the same scale because Vw and Va represent the same scale (no wind, no travel; fast wind, fast travel).

With the three aspect ratios now predicted (Dx/Dy, L/Dx, H/L) the evolutionary direction of the boat model selected in Fig. 1 is complete, and can be drawn in three dimensions. The shape viewed from above is L/Dx, while from the front and from the side it is respectively Dx/Dy and H/L. There are only three shapes because the configuration of the simplest model (Fig. 1) has only three degrees of freedom. Each of these shapes refers to the external look of the model.

Boat designs are more complicated because in addition to external shape they also have internal structure. The internal structure has additional geometric details, which have increased in number during boat evolution. Three frames from this evolutionary sequence are aligned chronologically in Fig. 3, from two thousand years ago (Egyptian galley) to Columbus crossing the Atlantic (1492) and Napoleon’s navy (1800). In antiquity the internal structure was the simplest: one mast supporting one sail. Over time, the sails and the masts became more numerous as the speed and carrying capacity of the boats increased for the benefit of the people who constructed, owned and operated them. Modern monohulled sailboats align more with the Egyptian galley in that there is typically a single mast. The fastest monohulls in the world have a single mast and achieve maximum speeds with 2–3 sails.

Figure 3
figure 3

The evolution of boats with sails over the past two thousand years: Egyptian galley, Columbus and Napoleon.

Full size image

In Fig. 3 the three boats are presented in frames of the same size in order to stress two additional points. In time, the complexity of the architecture increases as the internal structure morphs. Yet, in every frame the external shapes are the same as those that we predicted for the simplest model without internal structure (Fig. 1).

Features of internal structure can be predicted by continuing the analysis started in Eqs. (1–7). Assume that the lone sail in Fig. 1 is supported by one mast, which is modeled as an elastic rod of height H and diameter d. The mast bends under the horizontal force received from the sail, which is Fa, Eq. (1). The mast is a beam in pure bending, because it is slender enough so that its slenderness H/d exceeds 50.

The highest stresses occur at the base of the mast, where the bending moment is maximum and of order FaH. This moment is balanced by the moment due to the nonuniform distribution of stresses in the mast cross section. The stresses are tensile on the forward (convex) side of the mast in bending, and compressive on the aft (concave) side. If the material is such that σ is the order of magnitude of the highest allowable (tensile and compressive) stresses at the base, then the bending couple in the cross section is of order σd2 × d, where the σd2 are the forces of the couple (tensile and compressive, both aligned with the mast) and d is the arm of the couple, which is transversal to the mast. From the requirement of rotational equilibrium, FaH ~ σd3, we obtain

$$\frac{{{\rm{d}}}^{3}}{{{\rm{H}}}^{2}{\rm{L}}} \sim \frac{{\rho }_{{\rm{a}}}{{\rm{V}}}_{{\rm{a}}}^{2}}{\sigma }$$
(8)

which, in view of Eq. (7), becomes

$$\frac{{\rm{d}}}{{\rm{H}}} \sim {(\frac{{\rho }_{{\rm{a}}}{{\rm{V}}}_{{\rm{a}}}^{2}}{\sigma })}^{1/3}{(\frac{{{\rm{V}}}_{{\rm{a}}}}{{{\rm{V}}}_{{\rm{w}}}})}^{2/3}$$
(9)

To summarize, the predicted evolutionary design has the three external shapes discussed previously, plus one internal shape, d/H, the mast slenderness. One formula, Eq. (9), governs the evolution of this technology, past and future. A stronger material (larger σ) makes a more slender (thinner, lighter) mast, which in turn decreases the dead weight of the vessel (and the submerged portion of the hull), reduces hull friction and increases the boat speed.

Comparison with Current Designs

In the modern era, physics principles have played a guiding role in the improvements that have been made in the design of boats with sails. The icon of the central role of physics in boat design is Euler’s entry36 in the 1727 contest for the King’s prize for the solution to the nautical problem to determine the best way to place the masts on vessels, and the relation between their positions and the number and height of the masts. Since then, fluid dynamics and naval engineering grew as scientific domains, as did naval vessel technology before and after the advent of steam power37,38,39,40,41,42. In this section we compare the current state of sail boat architecture with the design features predicted theoretically.

Table 1 consists of 96 single hulled sailboats with a variety of models, years, dimensions, weights, and designs43. The variables Dx, Dy, L, and H correlate to Beam, Hull Depth, Overall Length, and Height, respectively, which are defined in Fig. 4. The displacement [kg] is the mass of the boat, which is equal to the mass of the water that is displaced by the boat.

Table 1 Sailboat model data.
Full size table
Figure 4
figure 4

The main dimensions used in yacht construction.

Full size image

PHRF is the performance indicator, which stands for Performance Handicap Racing Fleet. PHRF is a handicapping standardization that equates the performance of different boats. It is designed to rate the boat design characteristics only, and is impartial to the talent of the skipper and crew. With this in mind, the skipper and crew who sail the best overall race from boat handling and tactical perspective should be awarded as the victors.

Boats are given PHRF ratings based on empirical data including dimensional characteristics, materials, past race finishes, similar boats scaling, and comparisons to other handicap systems. The PHRF system also accounts for three ranges of wind conditions (light, moderate, heavy breeze) by utilizing distinct constants for the ratings formula. Like all handicapping systems, PHRF is imperfect due to the opportunity to inject subjectivisms but is the most-widely accepted handicapping systems in the U.S.

For the scope of this article, it should be known that the lower or negative ratings correlate to faster boats. For instance, the fastest boat in Table 1 is the DK 46 with a PHRF rating of 30, and the slowest is the Bullseye with a rating of 360. In moderate conditions, the DK 46 should be 1.75 times faster boat-for-boat than the Bullseye when utilizing the correction factor formula. The correction factor is then applied to the overall time, generating a “corrected time” that generates a one-to-one comparison between the different boats. The boat with the lowest corrected time is the winner of the race.

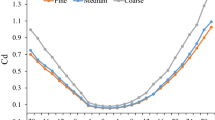
Plotted in Figs. 5–7 are the actual measurements extracted from Table 1. The three figures show the aspect ratios Dx/Dy, L/Dx and H/L versus the boat displacement. Noteworthy is that the three aspect ratios do not depend on the displacement. This means that the main aspects of the configuration did not change over time, as the displacement increased in history (cf. Fig. 3).

Figure 5
figure 5

The ratio Dx/Dy (beam/draft) according to the data presented in Table 1.

Full size image
Figure 6
figure 6

The ratio L/Dx (overall length/beam) according to the data presented in Table 1.

Full size image
Figure 7
figure 7

The ratio H/L (height/overall length) according to the data presented in Table 1.

Full size image

The results provided by Eqs. (3), (4) and (7) predict the convergent evolution observed in Figs. 5–7. The geometrical ratios that define the shape of the boats with sails are permanent characteristics over the ages and cultures.

Due to the number of unique designs, calculating the exact performance of a yacht is dependent on a significant number of intrinsic properties as well as external factors. Different boats achieve peak performances at specified degrees from the wind direction in specific conditions. ‘Degrees’ refers to the boat pointing angle relative to the true wind direction. Ultimately, as the boat moves faster and increases the apparent wind velocity (wind speed plus boat speed), the apparent wind angle becomes small relative to the true wind angle.

The fastest boats in the world are almost always sailing ‘upwind’ (with sails pulled in closer to the hull mid-line) because they can generate significant boat speed relative to the wind speed. This phenomenon is depicted in Fig. 2. Note the two distinct instances, where the boat speed (S1 < S2) and the angle of attack (β1 > β2) change, while the true wind speed W is constant. The apparent wind vector A increases with the boat speed when the boat direction and the true wind vector do not change. The sail’s (or airfoil’s) leading edge will point in the direction of the apparent wind to generate lift. As the boat speed continues to increase, the apparent wind angle moves forward until the sail is pulled to centerline (cf., Fig. 2), at which point the boat has reached is maximum speed for its relative boat angle from the true wind direction.

There are various calculations to generate boat speed using a characteristic dimension. One example is the critical velocity or \({{\rm{V}}}_{{\rm{c}}}\cong 1.25\,{({\rm{LWL}})}^{1/2}\). This formula follows from the fact that the bow of a sailboat moving through the water produces a transverse wave, and at critical velocity the wave extends the length of the waterline, which essential traps the boat in the trough, not allowing it to escape the transverse wave, and so capping its velocity. There are various circumstances that can allow a boat to go faster than Vc, such as “surfing” external waves or utilizing hydro-foils to lift the hull out of the water.