Abstract
Recent discovery of the pyrite FeO2, which can be an important ingredient of the Earth’s lower mantle and which in particular may serve as an extra source of water in the Earth’s interior, opens new perspectives for geophysics and geochemistry, but this is also an extremely interesting material from physical point of view. We found that in contrast to naive expectations Fe is nearly 3+ in this material, which strongly affects its magnetic properties and makes it qualitatively different from well known sulfide analogue - FeS2. Doping, which is most likely to occur in the Earth’s mantle, makes FeO2 much more magnetic. In addition we show that unique electronic structure places FeO2 “in between” the usual dioxides and peroxides making this system interesting both for physics and solid state chemistry.
Similar content being viewed by others
Introduction
Recent discovery of a new iron oxide FeO2, which does not exist at normal conditions, but can be stabilized at a very high pressure (76 GPa) and temperature (1800 K)1 may dramatically shift our understanding of how Earth is formed and what was a source of oxygen and water in interior of our planet. FeO2 is expected to appear in the Earth’s lower mantle below 1800 km, according to1 in the pyrite structure, and start to dominate over other Fe oxides at higher pressures. The composition of the mantle is extremely important for the seismology, since it determines convection processes. There were proposed a number of structural models based on different ratio of ferropericlase (a solid solution of FeO and MgO), bridgmanite (Mg,Fe,Al)(Al,Fe,Si)O3, (Mg,Fe)2SiO4 olivine and other compounds2,3,4,5, but none of them took into account the existence of FeO2. Moreover, physical properties of this material are completely unexplored. One might expect that they can be highly unusual, since on one hand Fe ion in FeO2 formally should have exceptionally high oxidation state, 4+. Since the O-O distance in FeO2 is 1.89 Å it is not likely that there can be a strong bonding between the O ions, like in molecular oxygen (where the O-O bond distance is 1.21 Å) and one may indeed expect that Fe ions will adopt 4+ valence state and then FeO2 is in a negative charge transfer regime6,7,8,9. This may result in self-doping10 and also to bond or charge disproportionation11,12, inversion of the crystal field splitting13 or nontrivial magnetic structures. On the other hand, the presence of the ligand-ligand dimers may also strongly affect physical properties of FeO2 as it does in the actual pyrite FeS2 (“the fool’s gold”). However, O-O distance in FeO2 is 1.89 Å, much larger than in molecular oxygen (1.21 Å) or (O2)2− ion as in the usual peroxides like BaO2, MgO2 (1.49 Å).
Iron peroxide was found to have the same pyrite crystal structure as FeS2 1, see Fig. 1a, and there is not much difference between oxygen and sulfur from chemical point of view. Thus, it is tempting to consider FeO2 as a complete analogue of FeS2 14. Since FeS2 is known to be a diamagnetic insulator with Fe ions adopting 2+ valence state15,16,17, one might expect that the same is true for FeO2. The first indication that such a picture is oversimplified follows from the recent theoretical study14, where FeO2 was found to be metallic at the pressures where it does exist.
In the present paper we describe electronic and magnetic properties of FeO2. We show that FeO2 is completely different from FeS2, and so are the physical properties of these compounds. The oxidation state of Fe ion in FeO2 is not 2+, as in FeS2, but close to 3+. This strongly affects magnetic properties of FeO2, since having 3d 5 electronic configuration, Fe3+ ions may have a magnetic moment. Our comprehensive theoretical calculations using combination of the density functional and dynamical mean-field theories (DFT + DMFT) demonstrate that there is indeed a highly nontrivial temperature dependence of the magnetic susceptibility in FeO2. We found out that the origin of the difference in magnetic properties between FeO2and FeS2 and of the metallic character of FeO2 is a much smaller bonding-antibonding splitting for ligand σ orbitals in the peroxide dimer O2 as compared with S2, and a total shift of oxygen 2p levels relative to 3p levels of sulfur. This feature of the electronic structure is rather general and important for other dioxides, which can exist in Earth’s mantle or in inner parts of exoplanets.
We start with FeS2, electronic and magnetic properties of which are well understood. As discussed above, one might naively expect to have Fe4+ ions with 3d 4 electronic configuration in FeS2, since usually sulfur has a valence 2-. This would shift Fe 3d band very low in energy, below S 3p, and would result in a self-doping and a metallic conductivity7, which strongly disagrees with the experimental fact that FeS2 is a semiconductor16,17.
The explanation of this contradiction lies in the specific features of its crystal structure, namely the presence of the S2 dimers. There are sulfurs 3p orbitals, which are directed exactly to each other in these dimers. They form such a strong bond that the antibonding \({{\sigma }}_{p}^{\ast }\) orbitals turn out to be higher in energy than the Fe e g orbitals, see Fig. 1(b2). This leads to a formal valency of sulfur “1-”, (or to (S2)2− dimers), and Fe ions become 2+ with the 3d 6 electronic configuration. Fe ions are in the ligand octahedra in pyrite structure. Strong crystal field splitting between the t 2g and e g bands (~3.5 eV in case of FeS2, see Supplemental materials - SM18) counteracts the Hund’s rule and stabilizes the low spin configuration with all six 3d electrons occupying t 2g sub-shell. This makes FeS2 diamagnetic and insulating19.
The electronic structure of FeO2 is rather different from a sulfide counterpart. We sketched how this difference appears in Fig. 1c (while the results of the actual calculations performed within generalized gradient approximation, GGA, as well as the details of such calculations are presented in Fig. S1 in SM18), starting from the hypothetical FeO2 having FCC lattice (like NaCl), where O ions do not form dimers and where there are basically three bands O p, Fe t 2g , and Fe e g , see Fig. 1(c1).
First of all, as follows from our GGA calculations, the oxygen 2p levels are shifted down relative to the Fe 3d states, as compared with the 3p levels of sulfur. Besides, as was mentioned above, the presence of the ligand-ligand dimers in real FeO2 results in bonding-antibonding splitting, but since oxygen 2p orbitals are much less extended than sulfur 3p orbitals, this bonding-antibonding splitting in the O2 dimer is expected to be much smaller. As a result the antibonding O \({{\sigma }}_{p}^{\ast }\) orbital appears not above e g (like in FeS2), but exactly in the place, where Fe t 2g bands lie, see Fig. 1(c2). Then, first of all, part of the Fe t 2g electrons would be transferred to oxygens, shifting Fe valence in the direction of 3+. Second, the hybridization between Fe 3d and O \({{\sigma }}_{p}^{\ast }\) orbitals again makes bonding and antibonding combinations, which are labeled as \({t}_{2g}+{{\sigma }}_{p}^{\ast }\) and \({t}_{2g}-{{\sigma }}_{p}^{\ast }\) in Fig. 1(c3) respectively. The density of states (DOS) plot in the vicinity of the Fermi energy as obtained in conventional GGA is presented in Fig. 2(a). These \({t}_{2g}+{{\sigma }}_{p}^{\ast }\) and \({t}_{2g}-{{\sigma }}_{p}^{\ast }\) bands are centered at −2 and 1 eV in Fig. 2(a). Note that these bands have nearly the same contributions from Fe t 2g and O 2p (\({{\sigma }}_{p}^{\ast }\)) states. Moreover, it is clear that peaks below and above the Fermi level are not bonding and antibonding, since there is no contribution from O 2p band below E F . These are nonbonding and antibonding states.
This salient feature of FeO2, that the antibonding σ * orbital falls exactly into the Fe t 2g band, determines the main physical properties of FeO2, which are very different from FeS2, see Table 1. First of all, since there appear additional bands at the Fermi level, while the number of electrons is the same, FeO2 is not a band insulator (as FeS2), but a metal.
There are eight t 2g bands, each doubly degenerate with respect to spin, below the Fermi energy for the unit cell consisting of four formula units (f.u.), which are occupied by 4 electrons per each Fe ion (Fig. S1 in SM18). In addition there are four bonding \({t}_{2g}+{{\sigma }}_{p}^{\ast }\) bands with nearly 50% contribution of the Fe t 2g states (see partial DOS presented in Fig. 2), which adds approximately one more electron to each Fe ions. As a result Fe ions in FeO2 are nearly 3+ with 3d 5 electronic configuration, while in FeS2 they are 2+.
In contrast to Fe2+, which is nonmagnetic with \({t}_{2g}^{6}\) configuration at large pressure, Fe3+ ion even in the low-spin state has a magnetic moment. Moreover, the oxygen σ * states are half-filled in FeO2, and thus they can also contribute to the total magnetic moment.
Second, the Fermi level appears to be in a very specific position. On one hand it is almost in the pseudogap, so that the Stoner criterion for ferromagnetism (FM) is formally not fulfilled, and this is the reason why magnetic solutions does not survive in the GGA (we also checked stability of magnetic solutions at other q-vectors, corresponding to AFM-I and AFM-II magnetic structure of FCC lattice of Fe ions20). On the other hand it is just on the border line between bands corresponding to localized t 2g electrons and antibonding molecular \({t}_{2p}-{{\sigma }}_{p}^{\ast }\) states. This is very important for magnetic properties of stoichiometric and non-stoichiometric FeO2 as we will show latter.
While conventional DFT is exceptionally useful for understanding of the basics of the electronic structure in FeO2, it does not take into account strong Coulomb correlations, which are known to be important for description of the physical properties of many transition metal compounds. We treated correlation effects using the DFT + DMFT method21. Hubbard U was calculated to be 6 eV, \({J}_{H}=0.9\) eV, other details can be found in SM18.
Correlation effects manifest themselves basically via the renormalization of the GGA DOS near the Fermi level, \({m}^{\ast }/m\)~1.2–1.6 (depending on the orbital), resulting spectral functions are shown in Fig. S2 of SM. FeO2 is a bad metal for experimental pressure of 76 GPa. There are 4.8 electrons in the t 2g shell, which certifies that Fe is 3+ in FeO2. The local magnetic moment \(\langle \sqrt{{m}_{z}^{2}}\rangle \) was found be 1.5 \({\mu }_{B}\). The contribution from the t 2g orbitals to the total local magnetic moment, \({\langle {m}_{z}^{2}\rangle }_{{t}_{2g}}=1.08{\mu }_{B}^{2}\), exactly corresponds to the low spin state of 3d 5 configuration. There is, however, also an additional contribution, \({\langle {m}_{z}^{2}\rangle }_{{e}_{g}}=1.04{\mu }_{B}^{2}\), due to a partial polarization of the ligand electrons residing e g shell of transition metal (see detailed discussion in Supplemental materials). In spite of the fact that there are magnetic moments on Fe ions, they do not order, so that FeO2 stays paramagnetic down to 190 K (we checked FM and AFM-I). Even lower temperatures can be reached in our calculations by using a truncated Hamiltonian, which includes only Fe t 2g and O 2p states (this choice of impurity orbitals gives the same spectral functions in vicinity of the Fermi level and very similar \(\chi (T)\) as full 3d Hamiltonian). In this case we were able to go down to 60 K, and again FeO2 does not order in our calculations even at these temperatures. This may seem somewhat surprising since having a rather large bandwidth (and hence hopping parameters) one might expect large superexchange interaction between Fe ions, if spins would have been localized.
In order to estimate the degree of the spin localization we calculated the analytical continuation on real frequency of the spin-spin correlator \(\langle {S}_{z}(i\omega ){S}_{z}(o)\rangle ={\int }_{0}^{\mathrm{1/}{k}_{B}T}d\tau \langle {S}^{z}(\tau ){S}^{z}(o)\rangle {e}^{i{\omega }_{n}\tau }\), where \(\tau \) is an imaginary time, see right panel in Fig. 3 22,23. The width of this correlator is inversely proportional to the lifetime of a magnetic moment. For example in a pure metallic iron, where \({t}_{2g}-{e}_{g}\) crystal field splitting is small, iron ion is in a high-spin state. The magnetic moment can be considered to be localized, with the full width at half maximum (FWHM) of about 0.2 eV for the less localized \(\gamma \)-Fe and 0.1 eV for the more localized \(\alpha \)-Fe22,24. From the inset of Fig. 3 one may see that in FeO2 FWHM of the spin-spin correlator is ~3 eV, which demonstrates that the magnetic moments can hardly be considered as localized.
Results of the DFT + DMFT calculations. Left panel: uniform magnetic susceptibility for pure FeO2 and \(\frac{1}{2}\) hole/Fe doping (red circles). Inset shows magnetic susceptibility for 0.5 hole per Fe atom as a function of 1/T. Right panel shows local magnetic susceptibility as a function of frequency for different doping.
In DMFT one can calculate the uniform magnetic susceptibility \({\chi }_{u}(T)\) as a response to an external magnetic field, which is introduced via Zeeman splitting \(\delta E\) in the Hamiltonian:
Here m is the magnetization, \({n}^{\uparrow }\) and \({n}^{\downarrow }\) are total occupations for spin up and down. This direct calculation of the uniform magnetic susceptibility, \({\chi }_{u}(T),\) shows that it has a nontrivial temperature dependence. Namely, with increasing temperature \({\chi }_{u}\) first decreases (for \(T < {T}^{\ast }\,=\) 750 K), and then starts to increase almost linearly above \({T}^{\ast }\), which resembles the behaviour of the pnictides25. Detailed analysis of these data18 shows that such an unusual for 3D system behavior is due to a specific position of the Fermi level in between the localized t 2g and antibonding \({t}_{2g}-{\sigma }_{p}^{\ast }\) states. At low temperature the particle-hole excitations occur within the localized Fe t 2g states and \({\chi }_{u}(T)\) goes down with temperature, resembling the Curie-Weiss law. Increasing temperature further (\(T > {T}^{\ast }\) K), we start to excite molecular-like \({t}_{2g}-{\sigma }_{p}^{\ast }\) states, which leads to a completely different temperature dependence.
This means that the electron and hole doping, which is likely to occur in Earth’s mantle, would result in a very different temperature dependences of magnetic susceptibility, since we shift the Fermi level to the peaks corresponding to qualitatively different states (localized and molecular-like). There are many different elements besides Fe (5.8%) and O (44.8%) in the Earth’s mantle, and one may expect that Mg (~22.8%), Si (~21.5%), Ca (~2.3%) or Na (0.3%)26 may dope FeO2 and change its properties dramatically. Indeed, the Fermi level in stoichiometric FeO2 is on the steep slope of a large peak in DOS, and changing its position we strongly affect both magnetic and electronic properties.
The electron doping will shift the Fermi level to antibonding molecular-like \({t}_{2g}-{\sigma }_{p}^{\ast }\) states, which is unlikely to provide a large magnetic response in the simplest rigid-band shift model. Moreover, by doing this we transform Fe ion into the nonmagnetic low-spin \(3{d}^{6}\) configuration, corresponding to the 2+ oxidation state, so that only a small electron doping can increase magnetic moment. In addition the electron doping is rather unfavourable from structural point of view: the population of the strongly antibonding \({t}_{2g}-{\sigma }_{p}^{\ast }\) orbital would significantly weaken O2 dimers, existing in the pyrite structure. Thus, at first sight the hole doping is expected to be much more effective for making FeO2 magnetic: the Fermi level would then be shifted to the large peak corresponding to localized Fe t 2g electrons.
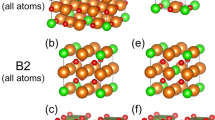
We checked different types of hole and electron dopings by the GGA calculations (for ferromagnetic order) performing full structural optimization, starting from the pyrite structure and substituting 25% of Fe by different ions such ions as Mg, Si, and Na. Mg doping formally changes valence of the peroxide O2 group from 3- in FeO2 to 2- in MgO2 (see Fig. 4(c) and (d)), but it has no influence either on band structure or on magnetic properties of the system: unoccupied σ * band corresponding to the Mg(O2)2− unit appears just above the Fermi level and does not provide any holes to the Fe ions. In NaO2 superoxide the O2 “molecule” is 1-, see Fig. 4(b) and also ref.27, and hence by Na we depopulate O \({\pi }^{\ast }\) bond, which will be immediately refilled by the Fe t 2g electrons. This leads to the shift of the Fermi level downwards, see Fig. 2b, and results in the magnetic instability. In the GGA calculations the magnetic moments on Fe ions were found to be ~0.4 \({\mu }_{B}\). Si doping keeps FeO2:Si nonmagnetic, but only in unrelaxed crystal structure. After lattice optimization there appears two very different O2 dimers, which help to form magnetic moment ~0.4 \({\mu }_{B}\) even in the case of the light electron doping. But the most effective are Fe vacancies (25%), which give magnetic ground state in the GGA calculations with magnetic moments ~0.6 \({\mu }_{B}\).
Thus, we see that there are plenty of possibilities for FeO2 to be magnetic due to different types of doping or because of non-stoichiometry. It is hard to expect, however, that FeO2 would order magnetically in the Earth’s mantle, because of very high temperatures, ~1000–2000 K, but even in a paramagnetic state it may still provide local magnetic moments. The direct DFT + DMFT calculations within the rigid-band shift approximation (as one can see from Fig. 2b, the band structure does not change dramatically with doping) show the drastic increase of the uniform magnetic susceptibility with hole doping, see Fig. 3. it is now Curie-Weiss like in a wide temperature range and the spin-spin correlation function demonstrates an increase of the local magnetic moments lifetime (i.e. decrease of the width of the correlator, see inset in Fig. 3) with doping.
In addition to a possible importance of our findings for geoscience, FeO2 represents an exceptional interest also for physics and solid state chemistry, since it lies on the borderline between the stable dioxides of transition metals, such as TiO2 VO2, CrO2 etc., and equally stable oxides and sulfides having pyrite structure, such as NaO2, KO2, FeS2 etc. FeO2 may thus be considered as a “bridge” between dioxides and peroxides/disulfides, and it displays properties of both.
There is a well known concept in physics, introduced by Zaanen, Sawatzky and Allen9, that going along a row in the periodic table from the left to the right, or increasing valence of a metal in a transition metal oxide, we go over from the Mott insulator, where the band gap is defined by Hubbard U, to a charge-transfer regime, where it is given by the charge transfer (CT) from a ligand to a metal, \({{\rm{\Delta }}}_{CT} > 0\), and finally to the state, where \({{\rm{\Delta }}}_{CT}\) becomes negative with ligands donating some of their electrons to a metal (so called self-doping)8,9.
In peroxides the situation with the CT energy is “inverted” from the beginning: as we have seen, in FeS2 part of electrons are transferred from sulfur to Fe. CoS2, NiS2, MgO2, KO2 and many other materials are just the same: ligand σ * and sometimes even \({\pi }^{\ast }\) orbitals donate (see Fig. 4) at least one electron for a metal, i.e. oxygen is 1- or even 1/2-. With FeO2 one returns to normal transition metal oxides, where oxygen’s valency is 2-, but there is still one step to make since O is 1.5- in FeO2. Thus, we see that FeO2 indeed lies “in between” oxides and peroxides/disulfides, which makes it an especially interesting material from physical point of view.
A simple qualitative difference between normal (di)oxides and peroxides is the following: On one hand, when the main “structural unit” in a system is a single O ion, like in dioxides of the type of TiO2, VO2, its “natural” state is O2−, and counting from that, we see that e.g. in FeO2 the CT energy would be negative, \({{\rm{\Delta }}}_{CT} < 0\), i.e. the electrons would be transferred from O2− to Fe (as it happens already in CrO2 10). But in peroxides, as well as, e.g., in FeS2, the natural “structural unit” is the O2 or S2 dimer. Such dimer can be in different charge states: neutral O2 molecule, Fig. 4a; (O2)− molecular ion (say in NaO2, KO2), Fig. 4b; or (O2)2− ion as in MgO2, Fig. 4c.
The more electrons we put on such a dimer, the more we fill antibonding states, which gradually destabilizes the very O2 dimers. But till (O2)2− it is still reasonably harmless, we fill “weakly” antibonding states (\({\pi }^{\ast }\)), see Fig. 4. But as soon as one starts to occupy the upper σ * states, the very dimers start to become more and more destabilised, which we indeed see in FeO2: the O-O distance in (O2)3− dimers is 1.89 Å1 - much larger than 1.49 Å for (O2)2− dimer in MgO2 28 or 1.32 Å for (O2)− in NaO2 29. Already MgO2, having 4 electrons on antibonding \({\pi }^{\ast }\) orbitals, see Fig. 4(c), readily decomposes at zero pressure30. In FeO2 we lose even more energy occupying antibonding σ * orbital, see Fig. 4(d). This makes FeO2 even less stable in the pyrite structure, than MgO2, so that it can be stabilised only at a very high pressure.
Summarising, we see that the recently discovered pyrite-like FeO2 1 is even more exotic than it was initially thought. Unexpected valence states, nontrivial magnetic properties, stabilization of local magnetic moments by non-stoichiometry or doping by such abundant constituents of Earth’s mantle such as Si (and Na) and finally its special place between (di)oxides and peroxides make FeO2 extremely interesting not only for geoscience, but also for the condensed matter physics and solid state chemistry.
Data availability statement
No datasets were generated or analysed during the current study.
References
Hu, Q. et al. FeO2 and FeOOH under deep lower-mantle conditions and Earth’s oxygen–hydrogen cycles. Nature 534, 241–244, https://doi.org/10.1038/nature18018 (2016).
Dziewonski, A. M. & Anderson, D. L. Preliminary reference Earth model. Physics of the Earth and Planetary Interiors 25, 297–356, https://doi.org/10.1016/0031-9201(81)90046-7 (1981).
Irifune, T. et al. Iron Partitioning and Density Changes of Pyrolite in Earth{\textquoteright}s Lower Mantle. Science 327, 193–195, https://doi.org/10.1126/science.1181443 (2010).
Murakami, M., Ohishi, Y., Hirao, N. & Hirose, K. A perovskitic lower mantle inferred from high-pressure, high-temperature sound velocity data. Nature 485, 90–94, https://doi.org/10.1038/nature11004 (2012).
Wang, X., Tsuchiya, T. & Hase, A. Computational support for a pyrolitic lower mantle containing ferric iron. Nature Geosci 8, 556–559, https://doi.org/10.1038/ngeo2458 (2015).
Khomskii, D. Unusual valence, negative charge-transfer gaps and self-doping in transition-metal compounds. Lithuanian Journal of Physics 37, 65 (1997).
Sawatzky, G., Green, R., Mall, E., Bc, V. & Vt, C. The Explicit Role of Anion States in High-Valence Metal Oxides. In Pavarini, E., Koch, E., van den Brink, J. & Sawatzky, G. (eds.) Quantum Materials: Experiments and Theory Modeling and Simulation, vol. 6, 1–36 (Verlag des Forschungszentrum, Jülich, 2016).
Khomskii, D. I. Transition Metal Compounds (Cambridge University Press, 2014).
Zaanen, J., Sawatzky, G. & Allen, J. Band gaps and electronic structure of transition-metal compounds. Physical Review Letters 55, 418–421, https://doi.org/10.1103/PhysRevLett.55.418 (1985).
Korotin, M. A., Anisimov, V. I., Khomskii, D. I. & Sawatzky, G. A. CrO$_2$: A Self-Doped Double Exchange Ferromagnet. Physical Review Letters 80, 4305, https://doi.org/10.1103/PhysRevLett.80.4305 (1998).
Bisogni, V. et al. Ground-state oxygen holes and the metal–insulator transition in the negative charge-transfer rare-earth nickelates. Nature Communications 7, 13017, https://doi.org/10.1038/ncomms13017 (2016).
Kawakami, T. et al. Two-Step Suppression of Charge Disproportionation. J. Phys. Soc. Jpn. 85, 034716, https://doi.org/10.7566/JPSJ.85.034716 (2016).
Ushakov, A. V., Streltsov, S. V. & Khomskii, D. I. Crystal field splitting in correlated systems with negative charge-transfer gap. J. Phys.: Condens. Matter 23, 445601, https://doi.org/10.1088/0953-8984/23/44/445601 (2011).
Jang, B. G., Kim, D. Y. & Shim, J. H. Metal-insulator transition and the role of electron correlation in FeO 2. Phys. Rev. B 95, 075144, https://doi.org/10.1103/PhysRevB.95.075144 (2017).
Burgardt, P. & Seehra, M. S. Magnetic susceptibility of iron pyrite (FeS2) between 4.2 and 620 K. Solid State Communications 22, 153 (1977).
Bullett, D. W., Khan, M. A., Schlegel, A. & Wachter, P. Optical properties, phonons and electronic structure of iron pyrite (FeS2). J. Phys. C: Solid State Phys. 9, 3363 (1976).
Ferrer, I. J., Nevskaia, D. M., las Heras, C. & Sanchez, C. About the band gap nature of FeS 2 as determined from optical and photoelectrochemical measurements. Solid State Communications 74, 913–916 (1990).
see Supplemental materials.
Eyert, V., Höck, K.-H., Fiechter, S. & Tributsch, H. Electronic structure of FeS2: The crucial role of electron-lattice interaction. Physical Review B 57, 6350–6359, https://doi.org/10.1103/PhysRevB.57.6350 (1998).
Smart, J. S. Effective Field Theories of Magnetism. Studies in physics and chemistry, 216 (Saunders, 1966).
Anisimov, V. I., Poteryaev, A. I., Korotin, M. A., Anokhin, A. O. & Kotliar, G. First-principles calculations of the electronic structure and spectra of strongly correlated systems: dynamical mean-field theory. Journal of Physics: Condensed Matter 9, 7359 (1997. http://iopscience.iop.org/0953-8984/9/35/010
Katanin, A. A. et al. Orbital-selective formation of local moments in alpha-iron: First-principles route to an effective model. Physical Review B - Condensed Matter and Materials Physics 81, 045117, https://doi.org/10.1103/PhysRevB.81.045117 (2010).
Georges, A., Krauth, W. & Rozenberg, M. J. Dynamical mean-field theory of strongly correlated fermion systems and the limit of infinite dimensions. Reviews of Modern Physics 68, 13–125, https://doi.org/10.1103/RevModPhys.68.13 (1996).
Igoshev, P. A., Efremov, A. V., Poteryaev, A. I., Katanin, A. A. & Anisimov, V. I. Magnetic fluctuations and effective magnetic moments in γ-iron due to electronic structure peculiarities. Phys. Rev. B 88, 155120, https://doi.org/10.1103/PhysRevB.88.155120 (2013).
Skornyakov, S. L., Katanin, A. A. & Anisimov, V. I. Linear-Temperature Dependence of Static Magnetic Susceptibility in LaFeAsO from Dynamical Mean-Field Theory. Phys. Rev. Lett. 106, 47007, https://doi.org/10.1103/PhysRevLett.106.047007 (2011).
Jackson, I. The Earth’s Mantle: Composition, Structure, and Evolution (Cambridge University Press, 2000). https://books.google.ru/books?id=RlixwcPYF2AC.
Solovyev, I. V., Pchelkina, Z. V. & Mazurenko, V. V. Magnetism of sodium superoxide. CrysEngComm 16, 522, https://doi.org/10.1039/b000000x (2014).
Vannerberg, N.-G. The Formation and Structure of Magnesium Peroxide. PArk. Kern. 14, 99 (1959).
Ziegler, M., Rosenfeld, M., Känzig, W. & P, F. Strukturuntersuchungen an Alkalihyperoxiden. Helv. Phys. Acta 57, 49 (1976).
Wriedt, H. A. TheMg-O (Magnesium-Oxygen) system. Bull. Alloy Phase Diagr. 8, 227 (1987).
Acknowledgements
We thank V. Irkhin and I. Mazin for various stimulating discussions. The study of electronic and magnetic properties of FeO2 performed by S.V.S. was supported by the grant of the Russian Science Foundation (project no. 17-12-01207), while work of D.I.Kh. was supported by the Deutsch Forschungsgemeinschaft through the CRC 1238 program.
Author information
Authors and Affiliations
Contributions
S.V.S. conceived the model describing electronic structures of FeO2 and FeS2. S.V.S. and D.I.Kh. discussed and analysed implications of features of the electronic structure for physical properties of FeO2. The DFT calculations were performed by S.V.S. and A.O.Sh. The DFT + DMFT calculations were carried out by A.O.Sh.; A.I.P., A.O.Sh., S.V.S., and S.L.S. analysed magnetic properties of FeO2. S.V.S. and D.I.Kh. wrote the manuscript with help of all co-authors.
Corresponding author
Ethics declarations
Competing Interests
The authors declare that they have no competing interests.
Additional information
Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Electronic supplementary material
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Streltsov, S.S., Shorikov, A.O., Skornyakov, S.L. et al. Unexpected 3+ valence of iron in FeO2, a geologically important material lying “in between” oxides and peroxides. Sci Rep 7, 13005 (2017). https://doi.org/10.1038/s41598-017-13312-4
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41598-017-13312-4
This article is cited by
-
Pressure-induced large volume collapse and possible spin transition in HP-PdF2-type FeCl2
Physics and Chemistry of Minerals (2024)
-
Solid state chemistry for developing better metal-ion batteries
Nature Communications (2020)
-
Altered chemistry of oxygen and iron under deep Earth conditions
Nature Communications (2019)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.