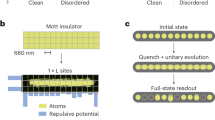
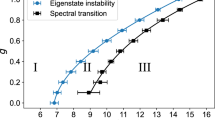
Abstract
Phase transitions are driven by collective fluctuations of a system’s constituents that emerge at a critical point1. This mechanism has been extensively explored for classical and quantum systems in equilibrium, whose critical behaviour is described by the general theory of phase transitions. Recently, however, fundamentally distinct phase transitions have been discovered for out-of-equilibrium quantum systems, which can exhibit critical behaviour that defies this description and is not well understood1. A paradigmatic example is the many-body localization (MBL) transition, which marks the breakdown of thermalization in an isolated quantum many-body system as its disorder increases beyond a critical value2,3,4,5,6,7,8,9,10,11. Characterizing quantum critical behaviour in an MBL system requires probing its entanglement over space and time4,5,7, which has proved experimentally challenging owing to stringent requirements on quantum state preparation and system isolation. Here we observe quantum critical behaviour at the MBL transition in a disordered Bose–Hubbard system and characterize its entanglement via its multi-point quantum correlations. We observe the emergence of strong correlations, accompanied by the onset of anomalous diffusive transport throughout the system, and verify their critical nature by measuring their dependence on the system size. The correlations extend to high orders in the quantum critical regime and appear to form via a sparse network of many-body resonances that spans the entire system12,13. Our results connect the macroscopic phenomenology of the transition to the system’s microscopic structure of quantum correlations, and they provide an essential step towards understanding criticality and universality in non-equilibrium systems1,7,13.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
Data availability
The data that support the findings of this study are available in the Dataverse repository at https://doi.org/10.7910/DVN/E2ROXU.
References
Täuber, U. C. Phase transitions and scaling in systems far from equilibrium. Annu. Rev. Condens. Matter Phys. 8, 185–210 (2017).
Basko, D. M., Aleiner, I. L. & Altshuler, B. L. On the problem of many-body localization. Ann. Phys. 321, 1126 (2006).
Pal, A. & Huse, D. A. Many-body localization phase transition. Phys. Rev. B 82, 174411 (2010).
Serbyn, M., Papic, Z. & Abanin, D. A. Local conservation laws and the structure of the many-body localized states. Phys. Rev. Lett. 111, 127201 (2013).
Huse, D. A., Nandkishore, R. & Oganesyan, V. Phenomenology of fully many- body-localized systems. Phys. Rev. B 90, 174202 (2014).
D’Alessio, L., Kafri, Y., Polkovnikov, A. & Rigol, M. From quantum chaos and eigenstate thermalization to statistical mechanics and thermodynamics. Adv. Phys. 65, 239–362 (2016).
Abanin, D. A., Altman, E., Bloch, I. & Serbyn, M. Ergodicity, entanglement and many-body localization. Preprint at https://arxiv.org/abs/1804.11065 (2018).
Schreiber, M. et al. Observation of many-body localization of interacting fermions in a quasirandom optical lattice. Science 349, 842–845 (2015).
Smith, J. et al. Many-body localization in a quantum simulator with programmable random disorder. Nat. Phys. 12, 907–911 (2016).
Choi, J. et al. Exploring the many-body localization transition in two dimensions. Science 352, 1547–1552 (2016).
Lukin, A. et al. Probing entanglement in a many-body–localized system. Science 364, 256–260 (2019).
Potter, A. C., Vasseur, R. & Parameswaran, S. A. Universal properties of many-body delocalization transitions. Phys. Rev. X 5, 031033 (2015).
Khemani, V., Lim, S. P., Sheng, D. N. & Huse, D. A. Critical properties of the many-body localization transition. Phys. Rev. X 7, 021013 (2017).
Neill, C. et al. Ergodic dynamics and thermalization in an isolated quantum system. Nat. Phys. 12, 1037–1041 (2016).
Kaufman, A. M. et al. Quantum thermalization through entanglement in an isolated many-body system. Science 353, 794–800 (2016).
Agarwal, K., Gopalakrishnan, S., Knap, M., Müller, M. & Demler, E. Anomalous diffusion and Griffiths effects near the many-body localization transition. Phys. Rev. Lett. 114, 160401 (2015).
Setiawan, F., Dong, L. D. & Pixley, J. H. Transport properties across the many-body localization transition in quasiperiodic and random systems. Phys. Rev. B 96, 104205 (2017).
Vosk, R., Huse, D. A. & Altman, E. Theory of the many-body localization transition in one-dimensional systems. Phys. Rev. X 5, 031032 (2015).
Dumitrescu, P. T., Vasseur, R. & Potter, A. C. Scaling theory of entanglement at the many-body localization transition. Phys. Rev. Lett. 119, 110604 (2017).
Goremykina, A., Vasseur, R. & Serbyn, M. Analytically solvable renormalization group for the many-body localization transition. Preprint at https://arxiv.org/abs/1807.04285 (2018).
Lüschen, H. P. et al. Observation of slow dynamics near the many-body local- ization transition in one-dimensional quasiperiodic systems. Phys. Rev. Lett. 119, 260401 (2017).
Bordia, P. et al. Probing slow relaxation and many-body localization in two-dimensional quasiperiodic systems. Phys. Rev. X 7, 041047 (2017).
Luitz, D. J., Laflorencie, N. & Alet, F. Extended slow dynamical regime close to the many-body localization transition. Phys. Rev. B 93, 060201 (2016).
Nandkishore, R., Gopalakrishnan, S. & Huse, D. A. Spectral features of a many-body-localized system weakly coupled to a bath. Phys. Rev. B 90, 064203 (2014).
Lüschen, H. P. et al. Signatures of many-body localization in a controlled open quantum system. Phys. Rev. X 7, 011034 (2017).
De Roeck, W. & Huveneers, F. Stability and instability towards delocalization in many-body localization systems. Phys. Rev. B 95, 155129 (2017).
Nandkishore, R. & Gopalakrishnan, S. Many body localized systems weakly cou-pled to baths. Ann. Phys. 529, 1600181 (2017).
Agarwal, K. et al. Rare-region effects and dynamics near the many-body localization transition. Ann. Phys. 529, 1600326 (2017).
Lucioni, E. et al. Observation of subdiffusion in a disordered interacting system. Phys. Rev. Lett. 106, 230403 (2011).
Liu, H.-C. High-order correlation of chaotic bosons and fermions. Phys. Rev. A 94, 023827 (2016).
Schweigler, T. et al. Experimental characterization of a many-body system via higher-order correlations. Nature 545, 323–326 (2017).
Hodgman, S. S., Khakimov, R. I., Truscott, A. G. & Kheruntsyan, K. V. Solving the quantum many-body problem via correlations measured with a momentum microscope. Phys. Rev. Lett. 118, 240402 (2017).
Grover, T. Certain general constraints on the many-body localization transition. Preprint at https://arxiv.org/abs/1405.1471 (2014).
Acknowledgements
We acknowledge discussions with D. Abanin, E. Altman, H. Bernien, C. Chiu, S. Choi, E. Demler, A. Hébert, W. W. Ho, V. Kasper, V. Khemani, J. Kwan, L. Santos and J. Schmiedmayer. We were supported by grants from the National Science Foundation, the Gordon and Betty Moore Foundations EPiQS Initiative, an Air Force Office of Scientific Research MURI programme, an Army Research Office MURI programme and the NSF Graduate Research Fellowship Program. J.L. acknowledges support from the Swiss National Science Foundation.
Author information
Authors and Affiliations
Contributions
All authors contributed extensively to the construction of the experiment, the collection and analysis of the data, and the writing of the manuscript. M.G. supervised the work.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Peer review information Nature thanks Maksym Serbyn and Jean-Philippe Brantut for their contribution to the peer review of this work.
Supplementary information
Supplementary Information
Supplementary Sections 1–9, including Supplementary Figs. 1–7 and Supplementary Table 1.
Rights and permissions
About this article
Cite this article
Rispoli, M., Lukin, A., Schittko, R. et al. Quantum critical behaviour at the many-body localization transition. Nature 573, 385–389 (2019). https://doi.org/10.1038/s41586-019-1527-2
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41586-019-1527-2
This article is cited by
-
Eigenstate properties of the disordered Bose–Hubbard chain
Frontiers of Physics (2024)
-
Probing the onset of quantum avalanches in a many-body localized system
Nature Physics (2023)
-
Many-body quantum chaos in stroboscopically-driven cold atoms
Communications Physics (2023)
-
Property of Many-Body Localization in Heisenberg Ising Chain Under Periodic Driving
International Journal of Theoretical Physics (2023)
-
Properties of many-body localization in quasi-disordered Haldane–Shastry model
Quantum Information Processing (2023)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.