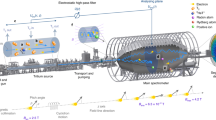
Abstract
About 99 per cent of solar energy is produced through sequences of nuclear reactions that convert hydrogen into helium, starting from the fusion of two protons (the pp chain). The neutrinos emitted by five of these reactions represent a unique probe of the Sun’s internal working and, at the same time, offer an intense natural neutrino beam for fundamental physics. Here we report a complete study of the pp chain. We measure the neutrino–electron elastic-scattering rates for neutrinos produced by four reactions of the chain: the initial proton–proton fusion, the electron-capture decay of beryllium-7, the three-body proton–electron–proton (pep) fusion, here measured with the highest precision so far achieved, and the boron-8 beta decay, measured with the lowest energy threshold. We also set a limit on the neutrino flux produced by the 3He–proton fusion (hep). These measurements provide a direct determination of the relative intensity of the two primary terminations of the pp chain (pp-I and pp-II) and an indication that the temperature profile in the Sun is more compatible with solar models that assume high surface metallicity. We also determine the survival probability of solar electron neutrinos at different energies, thus probing simultaneously and with high precision the neutrino flavour-conversion paradigm, both in vacuum and in matter-dominated regimes.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
Data availability
The datasets generated during the current study are freely available in the repository https://bxopen.lngs.infn.it/. Additional information is available from the Borexino Collaboration spokesperson (spokesperson-borex@lngs.infn.it) upon reasonable request.
References
Atkinson, R. & Houtermans, F. Zur Frage der Aufbaumöglichkeit der Elemente in Sternen. Z. Phys. 54, 656 (1929).
von Weizsäcker, C. F. Über Elementumwandlungen im Innern der Sterne I. Phys. Z. 38, 176 (1937).
Bethe, H. A. & Critchfield, C. L. The formation of deuterons by proton combination. Phys. Rev. 54, 248 (1938).
Bethe, H. Energy production in stars. Phys. Rev. 55, 434 (1939).
Bahcall, J. N. How the Sun Shines. https://www.nobelprize.org/nobel_prizes/themes/physics/fusion/ (Nobel Media, Stockholm, 2000).
Fowler, W. Experimental and theoretical nuclear astrophysics; the quest for the origin of the elements: Nobel prize lecture. Rev. Mod. Phys. 56, 149 (1984).
Davis, R. Nobel lecture: a half-century with solar neutrinos. Rev. Mod. Phys. 75, 985 (2003).
Abdurashitov, J. et al. Results from SAGE (the Russian-American gallium solar neutrino experiment). Phys. Lett. B 328, 234 (1994).
Anselmann, P. et al. Solar neutrinos observed by GALLEX at Gran Sasso. Phys. Lett. B 285, 376 (1992).
Hirata, K. et al. Observation of 8B solar neutrinos in the Kamiokande-II detector. Phys. Rev. Lett. 63, 16 (1989).
Ahmad, Q. et al. Direct evidence for neutrino flavor transformation from neutral-current interactions in the Sudbury Neutrino Observatory. Phys. Rev. Lett. 89, 011301 (2002).
Pontecorvo, B. Neutrino experiments and the problem of conservation of leptonic charge. Zh. Eksp. Teor. Fiz. 53, 1717 (1967).
Wolfenstein, L. Neutrino oscillations in matter. Phys. Rev. D 17, 2369 (1978).
Mikheyev, S. & Smirnov, A. Resonant amplification of neutrino oscillations in matter and spectroscopy of solar neutrinos. Sov. J. Nucl. Phys. 42, 913 (1985).
Bahcall, J. & Davis, R. The evolution of neutrino astronomy. Publ. Astron. Soc. Pacif. 112, 429 (2000).
Haxton, W., Hamish Robertson, R. & Serenelli, A. Solar neutrinos: status and prospects. Annu. Rev. Astron. Astrophys. 51, 21 (2013).
Bahcall, J. N. Neutrino Astrophysics (Cambridge Univ. Press, Cambridge, 1989).
Vinyoles, N. et al. A new generation of standard solar models. Astrophys. J. 835, 202 (2017).
Esteban, I. et al. Updated fit to three neutrino mixing: exploring the accelerator-reactor complementarity. J. High Energy Phys. 1701, 087 (2017).
Arpesella, C. et al. First real time detection of 7Be solar neutrinos by Borexino. Phys. Lett. B 658, 101 (2008).
Arpesella, C. et al. Direct measurement of the 7Be solar neutrino flux with 192 days of Borexino data. Phys. Rev. Lett. 101, 091302 (2008).
Bellini, G. et al. Precision measurement of the 7Be solar neutrino interaction rate in Borexino. Phys. Rev. Lett. 107, 141302 (2011).
Bellini, G. et al. First evidence of pep solar neutrinos by direct detection in Borexino. Phys. Rev. Lett. 108, 051302 (2012).
Bellini, G. et al. Measurement of the solar 8B neutrino rate with a liquid scintillator target and 3 MeV energy threshold in the Borexino detector. Phys. Rev. D 82, 033006 (2010).
Borexino Collaboration. Neutrinos from the primary proton-proton fusion process in the Sun. Nature 512, 383 (2014).
Alimonti, G. et al. The Borexino detector at the Laboratori Nazionali del Gran Sasso. Nucl. Instrum. Meth. A 600, 568 (2009).
Bellini, G. et al. Final results of Borexino Phase I on low-energy solar neutrino spectroscopy. Phys. Rev. D 89, 112007 (2014).
Back, H. et al. Borexino calibrations: hardware, methods and results. J. Instrum. 7, P10018 (2012).
Agostini, M. et al. The Monte Carlo simulation of the Borexino detector. Astropart. Phys. 97, 136 (2018).
Bellini, G. et al. Muon and cosmogenic neutron detection in Borexino. J. Instrum. 6, P05005 (2012).
Abe, K. et al. Solar neutrino measurements in Super-Kamiokande-IV. Phys. Rev. D 94, 052010 (2016).
Aharmim, B. et al. Combined analysis of all three phases of solar neutrino data from the Sudbury Neutrino Observatory. Phys. Rev. C 88, 025501 (2013).
Bergström, J. et al. Updated determination of the solar neutrino fluxes from solar neutrino data. J. High Energy Phys. 2016, 132 (2016).
Chapman, G. A. in Encyclopedia of Planetary Science and Encyclopedia of Earth Science 748 (Springer, 1997).
Fröhlich, C. & Lean, J. The Sun’s total irradiance: cycles, trends and related climate change uncertainties since 1976. Geophys. Res. Lett. 25, 4377 (1998).
Bahcall, J. & Pena-Garay, C. A road map to solar neutrino fluxes, neutrino oscillation parameters and tests for new physics. J. High Energy Phys. 2003, 4 (2003).
Caldwell, A., Kollar, D., Kroninger, K. BAT—the Bayesian Analysis Toolkit. Comput. Phys. Commun. 180, 2197 (2009).
Feng Pen An et al. Measurement of electron antineutrinon oscillation based on 1230 days of operation of the Daya Bay experiment. Phys. Rev. D 95, 072006 (2017).
Gando, A. et al. Reactor on-off antineutrino measurement with KamLAND. Phys. Rev. D 88, 033001 (2013).
Holmgren, H. & Johnston, R. He3(α,γ)Li7 and He3(α,γ)Be7 reactions. Phys. Rev. 113, 1556 (1959).
Asplund, M., Grevesse, N., Sauval, A. J. & Scott, P. The chemical composition of the Sun. Annu. Rev. Astron. Astrophys. 47, 481 (2009).
Caffau, E., Ludwig, H. G., Steffen, M., Freytag, B. & Bonifacio, P. Solar chemical abundances determined with a CO5BOLD 3D model atmosphere. Sol. Phys. 268, 255 (2011).
Asplund, M., Grevesse, N. & Sauval, A. J. The Solar Chemical Composition. (eds Barnes, T. G. & Bash, F. N.) Astronomical Society of the Pacific Conference Series 336, 25 (ASP, 2005).
Grevesse, N. & Sauval, A. J. Standard solar compositon. Space Sci. Rev. 85, 161 (1998).
Grevesse, N. & Noels, A. in Origin and Evolution of the Elements (eds Prantzos, N., Vangioni-Flam, E. & Casse, M.) 15 (Cambrige Univ. Press, Cambridge, 1993).
Franco, D., Consolati, G. & Trezzi, D. Positronium signature in organic liquid scintillators for neutrino experiments. Phys. Rev. C 83, 015504 (2011).
Geant4. A simulation toolkit. https://geant4.web.cern.ch/ (2018).
Bahcall, J. N. & Pena-Garay, C. Solar models and solar neutrino oscillations. New J. Phys. 6, 63 (2004).
Blennow, M. & Coloma, P. Quantifying the sensitivity of oscillation experiments to the neutrino mass ordering. J. High Energy Phys. 03, 028 (2013).
Acknowledgements
The Borexino programme is made possible by funding from INFN (Italy), NSF (USA), BMBF, DFG, HGF and MPG (Germany), RFBR (grants 16-29-13014ofi-m and 17-02-00305A), RSF (grant 17-12-01009) (Russia), and NCN (grant number UMO 2017/26/M/ST2/00915) (Poland). We acknowledge also the computing services of the Bologna INFN-CNAF data centre and LNGS Computing and Network Service (Italy), of Jülich Supercomputing Centre at FZJ (Germany), and of ACK Cyfronet AGH Cracow (Poland). We acknowledge the hospitality and support of the Laboratori Nazionali del Gran Sasso (Italy).
Reviewer information
Nature thanks A. Serenelli and the other anonymous reviewer(s) for their contribution to the peer review of this work.
Author information
Authors and Affiliations
Consortia
Contributions
The Borexino detector was designed, constructed, and commissioned by the Borexino Collaboration over the span of more than 15 years. The Borexino Collaboration sets the science goals. Scintillator purification and handling, source calibration campaigns, photomultiplier tube and electronics operations, signal processing and data acquisition, Monte Carlo simulations of the detector, and data analyses were performed by Borexino members, who also discussed and approved the scientific results. This manuscript was prepared by a subgroup of authors appointed by the Collaboration and subjected to an internal collaboration-wide review process. All authors reviewed and approved the final version of the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Extended data figures and tables
Extended Data Fig. 1 The Borexino detector.
Schematic view of the ‘onion-like’ structure of the Borexino apparatus. From outside to inside: the external water tank; the Stainless Steel Sphere, where about 2,200 photomultiplier tubes (PMTs) are mounted; the outermost nylon vessel, which serves as a barrier against radon; the innermost nylon vessel, which contains 300 t of liquid scintillator, the active detection medium.
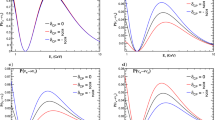
Extended Data Fig. 2 Frequentist hypothesis test of MSW-LMA versus vacuum-LMA.
The probability distribution of the test statistics t is obtained by simulating thousands of sets of Pee values (at the pp, 7Be, pep and 8B energies) in the MSW-LMA hypothesis (red curve on the left) and in the vacuum-LMA hypothesis (blue curve on the right). The dotted black line corresponds to the results of Borexino discussed in the main text.
Extended Data Fig. 3 Frequentist hypothesis test for LZ and HZ.
The probability distribution of the test statistics t is obtained by simulating thousands of fake sets of 8B–7Be values in the HZ hypothesis (red curve on the left) and in the LZ hypothesis (blue curve on the right). The dotted black line corresponds to the results of Borexino discussed in the main text.
Rights and permissions
About this article
Cite this article
The Borexino Collaboration. Comprehensive measurement of pp-chain solar neutrinos. Nature 562, 505–510 (2018). https://doi.org/10.1038/s41586-018-0624-y
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41586-018-0624-y
Keywords
This article is cited by
-
Discrimination of pp solar neutrinos and 14C double pile-up events in a large-scale LS detector
Nuclear Science and Techniques (2023)
-
Solar parameters in long-baseline accelerator neutrino oscillations
Journal of High Energy Physics (2023)
-
Non-standard neutrino interactions in light mediator models at reactor experiments
Journal of High Energy Physics (2023)
-
Testing non-standard neutrino interactions in (anti)-electron neutrino disappearance experiments
Journal of High Energy Physics (2023)
-
Towards resolving the gallium anomaly
Journal of High Energy Physics (2023)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.