Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

Hundred-fold enhancement in far-field radiative heat transfer over the blackbody limit

Nature volume 561, pages 216–221 (2018)Cite this article

Subjects

An Author Correction to this article was published on 05 March 2019

This article has been updated

Abstract

Radiative heat transfer (RHT) has a central role in entropy generation and energy transfer at length scales ranging from nanometres to light years1. The blackbody limit2, as established in Max Planck’s theory of RHT, provides a convenient metric for quantifying rates of RHT because it represents the maximum possible rate of RHT between macroscopic objects in the far field—that is, at separations greater than Wien’s wavelength3. Recent experimental work has verified the feasibility of overcoming the blackbody limit in the near field4,5,6,7, but heat-transfer rates exceeding the blackbody limit have not previously been demonstrated in the far field. Here we use custom-fabricated calorimetric nanostructures with embedded thermometers to show that RHT between planar membranes with sub-wavelength dimensions can exceed the blackbody limit in the far field by more than two orders of magnitude. The heat-transfer rates that we observe are in good agreement with calculations based on fluctuational electrodynamics. These findings may be directly relevant to various fields, such as energy conversion, atmospheric sciences and astrophysics, in which RHT is important.

This is a preview of subscription content, access via your institution

Relevant articles

Open Access articles citing this article.

Access options

Rent or buy this article

Get just this article for as long as you need it

$39.95

Learn more

Prices may be subject to local taxes which are calculated during checkout

Fig. 1: Probing RHT between sub-wavelength structures.
Fig. 2: Radiative conductance between membranes with sub-wavelength dimensions.
Fig. 3: Comparison of experimental data with FED-based simulations.
Fig. 4: Analysis of the observed violations of the blackbody limit.

Data availability

The data that support the findings of this study are available from the corresponding authors on reasonable request.

Change history

  • 05 March 2019

    We would like to correct the sentence in our Letter “However, both our detailed computational analysis (see Supplementary Fig. 2) and past computations13–15 suggest that such enhancements are not anticipated for metallic spheres, and very small increases (by a factor of a few) may be expected for dielectric spheres or metallic cylinders.” The work of ref. 13 is not limited to the structures described in this statement but also presents a computational study of radiative heat transfer between rectangular dielectric membranes that is consistent with our experimental and computational analysis, and supports our findings that the blackbody limit can be overcome in the far-field. See accompanying Amendment. The original Letter has not been corrected online.

References

  1. Chandrasekhar, S. Radiative Transfer (Clarendon Press, Oxford, 1950).

    Google Scholar 

  2. Planck, M. The Theory of Heat Radiation [transl. Masius, M.] (P. Blakiston’s Son & Co., Philadelphia, 1914).

    Google Scholar 

  3. Modest, M. F. Radiative Heat Transfer 3rd edn (Academic Press, New York, 2013).

    Google Scholar 

  4. Kim, K. et al. Radiative heat transfer in the extreme near field. Nature 528, 387–391 (2015).

    CAS  Google Scholar 

  5. Song, B. et al. Radiative heat conductances between dielectric and metallic parallel plates with nanoscale gaps. Nat. Nanotechnol. 11, 509–514 (2016).

    CAS  Google Scholar 

  6. Shen, S., Narayanaswamy, A. & Chen, G. Surface phonon polaritons mediated energy transfer between nanoscale gaps. Nano Lett. 9, 2909–2913 (2009).

    CAS  Google Scholar 

  7. Rousseau, E. et al. Radiative heat transfer at the nanoscale. Nat. Photon. 3, 514–517 (2009).

    CAS  Google Scholar 

  8. Reipurth, B., Jewitt, D. & Keil, K. (eds) Protostars and Planets V (Univ. Arizona Press, Tucson, 2007).

  9. Raman, A. P., Anoma, M. A., Zhu, L., Rephaeli, E. & Fan, S. Passive radiative cooling below ambient air temperature under direct sunlight. Nature 515, 540–544 (2014).

    CAS  Google Scholar 

  10. Guler, U., Boltasseva, A. & Shalaev, V. M. Refractory plasmonics. Science 344, 263–264 (2014).

    CAS  Google Scholar 

  11. Ben-Abdallah, P. & Biehs, S.-A. Near-field thermal transistor. Phys. Rev. Lett. 112, 044301 (2014).

    Google Scholar 

  12. Otey, C. R., Lau, W. T. & Fan, S. Thermal rectification through vacuum. Phys. Rev. Lett. 104, 154301 (2010).

    Google Scholar 

  13. Fernández-Hurtado, V., Fernández-Domínguez, A. I., Feist, J., García-Vidal, F. J. & Cuevas, J. C. Super-Planckian far-field radiative heat transfer. Phys. Rev. B 97, 045408 (2018).

    Google Scholar 

  14. Golyk, V. A., Krüger, M. & Kardar, M. Heat radiation from long cylindrical objects. Phys. Rev. E 85, 046603 (2012).

    Google Scholar 

  15. Kattawar, G. & Eisner, M. Radiation from a homogeneous isothermal sphere. Appl. Opt. 9, 2685–2690 (1970).

    CAS  Google Scholar 

  16. Biehs, S.-A. & Ben-Abdallah, P. Revisiting super-Planckian thermal emission in the far-field regime. Phys. Rev. B 93, 165405 (2016).

    Google Scholar 

  17. Au, Y.-Y., Skulason, H. S., Ingvarsson, S., Klein, L. J. & Hamann, H. F. Thermal radiation spectra of individual subwavelength microheaters. Phys. Rev. B 78, 085402 (2008).

    Google Scholar 

  18. Schuller, J. A., Taubner, T. & Brongersma, M. L. Optical antenna thermal emitters. Nat. Photon. 3, 658–661 (2009).

    CAS  Google Scholar 

  19. Wuttke, C. & Rauschenbeutel, A. Thermalization via heat radiation of an individual object thinner than the thermal wavelength. Phys. Rev. Lett. 111, 024301 (2013).

    CAS  Google Scholar 

  20. Hochbaum, A. I. et al. Enhanced thermoelectric performance of rough silicon nanowires. Nature 451, 163–167 (2008).

    CAS  Google Scholar 

  21. Kim, P., Shi, L., Majumdar, A. & McEuen, P. L. Thermal transport measurements of individual multiwalled nanotubes. Phys. Rev. Lett. 87, 215502 (2001).

    CAS  Google Scholar 

  22. Sadat, S. et al. Room temperature picowatt-resolution calorimetry. Appl. Phys. Lett. 99, 043106 (2011).

    Google Scholar 

  23. Sadat, S., Meyhofer, E. & Reddy, P. Resistance thermometry-based picowatt-resolution heat-flow calorimeter. Appl. Phys. Lett. 102, 163110 (2013).

    Google Scholar 

  24. St-Gelais, R., Zhu, L. X., Fan, S. H. & Lipson, M. Near-field radiative heat transfer between parallel structures in the deep subwavelength regime. Nat. Nanotechnol. 11, 515–519 (2016).

    CAS  Google Scholar 

  25. Polder, D. & Van Hove, M. Theory of radiative heat transfer between closely spaced bodies. Phys. Rev. B 4, 3303–3314 (1971).

    Google Scholar 

  26. Rytov, S. M., Kravtsov, I. A. & Tatarskii, V. I. Principles of Statistical Radiophysics 2nd edn (Springer, Berlin, 1987).

    Google Scholar 

  27. Otey, C. R., Zhu, L., Sandhu, S. & Fan, S. Fluctuational electrodynamics calculations of near-field heat transfer in non-planar geometries: a brief overview. J. Quant. Spectrosc. Radiat. Transf. 132, 3–11 (2014).

    CAS  Google Scholar 

  28. Rodriguez, A. W., Reid, M. H. & Johnson, S. G. Fluctuating-surface-current formulation of radiative heat transfer for arbitrary geometries. Phys. Rev. B 86, 220302 (2012).

    Google Scholar 

  29. Cataldo, G. et al. Infrared dielectric properties of low-stress silicon nitride. Opt. Lett. 37, 4200–4202 (2012).

    CAS  Google Scholar 

  30. Reid, M. T. H. & Johnson, S. G. Efficient computation of power, force and torque in BEM scattering calculations. IEEE Trans. Antenn. Propag. 63, 3588–3598 (2015).

    Google Scholar 

  31. Goody, R. M. & Yung, Y. L. Atmospheric Radiation: Theoretical Basis 2nd edn (Oxford Univ. Press, Oxford, 1989).

    Google Scholar 

  32. Draine, B. & Weingartner, J. C. Radiative torques on interstellar grains: I. Superthermal spinup. Astrophys. J. 470, 551–565 (1996).

    CAS  Google Scholar 

  33. Sultan, R., Avery, A., Stiehl, G. & Zink, B. Thermal conductivity of micromachined low-stress silicon-nitride beams from 77 to 325 K. J. Appl. Phys. 105, 043501 (2009).

    Google Scholar 

  34. Lee, S.-M. & Cahill, D. G. Heat transport in thin dielectric films. J. Appl. Phys. 81, 2590–2595 (1997).

    CAS  Google Scholar 

  35. Kennard, E. H. Kinetic Theory of Gases, with an Introduction to Statistical Mechanics (McGraw-Hill, New York, 1938).

  36. Horowitz, P. & Hill, W. The Art of Electronics (Cambridge Univ. Press, Cambridge, 1989).

    Google Scholar 

Download references

Acknowledgements

P.R. and E.M. acknowledge support from the Office of Naval Research under award number N00014-16-1-2672 (device fabrication), from the Army Research Office under award number W911NF-18-1-0004 (computational modelling) and from Department of Energy Basic Energy Sciences under award number DE-SC0004871 (instrumentation for radiative measurements). M.M.Q. is grateful for support from the National Science Foundation under award number DMR-1255156 (characterization of dielectric functions). L.Z. thanks C. R. Otey for discussions. We acknowledge the Lurie Nanofabrication Facility for facilitating the fabrication of the devices and the Advanced Research Computing Technology Services at the University of Michigan for computational resources.

Reviewer information

Nature thanks P. Bharadwaj and the other anonymous reviewer(s) for their contribution to the peer review of this work.

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, University of Michigan, Ann Arbor, MI, USA

    Dakotah Thompson, Linxiao Zhu, Rohith Mittapally, Seid Sadat, Pramod Reddy & Edgar Meyhofer

  2. Department of Physics, College of William and Mary, Williamsburg, VA, USA

    Zhen Xing, Patrick McArdle & M. Mumtaz Qazilbash

  3. Department of Materials Science and Engineering, University of Michigan, Ann Arbor, MI, USA

    Pramod Reddy

Authors
  1. Dakotah Thompson
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Linxiao Zhu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Rohith Mittapally
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Seid Sadat
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Zhen Xing
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Patrick McArdle
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. M. Mumtaz Qazilbash
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. Pramod Reddy
    View author publications

    You can also search for this author in PubMed Google Scholar

  9. Edgar Meyhofer
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

P.R. and E.M. conceived the work. D.T., R.M. and S.S. fabricated the devices and performed the RHT experiments. L.Z. performed the calculations. Z.X. and P.M. performed spectroscopic measurements of the dielectric functions of silicon nitride under the guidance of M.M.Q. The manuscript was written by D.T., L.Z., P.R. and E.M. with comments and input from all authors.

Corresponding authors

Correspondence to Pramod Reddy or Edgar Meyhofer.

Ethics declarations

Competing interests

The authors declare no competing interests.

Additional information

Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Extended data figures and tables

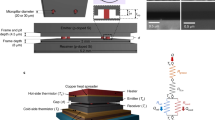
Extended Data Fig. 1 Fabrication of the devices.

a, Step 1: deposition of the silicon nitride (SiN) layer on a silicon handle wafer. Step 2: patterning of the Pt serpentine resistance thermometer. Step 3: patterning of gold leads. Step 4: front-side etching of suspended device contour. Step 5: etching of a window in SiN on the back side of the device. Step 6: potassium hydroxide (KOH) etching of silicon handle to release the suspended devices. Step 7: optional PECVD of SiN onto suspended 1,998-nm-thick devices to create even thicker membranes (6,712 and 11,405 nm thick). b, c, Scanning electron microscope (b) and optical microscope (c) images showing the geometry of the fabricated devices and relevant dimensions. Au wires (yellow) and suspended membranes (red and blue) were pseudo-coloured.

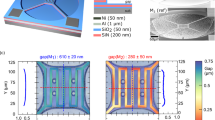
Extended Data Fig. 2 Characterization of the flatness and coplanarity of adjacent membranes.

a–d, Data from laser scanning confocal microscope scans across adjacent membranes showing the flatness of 270-nm-thick (a), 486-nm-thick (b), 6,712-nm-thick (c) and 11,405-nm-thick (d) membranes. Line profiles were taken over the red dashed line indicated in the surface plots in the insets.

Extended Data Fig. 3 Characterization of the electrical and thermal properties of the devices.

a, Temperature dependence of the resistance of the integrated PRT near room temperature. The inset shows a schematic of the measurement scheme used for resistance characterization. b, Thermal frequency response of membrane devices with various thicknesses measured at T = 300 K. The inset depicts a schematic of the measurement scheme employed for these tests. c, Temperature rise of the membrane as a function of the Joule heating in the PRT, measured at T = 300 K for devices of various thicknesses. d, Temperature rise of the receiver membrane as a function of the temperature increase of the emitter membrane for a 2-μm-thick device at 100 K. The noise-equivalent temperature is about 60 μK for a temperature modulation frequency of 0.5 Hz and a measurement bandwidth of 0.78 mHz, and Idc = 10 μA.

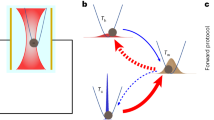
Extended Data Fig. 4 Quantification of parasitic energy transfer mechanisms.

a, Measured Grad,eff between 2-μm-thick SiN membranes at various temperatures and pressures. Measurements performed at 10−3 torr (black squares) and 10−6 torr (red diamonds) give virtually identical results, strongly supporting the expectation that conduction via remaining gas molecules plays no role. b, Measured Grad,eff between 270-nm-thick SiN membranes at various temperatures. The inset illustrates how thermal energy between the devices can potentially couple via conduction through the substrate. The plotted data correspond to two device geometries: devices with 400-μm-long support beams (black squares) and devices with 150-μm-long support beams (red squares). Because devices with short and long beams have identical Grad,eff, it is clear that coupling via the suspending beams is negligibly small. c, Schematic describing the control experiment, where an emitter and a receiver are separated by a gap of about 1 mm and an Al foil is placed between them, blocking direct RHT (not drawn to scale and proportion). d, Measured coupling signal between 2-μm-thick emitter and receiver membranes across a 1-mm-wide gap (T = 300 K). Signals without (black squares) and with (red circles) an Al foil in the gap are shown. The inset (cross-sectional view of the devices) illustrates how the Al foil shield blocks direct RHT between the membranes but potentially allows RHT via specular reflections. Because the signal obtained in the presence of the Al blocker represents the noise floor of our measurement, we conclude that there is negligible heat transfer (via RHT or otherwise) in the presence of the Al foil. This control experiment provides unequivocal evidence that energy transfer between the membranes is mediated exclusively by direct radiation. e, Scanning electron microscope image of two emitter and receiver pairs suspended in separate through-holes on the same handle substrate. f, Measured coupling signal between 486-nm-thick devices (T = 100 K). Signals from adjacent emitter and receiver devices (that is, in the same through-hole; see pair 1 in e) with a 20-μm-gap (black squares) are compared with the signals measured when the emitter and receiver devices are suspended in separate through-holes on the same silicon handle substrate (red circles).

Extended Data Fig. 5 Radiative conductance for devices with 486-nm-thick and 6,712-nm-thick membranes.

a, Grad,eff as a function of temperature. Experimental measurements (solid symbols) are compared with values calculated using FED (open symbols) and COMSOL-modelled blackbody values (dashed lines). b, BEM calculation of the normalized spectral radiative conductance at 300 K. The spectral conductance values are normalized by the thickness of each device.

Supplementary information

Supplementary Information

This file contains Supplementary Text, Supplementary Figures 1–20 and Supplementary References

Rights and permissions

Reprints and Permissions

About this article

Verify currency and authenticity via CrossMark

Cite this article

Thompson, D., Zhu, L., Mittapally, R. et al. Hundred-fold enhancement in far-field radiative heat transfer over the blackbody limit. Nature 561, 216–221 (2018). https://doi.org/10.1038/s41586-018-0480-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/s41586-018-0480-9

Keywords

  • Blackbody Limit
  • Thin Devices
  • Thick Device
  • Silicon Handle Wafer
  • Thermal Frequency Response

This article is cited by

Comments

By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing